Permutations and Combinations : Notes and Study Materials -pdf
- Concepts of Permutations and Combinations
- Permutations and Combinations Master File
- Permutations and Combinations Revision Notes
- R D Sharma Solution of Permutations
- R D Sharma Solution of Combinations
- NCERT Solution Permutations and Combinations
- NCERT Exemplar Solution Permutations and Combinations
- Permutations and Combinations: Solved Example 1
CBSE Class 11 Maths Notes Chapter 7 Permutations and Combinations
Fundamental Principles of Counting
Multiplication Principle: Suppose an operation A can be performed in m ways and associated with each way of performing of A, another operation B can be performed in n ways, then total number of performance of two operations in the given order is mxn ways. This can be extended to any finite number of operations.
Addition Principle: If an operation A can be performed in m ways and another operation S, which is independent of A, can be performed in n ways, then A and B can performed in (m + n) ways. This can be extended to any finite number of exclusive events.
Factorial
The continued product of first n natural number is called factorial ‘n’.
It is denoted by n! or n! = n(n – 1)(n – 2)… 3 × 2 × 1 and 0! = 1! = 1
Permutation
Each of the different arrangement which can be made by taking some or all of a number of objects is called permutation.
Permutation of n different objects
The number of arranging of n objects taking all at a time, denoted by nPn, is given by nPn = n!
The number of an arrangement of n objects taken r at a time, where 0 < r ≤ n, denoted by nPr is given by
nPr = n!(n−r)!
Properties of Permutation
Important Results on Permutation
The number of permutation of n things taken r at a time, when repetition of object is allowed is nr.
The number of permutation of n objects of which p1 are of one kind, p2 are of second kind,… pk are of kth kind such that p1 + p2 + p3 + … + pk = n is n!p1!p2!p3!…..pk!
Number of permutation of n different objects taken r at a time,
When a particular object is to be included in each arrangement is r. n-1Pr-1
When a particular object is always excluded, then number of arrangements = n-1Pr.
Number of permutations of n different objects taken all at a time when m specified objects always come together is m! (n – m + 1)!.
Number of permutation of n different objects taken all at a time when m specified objects never come together is n! – m! (n – m + 1)!.
Combinations
Each of the different selections made by taking some or all of a number of objects irrespective of their arrangements is called combinations. The number of selection of r objects from; the given n objects is denoted by nCr, and is given by
nCr = n!r!(n−r)!
Properties of Combinations
Permutation
What is factorial?
The product of first ‘n’ natural numbers is denoted by $n!$.
$n! = n(n-1) (n-2) ……3.2.1$
Example
$5! = 5 \times 4 \times 3 \times 2 \times 1 =120$
Note $0! =1$
Proof
$n! =n \times (n-1)!$
or $(n-1)! = \frac {n!} {n}$
Putting n = 1, we have
$0! = \frac {1!}{1}$
or $ 0! = 1$
$(n+2)!=2550 \times n!$
Solution
$(n+2)!=2550 \times n!$
$(n+2)(n+1)n!=2550 \times n!$
Canceling n!
$(n+2)(n+1)=2550$
$n=49$
Fundamental Principle of counting
Addition rule
: If an job can be performed in ‘n’ ways, & another job can be performed in ‘m’ ways then either of the two jobs can be performed in (m+n) ways. This rule can be extended to any finite number of jobs.
Example
In a class 12 , 20 students are from Maths streams while 30 students are from Biology stream.
Teacher has to choose 1 student either from Maths stream or Biology stream to represent the class
What ways the selection can be made?
Solution:
20 students are from Maths:
Students can be chosen from Maths stream in 20 ways
30 students are from Biology stream
Students can be chosen from Biology stream in 30 ways
So by the Fundamental Principle of addition rule, the selection of 1 student from either of two streams can be done (20+30)=50 ways
Multiplication Rule
: If a work can be done in m ways, another work can be done in ‘n’ ways, then both of the operations can be performed in $m \times n$ ways. It can be extended to any finite number of operations
Example:
In a class 12 , 20 students are from Maths streams while 30 students are from Biology stream.
Teacher has to choose 2 student one from Maths stream and one from Biology stream to represent the class in Quiz competition
What ways the selection can be made?
Solution:
20 students are from Maths:
1 Students can be chosen from Maths stream in 20 ways
30 students are from Biology stream
1Students can be chosen from Biology stream in 30 ways
So by the Fundamental Principle of Multiplication rule, the selection of 1 student from Maths streams and 1 students from Biology stream can be done $(20 \times 30)=600$ ways
Permutation
Permutation means arrangement of things. The word arrangement is used, if the order of things is considered
Theorem 1
Number of permutations of ‘n’ different things taken ‘r’ at a time is given by:-
$^{n}P_r=\frac { n!}{ (n-r)!}$
Proof:
Say we have ‘n’ different things $a_1 , a_2, … , a_n$
- Clearly the first place can be filled up in ‘n’ ways. Number of things left after filling-up the first place = $n-1$
- So the second-place can be filled-up in $(n-1)$ ways. Now number of things left after filling-up the first and second places = $n – 2$
- Now the third place can be filled-up in $(n-2)$ ways. Thus number of ways of filling-up first-place = $n$
Number of ways of filling-up second-place = $n-1$
Number of ways of filling-up third-place = $n-2$
Number of ways of filling-up r-th place = $n – (r-1) = n-r+1$
By multiplication – rule of counting, total no. of ways of filling up, first, second — rth-place together :-
$n (n-1) (n-2)….. (n-r+1)$
Hence:
$^{n}P_r = n (n-1)(n-2)…..(n-r+1)$
$= \frac {[n(n-1)(n-2)…(n-r+1)] [(n-r)(n-r-1)…3.2.1.]}{ [(n-r)(n-r-1)…3.2.1]}$
$^{n}P_r=\frac { n!}{ (n-r)!}$
Theorem 2:
Number of permutations of ‘n’ different things taken all at a time is given by:-
$^{n}P_n= n!$
Proof
Now we have ‘n’ objects, and n-places.
Number of ways of filling-up first-place = $n$
Number of ways of filling-up second-place = $n-1$
Number of ways of filling-up third-place = $n-2$
Number of ways of filling-up r-th place, i.e. last place =1
Number of ways of filling-up first, second, — n th place
$= n (n-1) (n-2) …. 2.1.$
$^{n}P_n= n!$
To prove
$0! = 1$
We have $^{n}P_r=\frac { n!}{ (n-r)!}$
Putting r = n, we have :-
$^{n}P_n =\frac { n!}{ (n-n)!}= \frac {n!}{0!}$
But $^{n}P_n= n!$
Clearly it is possible, only when $0! = 1$
Hence it is proof that $0! = 1$
Example
How many different signals can be made by 4 flags from 6-flags of different colours?
Solution :
Number of ways taking 4 flags out of 6-flags = $^{6}P_4=\frac { 6!}{ (6-4)!}$
$=6 \times 5 \times 4 \times 3 = 360$
Example
How many words can be made by using the letters of the word “ZEBRA” taken all at a time?
Solution
There are ‘5’ different letters of the word “ZEBRA”
Number of Permutations taking all the letters at a time = $^{n}P_n$
$=5! = 5 \times 4 \times 3 \times 2 \times 1=120$
Restricted – Permutations
Theorem -1
Number of permutations of ‘n’ things, taken ‘r’ at a time, when a particular thing is to be always included in each arrangement
$r ^{n}P_{r-1}$
Proof:
When one particular thing is always taken, then that can arranged in r ways
Now we have to arrange the remaining $r-1$ places with $n-1$ objects,so permutations will be $^{n-1}P_{r-1}$ . Now by principle of counting ,both of these can be arrange in $r ^{n}P_{r-1}$
Theorem – 2
Number of permutations of ‘n’ things, taken ‘r’ at a time, when a particular thing is fixed:
$= ^{n-1}P_{r-1}$
Proof:
When one particular thing is fixed ,then that is the only arrangement for that.Now we have to arrange the remaining $r-1$ places with $n-1$ objects,so permutations will be $^{n-1}P_{r-1}$ . Now by principle of counting ,both of these can be arrange in $^{n-1}P_{r-1}$
Theorem -3
Number of permutations of ‘n’ things, taken ‘r’ at a time, when a particular thing is never taken
$= ^{n-1}P_{r}$
Proof:
When one particular thing is never taken then we have to arrange the r- places with n-1 objects,so permutations will be $= ^{n-1}P_{r}$
Another Theorem of Permutation
Theorem
There are n subjects of which $p_1$ are alike of one kind; $p_2$ are alike of another kind; $p_3$ are alike of third kind and so on and pr are alike of rth kind,
such that $(p_1 + p_2 + … p_r) = n$.
Then, number of permutations of these n objects is =$\frac {n!}{p_1! p_2!….p_r!}$
Solved Examples
Question-1
How many words can be formed with the letters of the word ‘TIGER’ when:
- T’ and ‘R’ occupying end places.
- G’ being always in the middle
- Vowels occupying odd-places
- Vowels being never together.
- Find the number of words formed with out any restriction
Solution
- When ‘T’ and ‘R’ occupying end-places
IGE. (TR)
Here (TR) are fixed, hence I,E,G can be arranged in 3! ways
But (T,R) can be arranged themselves is 2! ways.
Total number of words =$3! \times 2! = 12$ ways. - When ‘G’ is fixed in the middle
T.I.(G), E.R
Hence four-letter T.I.E.R. can be arranged in 4! i.e 24 ways. - Two vowels (I,E) can be arranged in the odd-places (1st, 3rd and 5th) = 3! ways.
And three consonants (T,G,R) can be arranged in the remaining places = 3 ! ways
Total number of ways= $3! \times 3! = 36$ ways. - Total number of words = 5! = 120
If all the vowels come together, then we have: (I.E.), T,R,G
These can be arranged in 4! ways.
But (I,E.) can be arranged themselves in 2! ways.
Number of ways, when vowels come-together = $4! \times 2!= 48$ ways
Number of ways, when vowels being never-together
$= 120-48 =72$ ways. - Total number of words = 5! = 120
Question-2
Find the numbers of words formed by permuting all the letters of the following words
- INDIA
- RUSSIA
- AUGUST
- ARRANGE
Solution
- In INDIA, There are 2 I’s and all other are distinct
So Number of words formed= $\frac {n!}{p_1! p_2!…. p_r!}$
$=\frac {5!}{2!} =60$ words - In RUSSIA, There are 2 S’s and all other are distinct
So Number of words formed= $\frac {n!}{p_1! p_2!…. p_r!}$
$ =\frac {6!}{2!} =360$ words - In AUGUST, There are 2 U’s and all other are distinct
So Number of words formed= $\frac {n!}{p_1! p_2!…. p_r!}$
$ =\frac {6!}{2!} =360$ words
4) In ARRANGE, There are 2 R’s and 2A’s and all other are distinct
So Number of words formed= $\frac {n!}{p_1! p_2!…. p_r!}$
$ =\frac {7!}{2! \times 2!}= =1260$ words
Introduction to Combination:
- We have studied about arrangement i.e permutation in the previous chapter
- We learnt that arrangement of r objects taken from n objects is given by
$^{n}P_{r}=\frac {n!}{(n-r)!}$
We would be discussing the combination in this chapter - Combination is defined as the different selection made by taking some objects out of many objects irrespective of their arrangement
So arrangement does not matter in Combination while it matters in Permutation
Example
We have three letter XYZ,
We have to select two letters and arrange it
Different arrangement would be
XY YZ ZX YX,ZY,XZ
So this is permuatation
Now if we have to just select two letter and do not worry about the arrangement then
XY, YZ ,ZX
This is combination - Combination generally happens with committee selection problems as it does not matter how your committee is arranged. In other words, it generally does not matter whether you think about your committee as Vijay, Arun and Nita or as Nita, Vijay and Arun. In either case, the same three people are in the committee. Contrast this with permutations examples in previous where arrangement matters
Combination Formula
A formula for the number of possible combinations of $r$ objects from a set of $n$ objects. This is written in any of the ways shown below$^{n}C_{r} =\frac {n!}{r! (n-r)!}$
Proof:
Let $x$ be the combination of $n$ distinct objects taken $r$ at a time.
Now lets us consider one of the $x$ ways. There are $r$ objects in this one way which can be arranged in $r!$ways . Similarly all these $x$ ways can be arranged in this manner
So permutation of the n things taken r at a time will be given as
$^{n}P_{r}=x r!$
or $ x=\frac { n!}{r! (n-r)!}$
$^{n}C_{r} =\frac {n!}{r! (n-r)!}$
Eleven students put their names on slips of paper inside a box. Three names are going to be taken out. How many different ways can the three names be chosen?
Solution
As the arrangement does not matter, so different ways are
$^{n}C_{r} =\frac {n!}{r! (n-r)!}$
$^{11}C_{3} =\frac {11!}{3! (11-3)!}=165$
Difference Between Permutation and Combination
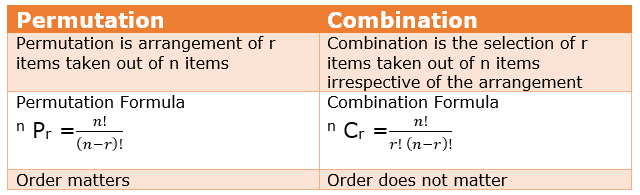
Properties of Combination Formula
$^{n}C_{r} =\frac {n!}{r! (n-r)!}$| 1 | $^{n}C_{n} = 1$ , $^{n}C_{0} = 1$ as $^{n}C_{n} =\frac {n!}{n! (n-n)!}= \frac {1}{0!} =1$ |
| 2 | for $0 \leq r <\leq n$ , we have $^{n}C_{r} = ^{n}C_{n-r}$ As $ ^{n}C_{r} =\frac {n!}{r! (n-r)!}$ $ ^{n}C_{n-r}= \frac {n!}{(n-r)! (n-n+r)!}= \frac {n!}{r! (n-r)!}$ |
| 3 | $ ^{n}C_{x} = ^{n}C_{y}$ Then $x=y$ or $x+y=n$ |
| 4 | $^{n}C_{r}+ ^{n}C_{r-1}= ^{n+1}C_{r}$ This property is called Pascal law’s |
| 5. | for $1 \leq r <\leq n$ , we have $^{n}C_{r} =\frac {n}{r} ^{n-1}C_{r-1}$ |
| 6. | for $1 \leq r <\leq n$, we have $n ^{n-1}C_{r-1} =(n-r+1) ^{n}C_{r-1}$ |
Solved Examples
Question -1$^{2n}C_{3} : ^{n}C_{3} =11 :1$
Find the value of n
Solution:
Writing this in factorial form
$\frac {\frac {2n!}{3! (2n-3)!}}{\frac {n!}{3!(n-3)!}} =\frac {11}{1}$
Simplifying it we get
$\frac {2n(2n-1)(2n-2)}{n(n-1)(n-2)}=\frac {11}{1}$
Solving this
$n=6$
Question-2
Over the weekend, your family is going on vacation, and your mom is letting you bring your favorite video game console as well as five of your games. How many ways can you choose the five games if you have 10 games in all?
Solution
Applying combination formula
$^{n}C_{r} =\frac {n!}{r! (n-r)!}$
$^{10}C_{5}=\frac {10!}{5! (10-5)!}=\frac {10 \times 9 \times 8 \times 6}{5 \times 4 \times 3 \times 2 \times 1}=36$
Question-3
In how many ways can a T20 eleven can be chosen out of batch of 15 players if
- There is no restriction on the selection
- A particular player is always chosen
- A particular player is never chosen
- If 11players to be chosen from 15 ,then $^{15}C_{11}=1365$
- If the particular player is always choosen,then we need to choose 10 players from remaining 14 players $^{14}C_{10} =1001$
- If the particular player is never choosen,then we need to choose 11 players from remaining 14 players $^{14}C_{11} =364$
Permutations and Combinations Class 11 MCQs Questions with Answers
Question 1.
There are 12 points in a plane out of which 5 are collinear. The number of triangles formed by the points as vertices is
(a) 185
(b) 210
(c) 220
(d) 175
Answer
Answer: (b) 210
Hint:
Total number of triangles that can be formed with 12 points (if none of them are collinear)
= 12C3
(this is because we can select any three points and form the triangle if they are not collinear)
With collinear points, we cannot make any triangle (as they are in straight line).
Here 5 points are collinear. Therefore we need to subtract 5C3 triangles from the above count.
Hence, required number of triangles = 12C3 – 5C3 = 220 – 10 = 210
Question 2.
The number of combination of n distinct objects taken r at a time be x is given by
(a) n/2Cr
(b) n/2Cr/2
(c) nCr/2
(d) nCr
Answer
Answer: (d) nCr
Hint:
The number of combination of n distinct objects taken r at a time be x is given by
nCr = n!/{(n – r)! × r!}
Let the number of combination of n distinct objects taken r at a time be x.
Now consider one of these n ways. There are e objects in this selection which can be arranged in r! ways.
So, each of the x combinations gives rise to r! permutations. So, x combinations will give rise to x×(r!).
Consequently, the number of permutations of n things, taken r at a time is x×(r!) and it is equal to nPr
So, x×(r!) = nPr
⇒ x×(r!) = n!/(n – r)!
⇒ x = n!/{(n – r)! × r!}
⇒ nCr = n!/{(n – r)! × r!}
Question 3.
Four dice are rolled. The number of possible outcomes in which at least one dice show 2 is
(a) 1296
(b) 671
(c) 625
(d) 585
Answer
Answer: (b) 671
Hint:
No. of ways in which any number appearing in one dice = 6
No. of ways in which 2 appear in one dice = 1
No. of ways in which 2 does not appear in one dice = 5
There are 4 dice.
Getting 2 in at least one dice = Getting any number in all the 4 dice – Getting not 2 in any of the 4 dice.
= (6×6×6×6) – (5×5×5×5)
= 1296 – 625
= 671
Question 4.
If repetition of the digits is allowed, then the number of even natural numbers having three digits is
(a) 250
(b) 350
(c) 450
(d) 550
Answer
Answer: (c) 450
Hint:
In a 3 digit number, 1st place can be filled in 5 different ways with (0, 2, 4, 6, 8)
10th place can be filled in 10 different ways.
100th place can be filled in 9 different ways.
So, the total number of ways = 5 × 10 × 9 = 450
Question 5.
The number of ways in which 8 distinct toys can be distributed among 5 children is
(a) 58
(b) 85
(c) 8P5
(d) 5P5
Answer
Answer: (a) 58
Hint:
Total number of toys = 8
Total number of children = 5
Now, each toy can be distributed in 5 ways.
So, total number of ways = 5 × 5 × 5 × 5 × 5 × 5 × 5 × 5
= 58
Question 6.
The value of P(n, n – 1) is
(a) n
(b) 2n
(c) n!
(d) 2n!
Answer
Answer: (c) n!
Hint:
Given,
Given, P(n, n – 1)
= n!/{(n – (n – 1)}
= n!/(n – n + 1)}
= n!
So, P(n, n – 1) = n!
Question 7.
In how many ways can 4 different balls be distributed among 5 different boxes when any box can have any number of balls?
(a) 54 – 1
(b) 54
(c) 45 – 1
(d) 45
Answer
Answer: (b) 54
Hint:
Here, both balls and boxes are different.
Now, 1st ball can be placed into any of the 5 boxes.
2nd ball can be placed into any of the 5 boxes.
3rd ball can be placed into any of the 5 boxes.
4th ball can be placed into any of the 5 boxes.
So, the required number of ways = 5 × 5 × 5 × 5 = 54
Question 8.
The number of ways of painting the faces of a cube with six different colors is
(a) 1
(b) 6
(c) 6!
(d) None of these
Answer
Answer: (a) 1
Hint:
Since the number of faces is same as the number of colors,
therefore the number of ways of painting them is 1
Question 9.
Out of 5 apples, 10 mangoes and 13 oranges, any 15 fruits are to be distributed among 2 persons. Then the total number of ways of distribution is
(a) 1800
(b) 1080
(c) 1008
(d) 8001
Answer
Answer: (c) 1008
Hint:
Given there are 5 apples, 10 mangoes and 13 oranges.
Let x1 is for apple, x2 is for mango and x3 is for orange.
Now, first we have to select total 15 fruits out of them.
x1 + x2 + x3 = 15 (where 0 ⇐ x1 ⇐ 5, 0 ⇐ x2 ⇐ 10, 0 ⇐ x3 ⇐ 13)
= (x0 + x1 + x2 +………+ x5)×(x0 + x1 + x2 +………+ x110)×(x0 + x1 + x2 +………+ x13)
= {(1- x6)/(1 – x)}×{(1- x11)/(1 – x)}×{(1- x14)/(1 – x)}
= {(1- x6)×(1- x11)×{(1- x14)}/(1 – x)³
= {(1- x6)×(1- x11)×{(1- x14)} × ∑3+r+1Cr × xr
= {(1- x11 – x6 + x17)×{(1- x14)} × ∑3+r+1Cr × xr
= {(1- x11 – x6 + x17 – x14 + x25 + x20 – x31)} × ∑2+rCr × xr
= 1 × ∑2+rCr × xr – x11 × ∑2+rCr × xr – x6 × ∑2+rCr × xr + x17 × ∑2+rCr × xr – x14 × ∑2+rCr × xr + x25 × ∑2+rCr × xr + x20 × ∑2+rCr × xr – x31 × ∑2+rCr × xr
= ∑2+rCr × xr – ∑2+rCr × xr+11 – ∑2+rCr × xr+6 + ∑2+rCr × xr+17 – ∑2+rCr × xr+14 + ∑2+rCr × xr+25 + ∑2+rCr × xr+20 – ∑2+rCr × xr+25
Now we have to find co-efficeient of x15
= 2+15C15 – 2+4C4 – 2+9C9 – 2+1C1 (rest all terms have greater than x15, so its coefficients are 0)
= 17C15 – 6C4 – 11C9 – 3C1
= 17C2 – 6C2 – 11C2 – 3C1
= {(17×16)/2} – {(6×5)/2} – {(11×10)/2} – 3
= (17×8) – (3×5) – (11×5) – 3
= 136 – 15 – 55 – 3
= 136 – 73
= 63
Again we have to distribute 15 fruits between 2 persons.
So x1 + x2 = 15
= 2-1+15C15
= 16C15
= 16C1
= 16
Now total number of ways of distribution = 16 × 63 = 1008
Question 10.
6 men and 4 women are to be seated in a row so that no two women sit together. The number of ways they can be seated is
(a) 604800
(b) 17280
(c) 120960
(d) 518400
Answer
Answer: (a) 604800
Hint:
6 men can be sit as
× M × M × M × M × M × M ×
Now, there are 7 spaces and 4 women can be sit as 7P4 = 7P3 = 7!/3! = (7 × 6 × 5 × 4 × 3!)/3!
= 7 × 6 × 5 × 4 = 840
Now, total number of arrangement = 6! × 840
= 720 × 840
= 604800
Question 11.
The number of ways can the letters of the word ASSASSINATION be arranged so that all the S are together is
(a) 152100
(b) 1512
(c) 15120
(d) 151200
Answer
Answer: (d) 151200
Hint:
Given word is : ASSASSINATION
Total number of words = 13
Number of A : 3
Number of S : 4
Number of I : 2
Number of N : 2
Number of T : 1
Number of O : 1
Now all S are taken together. So it forms a single letter.
Now total number of words = 10
Now number of ways so that all S are together = 10!/(3!×2!×2!)
= (10×9×8×7×6×5×4×3!)/(3! × 2×2)
= (10×9×8×7×6×5×4)/(2×2)
= 10×9×8×7×6×5
= 151200
So total number of ways = 151200
Question 12.
If repetition of the digits is allowed, then the number of even natural numbers having three digits is
(a) 250
(b) 350
(c) 450
(d) 550
Answer
Answer: (c) 450
Hint:
In a 3 digit number, 1st place can be filled in 5 different ways with (0, 2, 4, 6, 8)
10th place can be filled in 10 different ways.
100th place can be filled in 9 different ways.
So, the total number of ways = 5 × 10 × 9 = 450
Question 13.
Let Tn denote the number of triangles which can be formed using the vertices of a regular polygon on n sides. If Tn+1 – Tn = 21, then n equals
(a) 5
(b) 7
(c) 6
(d) 4
Answer
Answer: (b) 7
Hint:
The number of triangles that can be formed using the vertices of a regular polygon = nC3
Given, Tn+1 – Tn = 21
⇒ n+1C3 – nC3 = 21
⇒ nC2 + nC3 – nC3 = 21 {since n+1Cr = nCr-1 + nCr}
⇒ nC2 = 21
⇒ n(n – 1)/2 = 21
⇒ n(n – 1) = 21×2
⇒ n² – n = 42
⇒ n² – n – 42 = 0
⇒ (n – 7)×(n + 6) = 0
⇒ n = 7, -6
Since n can not be negative,
So, n = 7
Question 14.
How many ways are here to arrange the letters in the word GARDEN with the vowels in alphabetical order?
(a) 120
(b) 240
(c) 360
(d) 480
Answer
Answer: (c) 360
Hint:
Given word is GARDEN.
Total number of ways in which all letters can be arranged in alphabetical order = 6!
There are 2 vowels in the word GARDEN A and E.
So, the total number of ways in which these two vowels can be arranged = 2!
Hence, required number of ways = 6!/2! = 720/2 = 360
Question 15.
How many factors are 25 × 36 × 52 are perfect squares
(a) 24
(b) 12
(c) 16
(d) 22
Answer
Answer: (a) 24
Hint:
Any factors of 25 × 36 × 52 which is a perfect square will be of the form 2a × 3b × 5c
where a can be 0 or 2 or 4, So there are 3 ways
b can be 0 or 2 or 4 or 6, So there are 4 ways
a can be 0 or 2, So there are 2 ways
So, the required number of factors = 3 × 4 × 2 = 24
Question 16.
A student is to answer 10 out of 13 questions in an examination such that he must choose at least 4 from the first five questions. The number of choices available to him is
(a) 40
(b) 196
(c) 280
(d) 346
Answer
Answer: (b) 196
Hint:
There are two cases
1. When 4 is selected from the first 5 and rest 6 from remaining 8
Total arrangement = 5C4 × 8C6
= 5C1 × 8C2
= 5 × (8×7)/(2×1)
= 5 × 4 × 7
= 140
2. When all 5 is selected from the first 5 and rest 5 from remaining 8
Total arrangement = 5C5 × 8C5
= 1 × 8C3
= (8×7×6)/(3×2×1)
= 8×7
= 56
Now, total number of choices available = 140 + 56 = 196
Question 17.
Four dice are rolled. The number of possible outcomes in which at least one dice show 2 is
(a) 1296
(b) 671
(c) 625
(d) 585
Answer
Answer: (b) 671
Hint:
No. of ways in which any number appearing in one dice = 6
No. of ways in which 2 appear in one dice = 1
No. of ways in which 2 does not appear in one dice = 5
There are 4 dice.
Getting 2 in at least one dice = Getting any number in all the 4 dice – Getting not 2 in any of the 4 dice.
= (6×6×6×6) – (5×5×5×5)
= 1296 – 625
= 671
Question 18.
In how many ways in which 8 students can be sated in a line is
(a) 40230
(b) 40320
(c) 5040
(d) 50400
Answer
Answer: (b) 40320
Hint:
The number of ways in which 8 students can be sated in a line = 8P8
= 8!
= 40320
Question 19.
The number of squares that can be formed on a chess board is
(a) 64
(b) 160
(c) 224
(d) 204
Answer
Answer: (d) 204
Hint:
A chess board contains 9 lines horizontal and 9 lines perpendicular to them.
To obtain a square, we select 2 lines from each set lying at equal distance and this equal
distance may be 1, 2, 3, …… 8 units, which will be the length of the corresponding square.
Now, two lines from either set lying at 1 unit distance can be selected in 8C1 = 8 ways.
Hence, the number of squares with 1 unit side = 8²
Similarly, the number of squares with 2, 3, ….. 8 unit side will be 7², 6², …… 1²
Hence, total number of square = 8² + 7² + ……+ 1² = 204
Question 20.
How many 3-letter words with or without meaning, can be formed out of the letters of the word, LOGARITHMS, if repetition of letters is not allowed
(a) 720
(b) 420
(c) none of these
(d) 5040
Answer
Answer: (a) 720
Hint:
The word LOGARITHMS has 10 different letters.
Hence, the number of 3-letter words(with or without meaning) formed by using these letters
= 10P3
= 10 ×9 ×8
= 720
