Question
The set \({{\rm{S}}_1} = \left\{ {2,4,6,8} \right\}\) and \({ \times _{10}}\) denotes multiplication modulo \(10\).
(i) Write down the Cayley table for \(\left\{ {{{\rm{S}}_1},{ \times _{10}}} \right\}\) .
(ii) Show that \(\left\{ {{{\rm{S}}_1},{ \times _{10}}} \right\}\) is a group.
(iii) Show that this group is cyclic.
Now consider the group \(\left\{ {{{\rm{S}}_1},{ \times _{20}}} \right\}\) where \({{\rm{S}}_2} = \left\{ {1,9,11,19} \right\}\) and \({{ \times _{20}}}\) denotes multiplication modulo \(20\). Giving a reason, state whether or not \(\left\{ {{{\rm{S}}_1},{ \times _{10}}} \right\}\) and \(\left\{ {{{\rm{S}}_1},{ \times _{20}}} \right\}\) are isomorphic.
Answer/Explanation
Markscheme
(i)
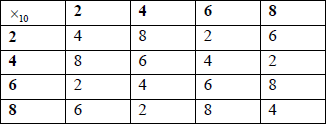 A2
A2
Note: Award A1 for one error.
(ii) closure: it is closed because no new elements are formed A1
identity: \(6\) is the identity element A1
inverses: \(4\) is self-inverse and (\(2\), \(8\)) form an inverse pair A1
associativity: multiplication is associative A1
the four group axioms are satisfied
(iii) any valid reason, e.g.
\(2\) (or \(8\)) has order \(4\), or \(2\) (or \(8\)) is a generator A2
[8 marks]
the groups are not isomorphic A1
any valid reason, e.g. \({{\rm{S}}_2}\) is not cyclic or all its elements are self-inverse R2
[3 marks]
Question
Consider the set \(S = \{ 0,{\text{ }}1,{\text{ }}2,{\text{ }}3,{\text{ }}4,{\text{ }}5\} \) under the operation of addition modulo \(6\), denoted by \({ + _6}\).
Construct the Cayley table for \(\{ S,{\text{ }}{ + _6}\} \).
Show that \(\{ S,{\text{ }}{ + _6}\} \) forms an Abelian group.
State the order of each element.
Explain whether or not the group is cyclic.
Answer/Explanation
Markscheme
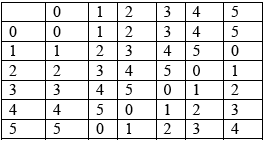 A2
A2
Note: A1 for one or two errors in the table, A0 otherwise.
closed no new elements A1
\(0\) is identity (since \(0 + a = a + 0 = a,{\text{ }}a \in S\)) A1
\(0\), \(3\) self inverse, \(1 \Leftrightarrow 5\) inverse pair, \(2 \Leftrightarrow 4\) inverse pair A1
all elements have an inverse
associativity is assumed over addition A1
since symmetry on leading diagonal in table or commutativity of addition A1
\( \Rightarrow \{ S,{\text{ }}{ + _6}\} \) is an Abelian group AG
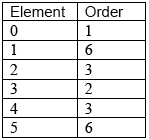 A2
A2
Note: A1 for one or two errors in the table, A0 otherwise.
since there is an element with order \(6\) OR \(1\) or \(5\) are generators R1
the group is cyclic A1
Question
The set \(S\) contains the eight matrices of the form\[\left( {\begin{array}{*{20}{c}}
a&0&0\\
0&b&0\\
0&0&c
\end{array}} \right)\]where \(a\), \(b\), \(c\) can each take one of the values \( + 1\) or \( – 1\) .
Show that any matrix of this form is its own inverse.
Show that \(S\) forms an Abelian group under matrix multiplication.
Giving a reason, state whether or not this group is cyclic.
Answer/Explanation
Markscheme
\(\left( {\begin{array}{*{20}{c}}
a&0&0\\
0&b&0\\
0&0&c
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
a&0&0\\
0&b&0\\
0&0&c
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{{a^2}}&0&0\\
0&{{b^2}}&0\\
0&0&{{c^2}}
\end{array}} \right)\) A1M1
\( = \left( {\begin{array}{*{20}{c}}
1&0&0\\
0&1&0\\
0&0&1
\end{array}} \right)\) A1
this shows that each matrix is self-inverse
[3 marks]
closure:
\(\left( {\begin{array}{*{20}{c}}
{{a_1}}&0&0\\
0&{{b_1}}&0\\
0&0&{{c_1}}
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
{{a_2}}&0&0\\
0&{{b_2}}&0\\
0&0&{{c_2}}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{{a_1}{a_2}}&0&0\\
0&{{b_1}{b_2}}&0\\
0&0&{{c_1}{c_2}}
\end{array}} \right)\) M1A1
\( = \left( {\begin{array}{*{20}{c}}
{{a_3}}&0&0\\
0&{{b_3}}&0\\
0&0&{{c_3}}
\end{array}} \right)\)
where each of \({a_3}\), \({b_3}\), \({c_3}\) can only be \( \pm 1\) A1
this proves closure
identity: the identity matrix is the group identity A1
inverse: as shown above, every element is self-inverse A1
associativity: this follows because matrix multiplication is associative A1
\(S\) is therefore a group AG
Abelian:
\(\left( {\begin{array}{*{20}{c}}
{{a_2}}&0&0\\
0&{{b_2}}&0\\
0&0&{{c_2}}
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
{{a_1}}&0&0\\
0&{{b_1}}&0\\
0&0&{{c_1}}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{{a_2}{a_1}}&0&0\\
0&{{b_2}{b_1}}&0\\
0&0&{{c_2}{c_1}}
\end{array}} \right)\) A1
\(\left( {\begin{array}{*{20}{c}}
{{a_1}}&0&0\\
0&{{b_1}}&0\\
0&0&{{c_1}}
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
{{a_2}}&0&0\\
0&{{b_2}}&0\\
0&0&{{c_2}}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{{a_1}{a_2}}&0&0\\
0&{{b_1}{b_2}}&0\\
0&0&{{c_1}{c_2}}
\end{array}} \right)\) A1
Note: Second line may have been shown whilst proving closure, however a reference to it must be made here.
we see that the same result is obtained either way which proves commutativity so that the group is Abelian R1
[9 marks]
since all elements (except the identity) are of order \(2\), the group is not cyclic (since \(S\) contains \(8\) elements) R1
[1 mark]

