Question
[Maximum mark: 6]
A random variable $X$ has a probability distribution given in the following table.
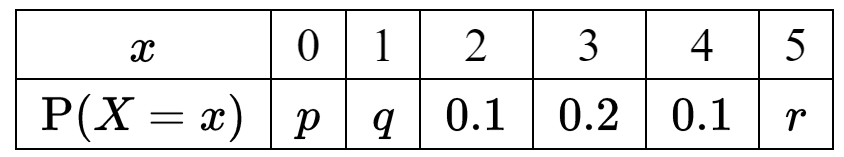
(a) Given that $\mathrm{P}(X>2)=0.6$, find the value of $r$. [2]
(b) Given that $\mathrm{E}[X]=2.9$, find the value of $p$ and the value of $q$. [4]
Answer/Explanation
(a) Using the probability distribution table for $X$, we get
$
\begin{array}{r}
\mathrm{P}(X>2)=0.6 \\
\mathrm{P}(X=3)+\mathrm{P}(X=4)+\mathrm{P}(X=5)=0.6 \\
0.2+0.1+r=0.6 \\
r=0.3
\end{array}
$
(b) Using the formula for the expected value of $X$, we have
$
\begin{aligned}
& \mathrm{E}[X]=\sum x \mathrm{P}(X=x) \\
& 2.9=(0)(p)+(1)(q)+(2)(0.1)+(3)(0.2)+\cdots \\
& +(4)(0.1)+(5)(0.3) \\
& 2.9=q+2.7 \\
& q=0.2 \\
&
\end{aligned}
$
Hence we obtain
$
\begin{aligned}
\sum \mathrm{P}(X=x) & =1 \\
p+0.2+0.1+0.2+0.1+0.3 & =1 \\
p+0.9 & =1 \\
p & =0.1
\end{aligned}
$
Question
[Maximum mark: 5]
The following diagram shows a radioactivity warning symbol made out of a circle in the centre and three equal blades.
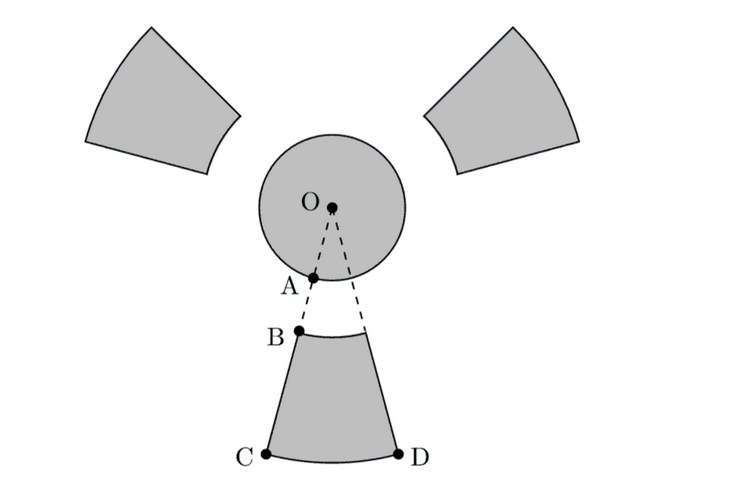
Given that $\mathrm{OA}=2 \mathrm{~cm}, \mathrm{AB}=1 \mathrm{~cm}, \mathrm{BC}=4 \mathrm{~cm}$, and $\mathrm{COD}=30^{\circ}$, find the area of the symbol.
Answer/Explanation
$\text { We have CÔD }=30^{\circ}=\frac{\pi}{6} \text {. }$
Using the area of a sector formula, we have
$
\begin{aligned}
A_{\text {blade }} & =\frac{1}{2}\left[\mathrm{OC}^2-\mathrm{OB}^2\right](\mathrm{C\hat{O}} D) \\
& =\frac{1}{2}\left[7^2-3^2\right]\left[\frac{\pi}{6}\right] \\
& =\frac{10 \pi}{3} \mathrm{~cm}^2
\end{aligned}
$
Hence the area of the symbol is
$
\begin{aligned}
A_{\text {symbol }} & =\pi\left[\mathrm{OA}^2\right]+3 A_{\text {blade }} \\
& =\pi\left[2^2\right]+3\left[\frac{10 \pi}{3}\right] \\
& =4 \pi+10 \pi \\
& =14 \pi \mathrm{cm}^2
\end{aligned}
$
