Download IITian Academy App for accessing Online Mock Tests and More..
Question
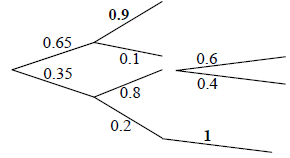
When Andy plays tennis, \(65\% \) of his first serves go into the correct area of the court.
If the first serve goes into the correct area, his chance of winning the point is \(90\% \).
If his first serve does not go into the correct area, Andy is allowed a second serve and, of these, \(80\% \) go into the correct area.
If the second serve goes into the correct area, his chance of winning the point is \(60\% \).
If neither serve goes into the correct area, Andy loses the point.
Complete the tree diagram below.
[2]
Find the probability that Andy loses the point.[4]
Answer/Explanation
Markscheme
(A1)(A1) (C2)[2 marks]
\(0.65 \times 0.1\) (\( = 0.065\)) (A1)
\(0.35 \times 0.8 \times 0.4\) (\( = 0.112\)) (A1)
\(0.35 \times 0.2 \times 1\) the 1 can be implied (\( = 0.07\)) (A1)(ft)
0.247 (A1)(ft) (C4)
Note: No (ft) for any probabilities greater than 1.[4 marks]
Question
The grades obtained by a group of \(20\) IB students are listed below:
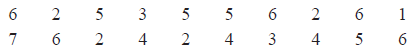
Complete the following table for the grades obtained by the students.
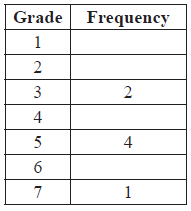[2]
Write down the modal grade obtained by the students.[1]
Calculate the median grade obtained by the students.[2]
One student is chosen at random from the group.
Find the probability that this student obtained either grade \(4\) or grade \(5\).[1]
Answer/Explanation
Markscheme
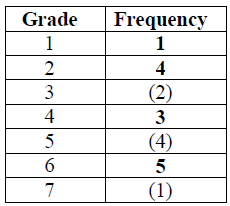(A2) (C2)
Notes: Award (A1) for three correct. Award (A0) for two or fewer correct.[2 marks]
\({\text{Mode}} = 6\) (A1)(ft) (C1)[1 mark]
\({\text{Median}} = 4.5\) (M1)(A1)(ft) (C2)
Note: (M1) for attempt to order raw data (if frequency table not used) or (M1) halfway between 10th and 11th result.[2 marks]
\(\frac{7}{{20}}{\text{ }}(0.35{\text{, }}35\% )\) (A1)(ft) (C1)[1 mark]
Question
A fair six-sided die has the numbers 1, 2, 3, 4, 5, 6 written on its faces. A fair four-sided die has the numbers 1, 2, 3, and 4 written on its faces. The two dice are rolled.
The following diagram shows the possible outcomes.
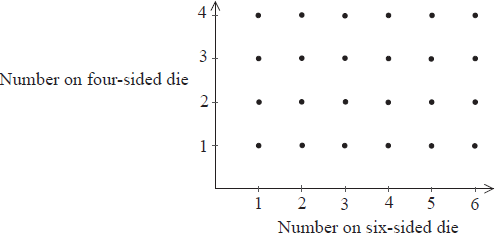
Find the probability that the two dice show the same number.[2]
Find the probability that the difference between the two numbers shown on the dice is 1.[2]
Find the probability that the number shown on the four-sided die is greater than the number shown on the six-sided die, given that the difference between the two numbers is 1.[2]
Answer/Explanation
Markscheme
\(\frac{4}{{24}}\) \(\left( {\frac{1}{6},0.167,16.7{\text{ }}\% } \right)\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
\(\frac{{7}}{{24}}\) \((0.292,29.2{\text{ }}\% )\) (A1)(A1)(ft) (C2)
Note: Award (A1)(ft) from the denominator used in (a).[2 marks]
\(\frac{{3}}{{7}}\) \((0.429,42.9{\text{ }}\% )\) (A1)(A1)(ft) (C2)
Note: Award (A1) for numerator (A1)(ft) for denominator, (ft) from their numerator in (b).[2 marks]
Question
For events A and B, the probabilities are \({\text{P}}(A) = \frac{4}{13}\) and \({\text{P}}(B) = \frac{5}{13}\).
If events A and B are mutually exclusive, write down the value of \({\text{P}} (A\cap B)\).[1]
If events A and B are independent, find the value of \({\text{P}} (A\cap B)\).[2]
If \({\text{P}} (A \cup B) = \frac{7}{13}\), find the value of \({\text{P}} (A \cap B)\).[3]
Answer/Explanation
Markscheme
\({\rm{P}}(A \cap B) = 0\) (A1) (C1)[1 mark]
\({\rm{P}}(A \cap B) = {\rm{P}}(A) \times {\rm{P}}(B)\)
\( = \frac{4}{{13}} \times \frac{5}{{13}}\) (M1)
Note: Award (M1) for product of two fractions, decimals or percentages.
\({\rm{P}}(A \cap B) = \frac{{20}}{{169}} (= 0.118)\) (A1) (C2)[2 marks]
\(\frac{7}{{13}} = \frac{4}{{13}} + \frac{5}{{13}} – {\rm{P}}(A \cap B)\) (M1)(M1)
Notes: Award (M1) for \(\frac{4}{{13}} + \frac{5}{{13}}\) seen, (M1) for subtraction of \(\frac{7}{{13}}\) shown.
OR
Award (M1) for Venn diagram with 2 intersecting circles, (A1) for correct probabilities in diagram.
\({\rm{P}}(A \cap B) = \frac{2}{{13}}( = 0.154)\) (A1) (C3)[3 marks]
Question
A weighted die has 2 red faces, 3 green faces and 1 black face. When the die is thrown, the black face is three times as likely to appear on top as one of the other five faces. The other five faces have equal probability of appearing on top.
The following table gives the probabilities.
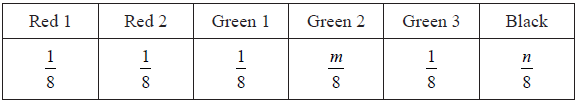
Find the value of
(i) m;
(ii) n.[2]
The die is thrown once.
Given that the face on top is not red, find the probability that it is black.[2]
The die is now thrown twice.
Calculate the probability that black appears on top both times.[2]
Answer/Explanation
Markscheme
(i) m = 1 (A1)
(ii) n = 3 (A1) (C2)
Note: Award (A0)(A1)(ft) for \(m = \frac{1}{8}, n = \frac{3}{8}\).
Award (A0)(A1)(ft) for m = 3, n = 1.[2 marks]
\({\rm{P}}(B/R’) = \frac{{\frac{3}{8}}}{{\frac{6}{8}}} = \frac{3}{6}\left( {\frac{1}{2},50\% ,0.5} \right)\) (M1)(A1)(ft) (C2)
Note: Award (M1) for correctly substituted conditional probability formula or for 6 seen as part of denominator.[2 marks]
\({\rm{P}}(B,B) = \frac{3}{8} \times \frac{3}{8} = \frac{9}{{64}}(0.141)\) (M1)(A1)(ft) (C2)
Note: Award (M1) for product of two correct fractions, decimals or percentages.
(ft) from their answer to part (a) (ii).[2 marks]
Question
The probability that it will snow tomorrow is 0.3.
If it snows tomorrow the probability that Chuck will be late for school is 0.8.
If it does not snow tomorrow the probability that Chuck will be late for school is 0.1.
Complete the tree diagram below.
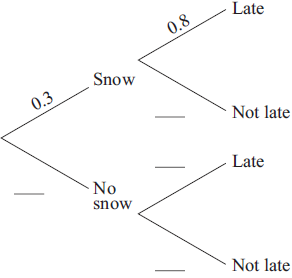[3]
Find the probability that it does not snow tomorrow and Chuck is late for school.[1]
Find the probability that Chuck is late for school.[2]
Answer/Explanation
Markscheme
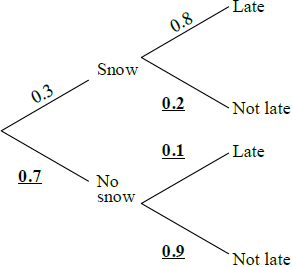(A1)(A1)(A1) (C3)
Note: Award (A1) for each correct pair.[3 marks]
\(0.7 \times 0.1\)
\( = 0.07(\frac{7}{{100}},{\text{ }}7\% )\) (A1)(ft) (C1)[1 mark]
\(0.3 \times 0.8 + 0.07\) (M1)
\( = 0.31(\frac{{31}}{{100}},{\text{ }}31\% )\) (A1)(ft)
Note: In (b) and (c) follow through from sensible answers only i.e. not a probability greater than one. (C2)[2 marks]
Question
A class consists of students studying Spanish or French or both. Fifteen students study Spanish and twelve study French.
The probability that a student studies French given that she studies Spanish is \(\frac{{7}}{{15}}\).
Draw a Venn diagram in the space below to illustrate this information.[3]
Find the probability that a student studies Spanish given that she studies one language only.[3]
Answer/Explanation
Markscheme
(A1)(A1)(A1) (C3)
Note: Award (A1) for a labeled Venn diagram with appropriate sets.
(A1) for 7, (A1) for 8 and 5.[3 marks]
\({\text{P (Spanish / one language only)}} = \frac{{\frac{8}{{20}}}}{{\frac{8}{{20}} + \frac{5}{{20}}}}\) (M1)(A1)(ft)
Note: Award (M1) for substituted conditional probability formula, (A1) for correct substitution. Follow through from their Venn diagram.
\( = \frac{8}{{13}}(0.615,{\text{ }}61.5\% )\) (A1)(ft)
OR
\({\text{P}}{\text{ (Spanish / one language only)}} = \frac{8}{{8 + 5}}\) (A1)(ft)(M1)
Note: Award (A1) for their correct numerator, (M1) for correct recognition of regions. Follow through from their Venn diagram.
\( = \frac{8}{{13}}(0.615,{\text{ }}61.5\% )\) (A1)(ft) (C3)[3 marks]
Question
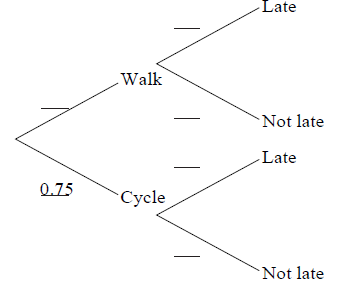
Maria travels to school either by walking or by bicycle. The probability she cycles to school is 0.75.
If she walks, the probability that she is late for school is 0.1.
If she cycles, the probability that she is late for school is 0.05.
Complete the tree diagram below, showing the appropriate probabilities.
[3]
Find the probability that Maria is late for school.[3]
Answer/Explanation
Markscheme
(A1)(A1)(A1) (C3)
Note: Award (A1) for 0.25, (A1) for 0.1 and 0.9, (A1) for 0.05 and 0.95[3 marks]
\({\text{P}}({\text{late}}) = 0.25 \times 0.1 + 0.75 \times 0.05\) (A1)(ft)(M1)
Note: Award (A1)(ft) for two correct products from their diagram and award (M1) for addition of their two products.
\( = 0.0625\left( {\frac{1}{{16}},{\text{ }}6.25\% } \right)\) (A1)(ft) (C3)[3 marks]
Question
In a research project on the relation between the gender of 150 science students at college and their degree subject, the following set of data is collected.
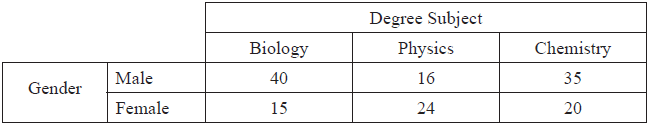
Find the probability that a student chosen at random is male.[2]
Find the probability that a student chosen at random is either male or studies Chemistry.[2]
Find the probability that a student chosen at random studies Physics, given that the student is male.[2]
Answer/Explanation
Markscheme
\( = \frac{{91}}{{150}}(0.607,{\text{ }}60.6\,\% ,{\text{ }}60.7\,\% )\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
\( = \frac{{111}}{{150}}\left( {\frac{{37}}{{50}},{\text{ }}0.74,{\text{ }}74\,\% } \right)\) (A1)(ft)(A1) (C2)
Note: Award (A1)(ft) for their numerator in (a) +20 provided the final answer is not greater than 1. (A1) for denominator.[2 marks]
\(\frac{{16}}{{91}}(0.176,{\text{ }}17.6\,\% )\) (A1)(A1)(ft) (C2)
Note: Award (A1) for numerator and (A1)(ft) for denominator. Follow through from their numerator in (a) provided answer is not greater than 1.[2 marks]
Question
Let \({\text{P}}(A) = 0.5\), \({\text{P}}(B) = 0.6\) and \({\text{P}}(A \cup B) = 0.8\).
Find \({\text{P}}(A \cap B)\).[2]
Find \({\text{P}}(A|B)\).[2]
Decide whether A and B are independent events. Give a reason for your answer.[2]
Answer/Explanation
Markscheme
\(0.8 = 0.5 + 0.6 – {\text{P}}(A \cap B)\) (M1)
\({\text{P}}(A \cap B) = 0.3\) (A1) (C2)
Note: Award (M1) for correct substitution, (A1) for correct answer.[2 marks]
\({\text{P}}(A|B) = \frac{{0.3}}{{0.6}}\) (M1)
= 0.5 (A1)(ft) (C2)
Note: Award (M1) for correct substitution in conditional probability formula. Follow through from their answer to part (a), provided probability is not greater than one.[2 marks]
\({\text{P}}(A \cap B) = {\text{P}}(A) \times {\text{P}}(B)\) or 0.3 = 0.5 × 0.6 (R1)
OR
\({\text{P}}(A|B) = {\text{P}}(A)\) (R1)
they are independent. (Yes) (A1)(ft) (C2)
Note: Follow through from their answers to parts (a) or (b).
Do not award (R0)(A1).[2 marks]
Question
A survey was carried out in a group of 200 people. They were asked whether they smoke or not. The collected information was organized in the following table.
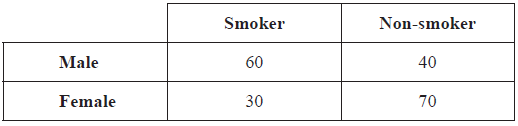
One person from this group is chosen at random.
Write down the probability that this person is a smoker.[2]
Write down the probability that this person is male given that they are a smoker.[2]
Find the probability that this person is a smoker or is male.[2]
Answer/Explanation
Markscheme
\(\frac{{90}}{{200}}(0.45,{\text{ }}45{\text{ }}\% )\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
\(\frac{{60}}{{90}}(0.\bar 6,{\text{ }}0.667,{\text{ }}66.\bar 6{\text{ }}\% ,{\text{ }}66.6 \ldots {\text{ }}\% ,{\text{ }}66.7{\text{ }}\% )\) (A1)(A1)(ft) (C2)
Notes: Award (A1) for numerator, (A1)(ft) for denominator, follow through from their numerator in part (a). Last mark is lost if answer is not a probability.[2 marks]
\(\frac{{90}}{{200}} + \frac{{100}}{{200}} – \frac{{60}}{{200}}\) (M1)
Note: Award (M1) for correct substitution in the combined events formula. Follow through from their answer to part (a).
\( = \frac{{130}}{{200}}(0.65,{\text{ }}65{\text{ }}\% )\) (A1)(ft)
OR
\(\frac{{60}}{{200}} + \frac{{40}}{{200}} + \frac{{30}}{{200}}\) (M1)
Note: Award (M1) for adding the correct fractions.
\( = \frac{{130}}{{200}}(0.65,{\text{ }}65{\text{ }}\% )\) (A1)
OR
\(1 – \frac{{70}}{{200}}\) (M1)
Note: Award (M1) for subtraction of correct fraction from 1.
\( = \frac{{130}}{{200}}(0.65,{\text{ }}65{\text{ }}\% )\) (A1) (C2)
[2 marks]
Question
The probability that it rains today is \(0.4\) . If it rains today, the probability that it will rain tomorrow is \(0.8\) . If it does not rain today, the probability that it will rain tomorrow is \(0.7\) .
Complete the tree diagram below.
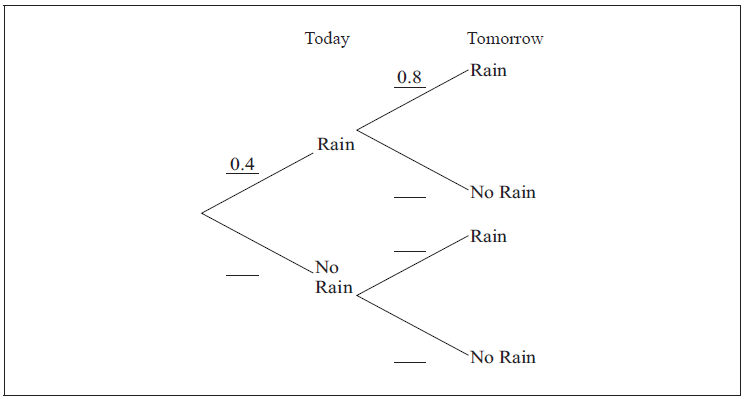[3]
Calculate the probability of rain tomorrow.[3]
Answer/Explanation
Markscheme
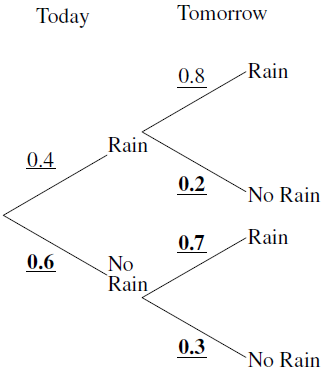(A1)(A1)(A1) (C3)
Note: Award (A1) for each correct pair.[3 marks]
\(0.4 \times 0.8 + 0.6 \times 0.7\) (A1)(ft)(M1)
Notes: Award (A1)(ft) for two consistent products from tree diagram, (M1) for addition of their products. Follow through from their tree diagram provided all probabilities are between 0 and 1.
\( = 0.74\) (A1)(ft) (C3)[3 marks]
Question
A survey was carried out at an international airport. A number of travellers were interviewed and asked for their flight destinations. The results are shown in the table below.
One traveller is to be chosen at random from all those interviewed.
Find the probability that this traveller was going to Africa.[2]
One female traveller is to be chosen at random from all those interviewed.
Find the probability that this female traveller was going to Asia.[2]
One traveller is to be chosen at random from those not going to America.
Find the probability that the chosen traveller is female.[2]
Answer/Explanation
Markscheme
\(\frac{{108}}{{250}}{\text{ }}\left( {\frac{{54}}{{125}}{\text{, }}0.432{\text{, }}43.2\% } \right)\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
\(\frac{{25}}{{106}}{\text{ }}\left( {0.236{\text{, }}23.6\% } \right)\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
\(\frac{{71}}{{170}}{\text{ }}\left( {0.418{\text{, }}41.8\% } \right)\) (A1)(A1) (C2)
Note: Award (A1) for numerator, (A1) for denominator.[2 marks]
Question
A bag contains 7 red discs and 4 blue discs. Ju Shen chooses a disc at random from the bag and removes it. Ramón then chooses a disc from those left in the bag.
Write down the probability that
(i) Ju Shen chooses a red disc from the bag;
(ii) Ramón chooses a blue disc from the bag, given that Ju Shen has chosen a red disc;
(iii) Ju Shen chooses a red disc and Ramón chooses a blue disc from the bag.[3]
Find the probability that Ju Shen and Ramón choose different coloured discs from the bag.[3]
Answer/Explanation
Markscheme
(i) \(\frac{7}{{11}}\) (\(0.636\), \(63.6\% \)) (\(0.636363 \ldots \)) (A1) (C1)
(ii) \(\frac{4}{{10}}\) \(\left( {\frac{2}{5}{\text{, }}0.4{\text{, }}40\% } \right)\) (A1) (C1)
(iii) \(\frac{{28}}{{110}}\) \(\left( {\frac{{14}}{{55}}{\text{, }}0.255{\text{, }}25.5\% } \right)\) \(0.254545 \ldots \) (A1)(ft) (C1)
Note: Follow through from the product of their answers to parts (a) (i) and (ii).[3 marks]
\(\frac{{28}}{{110}} + \left( {\frac{4}{{11}} \times \frac{7}{{10}}} \right)\) OR \(2 \times \frac{{28}}{{110}}\) (M1)(M1)
Notes: Award (M1) for using their \(\frac{{28}}{{110}}\) as part of a combined probability expression. (M1) for either adding \({\frac{4}{{11}} \times \frac{7}{{10}}}\) or for multiplying by 2.
\( = \frac{{56}}{{110}}\) \(\left( {\frac{{28}}{{55}}{\text{, }}0.509{\text{, }}50.9\% } \right)\) (\(0.509090 \ldots \)) (A1)(ft) (C3)
Note: Follow through applies from their answer to part (a) (iii) and only when their answer is between 0 and 1.[3 marks]
Question
Merryn plans to travel to a concert tomorrow. Due to bad weather, there is a 60 % chance that all flights will be cancelled tomorrow. If the flights are cancelled Merryn will travel by car.
If she travels by plane the probability that she will be late for the concert is 10 %.
If she travels by car, the probability that she will not be late for the concert is 25 %.
Complete the tree diagram below.
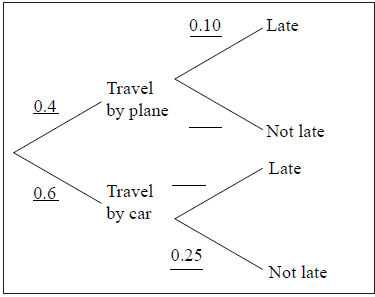[1]
Find the probability that Merryn will not be late for the concert.[3]
Merryn was not late for the concert the next day.
Given that, find the probability that she travelled to the concert by car.[2]
Answer/Explanation
Markscheme
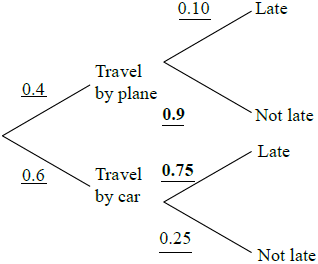(A1) (C1)
Note: Award (A1) for 0.9 and 0.75.[1 mark]
0.4 × 0.9 + 0.6 × 0.25 (M1)(M1)
Note: Award (M1) for their two relevant products, (M1) for adding their two products.
\(0.51\left( {\frac{{51}}{{100}},{\text{ }}51\% } \right)\) (A1)(ft) (C3)
Note: Follow through from their answers to part (a).[3 marks]
\(\frac{{0.6 \times 0.25}}{{0.51}}\) (M1)
Note: Award (M1) for correctly substituted conditional probability formula.
\(0.294\left( {\frac{5}{{17}}{\text{, }}0.294117…} \right)\) (A1)(ft) (C2)
Note: Follow through from their tree diagram and their part (b).[2 marks]
Question
The probability that Tanay eats lunch in the school cafeteria is \(\frac{3}{5}\).
If he eats lunch in the school cafeteria, the probability that he has a sandwich is \(\frac{3}{{10}}\).
If he does not eat lunch in the school cafeteria the probability that he has a sandwich is \(\frac{9}{{10}}\).
Complete the tree diagram below.
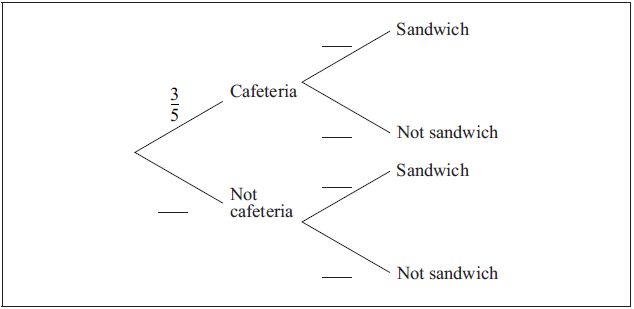[3]
Find the probability that Tanay has a sandwich for his lunch.[3]
Answer/Explanation
Markscheme
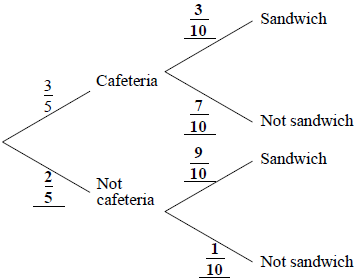(A1)(A1)(A1) (C3)
Note: Award (A1) for each correct pair of branches.
\(\frac{3}{5} \times \frac{3}{{10}} + \frac{2}{5} \times \frac{9}{{10}}\) (A1)(ft)(M1)
Notes: Award (A1)(ft) for their two correct products, (M1) for addition of their products. Follow through from their tree diagram.
\( = \frac{{27}}{{50}}(0.54,54\% )\) (A1)(ft) (C3)
Question
Alan’s laundry basket contains two green, three red and seven black socks. He selects one sock from the laundry basket at random.
Write down the probability that the sock is red.[1]
Alan returns the sock to the laundry basket and selects two socks at random.
Find the probability that the first sock he selects is green and the second sock is black.[2]
Alan returns the socks to the laundry basket and again selects two socks at random.
Find the probability that he selects two socks of the same colour.[3]
Answer/Explanation
Markscheme
\(\frac{3}{{12}}\left( {\frac{1}{4},0.25,25\% } \right)\) (A1) (C1)
\(\left( {\frac{2}{{12}}} \right) \times \left( {\frac{7}{{11}}} \right)\) (M1)
Note: Award (M1) for correct product.
\( = \frac{{14}}{{132}}\left( {\frac{7}{{66}},0.10606…,10.6\% } \right)\) (A1) (C2)
\(\left( {\frac{2}{{12}} \times \frac{1}{{11}}} \right) + \left( {\frac{3}{{12}} \times \frac{2}{{11}}} \right) + \left( {\frac{7}{{12}} \times \frac{6}{{11}}} \right)\) (M1)(M1)
Note: Award (M1) for addition of their 3 products, (M1) for 3 correct products.
\( = \frac{{50}}{{132}}\left( {\frac{25}{{66}},0.37878…,37.9\% } \right)\) (A1) (C3)
Question
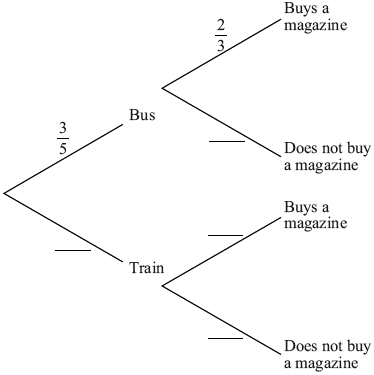
Ramzi travels to work each day, either by bus or by train. The probability that he travels by bus is \(\frac{3}{5}\). If he travels by bus, the probability that he buys a magazine is \(\frac{2}{3}\). If he travels by train, the probability that he buys a magazine is \(\frac{3}{4}\).
Complete the tree diagram.
[3]
Find the probability that Ramzi buys a magazine when he travels to work.[3]
Answer/Explanation
Markscheme
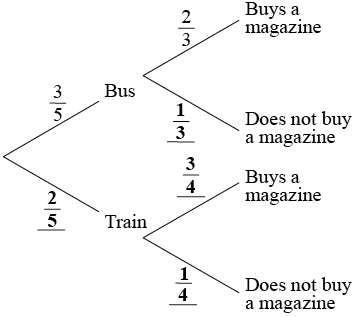(A1)(A1)(A1) (C3)
Note: Award (A1) for each correct pair of branches.[3 marks]
\(\frac{3}{5} \times \frac{2}{3} + \frac{2}{5} \times \frac{3}{4}\) (A1)(ft)(M1)
Notes: Award (A1)(ft) for two consistent products from tree diagram, (M1) for addition of their products.
Follow through from their tree diagram provided all probabilities are between \(0\) and \(1\).
\(\frac{7}{{10}}{\text{ }}\left( {{\text{0.7, 70% , }}\frac{{42}}{{60}}} \right)\) (A1)(ft) (C3)[3 marks]
Question
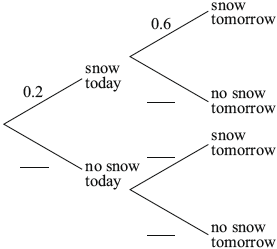
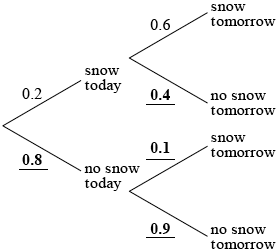
The probability that it snows today is 0.2. If it does snow today, the probability that it will snow tomorrow is 0.6. If it does not snow today, the probability that it will not snow tomorrow is 0.9.
Using the information given, complete the following tree diagram.
[3]
Calculate the probability that it will snow tomorrow.[3]
Answer/Explanation
Markscheme
(A1)(A1)(A1) (C3)
Note: Award (A1) for each correct pair of probabilities.[3 marks]
\(0.2 \times 0.6 + 0.8 \times 0.1\) (A1)(ft)(M1)
Note: Award (A1)(ft) for two correct products of probabilities taken from their diagram, (M1) for the addition of their products.
\( = 0.2{\text{ }}\left( {\frac{1}{5},{\text{ 20% }}} \right)\) (A1)(ft) (C3)
Note: Accept any equivalent correct fraction.
Follow through from their tree diagram.[3 marks]
Question
Aleph has an unbiased cubical (six faced) die on which are written the numbers
1 , 2 , 3 , 4 , 5 and 6.
Beth has an unbiased tetrahedral (four faced) die on which are written the numbers
2 , 3 , 5 and 7.
Complete the Venn diagram with the numbers written on Aleph’s die (\(A\)) and Beth’s die (\(B\)).
[2]
Find \(n(B \cap A’)\).[2]
Aleph and Beth are each going to roll their die once only. Shin says the probability that each die will show the same number is \(\frac{1}{8}\).
Determine whether Shin is correct. Give a reason.[2]
Answer/Explanation
Markscheme
(A1)(A1) (C2)
Note: Award (A1) for 2, 3, 5 in intersection, (A1) for 1, 4, 6, 7 correctly placed.
\(1\) (M1)(A1)(ft) (C2)
Notes: Award (M1)(A0) for listing the elements of their set \(B \cap A’\);shading the correct region on diagram; or an answer of \(1/7\) with a correct Venn diagram. Follow through from part (a).
Correct, from \((2,{\text{ }}2){\text{ }}(3,{\text{ }}3)\) and \((5,{\text{ }}5)\) on sample space
OR
Correct, from a labelled tree diagram
OR
Correct, from a sample space diagram
OR
Correct, from \(3 \times \frac{1}{4} \times \frac{1}{6}\;\;\;\)(or equivalent) (A1)(ft)(R1) (C2)
Notes: Do not award (A1)(ft)(R0). Award (R1) for a consistent reason with their part (a). Follow through from part (a).
Question
Peter either walks or cycles to work. The probability that he walks is 0.25. If Peter walks to work, the probability that he is late is 0.1. If he cycles to work, the probability that he is late is 0.05. The tree diagram for this information is shown.
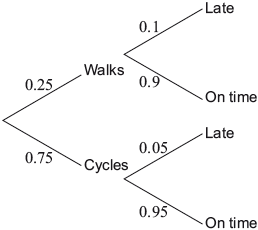
On a day chosen at random, Peter walked to work.
Write down the probability that he was on time.[1]
For a different day, also chosen at random,
find the probability that Peter cycled to work and was late.[2]
For a different day, also chosen at random,
find the probability that, given Peter was late, he cycled to work.[3]
Answer/Explanation
Markscheme
\(0.9\) (A1) (C1)
\(0.75 \times 0.05\) (M1)
\( = 0.0375\;\;\;\left( {\frac{3}{{80}},{\text{ 3,75% }}} \right)\) (A1) (C2)
\(\frac{{0.75 \times 0.05}}{{0.75 \times 0.05 + 0.25 \times 0.1}}\) (M1)(M1)
Note: Award (M1) for their correct numerator, (M1) for their correct denominator, ie, \(\left( {\frac{{{\text{their (b)}}}}{{{\text{their (b)}} + 0.25 \times 0.1}}} \right)\).
Do not award (M1) for their \(0.0375\) or \(0.0625\) if not a correct part of a fraction.
\( = 0.6\;\;\;\left( {\frac{3}{5},{\text{ }}60\% } \right)\) (A1)(ft) (C3)
Note: Follow through from part (b).
Question
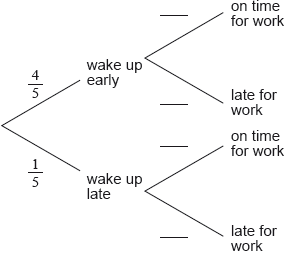
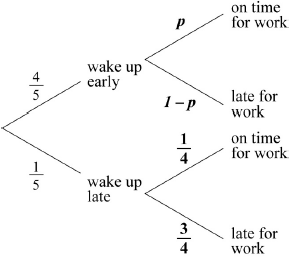
On a work day, the probability that Mr Van Winkel wakes up early is \(\frac{4}{5}\).
If he wakes up early, the probability that he is on time for work is \(p\).
If he wakes up late, the probability that he is on time for work is \(\frac{1}{4}\).
The probability that Mr Van Winkel arrives on time for work is \(\frac{3}{5}\).
Complete the tree diagram below.
[2]
Find the value of \(p\).[4]
Answer/Explanation
Markscheme
(A1)(A1) (C2)
Note: Award (A1) for each correct pair of probabilities.[2 marks]
\(\frac{4}{5}p + \frac{1}{5} \times \frac{1}{4} = \frac{3}{5}\) (A1)(ft)(M1)(M1)
Note: Award (A1)(ft) for two correct products from part (a), (M1) for adding their products, (M1) for equating the sum of any two probabilities to \(\frac{3}{5}\).
\((p = ){\text{ }}\frac{{11}}{{16}}{\text{ }}(0.688,{\text{ }}0.6875)\) (A1)(ft) (C4)
Note: Award the final (A1)(ft) only if \(0 \leqslant p \leqslant 1\). Follow through from part (a).[4 marks]
Question
In the Canadian city of Ottawa:
\[\begin{array}{*{20}{l}} {{\text{97% of the population speak English,}}} \\ {{\text{38% of the population speak French,}}} \\ {{\text{36% of the population speak both English and French.}}} \end{array}\]
The total population of Ottawa is \(985\,000\).
Calculate the percentage of the population of Ottawa that speak English but not French.[2]
Calculate the number of people in Ottawa that speak both English and French.[2]
Write down your answer to part (b) in the form \(a \times {10^k}\) where \(1 \leqslant a < 10\) and k \( \in \mathbb{Z}\).[2]
Answer/Explanation
Markscheme
\(97 – 36\) (M1)
Note: Award (M1) for subtracting 36 from 97.
OR
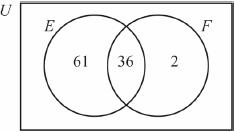
(M1)
Note: Award (M1) for 61 and 36 seen in the correct places in the Venn diagram.
\( = 61{\text{ }}(\% )\) (A1) (C2)
Note: Accept 61.0 (%).[2 marks]
\(\frac{{36}}{{100}} \times 985\,000\) (M1)
Note: Award (M1) for multiplying 0.36 (or equivalent) by \(985\,000\).
\( = 355\,000{\text{ }}(354\,600)\) (A1) (C2)[2 marks]
\(3.55 \times {10^5}{\text{ }}(3.546 \times {10^5})\) (A1)(ft)(A1)(ft) (C2)
Note: Award (A1)(ft) for 3.55 (3.546) must match part (b), and (A1)(ft) \( \times {10^5}\).
Award (A0)(A0) for answers of the type: \(35.5 \times {10^4}\). Follow through from part (b).[2 marks]
Question
The Home Shine factory produces light bulbs, 7% of which are found to be defective.
Francesco buys two light bulbs produced by Home Shine.
The Bright Light factory also produces light bulbs. The probability that a light bulb produced by Bright Light is not defective is \(a\).
Deborah buys three light bulbs produced by Bright Light.
Write down the probability that a light bulb produced by Home Shine is not defective.[1]
Find the probability that both light bulbs are not defective.[2]
Find the probability that at least one of Francesco’s light bulbs is defective.[2]
Write down an expression, in terms of \(a\), for the probability that at least one of Deborah’s three light bulbs is defective.[1]
Answer/Explanation
Markscheme
0.93 (93%) (A1) (C1)[1 mark]
\(0.93 \times 0.93\) (M1)
Note: Award (M1) for squaring their answer to part (a).
0.865 (0.8649; 86.5%) (A1)(ft) (C2)
Notes: Follow through from part (a).
Accept \(0.86{\text{ }}\left( {{\text{unless it follows }}\frac{{93}}{{100}} \times \frac{{92}}{{99}}} \right)\).[2 marks]
\(1 – 0.8649\) (M1)
Note: Follow through from their answer to part (b)(i).
OR
\(0.07 \times 0.07 + 2 \times (0.07 \times 0.93)\) (M1)
Note: Follow through from part (a).
0.135 (0.1351; 13.5%) (A1)(ft) (C2)[2 marks]
\(1 – {a^3}\) (A1) (C1)
Note: Accept \(3{a^2}(1 – a) + 3a{(1 – a)^2} + {(1 – a)^3}\) or equivalent.[1 mark]
Question
Rosewood College has 120 students. The students can join the sports club (\(S\)) and the music club (\(M\)).
For a student chosen at random from these 120, the probability that they joined both clubs is \(\frac{1}{4}\) and the probability that they joined the music club is\(\frac{1}{3}\).
There are 20 students that did not join either club.
Complete the Venn diagram for these students.
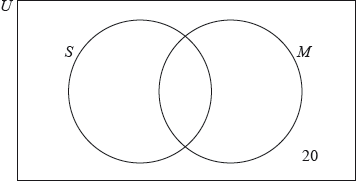[2]
One of the students who joined the sports club is chosen at random. Find the probability that this student joined both clubs.[2]
Determine whether the events \(S\) and \(M\) are independent.[2]
Answer/Explanation
Markscheme
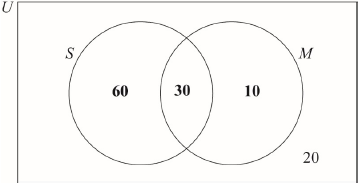(A1)(A1) (C2)
Note: Award (A1) for 30 in correct area, (A1) for 60 and 10 in the correct areas.[2 marks]
\(\frac{{30}}{{90}}{\text{ }}\left( {\frac{1}{3},{\text{ }}0.333333 \ldots ,{\text{ }}33.3333 \ldots \% } \right)\) (A1)(ft)(A1)(ft) (C2)
Note: Award (A1)(ft) for correct numerator of 30, (A1)(ft) for correct denominator of 90. Follow through from their Venn diagram.[2 marks]
\({\text{P}}(S) \times {\text{P}}(M) = \frac{3}{4} \times \frac{1}{3} = \frac{1}{4}\) (R1)
Note: Award (R1) for multiplying their by \(\frac{1}{3}\).
therefore the events are independent \(\left( {{\text{as P}}(S \cap M) = \frac{1}{4}} \right)\) (A1)(ft) (C2)
Note: Award (R1)(A1)(ft) for an answer which is consistent with their Venn diagram.
Do not award (R0)(A1)(ft).
Do not award final (A1) if \({\text{P}}(S) \times {\text{P}}(M)\) is not calculated. Follow through from part (a).[2 marks]
Question
In an international competition, participants can answer questions in only one of the three following languages: Portuguese, Mandarin or Hindi. 80 participants took part in the competition. The number of participants answering in Portuguese, Mandarin or Hindi is shown in the table.
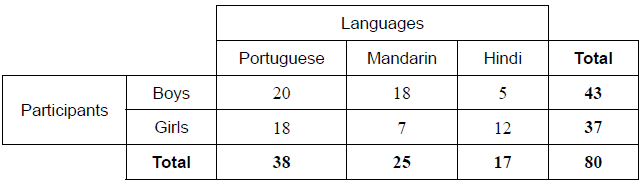
A boy is chosen at random.
State the number of boys who answered questions in Portuguese.[1]
Find the probability that the boy answered questions in Hindi.[2]
Two girls are selected at random.
Calculate the probability that one girl answered questions in Mandarin and the other answered questions in Hindi.[3]
Answer/Explanation
Markscheme
20 (A1) (C1)[1 mark]
\(\frac{5}{{43}}\,\,\,\left( {0.11627 \ldots ,\,\,11.6279 \ldots {\text{% }}} \right)\) (A1)(A1) (C2)
Note: Award (A1) for correct numerator, (A1) for correct denominator.[2 marks]
\(\frac{7}{{37}} \times \frac{{12}}{{36}} + \frac{{12}}{{37}} \times \frac{7}{{36}}\) (A1)(M1)
Note: Award (A1) for first or second correct product seen, (M1) for adding their two products or for multiplying their product by two.
\( = \frac{{14}}{{111}}\,\,\left( {\,0.12612 \ldots ,\,\,12.6126\,{\text{% }}} \right)\) (A1) (C3)[3 marks]