Download IITian Academy App for accessing Online Mock Tests and More..
Question
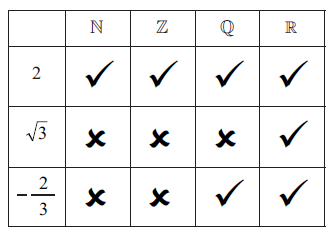
Consider the numbers \(2\), \(\sqrt 3 \), \( – \frac{2}{3}\) and the sets \(\mathbb{N}\), \(\mathbb{Z}\), \(\mathbb{Q}\) and \(\mathbb{R}\).
Complete the table below by placing a tick in the appropriate box if the number is an element of the set, and a cross if it is not.
[3]
A function \(f\) is given by \(f(x) = 2{x^2} – 3x{\text{, }}x \in \{ – 2{\text{, }}2{\text{, }}3\} \).
Write down the range of function \(f\).[1]
Answer/Explanation
Markscheme
(A1)(A1)(A1) (C3)
Note: Accept any symbol for ticks. Do not penalise if the other boxes are left blank.[3 marks]
\({\text{Range}} = \{ 2{\text{, }}9{\text{, }}14\} \) (A1)(ft) (C1)
Note: Brackets not required.[1 mark]
Question
Factorise the expression \({x^2} – 3x – 10\).[2]
A function is defined as \(f(x) = 1 + {x^3}\) for \(x \in \mathbb{Z}{\text{, }} {- 3} \leqslant x \leqslant 3\).
(i) List the elements of the domain of \(f(x)\).
(ii) Write down the range of \(f(x)\).[4]
Answer/Explanation
Markscheme
\((x – 5)(x + 2)\) (A1)(A1) (C2)
Note: Award (A1) for \((x + 5)(x – 2)\), (A0) otherwise. If equation is equated to zero and solved by factorizing award (A1) for both correct factors, followed by (A0).[2 marks]
(i) \( – 3\), \( – 2\), \( – 1\), \(0\), \(1\), \(2\), \(3\) (A1)(A1) (C2)
Note: Award (A2) for all correct answers seen and no others. Award (A1) for 3 correct answers seen.
(ii) \( – 26\), \( – 7\), 0,1, 2, 9, 28 (A1)(A1) (C2)
Note: Award (A2) for all correct answers seen and no others. Award (A1) for 3 correct answers seen. If domain and range are interchanged award (A0) for (b)(i) and (A1)(ft)(A1)(ft) for (b)(ii).[4 marks]
Question
The graph of a quadratic function \(y = f (x)\) is given below.
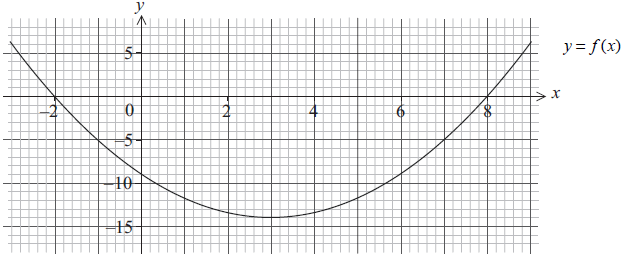
Write down the equation of the axis of symmetry.[2]
Write down the coordinates of the minimum point.[2]
Write down the range of \(f (x)\).[2]
Answer/Explanation
Markscheme
x = 3 (A1)(A1) (C2)
Notes: Award (A1) for “ x = ” (A1) for 3.
The mark for x = is not awarded unless a constant is seen on the other side of the equation.[2 marks]
(3, −14) (Accept x = 3, y = −14) (A1)(ft)(A1) (C2)Note: Award (A1)(A0) for missing coordinate brackets.[2 marks]
y ≥ −14 (A1)(A1)(ft) (C2)
Notes: Award (A1) for y ≥ , (A1)(ft) for –14.
Accept alternative notation for intervals.[2 marks]
Question
Given the function \(f (x) = 2 \times 3^x\) for −2 \( \leqslant \) x \( \leqslant \) 5,
find the range of \(f\).[4]
find the value of \(x\) given that \(f (x) =162\).[2]
Answer/Explanation
Markscheme
\(f (-2) = 2 \times 3^{-2}\) (M1)
\(= \frac{{2}}{{9}}(0.222)\) (A1)
\(f (5) = 2 \times 3^5\)
\(= 486\) (A1)
\({\text{Range }}\frac{2}{9} \leqslant f(x) \leqslant 486\) OR \(\left[ {\frac{2}{9},{\text{ }}486} \right]\) (A1) (C4)
Note: Award (M1) for correct substitution of –2 or 5 into \(f (x)\), (A1)(A1) for each correct end point.[4 marks]
\(2 \times 3^x = 162\) (M1)
\(x = 4\) (A1) (C2)[2 marks]
Question
A quadratic function, \(f(x) = a{x^2} + bx\), is represented by the mapping diagram below.
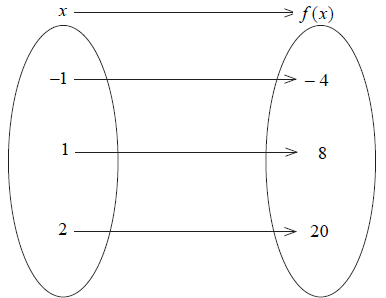
Use the mapping diagram to write down two equations in terms of a and b.[2]
Find the value of a.[1]
Find the value of b.[1]
Calculate the x-coordinate of the vertex of the graph of f (x).[2]
Answer/Explanation
Markscheme
4a + 2b = 20
a + b = 8 (A1)
a – b = –4 (A1) (C2)
Note: Award (A1)(A1) for any two of the given or equivalent equations.[2 marks]
a = 2 (A1)(ft)[1 mark]
b = 6 (A1)(ft) (C2)
Note: Follow through from their (a).[1 mark]
\(x = – \frac{6}{{2(2)}}\) (M1)
Note: Award (M1) for correct substitution in correct formula.
\( = -1.5\) (A1)(ft) (C2) [2 marks]
Question
The graph of y = 2x2 \( – \) rx + q is shown for \( – 5 \leqslant x \leqslant 7\).
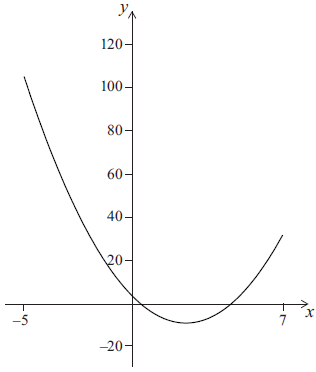
The graph cuts the y axis at (0, 4).
Write down the value of q.[1]
The axis of symmetry is x = 2.5.
Find the value of r.[2]
The axis of symmetry is x = 2.5.
Write down the minimum value of y.[1]
The axis of symmetry is x = 2.5.x
Write down the range of y.[2]
Answer/Explanation
Markscheme
q = 4 (A1) (C1)[1 mark]
\(2.5 = \frac{r}{4}\) (M1)
r = 10 (A1) (C2)[2 marks]
–8.5 (A1)(ft) (C1)[1 mark]
\(-8.5 \leqslant y \leqslant 104\) (A1)(ft)(A1)(ft) (C2)
Notes: Award (A1)(ft) for their answer to part (c) with correct inequality signs, (A1)(ft) for 104. Follow through from their values of q and r.
Accept 104 ±2 if read from graph.[2 marks]
Question
The following is the graph of the quadratic function y = f (x).
Write down the solutions to the equation f (x) = 0.[2]
Write down the equation of the axis of symmetry of the graph of f (x).[2]
The equation f (x) = 12 has two solutions. One of these solutions is x = 6. Use the symmetry of the graph to find the other solution.[1]
The minimum value for y is – 4. Write down the range of f (x).[1]
Answer/Explanation
Markscheme
x = 0, x = 4 (A1)(A1) (C2)
Notes: Accept 0 and 4.[2 marks]
x = 2 (A1)(A1) (C2)
Note: Award (A1) for x = constant, (A1) for 2.[2 marks]x = –2 (A1) (C1)
Note: Accept –2.[1 mark]
\(y \geqslant -4{\text{ }}(f(x) \geqslant -4)\) (A1) (C1)
Notes: Accept alternative notations.
Award (A0) for use of strict inequality.[1 mark]
Question
The \(x\)-coordinate of the minimum point of the quadratic function \(f(x) = 2{x^2} + kx + 4\) is \(x =1.25\).
(i) Find the value of \(k\) .
(ii) Calculate the \(y\)-coordinate of this minimum point.[4]
Sketch the graph of \(y = f(x)\) for the domain \( – 1 \leqslant x \leqslant 3\).
[2]
Answer/Explanation
Markscheme
(i) \(1.25 = – \frac{k}{{2(2)}}\) (M1)
OR
\(f'(x) = 4x + k = 0\) (M1)
Note: Award (M1) for setting the gradient function to zero.
\(k = – 5\) (A1) (C2)
(ii) \(2{(1.25)^2} – 5(1.25) + 4\) (M1)
\( = 0.875\) (A1)(ft) (C2)
Note: Follow through from their \(k\).[4 marks]
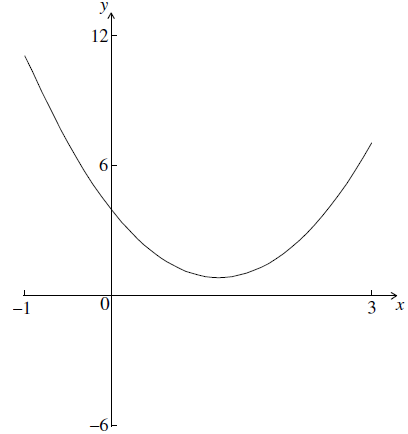(A1)(ft)(A1)(ft) (C2)
Notes: Award (A1)(ft) for a curve with correct concavity consistent with their \(k\) passing through (0, 4).
(A1)(ft) for minimum in approximately the correct place. Follow through from their part (a).[2 marks]
Question
A function f (x) = p×2x + q is defined by the mapping diagram below.
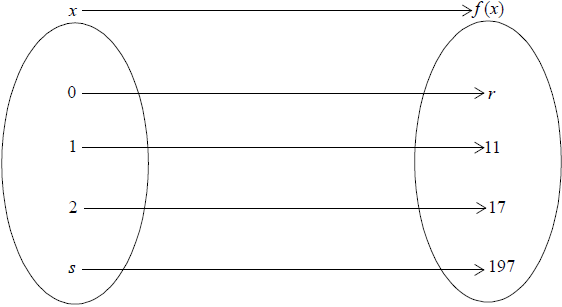
Find the value of
(i) p ;
(ii) q .[3]
Write down the value of r .[1]
Find the value of s .[2]
Answer/Explanation
Markscheme
(i) 2p + q = 11 and 4p + q = 17 (M1)
Note: Award (M1) for either two correct equations or a correct equation in one unknown equivalent to 2p = 6 .
p = 3 (A1)
(ii) q = 5 (A1) (C3)
Notes: If only one value of p and q is correct and no working shown, award (M0)(A1)(A0).[3 marks]
r = 8 (A1)(ft) (C1)
Note: Follow through from their answers for p and q irrespective of whether working is seen.[1 mark]
3 × 2s + 5 = 197 (M1)
Note: Award (M1) for setting the correct equation.
s = 6 (A1)(ft) (C2)
Note: Follow through from their values of p and q.[2 marks]
Question
Part of the graph of the quadratic function f is given in the diagram below.
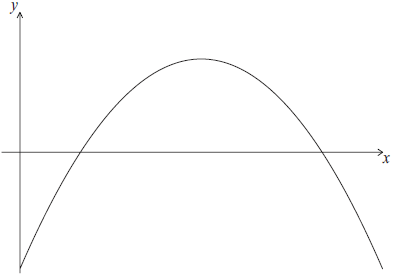
On this graph one of the x-intercepts is the point (5, 0). The x-coordinate of the maximum point is 3.
The function f is given by \( f (x) = -x^2 + bx + c \), where \(b,c \in \mathbb{Z}\)
Find the value of
(i) b ;
(ii) c .[3]
The domain of f is 0 ≤ x ≤ 6.
Find the range of f .[3]
Answer/Explanation
Markscheme
(i) \(3 = \frac{{-b}}{{-2}}\) (M1)
Note: Award (M1) for correct substitution in formula.
OR
\(-1 + b + c = 0\)
\(-25+5b + c = 0\)
\(-24 + 4b = 0\) (M1)
Note: Award (M1) for setting up 2 correct simultaneous equations.
OR
\(-2x + b = 0\) (M1)
Note: Award (M1) for correct derivative of \(f (x)\) equated to zero.
\(b = 6\) (A1) (C2)
(ii) \(0 = -(5)^2 + 6 \times 5 + c\)
\(c =-5\) (A1)(ft) (C1)
Note: Follow through from their value for b.
Note: Alternatively candidates may answer part (a) using the method below, and not as two separate parts.
\( (x – 5)(-x +1)\) (M1)
\(-x^2 +6x – 5\) (A1)
\(b = 6{\text{ }} c = -5\) (A1) (C3)[3 marks]
–5 ≤ y ≤ 4 (A1)(ft)(A1)(ft)(A1) (C3)
Notes: Accept [–5, 4]. Award (A1)(ft) for –5, (A1)(ft) for 4. (A1) for inequalities in the correct direction or brackets with values in the correct order or a clear word statement of the range. Follow through from their part (a).[3 marks]
Question
Consider the quadratic function y = f (x) , where f (x) = 5 − x + ax2.
It is given that f (2) = −5 . Find the value of a .[2]
Find the equation of the axis of symmetry of the graph of y = f (x) .[2]
Write down the range of this quadratic function.[2]
Answer/Explanation
Markscheme
−5 = 5 − (2) + a(2)2 (M1)
Note: Award (M1) for correct substitution in equation.
(a =) −2 (A1) (C2)[2 marks]
\(x = – \frac{1}{4}\) (–0.25) (A1)(A1)(ft) (C2)
Notes: Follow through from their part (a). Award (A1)(A0)(ft) for “ x = constant”. Award (A0)(A1)(ft) for \(y = – \frac{1}{4}\).[2 marks]
f (x) ≤ 5.125 (A1)(A1)(ft) (C2)
Notes: Award (A1) for f (x) ≤ (accept y). Do not accept strict inequality. Award (A1)(ft) for 5.125 (accept 5.13). Accept other correct notation, for example, (−∞, 5.125]. Follow through from their answer to part (b).[2 marks]
Question
A quadratic function \(f:x \mapsto a{x^2} + b\), where \(a\) and \(b \in \mathbb{R}\) and \(x \geqslant 0\), is represented by the mapping diagram.
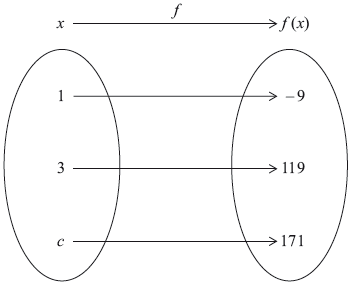
Using the mapping diagram, write down two equations in terms of \(a\) and \(b\).[2]
Solve the equations to find the value of
(i) \(a\);
(ii) \(b\).[2]
Find the value of \(c\).[2]
Answer/Explanation
Markscheme
\(a{(1)^2} + b = – 9\) (A1)
\(a{(3)^2} + b = 119\) (A1) (C2)
Note: Accept equivalent forms of the equations.[2 marks]
(i) \(a = 16\) (A1)(ft)
(ii) \(b = – 25\) (A1)(ft) (C2)
Note: Follow through from part (a) irrespective of whether working is seen.
If working is seen follow through from part (i) to part (ii).[2 marks]
\(16{c^2} – 25 = 171\) (M1)
Note: Award (M1) for correct quadratic with their \(a\) and \(b\) substituted.
\(c = 3.5\) (A1)(ft) (C2)
Note: Accept \(x\) instead of \(c\).
Follow through from part (b).
Award (A1) only, for an answer of \( \pm 3.5\) with or without working.[2 marks]
Question
Consider the functions \(f(x) = x + 1\) and \(g(x) = {3^x} – 2\).
Write down
(i) the \(x\)-intercept of the graph of \(y = {\text{ }}f(x)\);
(ii) the \(y\)-intercept of the graph of \(y = {\text{ }}g(x)\).[2]
Solve \(f(x) = g(x)\).[2]
Write down the interval for the values of \(x\) for which \(f(x) > g(x)\).[2]
Answer/Explanation
Markscheme
(i) \(( – 1,{\text{ }}0)\) (A1)
Note: Accept \( – 1\).
(ii) \((0,{\text{ }} – 1)\) (A1) (C2)
Note: Accept \( – 1\).
\((x = ){\text{ }} – 2.96{\text{ }}( – 2.96135 \ldots )\) (A1)
\((x = ){\text{ }}1.34{\text{ }}(1.33508 \ldots )\) (A1) (C2)
\( – 2.96 < x < 1.34\;\;\;{\mathbf{OR}}\;\;\;\left] { – 2.96,{\text{ }}1.34} \right[\;\;\;{\mathbf{OR}}\;\;\;( – 2.96,{\text{ }}1.34)\) (A1)(ft)(A1) (C2)
Notes: Award (A1)(ft) for both correct endpoints of the interval, (A1) for correct strict inequalities (or correct open interval notation).
Follow through from part (b).
Question
Consider the quadratic function, \(f(x) = px(q – x)\), where \(p\) and \(q\) are positive integers.
The graph of \(y = f(x)\) passes through the point \((6,{\text{ }}0)\).
Calculate the value of \(q\).[2]
The vertex of the function is \((3,{\text{ }}27)\).
Find the value of \(p\).[2]
The vertex of the function is \((3,{\text{ }}27)\).
Write down the range of \(f\).[2]
Answer/Explanation
Markscheme
\(0 = p(6)(q – 6)\) (M1)
\(q = 6\) (A1)
OR
\(f(x) = – p{x^2} + pqx\)
\(3 = \frac{{ – pq}}{{ – 2p}}\) (M1)
\(q = 6\) (A1)
OR
\(f(x) = – p{x^2} + pqx\)
\(f'(x) = pq – 2px\) (M1)
\(pq – 2p(3) = 0\)
\(q = 6\) (A1) (C2)
\(27 = p(3)(6 – 3)\) (M1)
Note: Award (M1) for correct substitution of the vertex \((3,{\text{ }}27)\) and their \(q\) into or equivalent \(f(x) = px(q – x)\) or equivalent.
\(p = 3\) (A1)(ft) (C2)
Note: Follow through from part (a).
\(y \leqslant 27\;\;\;\left( {f(x) \leqslant 27} \right)\) (A1)(A1) (C2)
Notes: Award (A1) for \(y \leqslant {\text{ }}\left( {{\text{or }}f(x) \leqslant } \right)\), (A1) for \(27\) as part of an inequality.
Accept alternative notation: \(( – \infty ,{\text{ }}27],{\text{ }}] – \infty ,{\text{ }}27]\).
Award (A0)(A1) for \([27,{\text{ }}- \infty )\).
Award (A0)(A0) for \(( – \infty ,{\text{ }}\infty )\).
Question
A building company has many rectangular construction sites, of varying widths, along a road.
The area, \(A\), of each site is given by the function
\[A(x) = x(200 – x)\]
where \(x\) is the width of the site in metres and \(20 \leqslant x \leqslant 180\).
Site S has a width of \(20\) m. Write down the area of S.[1]
Site T has the same area as site S, but a different width. Find the width of T.[2]
When the width of the construction site is \(b\) metres, the site has a maximum area.
(i) Write down the value of \(b\).
(ii) Write down the maximum area.[2]
The range of \(A(x)\) is \(m \leqslant A(x) \leqslant n\).
Hence write down the value of \(m\) and of \(n\).[1]
Answer/Explanation
Markscheme
\(3600{\text{ (}}{{\text{m}}^2})\) (A1)(C1)
\(x(200 – x) = 3600\) (M1)
Note: Award (M1) for setting up an equation, equating to their \(3600\).
\(180{\text{ (m)}}\) (A1)(ft) (C2)
Note: Follow through from their answer to part (a).
(i) \(100{\text{ (m)}}\) (A1) (C1)
(ii) \(10\,000{\text{ (}}{{\text{m}}^2})\) (A1)(ft)(C1)
Note: Follow through from their answer to part (c)(i).
\(m = 3600\;\;\;\)and\(\;\;\;n = 10\,000\) (A1)(ft) (C1)
Notes: Follow through from part (a) and part (c)(ii), but only if their \(m\) is less than their \(n\). Accept the answer \(3600 \leqslant A \leqslant 10\,000\).
Question
A population of \(200\) rabbits was introduced to an island. One week later the number of rabbits was \(210\). The number of rabbits, \(N\) , can be modelled by the function
\[N(t) = 200 \times {b^t},\,\,t \geqslant 0\,,\]
where \(t\) is the time, in weeks, since the rabbits were introduced to the island.
Find the value of \(b\) .[2]
Calculate the number of rabbits on the island after 10 weeks.[2]
An ecologist estimates that the island has enough food to support a maximum population of 1000 rabbits.
Calculate the number of weeks it takes for the rabbit population to reach this maximum.[2]
Answer/Explanation
Markscheme
\(210 = 200 \times {b^1}\) (M1)
Note: Award (M1) for correct substitution into equation.
\((b = )\,\,1.05\) (A1) (C2)
\(200 \times {1.05^{10}}\) (M1)
Note: Award (M1) for correct substitution into formula. Follow through from part (a).
\( = 325\) (A1)(ft) (C2)
Note: The answer must be an integer.
\(200 \times {1.05^t} = 1000\) (M1)
\(t = 33.0\,\,\,(32.9869…)\) (A1)(ft) (C2)
Note: Award (M1) for setting up the equation. Accept alternative methods such as \(t = \frac{{\log \,(5)}}{{\log \,(1.05)}}\) , or a sketch of \(y = 200 \times {1.05^t}\) and \(y = 1000\) with indication of point of intersection. Follow through from (a).
Question
Consider the curve \(y = 1 + \frac{1}{{2x}},\,\,x \ne 0.\)
For this curve, write down
i) the value of the \(x\)-intercept;
ii) the equation of the vertical asymptote.[3]
Sketch the curve for \( – 2 \leqslant x \leqslant 4\) on the axes below.
[3]
Answer/Explanation
Markscheme
i) \(\left( {x = } \right) – 0.5\,\,\left( { – \frac{1}{2}} \right)\) (A1)
ii) \(x = 0\) (A1)(A1) (C3)
Note: Award (A1) for “\(x = \)” and (A1) for “\(0\)” seen as part of an equation.
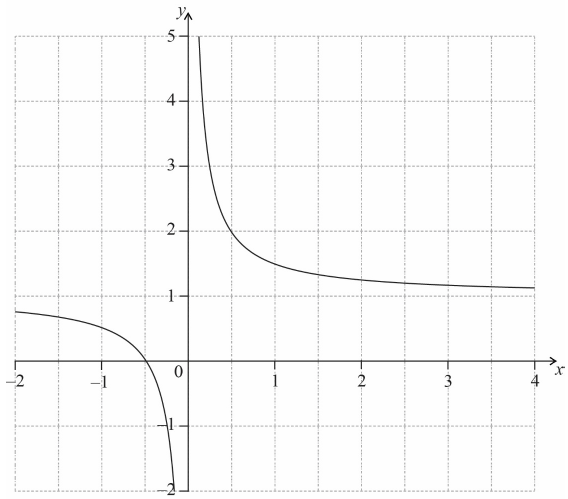
(A1)(ft)(A1)(ft)(A1) (C3)
Note: Award (A1)(ft) for correct \(x\)-intercept, (A1)(ft) for asymptotic behaviour at \(y\)-axis, (A1) for approximately correct shape (cannot intersect the horizontal asymptote of \(y = 1\)). Follow through from part (a).
Question
The graph of the quadratic function \(f(x) = c + bx – {x^2}\) intersects the \(x\)-axis at the point \({\text{A}}( – 1,{\text{ }}0)\) and has its vertex at the point \({\text{B}}(3,{\text{ }}16)\).
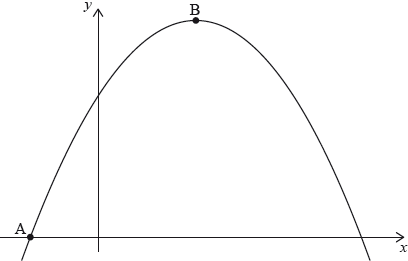
Write down the equation of the axis of symmetry for this graph.[2]
Find the value of \(b\).[2]
Write down the range of \(f(x)\).[2]
Answer/Explanation
Markscheme
\(x = 3\) (A1)(A1) (C2)
Note: Award (A1) for \(x = \) constant, (A1) for the constant being 3.
The answer must be an equation.[2 marks]
\(\frac{{ – b}}{{2( – 1)}} = 3\) (M1)
Note: Award (M1) for correct substitution into axis of symmetry formula.
OR
\(b – 2x = 0\) (M1)
Note: Award (M1) for correctly differentiating and equating to zero.
OR
\(c + b( – 1) – {( – 1)^2} = 0\) (or equivalent)
\(c + b(3) – {(3)^2} = 16\) (or equivalent) (M1)
Note: Award (M1) for correct substitution of \(( – 1,{\text{ }}0)\) and \((3,{\text{ }}16)\) in the original quadratic function.
\((b = ){\text{ }}6\) (A1)(ft) (C2)
Note: Follow through from part (a).[2 marks]
\(( – \infty ,{\text{ 16]}}\) OR \(] – \infty ,{\text{ }}16]\) (A1)(A1)
Note: Award (A1) for two correct interval endpoints, (A1) for left endpoint excluded and right endpoint included.[2 marks]
Question
The function \(f\) is of the form \(f(x) = ax + b + \frac{c}{x}\), where \(a\) , \(b\) and \(c\) are positive integers.
Part of the graph of \(y = f(x)\) is shown on the axes below. The graph of the function has its local maximum at \(( – 2,{\text{ }} – 2)\) and its local minimum at \((2,{\text{ }}6)\).
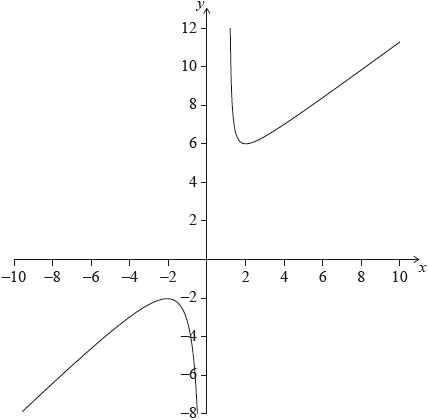
Write down the domain of the function.[2]
Draw the line \(y = – 6\) on the axes.[1]
Write down the number of solutions to \(f(x) = – 6\).[1]
Find the range of values of \(k\) for which \(f(x) = k\) has no solution.[2]
Answer/Explanation
Markscheme
\((x \in \mathbb{R}),{\text{ }}x \ne 0\) (A2) (C2)
Note: Accept equivalent notation. Award (A1)(A0) for \(y \ne 0\).
Award (A1) for a clear statement that demonstrates understanding of the meaning of domain. For example, \({\text{D}}:( – \infty ,{\text{ }}0) \cup (1,{\text{ }}\infty )\) should be awarded (A1)(A0).[2 marks]
(A1) (C1)
Note: The command term “Draw” states: “A ruler (straight edge) should be used for straight lines”; do not accept a freehand \(y = – 6\) line.[1 mark]
2 (A1)(ft) (C1)
Note: Follow through from part (b)(i).[1 mark]
\( – 2 < k < 6\) (A1)(A1) (C2)
Note: Award (A1) for both end points correct and (A1) for correct strict inequalities.
Award at most (A1)(A0) if the stated variable is different from \(k\) or \(y\) for example \( – 2 < x < 6\) is (A1)(A0).[2 marks]
Question
Jashanti is saving money to buy a car. The price of the car, in US Dollars (USD), can be modelled by the equation
\[P = 8500{\text{ }}{(0.95)^t}.\]
Jashanti’s savings, in USD, can be modelled by the equation
\[S = 400t + 2000.\]
In both equations \(t\) is the time in months since Jashanti started saving for the car.
Jashanti does not want to wait too long and wants to buy the car two months after she started saving. She decides to ask her parents for the extra money that she needs.
Write down the amount of money Jashanti saves per month.[1]
Use your graphic display calculator to find how long it will take for Jashanti to have saved enough money to buy the car.[2]
Calculate how much extra money Jashanti needs.[3]
Answer/Explanation
Markscheme
400 (USD) (A1) (C1)[1 mark]
\(8500{\text{ }}{(0.95)^t} = 400 \times t + 2000\) (M1)
Note: Award (M1) for equating \(8500{(0.95)^t}\) to \(400 \times t + 2000\) or for comparing the difference between the two expressions to zero or for showing a sketch of both functions.
\((t = ){\text{ }}8.64{\text{ (months) }}\left( {8.6414 \ldots {\text{ (months)}}} \right)\) (A1) (C2)
Note: Accept 9 months.[2 marks]
\(8500{(0.95)^2} – (400 \times 2 + 2000)\) (M1)(M1)
Note: Award (M1) for correct substitution of \(t = 2\) into equation for \(P\), (M1) for finding the difference between a value/expression for \(P\) and a value/expression for \(S\). The first (M1) is implied if 7671.25 seen.
4870 (USD) (4871.25) (A1) (C3)
Note: Accept 4871.3.[3 marks]
Question
Consider the functions \(f\left( x \right) = {x^4} – 2\) and \(g\left( x \right) = {x^3} – 4{x^2} + 2x + 6\)
The functions intersect at points P and Q. Part of the graph of \(y = f\left( x \right)\) and part of the graph of \(y = g\left( x \right)\) are shown on the diagram.
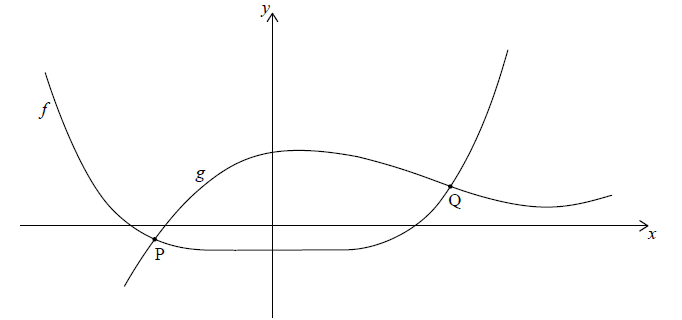
Find the range of f.[2]
Write down the x-coordinate of P and the x-coordinate of Q.[2]
Write down the values of x for which \(f\left( x \right) > g\left( x \right)\).[2]
Answer/Explanation
Markscheme
\(\left[ { – 2,\,\,\infty } \right[{\text{ or }}\left[ { – 2,\,\,\infty } \right)\) OR \(f\left( x \right) \geqslant – 2{\text{ or }}y \geqslant – 2\) OR \( – 2 \leqslant f\left( x \right) < \infty \) (A1)(A1) (C2)
Note: Award (A1) for −2 and (A1) for completely correct mathematical notation, including weak inequalities. Accept \(f \geqslant – 2\).[2 marks]
–1 and 1.52 (1.51839…) (A1)(A1) (C2)
Note: Award (A1) for −1 and (A1) for 1.52 (1.51839).[2 marks]
\(x < – 1,\,\,\,x > 1.52\) OR \(\left( { – \infty ,\,\, – 1} \right) \cup \left( {1.52,\,\,\infty } \right)\). (A1)(ft)(A1)(ft) (C2)
Note: Award (A1)(ft) for both critical values in inequality or range statements such as \(x < – 1,\,\,\left( { – \infty ,\,\, – 1} \right),\,\,x > 1.52\,{\text{ or }}\left( {1.52,\,\,\infty } \right)\).
Award the second (A1)(ft) for correct strict inequality statements used with their critical values. If an incorrect use of strict and weak inequalities has already been penalized in (a), condone weak inequalities for this second mark and award (A1)(ft).[2 marks]
Question
On the grid below sketch the graph of the function \(f(x) = 2{(1.6)^x}\) for the domain \(0 \leqslant x \leqslant 3\) .
[2]
Write down the coordinates of the \(y\)-intercept of the graph of \(y = f(x)\) .[1]
On the grid draw the graph of the function \(g(x) = 5 – 2x\) for the domain \(0 \leqslant x \leqslant 3\).[2]
Use your graphic display calculator to solve \(f(x) = g(x)\) .[1]
Answer/Explanation
Markscheme
Note: Award (A1) correct endpoints, (A1) for smooth curve. (A1)(A1) (C2)
\((0{\text{, }}2)\) (A1) (C1)
Note: Accept \(x = 0\), \(y = 2\)
Straight line in the given domain (A1)
Axes intercepts in the correct positions (A1) (C2)
\(x = 0.943\) (\(0.94259 \ldots \)) (A1) (C1)
Note: Award (A0) if \(y\)-coordinate given.