Question
The following curves are sketches of the graphs of the functions given below, but in a different order. Using your graphic display calculator, match the equations to the curves, writing your answers in the table below.
(the diagrams are not to scale)
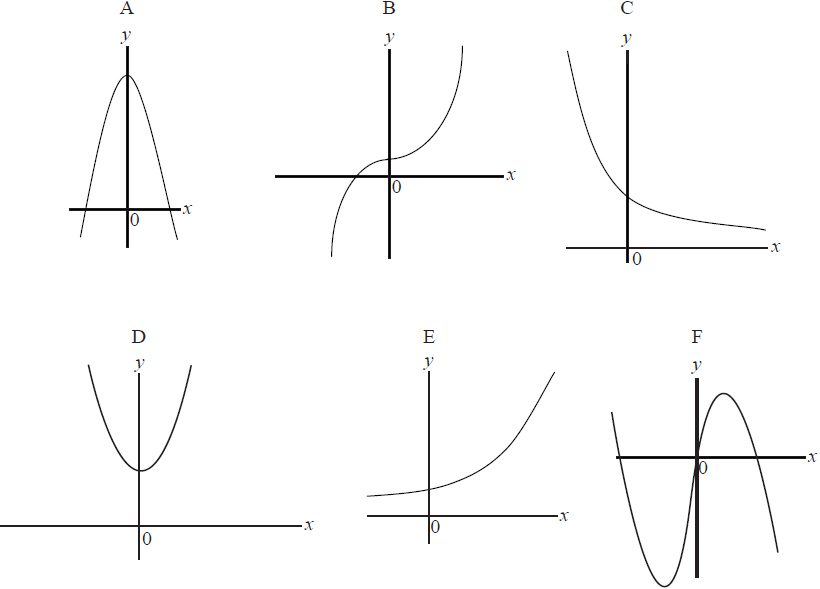
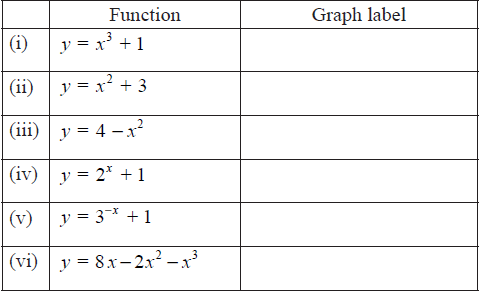
Answer/Explanation
Markscheme
(i) B (A1)
(ii) D (A1)
(iii) A (A1)
(iv) E (A1)
(v) C (A1)
(vi) F (A1) (C6)[6 marks]
Question
The straight line, L, has equation \(2y – 27x – 9 = 0\).
Find the gradient of L.[2]
Sarah wishes to draw the tangent to \(f (x) = x^4\) parallel to L.
Write down \(f ′(x)\).[1]
Find the x coordinate of the point at which the tangent must be drawn.[2]
Write down the value of \(f (x)\) at this point.[1]
Answer/Explanation
Markscheme
y = 13.5x + 4.5 (M1)
Note: Award (M1) for 13.5x seen.
gradient = 13.5 (A1) (C2)[2 marks]
4x3 (A1) (C1)[1 mark]
4x3 = 13.5 (M1)
Note: Award (M1) for equating their answers to (a) and (b).
x = 1.5 (A1)(ft)[2 marks]
\(\frac{{81}}{{16}}\) (5.0625, 5.06) (A1)(ft) (C3)
Note: Award (A1)(ft) for substitution of their (c)(i) into x4 with working seen.[1 mark]
Question
Consider the curve \(y = 1 + \frac{1}{{2x}},\,\,x \ne 0.\)
For this curve, write down
i) the value of the \(x\)-intercept;
ii) the equation of the vertical asymptote.[3]
Sketch the curve for \( – 2 \leqslant x \leqslant 4\) on the axes below.
 [3]
[3]
Answer/Explanation
Markscheme
i) \(\left( {x = } \right) – 0.5\,\,\left( { – \frac{1}{2}} \right)\) (A1)
ii) \(x = 0\) (A1)(A1) (C3)
Note: Award (A1) for “\(x = \)” and (A1) for “\(0\)” seen as part of an equation.
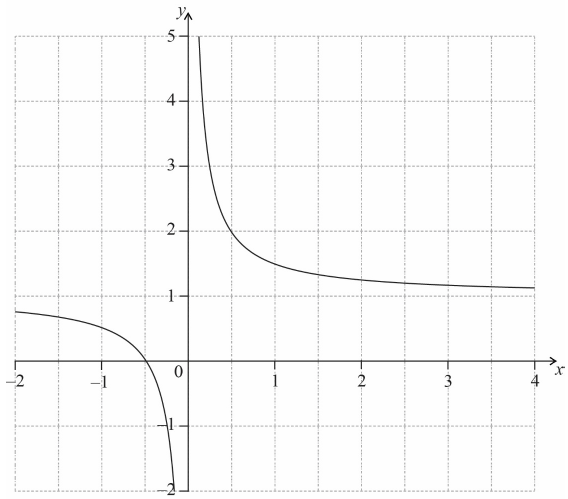
(A1)(ft)(A1)(ft)(A1) (C3)
Note: Award (A1)(ft) for correct \(x\)-intercept, (A1)(ft) for asymptotic behaviour at \(y\)-axis, (A1) for approximately correct shape (cannot intersect the horizontal asymptote of \(y = 1\)). Follow through from part (a).
Question
A function \(f\) is given by \(f(x) = 4{x^3} + \frac{3}{{{x^2}}} – 3,{\text{ }}x \ne 0\).
Write down the derivative of \(f\).[3]
Find the point on the graph of \(f\) at which the gradient of the tangent is equal to 6.[3]
Answer/Explanation
Markscheme
\(12{x^2} – \frac{6}{{{x^3}}}\) or equivalent (A1)(A1)(A1) (C3)
Note: Award (A1) for \(12{x^2}\), (A1) for \( – 6\) and (A1) for \(\frac{1}{{{x^3}}}\) or \({x^{ – 3}}\). Award at most (A1)(A1)(A0) if additional terms seen.[3 marks]
\(12{x^2} – \frac{6}{{{x^3}}} = 6\) (M1)
Note: Award (M1) for equating their derivative to 6.
\((1,{\text{ }}4)\)\(\,\,\,\)OR\(\,\,\,\)\(x = 1,{\text{ }}y = 4\) (A1)(ft)(A1)(ft) (C3)
Note: A frequent wrong answer seen in scripts is \((1,{\text{ }}6)\) for this answer with correct working award (M1)(A0)(A1) and if there is no working award (C1).[3 marks]
Question
Consider the functions \(f\left( x \right) = {x^4} – 2\) and \(g\left( x \right) = {x^3} – 4{x^2} + 2x + 6\)
The functions intersect at points P and Q. Part of the graph of \(y = f\left( x \right)\) and part of the graph of \(y = g\left( x \right)\) are shown on the diagram.
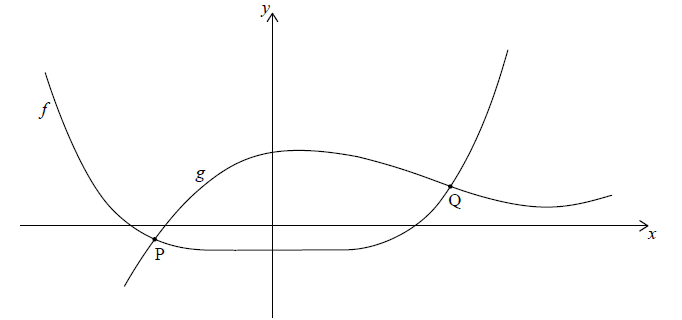
Find the range of f.[2]
Write down the x-coordinate of P and the x-coordinate of Q.[2]
Write down the values of x for which \(f\left( x \right) > g\left( x \right)\).[2]
Answer/Explanation
Markscheme
\(\left[ { – 2,\,\,\infty } \right[{\text{ or }}\left[ { – 2,\,\,\infty } \right)\) OR \(f\left( x \right) \geqslant – 2{\text{ or }}y \geqslant – 2\) OR \( – 2 \leqslant f\left( x \right) < \infty \) (A1)(A1) (C2)
Note: Award (A1) for −2 and (A1) for completely correct mathematical notation, including weak inequalities. Accept \(f \geqslant – 2\).[2 marks]
–1 and 1.52 (1.51839…) (A1)(A1) (C2)
Note: Award (A1) for −1 and (A1) for 1.52 (1.51839).[2 marks]
\(x < – 1,\,\,\,x > 1.52\) OR \(\left( { – \infty ,\,\, – 1} \right) \cup \left( {1.52,\,\,\infty } \right)\). (A1)(ft)(A1)(ft) (C2)
Note: Award (A1)(ft) for both critical values in inequality or range statements such as \(x < – 1,\,\,\left( { – \infty ,\,\, – 1} \right),\,\,x > 1.52\,{\text{ or }}\left( {1.52,\,\,\infty } \right)\).
Award the second (A1)(ft) for correct strict inequality statements used with their critical values. If an incorrect use of strict and weak inequalities has already been penalized in (a), condone weak inequalities for this second mark and award (A1)(ft).[2 marks]
Question
Consider the graph of the function \(f\left( x \right) = \frac{3}{x} – 2,\,\,\,x \ne 0\).
Write down the equation of the vertical asymptote.[2]
Write down the equation of the horizontal asymptote.[2]
Calculate the value of x for which f(x) = 0 .[2]
Answer/Explanation
Markscheme
x = 0 (A1)(A1) (C2)
Note: Award (A1) for x = “a constant” (A1) for = 0. Award (A0)(A0) for an answer of “0”.[2 marks]
f(x) = −2 (y = −2) (A1)(A1) (C2)
Note: Award (A1) for y = “a constant” (A1) for = −2. Award (A0)(A0) for an answer of “−2”.[2 marks]
\(\frac{3}{x} – 2 = 0\) (M1)
Note: Award (M1) for equating f(x) to 0.
\(\left( {x = } \right)\frac{3}{2}\,\,\,\,\,\left( {1.5} \right)\) (A1) (C2)[2 marks]

