Question
In an experiment it is found that a culture of bacteria triples in number every four hours. There are \(200\) bacteria at the start of the experiment.

Find the value of \(a\).[1]
Calculate how many bacteria there will be after one day.[2]
Find how long it will take for there to be two million bacteria.[3]
Answer/Explanation
Markscheme
\(a = 1800\) (A1) (C1)[1 mark]
\(200 \times {3^6}{\text{ (or }}16200 \times 9{\text{) }} = 145800\) (M1)(A1) (C2)[2 marks]
\(200 \times {3^n} = 2 \times {10^6}\) (where \(n\) is each \(4\) hour interval) (M1)
Note: Award (M1) for attempting to set up the equation or writing a list of numbers.
\({3^n} = {10^4}\)
\(n = 8.38{\text{ }}(8.383613097)\) correct answer only (A1)
\({\text{Time}} = 33.5{\text{ hours}}\) (accept \(34\), \(35\) or \(36\) if previous A mark awarded) (A1)(ft) (C3)
Note: (A1)(ft) for correctly multiplying their answer by \(4\). If \(34\), \(35\) or \(36\) seen, or \(32 – 36\) seen, award (M1)(A0)(A0).[3 marks]
Question
Given the function \(f (x) = 2 \times 3^x\) for −2 \( \leqslant \) x \( \leqslant \) 5,
find the range of \(f\).[4]
find the value of \(x\) given that \(f (x) =162\).[2]
Answer/Explanation
Markscheme
\(f (-2) = 2 \times 3^{-2}\) (M1)
\(= \frac{{2}}{{9}}(0.222)\) (A1)
\(f (5) = 2 \times 3^5\)
\(= 486\) (A1)
\({\text{Range }}\frac{2}{9} \leqslant f(x) \leqslant 486\) OR \(\left[ {\frac{2}{9},{\text{ }}486} \right]\) (A1) (C4)
Note: Award (M1) for correct substitution of –2 or 5 into \(f (x)\), (A1)(A1) for each correct end point.[4 marks]
\(2 \times 3^x = 162\) (M1)
\(x = 4\) (A1) (C2)[2 marks]
Question
The number of bacteria in a colony is modelled by the function
\(N(t) = 800 \times 3^{0.5t}, {\text{ }} t \geqslant 0\),
where \(N\) is the number of bacteria and \(t\) is the time in hours.
Write down the number of bacteria in the colony at time \(t = 0\).[1]
Calculate the number of bacteria present at 2 hours and 30 minutes. Give your answer correct to the nearest hundred bacteria.[3]
Calculate the time, in hours, for the number of bacteria to reach 5500.[2]
Answer/Explanation
Markscheme
800 (A1) (C1)
\(800 \times {3^{(0.5 \times 2.5)}}\) (M1)
Note: Award (M1) for correctly substituted formula.
\( = 3158.57\)… (A1)
\(= 3200\) (A1) (C3)
Notes: Final (A1) is given for correctly rounding their answer. This may be awarded regardless of a preceding (A0).
\(5500 = 800 \times {3^{(0.5 \times t)}}\) (M1)
Notes: Award (M1) for equating function to 5500. Accept correct alternative methods.
\(= 3.51{\text{ hours}}\) (3.50968…) (A1) (C2)
Question
Consider the two functions, \(f\) and \(g\), where
\(f(x) = \frac{5}{{{x^2} + 1}}\)
\(g(x) = {(x – 2)^2}\)
Sketch the graphs of \(y = f(x)\) and \(y = g(x)\) on the axes below. Indicate clearly the points where each graph intersects the y-axis.
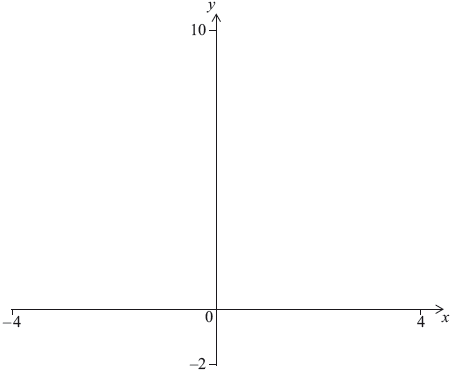 [4]
[4]Use your graphic display calculator to solve \(f(x) = g(x)\).[2]
Answer/Explanation
Markscheme
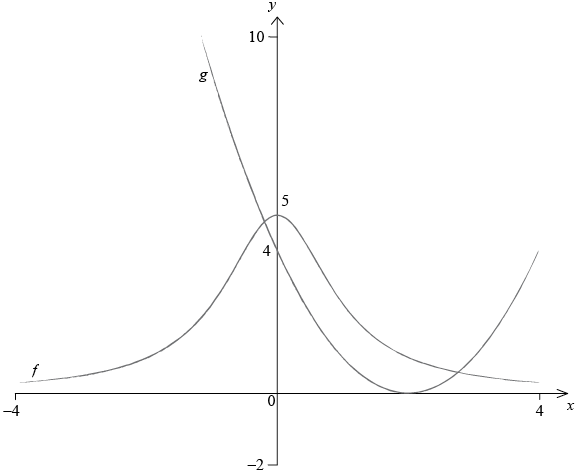
\(f(x)\): a smooth curve symmetrical about y-axis, \(f(x) > 0\) (A1)
Note: If the graph crosses the x-axis award (A0).
Intercept at their numbered \(y = 5\) (A1)
Note: Accept clear scale marks instead of a number.
\(g(x)\): a smooth parabola with axis of symmetry at about \(x = 2\) (the 2 does not need to be numbered) and \(g(x) \geqslant 0\) (A1)
Note: Right hand side must not be higher than the maximum of \(f(x)\) at \(x = 4\).
Accept the quadratic correctly drawn beyond \(x = 4\).
Intercept at their numbered \(y = 4\) (A1) (C4)
Note: Accept clear scale marks instead of a number.[4 marks]
\(–0.195, 2.76\) \((–0.194808…, 2.761377…)\) (A1)(ft)(A1)(ft) (C2)
Note: Award (A0)(A1)(ft) if both coordinates are given.
Follow through only if \(f(x) = \frac{5}{{{x^2}}} + 1\) is sketched; the solutions are \(–0.841, 3.22\) \((–0.840913…, 3.217747…)\)[2 marks]
Question
The amount of electrical charge, C, stored in a mobile phone battery is modelled by \(C(t) = 2.5 – {2^{ – t}}\), where t, in hours, is the time for which the battery is being charged.
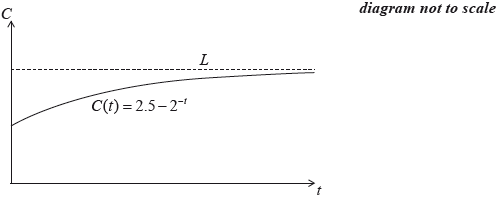
Write down the amount of electrical charge in the battery at \(t = 0\).[1]
The line \(L\) is the horizontal asymptote to the graph.
Write down the equation of \(L\).[2]
To download a game to the mobile phone, an electrical charge of 2.4 units is needed.
Find the time taken to reach this charge. Give your answer correct to the nearest minute.[3]
Answer/Explanation
Markscheme
\(1.5\) (A1) (C1)[1 mark]
\(C = 2.5\) (accept \(y = 2.5\)) (A1)(A1) (C2)
Notes: Award (A1) for \(C{\text{ (or }}y) = \) a positive constant, (A1) for the constant \(= 2.5\).
Answer must be an equation.[2 marks]
\(2.4 = 2.5 – {2^{ – t}}\) (M1)
Note: Award (M1) for setting the equation equal to 2.4 or for a horizontal line drawn at approximately \(C = 2.4\).
Allow \(x\) instead of \(t\).
OR
\( – t\ln (2) = \ln (0.1)\) (M1)
\(t = 3.32192 \ldots \) (A1)
\(t = 3{\text{ hours and 19 minutes (199 minutes)}}\) (A1)(ft) (C3)
Note: Award the final (A1)(ft) for correct conversion of their time in hours to the nearest minute.[3 marks]
Question
In a trial for a new drug, scientists found that the amount of the drug in the bloodstream decreased over time, according to the model
\[D(t) = 1.2 \times {(0.87)^t},{\text{ }}t \geqslant 0\]
where \(D\) is the amount of the drug in the bloodstream in mg per litre \({\text{(mg}}\,{{\text{l}}^{ – 1}}{\text{)}}\) and \(t\) is the time in hours.
Write down the amount of the drug in the bloodstream at \(t = 0\).[1]
Calculate the amount of the drug in the bloodstream after 3 hours.[2]
Use your graphic display calculator to determine the time it takes for the amount of the drug in the bloodstream to decrease to \(0.333{\text{ mg}}{{\text{1}}^{ – 1}}\).[3]
Answer/Explanation
Markscheme
\(1.2{\text{ (mg}}\,{{\text{l}}^{ – 1}}{\text{)}}\) (A1) (C1)[1 mark]
\(1.2 \times {(0.87)^3}\) (M1)
Note: Award (M1) for correct substitution into given formula.
\( = {\text{0.790 (mg}}\,{{\text{l}}^{ – 1}}{\text{) (0.790203}} \ldots {\text{)}}\) (A1) (C2)[2 marks]
\(1.2 \times {0.87^t} = 0.333\) (M1)
Note: Award (M1) for setting up the equation. (M1)
(M1)
Notes: Some indication of scale is to be shown, for example the window used on the calculator.
Accept alternative methods.
\(9.21\) (hours) (\(9.20519…\), 9 hours 12 minutes, 9:12) (A1) (C3)[3 marks]
Question
A hotel has a rectangular swimming pool. Its length is \(x\) metres, its width is \(y\) metres and its perimeter is \(44\) metres.
Write down an equation for \(x\) and \(y\).[1]
The area of the swimming pool is \({\text{112}}{{\text{m}}^2}\).
Write down a second equation for \(x\) and \(y\).[1]
Use your graphic display calculator to find the value of \(x\) and the value of \(y\).[2]
An Olympic sized swimming pool is \(50\) m long and \(25\) m wide.
Determine the area of the hotel swimming pool as a percentage of the area of an Olympic sized swimming pool.[2]
Answer/Explanation
Markscheme
\(2x + 2y = 44\) (A1) (C1)
Note: Accept equivalent forms.
\(xy = 112\) (A1) (C1)
\(8\), \(14\) (A1)(ft)(A1)(ft) (C2)
Notes: Accept \(x = 8\), \(y = 14\) OR \(x = 14\), \(y = 8\)
Follow through from their answers to parts (a) and (b) only if both values are positive.
\(\frac{{112}}{{1250}} \times 100\) (M1)
Note: Award (M1) for \(112\) divided by \(1250\).
\( = 8.96\) (A1) (C2)
Note: Do not penalize if percentage sign seen.
Question
Consider the functions \(f(x) = x + 1\) and \(g(x) = {3^x} – 2\).
Write down
(i) the \(x\)-intercept of the graph of \(y = {\text{ }}f(x)\);
(ii) the \(y\)-intercept of the graph of \(y = {\text{ }}g(x)\).[2]
Solve \(f(x) = g(x)\).[2]
Write down the interval for the values of \(x\) for which \(f(x) > g(x)\).[2]
Answer/Explanation
Markscheme
(i) \(( – 1,{\text{ }}0)\) (A1)
Note: Accept \( – 1\).
(ii) \((0,{\text{ }} – 1)\) (A1) (C2)
Note: Accept \( – 1\).
\((x = ){\text{ }} – 2.96{\text{ }}( – 2.96135 \ldots )\) (A1)
\((x = ){\text{ }}1.34{\text{ }}(1.33508 \ldots )\) (A1) (C2)
\( – 2.96 < x < 1.34\;\;\;{\mathbf{OR}}\;\;\;\left] { – 2.96,{\text{ }}1.34} \right[\;\;\;{\mathbf{OR}}\;\;\;( – 2.96,{\text{ }}1.34)\) (A1)(ft)(A1) (C2)
Notes: Award (A1)(ft) for both correct endpoints of the interval, (A1) for correct strict inequalities (or correct open interval notation).
Follow through from part (b).
Question
The golden ratio, \(r\) , was considered by the Ancient Greeks to be the perfect ratio between the lengths of two adjacent sides of a rectangle. The exact value of \(r\) is \(\frac{{1 + \sqrt 5 }}{2}\).
Write down the value of \(r\)
i) correct to \(5\) significant figures;
ii) correct to \(2\) decimal places.[2]
Phidias is designing rectangular windows with adjacent sides of length \(x\) metres and \(y\) metres. The area of each window is \(1\,{{\text{m}}^2}\).
Write down an equation to describe this information.[1]
Phidias designs the windows so that the ratio between the longer side, \(y\) , and the shorter side, \(x\) , is the golden ratio, \(r\).
Write down an equation in \(y\) , \(x\) and \(r\) to describe this information.[1]
Find the value of \(x\) .[2]
Answer/Explanation
Markscheme
i) \(1.6180\) (A1)
ii) \(1.62\) (A1)(ft) (C2)
Note: Follow through from part (a)(i).
\(xy = 1\) (A1) (C1)
\(\frac{y}{x} = r\) OR \(\frac{y}{x} = \frac{{1 + \sqrt 5 }}{2}\) OR equivalent (A1) (C1)
Note: Accept \(\frac{y}{x} = \) their part (a)(i) or (a)(ii).
\({x^2}r = 1\) or eqivalent (M1)
\(x = 0.786\,\,\,(0.78615…)\) (A1)(ft) (C2)
Note: Award (M1) for substituting their part (c) into their equation from part (b). Follow through from parts (a), (b) and (c). Use of \(r = 1.62\) gives \(0.785674…\)
Question
A population of \(200\) rabbits was introduced to an island. One week later the number of rabbits was \(210\). The number of rabbits, \(N\) , can be modelled by the function
\[N(t) = 200 \times {b^t},\,\,t \geqslant 0\,,\]
where \(t\) is the time, in weeks, since the rabbits were introduced to the island.
Find the value of \(b\) .[2]
Calculate the number of rabbits on the island after 10 weeks.[2]
An ecologist estimates that the island has enough food to support a maximum population of 1000 rabbits.
Calculate the number of weeks it takes for the rabbit population to reach this maximum.[2]
Answer/Explanation
Markscheme
\(210 = 200 \times {b^1}\) (M1)
Note: Award (M1) for correct substitution into equation.
\((b = )\,\,1.05\) (A1) (C2)
\(200 \times {1.05^{10}}\) (M1)
Note: Award (M1) for correct substitution into formula. Follow through from part (a).
\( = 325\) (A1)(ft) (C2)
Note: The answer must be an integer.
\(200 \times {1.05^t} = 1000\) (M1)
\(t = 33.0\,\,\,(32.9869…)\) (A1)(ft) (C2)
Note: Award (M1) for setting up the equation. Accept alternative methods such as \(t = \frac{{\log \,(5)}}{{\log \,(1.05)}}\) , or a sketch of \(y = 200 \times {1.05^t}\) and \(y = 1000\) with indication of point of intersection. Follow through from (a).
Question
A company sells fruit juices in cylindrical cans, each of which has a volume of \(340\,{\text{c}}{{\text{m}}^3}\). The surface area of a can is \(A\,{\text{c}}{{\text{m}}^2}\) and is given by the formula
\(A = 2\pi {r^2} + \frac{{680}}{r}\) ,
where \(r\) is the radius of the can, in \({\text{cm}}\).
To reduce the cost of a can, its surface area must be minimized.
Find \(\frac{{{\text{d}}A}}{{{\text{d}}r}}\)[3]
Calculate the value of \(r\) that minimizes the surface area of a can.[3]
Answer/Explanation
Markscheme
\(\left( {\frac{{{\text{d}}A}}{{{\text{d}}r}}} \right) = 4\pi r – \frac{{680}}{{{r^2}}}\) (A1)(A1)(A1) (C3)
Note: Award (A1) for \(4\pi r\) (accept \(12.6r\)), (A1) for \( – 680\), (A1) for \(\frac{1}{{{r^2}}}\) or \({r^{ – 2}}\)
Award at most (A1)(A1)(A0) if additional terms are seen.
\(4\pi r – \frac{{680}}{{{r^2}}} = 0\) (M1)
Note: Award (M1) for equating their \(\frac{{{\text{d}}A}}{{{\text{d}}r}}\) to zero.
\(4\pi {r^3} – 680 = 0\) (M1)
Note: Award (M1) for initial correct rearrangement of the equation. This may be assumed if \({r^3} = \frac{{680}}{{4\pi }}\) or \(r = \sqrt[3]{{\frac{{680}}{{4\pi }}}}\) seen.
OR
sketch of \(A\) with some indication of minimum point (M1)(M1)
Note: Award (M1) for sketch of \(A\), (M1) for indication of minimum point.
OR
sketch of \(\frac{{{\text{d}}A}}{{{\text{d}}r}}\) with some indication of zero (M1)(M1)
Note: Award (M1) for sketch of \(\frac{{{\text{d}}A}}{{{\text{d}}r}}\), (M1) for indication of zero.
\((r = )\,\,3.78\,({\text{cm}})\,\,\,\,\,(3.78239…)\) (A1)(ft) (C3)
Note: Follow through from part (a).
Question
A population of mosquitoes decreases exponentially. The size of the population, \(P\) , after \(t\) days is modelled by
\(P = 3200 \times {2^{ – t}} + 50\) , where \(t \geqslant 0\) .
Write down the exact size of the initial population.[1]
Find the size of the population after \(4\) days.[2]
Calculate the time it will take for the size of the population to decrease to \(60\).[2]
The population will stabilize when it reaches a size of \(k\) .
Write down the value of \(k\) .[1]
Answer/Explanation
Markscheme
\(3250\) (A1) (C1)
\(3200 \times {2^{ – 4}} + 50\) (M1)
Note: Award (M1) for substituting \(t\) into exponential equation.
\( = 250\) (A1) (C2)
\(3200 \times {2^{ – t}} + 50 = 60\) (M1)
Note: Award (M1) for setting up the equation used in part (b).
OR
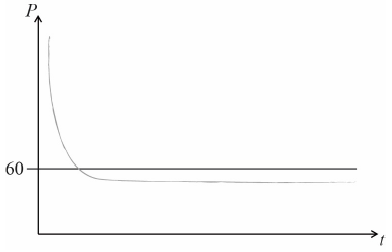
(M1)
Note: Award (M1) for a decreasing exponential graph intersecting a horizontal line.
\((t = )\,\,8.32\,\,(8.32192…)\) (days) (A1) (C2)
Note: Accept a final answer of “\(8\) days, \(7\) hours and \(44\) minutes”, or equivalent. Award (M0)(A0) for an answer of \(8\) days with no working
\(50\) (A1) (C1)
Question
Consider the function \(f(x) = {x^3} – 3{x^2} + 2x + 2\) . Part of the graph of \(f\) is shown below.
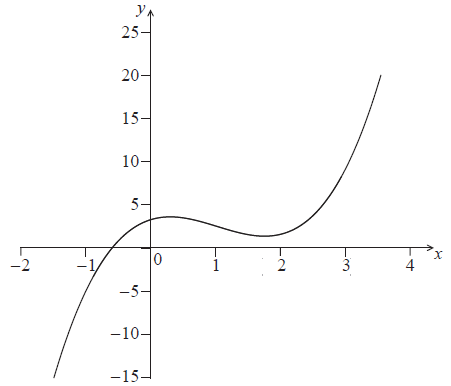
Find \(f'(x)\) .[3]
There are two points at which the gradient of the graph of \(f\) is \(11\). Find the \(x\)-coordinates of these points.[3]
Answer/Explanation
Markscheme
\((f'(x) = )\,\,3{x^2} – 6x + 2\) (A1)(A1)(A1) (C3)
Note: Award (A1) for \(3{x^2}\), (A1) for \( – 6x\) and (A1) for \( + 2\).
Award at most (A1)(A1)(A0) if there are extra terms present.
\(11 = 3{x^2} – 6x + 2\) (M1)
Note: Award (M1) for equating their answer from part (a) to \(11\), this may be implied from \(0 = 3{x^2} – 6x – 9\) .
\((x = )\,\, – 1\,\,,\,\,\,\,(x = )\,\,3\) (A1)(ft)(A1)(ft) (C3)
Note: Follow through from part (a).
If final answer is given as coordinates, award at most (M1)(A0)(A1)(ft) for \(( – 1,\,\, – 4)\) and \((3,\,\,8)\) .
Question
Gabriella purchases a new car.
The car’s value in dollars, \(V\), is modelled by the function
\[V(t) = 12870 – k{(1.1)^t},{\text{ }}t \geqslant 0\]
where \(t\) is the number of years since the car was purchased and \(k\) is a constant.
After two years, the car’s value is $9143.20.
This model is defined for \(0 \leqslant t \leqslant n\). At \(n\) years the car’s value will be zero dollars.
Write down, and simplify, an expression for the car’s value when Gabriella purchased it.[2]
Find the value of \(k\).[2]
Find the value of \(n\).[2]
Answer/Explanation
Markscheme
\(12870 – k{(1.1)^0}\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
\( = 12870 – k\) (A1) (C2)
Note: Accept \(12870 – 3080\) OR 9790 for a final answer.[2 marks]
\(9143.20 = 12870 – k{(1.1)^2}\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
\((k = ){\text{ }}3080\) (A1) (C2)[2 marks]
\(12870 – 3080{(1.1)^n} = 0\) (M1)
Note: Award (M1) for correct substitution into \(V(t)\).
OR
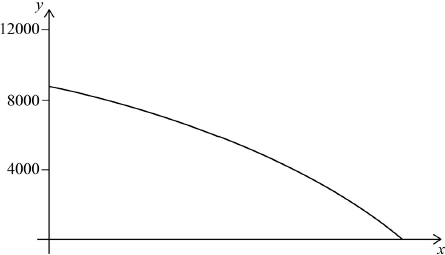 (M1)
(M1)
Note: Award (M1) for a correctly shaped curve with some indication of scale on the vertical axis.
\((n = ){\text{ }}15.0{\text{ }}(15.0033 \ldots )\) (A1)(ft) (C2)
Note: Follow through from part (b).[2 marks]
Question
The graph of a quadratic function has \(y\)-intercept 10 and one of its \(x\)-intercepts is 1.
The \(x\)-coordinate of the vertex of the graph is 3.
The equation of the quadratic function is in the form \(y = a{x^2} + bx + c\).
Write down the value of \(c\).[1]
Find the value of \(a\) and of \(b\).[4]
Write down the second \(x\)-intercept of the function.[1]
Answer/Explanation
Markscheme
10 (A1) (C1)
Note: Accept \((0,{\text{ }}10)\).[1 mark]
\(3 = \frac{{ – b}}{{2a}}\)
\(0 = a{(1)^2} + b(1) + c\)
\(10 = a{(6)^2} + b(6) + c\)
\(0 = a{(5)^2} + b(5) + c\) (M1)(M1)
Note: Award (M1) for each of the above equations, provided they are not equivalent, up to a maximum of (M1)(M1). Accept equations that substitute their 10 for \(c\).
OR
sketch graph showing given information: intercepts \((1,{\text{ }}0)\) and \((0,{\text{ }}10)\) and line \(x = 3\) (M1)
\(y = a(x – 1)(x – 5)\) (M1)
Note: Award (M1) for \((x – 1)(x – 5)\) seen.
\(a = 2\) (A1)(ft)
\(b = – 12\) (A1)(ft) (C4)
Note: Follow through from part (a).
If it is not clear which is \(a\) and which is \(b\) award at most (A0)(A1)(ft).[4 marks]
5 (A1) (C1)[1 mark]
Question
Jashanti is saving money to buy a car. The price of the car, in US Dollars (USD), can be modelled by the equation
\[P = 8500{\text{ }}{(0.95)^t}.\]
Jashanti’s savings, in USD, can be modelled by the equation
\[S = 400t + 2000.\]
In both equations \(t\) is the time in months since Jashanti started saving for the car.
Jashanti does not want to wait too long and wants to buy the car two months after she started saving. She decides to ask her parents for the extra money that she needs.
Write down the amount of money Jashanti saves per month.[1]
Use your graphic display calculator to find how long it will take for Jashanti to have saved enough money to buy the car.[2]
Calculate how much extra money Jashanti needs.[3]
Answer/Explanation
Markscheme
400 (USD) (A1) (C1)[1 mark]
\(8500{\text{ }}{(0.95)^t} = 400 \times t + 2000\) (M1)
Note: Award (M1) for equating \(8500{(0.95)^t}\) to \(400 \times t + 2000\) or for comparing the difference between the two expressions to zero or for showing a sketch of both functions.
\((t = ){\text{ }}8.64{\text{ (months) }}\left( {8.6414 \ldots {\text{ (months)}}} \right)\) (A1) (C2)
Note: Accept 9 months.[2 marks]
\(8500{(0.95)^2} – (400 \times 2 + 2000)\) (M1)(M1)
Note: Award (M1) for correct substitution of \(t = 2\) into equation for \(P\), (M1) for finding the difference between a value/expression for \(P\) and a value/expression for \(S\). The first (M1) is implied if 7671.25 seen.
4870 (USD) (4871.25) (A1) (C3)
Note: Accept 4871.3.[3 marks]
Question
On the grid below sketch the graph of the function \(f(x) = 2{(1.6)^x}\) for the domain \(0 \leqslant x \leqslant 3\) .
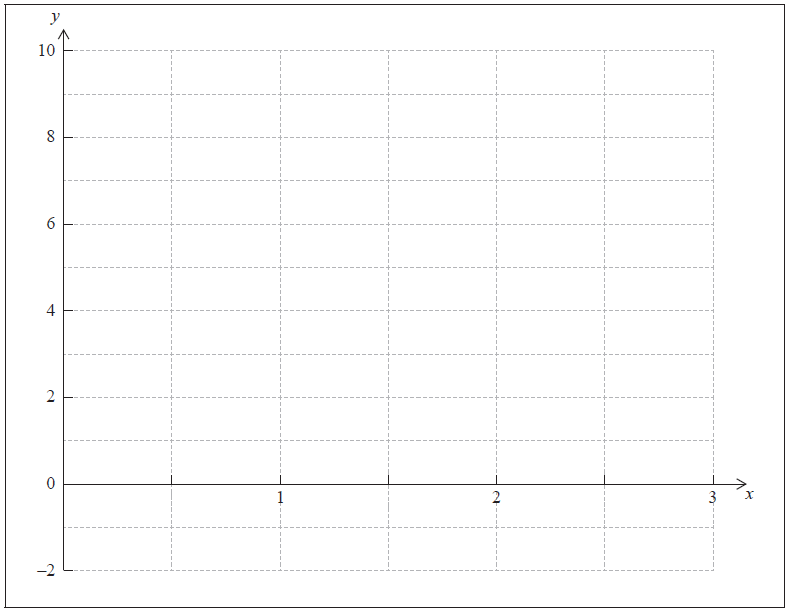 [2]
[2]
Write down the coordinates of the \(y\)-intercept of the graph of \(y = f(x)\) .[1]
On the grid draw the graph of the function \(g(x) = 5 – 2x\) for the domain \(0 \leqslant x \leqslant 3\).[2]
Use your graphic display calculator to solve \(f(x) = g(x)\) .[1]
Answer/Explanation
Markscheme

Note: Award (A1) correct endpoints, (A1) for smooth curve. (A1)(A1) (C2)
\((0{\text{, }}2)\) (A1) (C1)
Note: Accept \(x = 0\), \(y = 2\)
Straight line in the given domain (A1)
Axes intercepts in the correct positions (A1) (C2)
\(x = 0.943\) (\(0.94259 \ldots \)) (A1) (C1)
Note: Award (A0) if \(y\)-coordinate given.
Question
A sketch of the function \(f(x) = 5{x^3} – 3{x^5} + 1\) is shown for \( – 1.5 \leqslant x \leqslant 1.5\) and \( – 6 \leqslant y \leqslant 6\) .
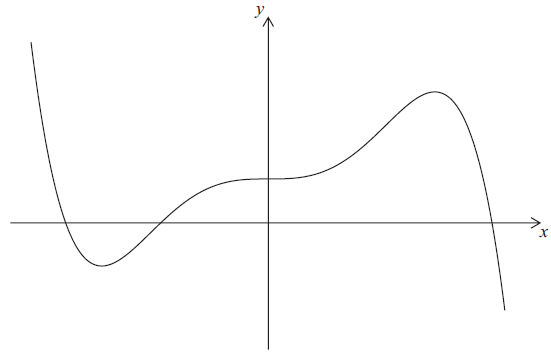
Write down \(f'(x)\) .[2]
Find the equation of the tangent to the graph of \(y = f(x)\) at \((1{\text{, }}3)\) .[2]
Write down the coordinates of the second point where this tangent intersects the graph of \(y = f(x)\) .[2]
Answer/Explanation
Markscheme
\(f'(x) = 15{x^2} – 15{x^4}\) (A1)(A1) (C2)
Note: Award a maximum of (A1)(A0) if extra terms seen.
\(f'(1) = 0\) (M1)
Note: Award (M1) for \(f'(x) = 0\) .
\(y = 3\) (A1)(ft) (C2)
Note: Follow through from their answer to part (a).
\(( – 1.38{\text{, }}3)\) \(( – 1.38481 \ldots {\text{, }}3)\) (A1)(ft)(A1)(ft) (C2)
Note: Follow through from their answer to parts (a) and (b).
Note: Accept \(x = – 1.38\), \(y = 3\) (\(x = – 1.38481 \ldots\) , \(y = 3\)) .

