IB DP Maths Topic 1.4 :Proof by mathematical induction HL Paper 2

prove that \(1 + 2\left( {\frac{1}{2}} \right) + 3{\left( {\frac{1}{2}} \right)^2} + 4{\left( {\frac{1}{2}} \right)^3} + … + n{\left( {\frac{1}{2}} \right)^{n – 1}} = 4 – \frac{{n + 2}}{{{2^{n – 1}}}}\)

for n = 1

\({\text{LHS}} = 1,{\text{ RHS}} = 4 – \frac{{1 + 2}}{{{2^0}}} = 4 – 3 = 1\)

so true for n = 1     R1

assume true for n = k     M1

so \(1 + 2\left( {\frac{1}{2}} \right) + 3{\left( {\frac{1}{2}} \right)^2} + 4{\left( {\frac{1}{2}} \right)^3} + … + k{\left( {\frac{1}{2}} \right)^{k – 1}} = 4 – \frac{{k + 2}}{{{2^{k – 1}}}}\)

now for n = k +1

LHS: \(1 + 2\left( {\frac{1}{2}} \right) + 3{\left( {\frac{1}{2}} \right)^2} + 4{\left( {\frac{1}{2}} \right)^3} + … + k{\left( {\frac{1}{2}} \right)^{k – 1}} + (k + 1){\left( {\frac{1}{2}} \right)^k}\)     A1

\( = 4 – \frac{{k + 2}}{{{2^{k – 1}}}} + (k + 1){\left( {\frac{1}{2}} \right)^k}\)     M1A1

\( = 4 – \frac{{2(k + 2)}}{{{2^k}}} + \frac{{k + 1}}{{{2^k}}}\,\,\,\,\,\)(or equivalent)     A1

\( = 4 – \frac{{(k + 1) + 2}}{{{2^{(k + 1) – 1}}}}\,\,\,\,\,\)(accept \(4 – \frac{{k + 3}}{{{2^k}}}\))     A1

Therefore if it is true for n = k it is true for n = k + 1. It has been shown to be true for n = 1 so it is true for all \(n{\text{ }}( \in {\mathbb{Z}^ + })\).     R1

Note: To obtain the final R mark, a reasonable attempt at induction must have been made.

[8 marks]

(a)

METHOD 1

\(\int {{{\text{e}}^{2x}}\sin x{\text{d}}x = – \cos x{{\text{e}}^{2x}} + \int {2{{\text{e}}^{2x}}\cos x{\text{d}}x} } \)     M1A1A1

\( = – \cos x{{\text{e}}^{2x}} + 2{{\text{e}}^{2x}}\sin x – \int {4{{\text{e}}^{2x}}\sin x{\text{d}}x} \)     A1A1

\(5\int {{{\text{e}}^{2x}}\sin x{\text{d}}x = – \cos x{{\text{e}}^{2x}} + 2{{\text{e}}^{2x}}\sin x} \)     M1

\(\int {{{\text{e}}^{2x}}\sin x{\text{d}}x = \frac{1}{5}{{\text{e}}^{2x}}(2\sin x – \cos x) + C} \)     AG 

METHOD 2

\(\int {\sin x{{\text{e}}^{2x}}{\text{d}}x = \frac{{\sin x{{\text{e}}^{2x}}}}{2} – \int {\cos x\frac{{{{\text{e}}^{2x}}}}{2}{\text{d}}x} } \)     M1A1A1

\( = \frac{{\sin x{{\text{e}}^{2x}}}}{2} – \cos x\frac{{{{\text{e}}^{2x}}}}{4} – \int {\sin x\frac{{{{\text{e}}^{2x}}}}{4}{\text{d}}x} \)     A1A1

\(\frac{5}{4}\int {{{\text{e}}^{2x}}\sin x{\text{d}}x = \frac{{{{\text{e}}^{2x}}\sin x}}{2} – \frac{{\cos x{{\text{e}}^{2x}}}}{4}} \)     M1

\(\int {{{\text{e}}^{2x}}\sin x{\text{d}}x = \frac{1}{5}{{\text{e}}^{2x}}(2\sin x – \cos x) + C} \)     AG

[6 marks]

(b)

\(\int {\frac{{{\text{d}}y}}{{\sqrt {1 – {y^2}} }} = \int {{{\text{e}}^{2x}}\sin x{\text{d}}x} } \)     M1A1

\(\arcsin y = \frac{1}{5}{{\text{e}}^{2x}}(2\sin x – \cos x)( + C)\)     A1

when \(x = 0,{\text{ }}y = 0 \Rightarrow C = \frac{1}{5}\)     M1

\(y = \sin \left( {\frac{1}{5}{{\text{e}}^{2x}}(2\sin x – \cos x) + \frac{1}{5}} \right)\)     A1

[5 marks]

(c)

(i) 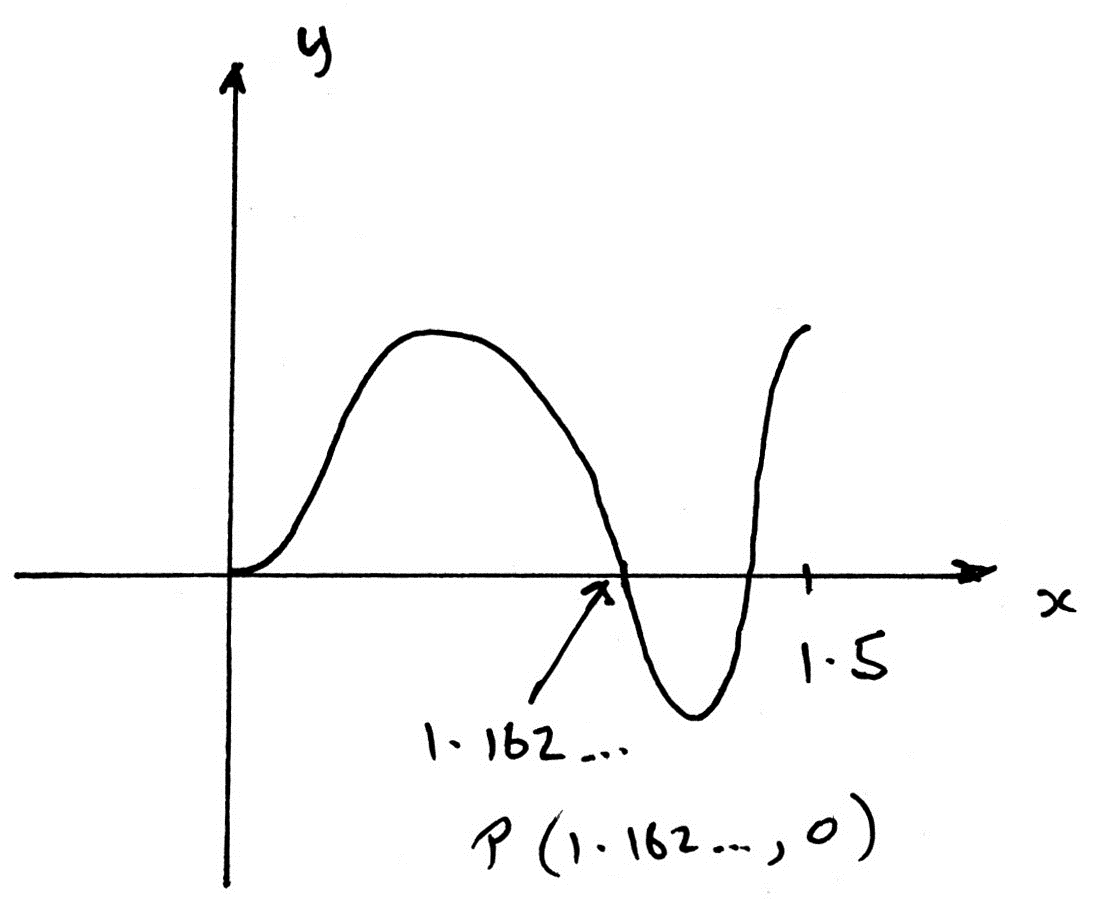        A1

P is (1.16, 0)     A1

Note: Award A1 for 1.16 seen anywhere, A1 for complete sketch.

Note: Allow FT on their answer from (b)

(ii)     \(V = \int_0^{1.162…} {\pi {y^2}{\text{d}}x} \)     M1A1

\( = 1.05\)     A2

Note: Allow FT on their answers from (b) and (c)(i).

[6 marks]

Part A: Given that this question is at the easier end of the ‘proof by induction’ spectrum, it was disappointing that so many candidates failed to score full marks. The n = 1 case was generally well done. The whole point of the method is that it involves logic, so ‘let n = k’ or ‘put n = k’, instead of ‘assume … to be true for n = k’, gains no marks. The algebraic steps need to be more convincing than some candidates were able to show. It is astonishing that the R1 mark for the final statement was so often not awarded.

Part B: Part (a) was often well done, although some faltered after the first integration. Part (b) was also generally well done, although there were some errors with the constant of integration. In (c) the graph was often attempted, but errors in (b) usually led to manifestly incorrect plots. Many attempted the volume of integration and some obtained the correct value.