Question
Part of the graph of a function f is shown in the diagram below.
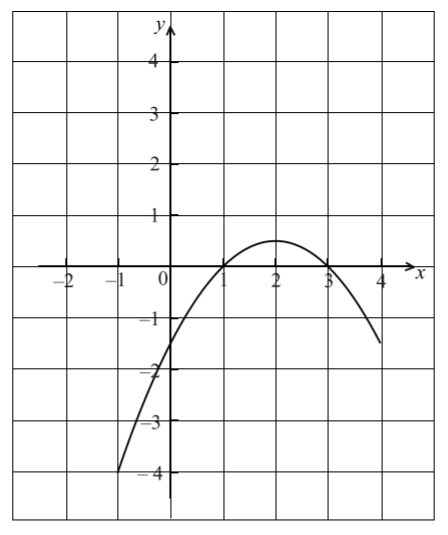
On the same diagram sketch the graph of \(y = – f(x)\) .
Let \(g(x) = f(x + 3)\) .
(i) Find \(g( – 3)\) .
(ii) Describe fully the transformation that maps the graph of f to the graph of g.
Answer/Explanation
Markscheme
 M1A1 N2
M1A1 N2
Note: Award M1 for evidence of reflection in x-axis, A1 for correct vertex and all intercepts approximately correct.
(i) \(g( – 3) = f(0)\) (A1)
\(f(0) = – 1.5\) A1 N2
(ii) translation (accept shift, slide, etc.) of \(\left( {\begin{array}{*{20}{c}}
{ – 3}\\
0
\end{array}} \right)\) A1A1 N2
[4 marks]
Question
Let f be the function given by \(f(x) = {{\rm{e}}^{0.5x}}\) , \(0 \le x \le 3.5\) . The diagram shows the graph of f .
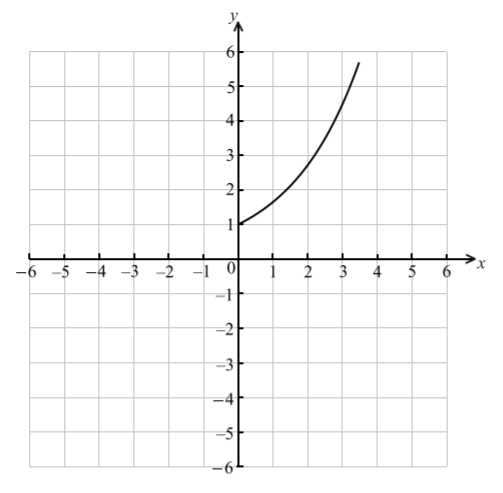
 On the same diagram, sketch the graph of \({f^{ – 1}}\) .
On the same diagram, sketch the graph of \({f^{ – 1}}\) .
Write down the range of \({f^{ – 1}}\) .
Find \({f^{ – 1}}(x)\) .
Answer/Explanation
Markscheme

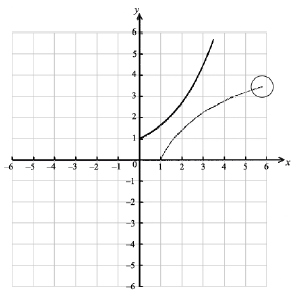
A1A1A1 N3
Note: Award A1 for approximately correct (reflected) shape, A1 for right end point in circle, A1 for through \((1{\text{, }}0)\) .
\(0 \le y \le 3.5\) A1 N1
[1 mark]
interchanging x and y (seen anywhere) M1
e.g. \(x = {e^{0.5y}}\)
evidence of changing to log form A1
e.g. \(\ln x = 0.5y\) , \(\ln x = \ln {{\rm{e}}^{0.5y}}\) (any base), \(\ln x = 0.5y\ln {\rm{e}}\) (any base)
\({f^{ – 1}}(x) = 2\ln x\) A1 N1
[3 marks]
Question
Let \(f(x) = 2{x^3} + 3\) and \(g(x) = {{\rm{e}}^{3x}} – 2\) .
(i) Find \(g(0)\) .
(ii) Find \((f \circ g)(0)\) .
Find \({f^{ – 1}}(x)\) .
Answer/Explanation
Markscheme
(i) \(g(0) = {{\rm{e}}^0} – 2\) (A1)
\( = – 1\) A1 N2
(ii) METHOD 1
substituting answer from (i) (M1)
e.g. \((f \circ g)(0) = f( – 1)\)
correct substitution \(f( – 1) = 2{( – 1)^3} + 3\) (A1)
\(f( – 1) = 1\) A1 N3
METHOD 2
attempt to find \((f \circ g)(x)\) (M1)
e.g. \((f \circ g)(x) = f({{\rm{e}}^{3x}} – 2)\) \( = 2{({{\rm{e}}^{3x}} – 2)^3} + 3\)
correct expression for \((f \circ g)(x)\) (A1)
e.g. \(2{({{\rm{e}}^{3x}} – 2)^3} + 3\)
\((f \circ g)(0) = 1\) A1 N3
[5 marks]
interchanging x and y (seen anywhere) (M1)
e.g. \(x = 2{y^3} + 3\)
attempt to solve (M1)
e.g. \({y^3} = \frac{{x – 3}}{2}\)
\({f^{ – 1}}(x) = \sqrt[3]{{\frac{{x – 3}}{2}}}\) A1 N3
[3 marks]
Question
Consider \(f(x) = \ln ({x^4} + 1)\) .
The second derivative is given by \(f”(x) = \frac{{4{x^2}(3 – {x^4})}}{{{{({x^4} + 1)}^2}}}\) .
The equation \(f”(x) = 0\) has only three solutions, when \(x = 0\) , \( \pm \sqrt[4]{3}\) \(( \pm 1.316 \ldots )\) .
Find the value of \(f(0)\) .
Find the set of values of \(x\) for which \(f\) is increasing.
(i) Find \(f”(1)\) .
(ii) Hence, show that there is no point of inflexion on the graph of \(f\) at \(x = 0\) .
There is a point of inflexion on the graph of \(f\) at \(x = \sqrt[4]{3}\) \((x = 1.316 \ldots )\) .
Sketch the graph of \(f\) , for \(x \ge 0\) .
Answer/Explanation
Markscheme
substitute \(0\) into \(f\) (M1)
eg \(\ln (0 + 1)\) , \(\ln 1\)
\(f(0) = 0\) A1 N2
[2 marks]
\(f'(x) = \frac{1}{{{x^4} + 1}} \times 4{x^3}\) (seen anywhere) A1A1
Note: Award A1 for \(\frac{1}{{{x^4} + 1}}\) and A1 for \(4{x^3}\) .
recognizing \(f\) increasing where \(f'(x) > 0\) (seen anywhere) R1
eg \(f'(x) > 0\) , diagram of signs
attempt to solve \(f'(x) > 0\) (M1)
eg \(4{x^3} = 0\) , \({x^3} > 0\)
\(f\) increasing for \(x > 0\) (accept \(x \ge 0\) ) A1 N1
[5 marks]
(i) substituting \(x = 1\) into \(f”\) (A1)
eg \(\frac{{4(3 – 1)}}{{{{(1 + 1)}^2}}}\) , \(\frac{{4 \times 2}}{4}\)
\(f”(1) = 2\) A1 N2
(ii) valid interpretation of point of inflexion (seen anywhere) R1
eg no change of sign in \(f”(x)\) , no change in concavity,
\(f’\) increasing both sides of zero
attempt to find \(f”(x)\) for \(x < 0\) (M1)
eg \(f”( – 1)\) , \(\frac{{4{{( – 1)}^2}(3 – {{( – 1)}^4})}}{{{{({{( – 1)}^4} + 1)}^2}}}\) , diagram of signs
correct working leading to positive value A1
eg \(f”( – 1) = 2\) , discussing signs of numerator and denominator
there is no point of inflexion at \(x = 0\) AG N0
[5 marks]
 A1A1A1 N3
A1A1A1 N3
Notes: Award A1 for shape concave up left of POI and concave down right of POI.
Only if this A1 is awarded, then award the following:
A1 for curve through (\(0\), \(0\)) , A1 for increasing throughout.
Sketch need not be drawn to scale. Only essential features need to be clear.
[3 marks]
Question
The diagram below shows the graph of a function \(f\) , for \( – 1 \le x \le 2\) .
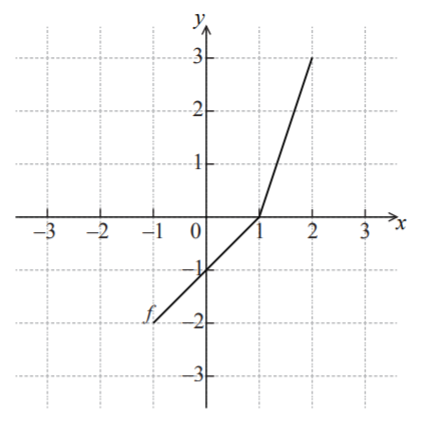

Write down the value of \(f(2)\).
Write down the value of \({f^{ – 1}}( – 1)\) .
Sketch the graph of \({f^{ – 1}}\) on the grid below.


Answer/Explanation
Markscheme
\(f(2) = 3\) A1 N1
[1 mark]
\({f^{ – 1}}( – 1) = 0\) A2 N2
[2 marks]
EITHER
attempt to draw \(y = x\) on grid (M1)
OR
attempt to reverse x and y coordinates (M1)
eg writing or plotting at least two of the points
\(( – 2, – 1)\) , \(( – 1,0)\) , \((0,1)\) , \((3,2)\)
THEN
correct graph A2 N3


[3 marks]
Question
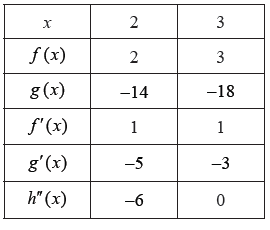
Consider the functions \(f(x)\) , \(g(x)\) and \(h(x)\) . The following table gives some values associated with these functions.

The following diagram shows parts of the graphs of \(h\) and \(h”\) .
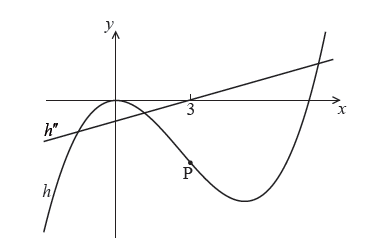

There is a point of inflexion on the graph of \(h\) at P, when \(x = 3\) .
Given that \(h(x) = f(x) \times g(x)\) ,
Write down the value of \(g(3)\) , of \(f'(3)\) , and of \(h”(2)\) .
Explain why P is a point of inflexion.
find the \(y\)-coordinate of P.
find the equation of the normal to the graph of \(h\) at P.
Answer/Explanation
Markscheme
\(g(3) = – 18\) , \(f'(3) = 1\) , \(h”(2) = – 6\) A1A1A1 N3
[3 marks]
\(h”(3) = 0\) (A1)
valid reasoning R1
eg \({h”}\) changes sign at \(x = 3\) , change in concavity of \(h\) at \(x = 3\)
so P is a point of inflexion AG N0
[2 marks]
writing \(h(3)\) as a product of \(f(3)\) and \(g(3)\) A1
eg \(f(3) \times g(3)\) , \(3 \times ( – 18)\)
\(h(3) = – 54\) A1 N1
[2 marks]
recognizing need to find derivative of \(h\) (R1)
eg \({h’}\) , \(h'(3)\)
attempt to use the product rule (do not accept \(h’ = f’ \times g’\) ) (M1)
eg \(h’ = fg’ + gf’\) , \(h'(3) = f(3) \times g'(3) + g(3) \times f'(3)\)
correct substitution (A1)
eg \(h'(3) = 3( – 3) + ( – 18) \times 1\)
\(h'(3) = – 27\) A1
attempt to find the gradient of the normal (M1)
eg \( – \frac{1}{m}\) , \( – \frac{1}{{27}}x\)
attempt to substitute their coordinates and their normal gradient into the equation of a line (M1)
eg \( – 54 = \frac{1}{{27}}(3) + b\) , \(0 = \frac{1}{{27}}(3) + b\) , \(y + 54 = 27(x – 3)\) , \(y – 54 = \frac{1}{{27}}(x + 3)\)
correct equation in any form A1 N4
eg \(y + 54 = \frac{1}{{27}}(x – 3)\) , \(y = \frac{1}{{27}}x – 54\frac{1}{9}\)
[7 marks]
Question
The following diagram shows the graph of \(y = f(x)\), for \( – 4 \le x \le 5\).


Write down the value of \(f( – 3)\).
Write down the value of \({f^{ – 1}}(1)\).
Find the domain of \({f^{ – 1}}\).
On the grid above, sketch the graph of \({f^{ – 1}}\).
Answer/Explanation
Markscheme
\(f( – 3) = – 1\) A1 N1
[1 mark]
\({f^{ – 1}}(1) = 0\) (accept \(y = 0\)) A1 N1
[1 mark]
domain of \({f^{ – 1}}\) is range of \(f\) (R1)
eg \({\text{R}}f = {\text{D}}{f^{ – 1}}\)
correct answer A1 N2
eg \( – 3 \leqslant x \leqslant 3,{\text{ }}x \in [ – 3,{\text{ }}3]{\text{ (accept }} – 3 < x < 3,{\text{ }} – 3 \leqslant y \leqslant 3)\)
[2 marks]
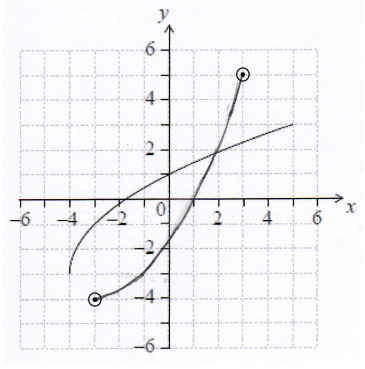
 A1A1 N2
A1A1 N2
Note: Graph must be approximately correct reflection in \(y = x\).
Only if the shape is approximately correct, award the following:
A1 for x-intercept at \(1\), and A1 for endpoints within circles.
[2 marks]
Question
Let \(f(x) = 8x + 3\) and \(g(x) = 4x\), for \(x \in \mathbb{R}\).
Write down \(g(2)\).
Find \((f \circ g)(x)\).
Find \({f^{ – 1}}(x)\).
Answer/Explanation
Markscheme
\(g(2) = 8\) A1 N1
[1 mark]
attempt to form composite (in any order) (M1)
eg\(\,\,\,\,\,\)\(f(4x),{\text{ }}4 \times (8x + 3)\)
\((f \circ g)(x) = 32x + 3\) A1 N2
[2 marks]
interchanging \(x\) and \(y\) (may be seen at any time) (M1)
eg\(\,\,\,\,\,\)\(x = 8y + 3\)
\({f^{ – 1}}(x) = \frac{{x – 3}}{8}\,\,\,\,\,\left( {{\text{accept }}\frac{{x – 3}}{8},{\text{ }}y = \frac{{x – 3}}{8}} \right)\) A1 N2
[2 marks]
Question
The following diagram shows the graph of a function \(f\), with domain \( – 2 \leqslant x \leqslant 4\).
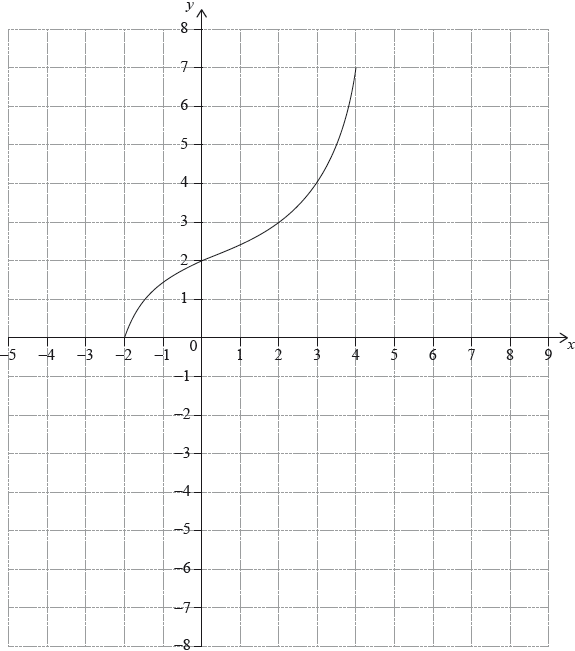
The points \(( – 2,{\text{ }}0)\) and \((4,{\text{ }}7)\) lie on the graph of \(f\).
Write down the range of \(f\).
Write down \(f(2)\);
Write down \({f^{ – 1}}(2)\).
On the grid, sketch the graph of \({f^{ – 1}}\).
Answer/Explanation
Markscheme
correct range (do not accept \(0 \leqslant x \leqslant 7\)) A1 N1
eg\(\,\,\,\,\,\)\([0,{\text{ }}7],{\text{ }}0 \leqslant y \leqslant 7\)
[1 mark]
\(f(2) = 3\) A1 N1
[1 mark]
\({f^{ – 1}}(2) = 0\) A1 N1
[1 mark]
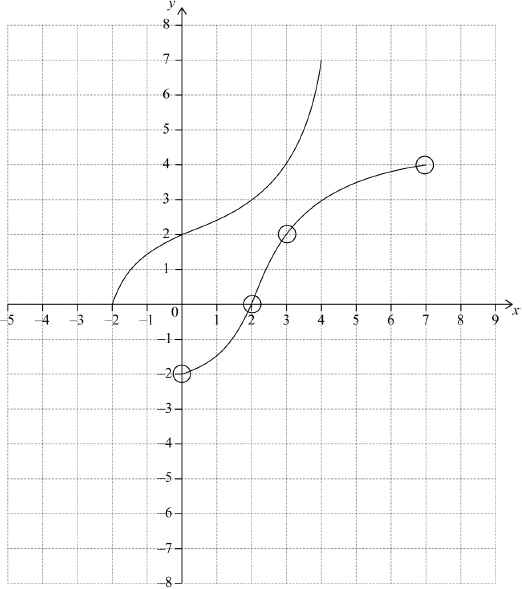 A1A1A1 N3
A1A1A1 N3
Notes: Award A1 for both end points within circles,
A1 for images of \((2,{\text{ }}3)\) and \((0,{\text{ }}2)\) within circles,
A1 for approximately correct reflection in \(y = x\), concave up then concave down shape (do not accept line segments).
[3 marks]
Question
Let \(f\left( x \right) = \sqrt {x + 2} \) for x ≥ 2 and g(x) = 3x − 7 for \(x \in \mathbb{R}\).
Write down f (14).
Find \(\left( {g \circ f} \right)\) (14).
Find g−1(x).
Answer/Explanation
Markscheme
f (14) = 4 A1 N1
[1 mark]
attempt to substitute (M1)
eg g (4), 3 × 4 − 7
5 A1 N2
[2 marks]
interchanging x and y (seen anywhere) (M1)
eg x = 3y − 7
evidence of correct manipulation (A1)
eg x + 7 = 3y
\({g^{ – 1}}\left( x \right) = \frac{{x + 7}}{3}\) A1 N3
[3 marks]
Question
Consider a function f (x) , for −2 ≤ x ≤ 2 . The following diagram shows the graph of f.
Write down the value of f (0).
Write down the value of f −1 (1).
Write down the range of f −1.
On the grid above, sketch the graph of f −1.
Answer/Explanation
Markscheme
\(f\left( 0 \right) = – \frac{1}{2}\) A1 N1
[1 mark]
f −1 (1) = 2 A1 N1
[1 mark]
−2 ≤ y ≤ 2, y∈ [−2, 2] (accept −2 ≤ x ≤ 2) A1 N1
[1 mark]
A1A1A1A1 N4
Note: Award A1 for evidence of approximately correct reflection in y = x with correct curvature.
(y = x does not need to be explicitly seen)
Only if this mark is awarded, award marks as follows:
A1 for both correct invariant points in circles,
A1 for the three other points in circles,
A1 for correct domain.
[4 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]

