Question
Let \(p = \sin 40^\circ \) and \(q = \cos 110^\circ \) . Give your answers to the following in terms of p and/or q .
Write down an expression for
(i) \(\sin 140^\circ \) ;
(ii) \(\cos 70^\circ \) .
Find an expression for \(\cos 140^\circ \) .
Find an expression for \(\tan 140^\circ \) .
Answer/Explanation
Markscheme
(i) \(\sin 140^\circ = p\) A1 N1
(ii) \(\cos 70^\circ = – q\) A1 N1
[2 marks]
METHOD 1
evidence of using \({\sin ^2}\theta + {\cos ^2}\theta = 1\) (M1)
e.g. diagram, \(\sqrt {1 – {p^2}} \) (seen anywhere)
\(\cos 140^\circ = \pm \sqrt {1 – {p^2}} \) (A1)
\(\cos 140^\circ = – \sqrt {1 – {p^2}} \) A1 N2
METHOD 2
evidence of using \(\cos 2\theta = 2{\cos ^2}\theta – 1\) (M1)
\(\cos 140^\circ = 2{\cos ^2}70 – 1\) (A1)
\(\cos 140^\circ = 2{( – q)^2} – 1\) \(( = 2{q^2} – 1)\) A1 N2
[3 marks]
METHOD 1
\(\tan 140^\circ = \frac{{\sin 140^\circ }}{{\cos 140^\circ }} = – \frac{p}{{\sqrt {1 – {p^2}} }}\) A1 N1
METHOD 2
\(\tan 140^\circ = \frac{p}{{2{q^2} – 1}}\) A1 N1
[1 mark]
Question
Let \(f(x) = {\sin ^3}x + {\cos ^3}x\tan x,\frac{\pi }{2} < x < \pi \) .
Show that \(f(x) = \sin x\) .
Let \(\sin x = \frac{2}{3}\) . Show that \(f(2x) = – \frac{{4\sqrt 5 }}{9}\) .
Answer/Explanation
Markscheme
changing \(\tan x\) into \(\frac{{\sin x}}{{\cos x}}\) A1
e.g. \({\sin ^3}x + {\cos ^3}x\frac{{\sin x}}{{\cos x}}\)
simplifying A1
e.g \(\sin x({\sin ^2}x + {\cos ^2}x)\) , \({\sin ^3}x + \sin x – {\sin ^3}x\)
\(f(x) = \sin x\) AG N0
[2 marks]
recognizing \(f(2x) = \sin 2x\) , seen anywhere (A1)
evidence of using double angle identity \(\sin (2x) = 2\sin x\cos x\) , seen anywhere (M1)
evidence of using Pythagoras with \(\sin x = \frac{2}{3}\) M1
e.g. sketch of right triangle, \({\sin ^2}x + {\cos ^2}x = 1\)
\(\cos x = – \frac{{\sqrt 5 }}{3}\) (accept \(\frac{{\sqrt 5 }}{3}\) ) (A1)
\(f(2x) = 2\left( {\frac{2}{3}} \right)\left( { – \frac{{\sqrt 5 }}{3}} \right)\) A1
\(f(2x) = – \frac{{4\sqrt 5 }}{9}\) AG N0
[5 marks]
Question
Solve \(\cos 2x – 3\cos x – 3 – {\cos ^2}x = {\sin ^2}x\) , for \(0 \le x \le 2\pi \) .
Answer/Explanation
Markscheme
evidence of substituting for \(\cos 2x\) (M1)
evidence of substituting into \({\sin ^2}x + {\cos ^2}x = 1\) (M1)
correct equation in terms of \(\cos x\) (seen anywhere) A1
e.g. \(2{\cos ^2}x – 1 – 3\cos x – 3 = 1\) , \(2{\cos ^2}x – 3\cos x – 5 = 0\)
evidence of appropriate approach to solve (M1)
e.g. factorizing, quadratic formula
appropriate working A1
e.g. \((2\cos x – 5)(\cos x + 1) = 0\) , \((2x – 5)(x + 1)\) , \(\cos x = \frac{{3 \pm \sqrt {49}}}{4}\)
correct solutions to the equation
e.g. \(\cos x = \frac{5}{2}\) , \(\cos x = – 1\) , \(x = \frac{5}{2}\) , \(x = – 1\) (A1)
\(x = \pi \) A1 N4
[7 marks]
Question
Let \(f(x) = {(\sin x + \cos x)^2}\) .
Show that \(f(x)\) can be expressed as \(1 + \sin 2x\) .
The graph of f is shown below for \(0 \le x \le 2\pi \) .
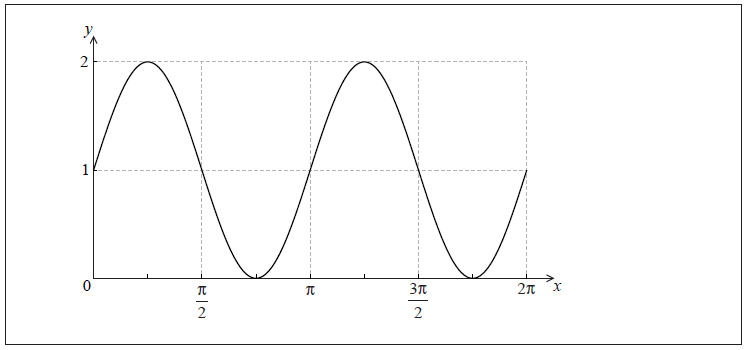
Let \(g(x) = 1 + \cos x\) . On the same set of axes, sketch the graph of g for \(0 \le x \le 2\pi \) .
The graph of g can be obtained from the graph of f under a horizontal stretch of scale factor p followed by a translation by the vector \(\left( {\begin{array}{*{20}{c}}
k\\
0
\end{array}} \right)\) .
Write down the value of p and a possible value of k .
Answer/Explanation
Markscheme
attempt to expand (M1)
e.g. \((\sin x + \cos x)(\sin x + \cos x)\) ; at least 3 terms
correct expansion A1
e.g. \({\sin ^2}x + 2\sin x\cos x + {\cos ^2}x\)
\(f(x) = 1 + \sin 2x\) AG N0
[2 marks]
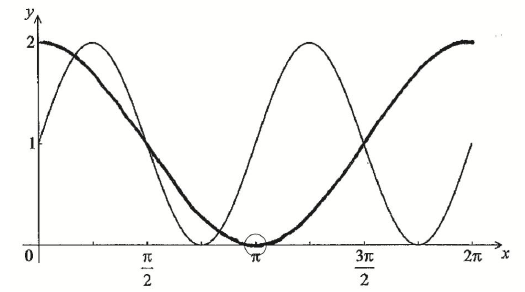A1A1 N2
Note: Award A1 for correct sinusoidal shape with period \(2\pi \) and range \([0{\text{, }}2]\), A1 for minimum in circle.
\(p = 2\) , \(k = – \frac{\pi }{2}\) A1A1 N2
[2 marks]
Question
Given that \(\sin x = \frac{3}{4}\), where \(x\) is an obtuse angle,
find the value of \(\cos x;\)
find the value of \(\cos 2x.\)
Answer/Explanation
Markscheme
valid approach (M1)
eg\(\;\;\;\)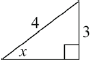
, \({\sin ^2}x + {\cos ^2}x = 1\)
correct working (A1)
eg\(\;\;\;{4^2} – {3^2},{\text{ }}{\cos ^2}x = 1 – {\left( {\frac{3}{4}} \right)^2}\)
correct calculation (A1)
eg\(\;\;\;\frac{{\sqrt 7 }}{4},{\text{ }}{\cos ^2}x = \frac{7}{{16}}\)
\(\cos x = – \frac{{\sqrt 7 }}{4}\) A1 N3
[4 marks]
correct substitution (accept missing minus with cos) (A1)
eg\(\;\;\;1 – 2{\left( {\frac{3}{4}} \right)^2},{\text{ }}2{\left( { – \frac{{\sqrt 7 }}{4}} \right)^2} – 1,{\text{ }}{\left( {\frac{{\sqrt 7 }}{4}} \right)^2} – {\left( {\frac{3}{4}} \right)^2}\)
correct working A1
eg\(\;\;\;2\left( {\frac{7}{{16}}} \right) – 1,{\text{ }}1 – \frac{{18}}{{16}},{\text{ }}\frac{7}{{16}} – \frac{9}{{16}}\)
\(\cos 2x = – \frac{2}{{16}}\;\;\;\left( { = – \frac{1}{8}} \right)\) A1 N2
[3 marks]
Total [7 marks]
Question
Let \(f(x) = 6x\sqrt {1 – {x^2}} \), for \( – 1 \leqslant x \leqslant 1\), and \(g(x) = \cos (x)\), for \(0 \leqslant x \leqslant \pi \).
Let \(h(x) = (f \circ g)(x)\).
Write \(h(x)\) in the form \(a\sin (bx)\), where \(a,{\text{ }}b \in \mathbb{Z}\).
Hence find the range of \(h\).
Answer/Explanation
Markscheme
attempt to form composite in any order (M1)
eg\(\,\,\,\,\,\)\(f\left( {g(x)} \right),{\text{ }}\cos \left( {6x\sqrt {1 – {x^2}} } \right)\)
correct working (A1)
eg\(\,\,\,\,\,\)\(6\cos x\sqrt {1 – {{\cos }^2}x} \)
correct application of Pythagorean identity (do not accept \({\sin ^2}x + {\cos ^2}x = 1\)) (A1)
eg\(\,\,\,\,\,\)\({\sin ^2}x = 1 – {\cos ^2}x,{\text{ }}6\cos x\sin x,{\text{ }}6\cos x \left| \sin x\right|\)
valid approach (do not accept \(2\sin x\cos x = \sin 2x\)) (M1)
eg\(\,\,\,\,\,\)\(3(2\cos x\sin x)\)
\(h(x) = 3\sin 2x\) A1 N3
[5 marks]
valid approach (M1)
eg\(\,\,\,\,\,\)amplitude \( = 3\), sketch with max and min \(y\)-values labelled, \( – 3 < y < 3\)
correct range A1 N2
eg\(\,\,\,\,\,\)\( – 3 \leqslant y \leqslant 3\), \([ – 3,{\text{ }}3]\) from \( – 3\) to 3
Note: Do not award A1 for \( – 3 < y < 3\) or for “between \( – 3\) and 3”.
[2 marks]
Examiners report
In part (a), nearly all candidates found the correct composite function in terms of \(\cos x\), though many did not get any further than this first step in their solution to the question. While some candidates seemed to recognize the need to use trigonometric identities, most were unsuccessful in finding the correct expression in the required form.
In part (b), very few candidates were able to provide the correct range of the function.
Question
Let \(\sin \theta = \frac{{\sqrt 5 }}{3}\), where \(\theta \) is acute.
Find \(\cos \theta \).
Find \(\cos 2\theta \).
Answer/Explanation
Markscheme
evidence of valid approach (M1)
eg\(\,\,\,\,\,\)right triangle, \({\cos ^2}\theta = 1 – {\sin ^2}\theta \)
correct working (A1)
eg\(\,\,\,\,\,\)missing side is 2, \(\sqrt {1 – {{\left( {\frac{{\sqrt 5 }}{3}} \right)}^2}} \)
\(\cos \theta = \frac{2}{3}\) A1 N2
[3 marks]
correct substitution into formula for \(\cos 2\theta \) (A1)
eg\(\,\,\,\,\,\)\(2 \times {\left( {\frac{2}{3}} \right)^2} – 1,{\text{ }}1 – 2{\left( {\frac{{\sqrt 5 }}{3}} \right)^2},{\text{ }}{\left( {\frac{2}{3}} \right)^2} – {\left( {\frac{{\sqrt 5 }}{3}} \right)^2}\)
\(\cos 2\theta = – \frac{1}{9}\) A1 N2
[2 marks]
Question
Solve \({\log _2}(2\sin x) + {\log _2}(\cos x) = – 1\), for \(2\pi < x < \frac{{5\pi }}{2}\).
Answer/Explanation
Markscheme
correct application of \(\log a + \log b = \log ab\) (A1)
eg\(\,\,\,\,\,\)\({\log _2}(2\sin x\cos x),{\text{ }}\log 2 + \log (\sin x) + \log (\cos x)\)
correct equation without logs A1
eg\(\,\,\,\,\,\)\(2\sin x\cos x = {2^{ – 1}},{\text{ }}\sin x\cos x = \frac{1}{4},{\text{ }}\sin 2x = \frac{1}{2}\)
recognizing double-angle identity (seen anywhere) A1
eg\(\,\,\,\,\,\)\(\log (\sin 2x),{\text{ }}2\sin x\cos x = \sin 2x,{\text{ }}\sin 2x = \frac{1}{2}\)
evaluating \({\sin ^{ – 1}}\left( {\frac{1}{2}} \right) = \frac{\pi }{6}{\text{ }}(30^\circ )\) (A1)
correct working A1
eg\(\,\,\,\,\,\)\(x = \frac{\pi }{{12}} + 2\pi ,{\text{ }}2x = \frac{{25\pi }}{6},{\text{ }}\frac{{29\pi }}{6},{\text{ }}750^\circ ,{\text{ }}870^\circ ,{\text{ }}x = \frac{\pi }{{12}}\)and \(x = \frac{{5\pi }}{{12}}\), one correct final answer
\(x = \frac{{25\pi }}{{12}},{\text{ }}\frac{{29\pi }}{{12}}\) (do not accept additional values) A2 N0
[7 marks]
Question
Let \(f(x) = 15 – {x^2}\), for \(x \in \mathbb{R}\). The following diagram shows part of the graph of \(f\) and the rectangle OABC, where A is on the negative \(x\)-axis, B is on the graph of \(f\), and C is on the \(y\)-axis.
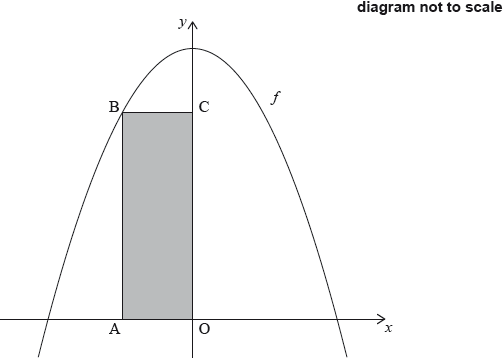
Find the \(x\)-coordinate of A that gives the maximum area of OABC.
Answer/Explanation
Markscheme
attempt to find the area of OABC (M1)
eg\(\,\,\,\,\,\)\({\text{OA}} \times {\text{OC, }}x \times f(x),{\text{ }}f(x) \times ( – x)\)
correct expression for area in one variable (A1)
eg\(\,\,\,\,\,\)\({\text{area}} = x(15 – {x^2}),{\text{ }}15x – {x^3},{\text{ }}{x^3} – 15x\)
valid approach to find maximum area (seen anywhere) (M1)
eg\(\,\,\,\,\,\)\(A’(x) = 0\)
correct derivative A1
eg\(\,\,\,\,\,\)\(15 – 3{x^2},{\text{ }}(15 – {x^2}) + x( – 2x) = 0,{\text{ }} – 15 + 3{x^2}\)
correct working (A1)
eg\(\,\,\,\,\,\)\(15 = 3{x^2},{\text{ }}{x^2} = 5,{\text{ }}x = \sqrt 5 \)
\(x = – \sqrt 5 {\text{ }}\left( {{\text{accept A}}\left( { – \sqrt 5 ,{\text{ }}0} \right)} \right)\) A2 N3
[7 marks]
Examiners report
Question
The first two terms of an infinite geometric sequence are u1 = 18 and u2 = 12sin2 θ , where 0 < θ < 2\(\pi \) , and θ ≠ \(\pi \).
Find an expression for r in terms of θ.
Find the possible values of r.
Show that the sum of the infinite sequence is \(\frac{{54}}{{2 + {\text{cos}}\,\left( {2\theta } \right)}}\).
Find the values of θ which give the greatest value of the sum.
Answer/Explanation
Markscheme
valid approach (M1)
eg \(\frac{{{u_2}}}{{{u_1}}},\,\,\frac{{{u_1}}}{{{u_2}}}\)
\(r = \frac{{12\,{{\sin }^2}\,\theta }}{{18}}\left( { = \frac{{2\,{{\sin }^2}\,\theta }}{3}} \right)\) A1 N2
[2 marks]
recognizing that sinθ is bounded (M1)
eg 0 ≤ sin2 θ ≤ 1, −1 ≤ sinθ ≤ 1, −1 < sinθ < 1
0 < r ≤ \(\frac{2}{3}\) A2 N3
Note: If working shown, award M1A1 for correct values with incorrect inequality sign(s).
If no working shown, award N1 for correct values with incorrect inequality sign(s).
[3 marks]
correct substitution into formula for infinite sum A1
eg \(\frac{{18}}{{1 – \frac{{2\,{\text{si}}{{\text{n}}^2}\,\theta }}{3}}}\)
evidence of choosing an appropriate rule for cos 2θ (seen anywhere) (M1)
eg cos 2θ = 1 − 2 sin2 θ
correct substitution of identity/working (seen anywhere) (A1)
eg \(\frac{{18}}{{1 – \frac{2}{3}\left( {\frac{{1 – {\text{cos}}\,2\theta }}{2}} \right)}},\,\,\frac{{54}}{{3 – 2\left( {\frac{{1 – {\text{cos}}\,2\theta }}{2}} \right)}},\,\,\frac{{18}}{{\frac{{3 – 2\,{\text{si}}{{\text{n}}^2}\,\theta }}{3}}}\)
correct working that clearly leads to the given answer A1
eg \(\frac{{18 \times 3}}{{2 + \left( {1 – 2\,{\text{si}}{{\text{n}}^2}\,\theta } \right)}},\,\,\frac{{54}}{{3 – \left( {1 – {\text{cos}}\,2\theta } \right)}}\)
\(\frac{{54}}{{2 + {\text{cos}}\left( {2\theta } \right)}}\) AG N0
[4 marks]
METHOD 1 (using differentiation)
recognizing \(\frac{{{\text{d}}{S_\infty }}}{{{\text{d}}\theta }} = 0\) (seen anywhere) (M1)
finding any correct expression for \(\frac{{{\text{d}}{S_\infty }}}{{{\text{d}}\theta }}\) (A1)
eg \(\frac{{0 – 54 \times \left( { – 2\,{\text{sin}}\,2\,\theta } \right)}}{{{{\left( {2 + {\text{cos}}\,2\,\theta } \right)}^2}}},\,\, – 54{\left( {2 + {\text{cos}}\,2\,\theta } \right)^{ – 2}}\,\left( { – 2\,{\text{sin}}\,2\,\theta } \right)\)
correct working (A1)
eg sin 2θ = 0
any correct value for sin−1(0) (seen anywhere) (A1)
eg 0, \(\pi \), … , sketch of sine curve with x-intercept(s) marked both correct values for 2θ (ignore additional values) (A1)
2θ = \(\pi \), 3\(\pi \) (accept values in degrees)
both correct answers \(\theta = \frac{\pi }{2},\,\frac{{3\pi }}{2}\) A1 N4
Note: Award A0 if either or both correct answers are given in degrees.
Award A0 if additional values are given.
METHOD 2 (using denominator)
recognizing when S∞ is greatest (M1)
eg 2 + cos 2θ is a minimum, 1−r is smallest
correct working (A1)
eg minimum value of 2 + cos 2θ is 1, minimum r = \(\frac{2}{3}\)
correct working (A1)
eg \({\text{cos}}\,2\,\theta = – 1,\,\,\frac{2}{3}\,{\text{si}}{{\text{n}}^2}\,\theta = \frac{2}{3},\,\,{\text{si}}{{\text{n}}^2}\theta = 1\)
EITHER (using cos 2θ)
any correct value for cos−1(−1) (seen anywhere) (A1)
eg \(\pi \), 3\(\pi \), … (accept values in degrees), sketch of cosine curve with x-intercept(s) marked
both correct values for 2θ (ignore additional values) (A1)
2θ = \(\pi \), 3\(\pi \) (accept values in degrees)
OR (using sinθ)
sinθ = ±1 (A1)
sin−1(1) = \(\frac{\pi }{2}\) (accept values in degrees) (seen anywhere) A1
THEN
both correct answers \(\theta = \frac{\pi }{2},\,\frac{{3\pi }}{2}\) A1 N4
Note: Award A0 if either or both correct answers are given in degrees.
Award A0 if additional values are given.
[6 marks]