Question
The function f is defined by \(f(x) = x\sqrt {9 – {x^2}} + 2\arcsin \left( {\frac{x}{3}} \right)\).
(a) Write down the largest possible domain, for each of the two terms of the function, f , and hence state the largest possible domain, D , for f .
(b) Find the volume generated when the region bounded by the curve y = f(x) , the x-axis, the y-axis and the line x = 2.8 is rotated through \(2\pi \) radians about the x-axis.
(c) Find \(f'(x)\) in simplified form.
(d) Hence show that \(\int_{ – p}^p {\frac{{11 – 2{x^2}}}{{\sqrt {9 – {x^2}} }}} {\text{d}}x = 2p\sqrt {9 – {p^2}} + 4\arcsin \left( {\frac{p}{3}} \right)\), where \(p \in D\) .
(e) Find the value of p which maximises the value of the integral in (d).
(f) (i) Show that \(f”(x) = \frac{{x(2{x^2} – 25)}}{{{{(9 – {x^2})}^{\frac{3}{2}}}}}\).
(ii) Hence justify that f(x) has a point of inflexion at x = 0 , but not at \(x = \pm \sqrt {\frac{{25}}{2}} \) .
Answer/Explanation
Markscheme
(a) For \(x\sqrt {9 – {x^2}} \), \( – 3 \leqslant x \leqslant 3\) and for \(2\arcsin \left( {\frac{x}{3}} \right)\), \( – 3 \leqslant x \leqslant 3\) A1
\( \Rightarrow D{\text{ is }} – 3 \leqslant x \leqslant 3\) A1
[2 marks]
(b) \(V = \pi \int_0^{2.8} {{{\left( {x\sqrt {9 – {x^2}} = 2\arcsin \frac{x}{3}} \right)}^2}{\text{d}}x} \) M1A1
= 181 A1
[3 marks]
(c) \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {(9 – {x^2})^{\frac{1}{2}}} – \frac{{{x^2}}}{{{{(9 – {x^2})}^{\frac{1}{2}}}}} + \frac{{\frac{2}{3}}}{{\sqrt {1 – \frac{{{x^2}}}{9}} }}\) M1A1
\( = {(9 – {x^2})^{\frac{1}{2}}} – \frac{{{x^2}}}{{{{(9 – {x^2})}^{\frac{1}{2}}}}} + \frac{2}{{{{(9 – {x^2})}^{\frac{1}{2}}}}}\) A1
\( = \frac{{9 – {x^2} – {x^2} + 2}}{{{{(9 – {x^2})}^{\frac{1}{2}}}}}\) A1
\( = \frac{{11 – 2{x^2}}}{{\sqrt {9 – {x^2}} }}\) A1
[5 marks]
(d) \(\int_{ – p}^p {\frac{{11 – 2{x^2}}}{{\sqrt {9 – {x^2}} }}{\text{d}}x = \left[ {x\sqrt {9 – {x^2}} + 2\arcsin \frac{x}{3}} \right]_{ – p}^p} \) M1
\( = p\sqrt {9 – {p^2}} + 2\arcsin \frac{p}{3} + p\sqrt {9 – {p^2}} + 2\arcsin \frac{p}{3}\) A1
\( = 2p\sqrt {9 – {p^2}} + 4\arcsin \left( {\frac{p}{3}} \right)\) AG
[2 marks]
(e) \(11 – 2{p^2} = 0\) M1
\(p = 2.35\,\,\,\,\,\left( {\sqrt {\frac{{11}}{2}} } \right)\) A1
Note: Award A0 for \(p = \pm 2.35\) .
[2 marks]
(f) (i) \(f”(x) = \frac{{{{(9 – {x^2})}^{\frac{1}{2}}}( – 4x) + x(11 – 2{x^2}){{(9 – {x^2})}^{ – \frac{1}{2}}}}}{{9 – {x^2}}}\) M1A1
\( = \frac{{ – 4x(9 – {x^2}) + x(11 – 2{x^2})}}{{{{(9 – {x^2})}^{\frac{3}{2}}}}}\) A1
\( = \frac{{ – 36x + 4{x^3} + 11x – 2{x^3}}}{{{{(9 – {x^2})}^{\frac{3}{2}}}}}\) A1
\( = \frac{{x(2{x^2} – 25)}}{{{{(9 – {x^2})}^{\frac{3}{2}}}}}\) AG
(ii) EITHER
When \(0 < x < 3\), \(f”(x) < 0\). When \( – 3 < x < 0\), \(f”(x) > 0\). A1
OR
\(f”(0) = 0\) A1
THEN
Hence \(f”(x)\) changes sign through x = 0 , giving a point of inflexion. R1
EITHER
\(x = \pm \sqrt {\frac{{25}}{2}} \) is outside the domain of f. R1
OR
\(x = \pm \sqrt {\frac{{25}}{2}} \) is not a root of \(f”(x) = 0\) . R1
[7 marks]
Total [21 marks]
Examiners report
It was disappointing to note that some candidates did not know the domain for arcsin. Most candidates knew what to do in (b) but sometimes the wrong answer was obtained due to the calculator being in the wrong mode. In (c), the differentiation was often disappointing with \(\arcsin \left( {\frac{x}{3}} \right)\) causing problems. In (f)(i), some candidates who failed to do (c) guessed the correct form of \(f'(x)\) (presumably from (d)) and then went on to find \(f”(x)\) correctly. In (f)(ii), the justification of a point of inflexion at x = 0 was sometimes incorrect – for example, some candidates showed simply that \(f'(x)\) is positive on either side of the origin which is not a valid reason.
Question
The diagram shows the plan of an art gallery a metres wide. [AB] represents a doorway, leading to an exit corridor b metres wide. In order to remove a painting from the art gallery, CD (denoted by L ) is measured for various values of \(\alpha \) , as represented in the diagram.
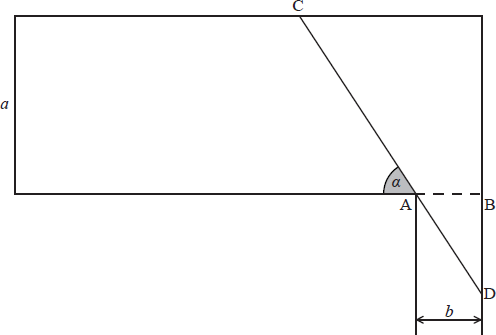
If \(\alpha \) is the angle between [CD] and the wall, show that \(L = \frac{a }{{\sin \alpha }} + \frac{b}{{\cos \alpha }}{\text{, }}0 < \alpha < \frac{\pi }{2}\).
If a = 5 and b = 1, find the maximum length of a painting that can be removed through this doorway.
Let a = 3k and b = k .
Find \(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }}\).
Let a = 3k and b = k .
Find, in terms of k , the maximum length of a painting that can be removed from the gallery through this doorway.
Let a = 3k and b = k .
Find the minimum value of k if a painting 8 metres long is to be removed through this doorway.
Answer/Explanation
Markscheme
\(L = {\text{CA}} + {\text{AD}}\) M1
\({\text{sin}}\alpha {\text{ = }}\frac{a}{{{\text{CA}}}} \Rightarrow {\text{CA}} = \frac{a}{{\sin \alpha }}\) A1
\(\cos \alpha = \frac{b}{{{\text{AD}}}} \Rightarrow {\text{AD}} = \frac{b}{{\cos \alpha }}\) A1
\(L = \frac{a}{{\sin \alpha }} + \frac{b}{{\cos \alpha }}\) AG
[2 marks]
\(a = 5{\text{ and }}b = 1 \Rightarrow L = \frac{5}{{\sin \alpha }} + \frac{1}{{\cos \alpha }}\)
METHOD 1
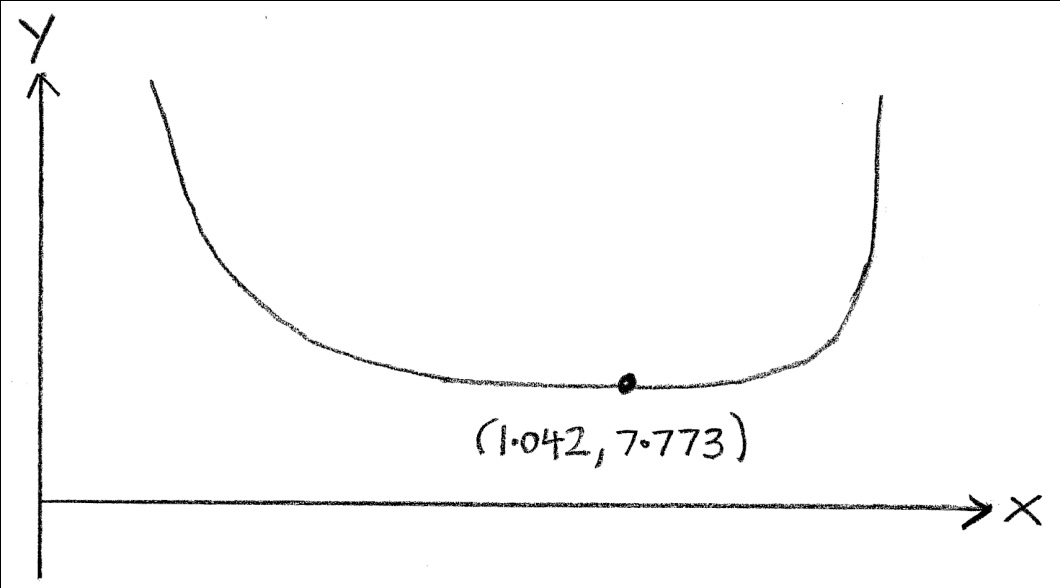 (M1)
(M1)
minimum from graph \( \Rightarrow L = 7.77\) (M1)A1
minimum of L gives the max length of the painting R1
[4 marks]
METHOD 2
\(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }} = \frac{{ – 5\cos \alpha }}{{{{\sin }^2}\alpha }} + \frac{{\sin \alpha }}{{{{\cos }^2}\alpha }}\) (M1)
\(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }} = 0 \Rightarrow \frac{{{{\sin }^3}\alpha }}{{{{\cos }^3}\alpha }} = 5 \Rightarrow \tan \alpha = \sqrt[{3{\text{ }}}]{5}{\text{ }}(\alpha = 1.0416…)\) (M1)
minimum of L gives the max length of the painting R1
maximum length = 7.77 A1
[4 marks]
\(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }} = \frac{{ – 3k\cos \alpha }}{{{{\sin }^2}\alpha }} + \frac{{k\sin \alpha }}{{{{\cos }^2}\alpha }}\,\,\,\,\,{\text{(or equivalent)}}\) M1A1A1
[3 marks]
\(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }} = \frac{{ – 3k{{\cos }^3}\alpha + k{{\sin }^3}\alpha }}{{{{\sin }^2}\alpha {{\cos }^2}\alpha }}\) (A1)
\(\frac{{{\text{d}}L}}{{{\text{d}}\alpha }} = 0 \Rightarrow \frac{{{{\sin }^3}\alpha }}{{{{\cos }^3}\alpha }} = \frac{{3k}}{k} \Rightarrow \tan \alpha = \sqrt[3]{3}\,\,\,\,\,(\alpha = 0.96454…)\) M1A1
\(\tan \alpha = \sqrt[3]{3} \Rightarrow \frac{1}{{\cos \alpha }} = \sqrt {1 + \sqrt[3]{9}} \,\,\,\,\,(1.755…)\) (A1)
\({\text{and }}\frac{1}{{\sin \alpha }} = \frac{{\sqrt {1 + \sqrt[3]{9}} }}{{\sqrt[3]{3}}}\,\,\,\,\,(1.216…)\) (A1)
\(L = 3k\left( {\frac{{\sqrt {1 + \sqrt[3]{9}} }}{{\sqrt[3]{3}}}} \right) + k\sqrt {1 + \sqrt[3]{9}} \,\,\,\,\,(L = 5.405598…k)\) A1 N4
[6 marks]
\(L \leqslant 8 \Rightarrow k \geqslant 1.48\) M1A1
the minimum value is 1.48
[2 marks]
Examiners report
Part (a) was very well done by most candidates. Parts (b), (c) and (d) required a subtle balance between abstraction, differentiation skills and use of GDC.
In part (b), although candidates were asked to justify their reasoning, very few candidates offered an explanation for the maximum. Therefore most candidates did not earn the R1 mark in part (b). Also not as many candidates as anticipated used a graphical approach, preferring to use the calculus with varying degrees of success. In part (c), some candidates calculated the derivatives of inverse trigonometric functions. Some candidates had difficulty with parts (d) and (e). In part (d), some candidates erroneously used their alpha value from part (b). In part (d) many candidates used GDC to calculate decimal values for \(\alpha \) and L. The premature rounding of decimals led sometimes to inaccurate results. Nevertheless many candidates got excellent results in this question.
Part (a) was very well done by most candidates. Parts (b), (c) and (d) required a subtle balance between abstraction, differentiation skills and use of GDC.
In part (b), although candidates were asked to justify their reasoning, very few candidates offered an explanation for the maximum. Therefore most candidates did not earn the R1 mark in part (b). Also not as many candidates as anticipated used a graphical approach, preferring to use the calculus with varying degrees of success. In part (c), some candidates calculated the derivatives of inverse trigonometric functions. Some candidates had difficulty with parts (d) and (e). In part (d), some candidates erroneously used their alpha value from part (b). In part (d) many candidates used GDC to calculate decimal values for \(\alpha \) and L. The premature rounding of decimals led sometimes to inaccurate results. Nevertheless many candidates got excellent results in this question.
Part (a) was very well done by most candidates. Parts (b), (c) and (d) required a subtle balance between abstraction, differentiation skills and use of GDC.
In part (b), although candidates were asked to justify their reasoning, very few candidates offered an explanation for the maximum. Therefore most candidates did not earn the R1 mark in part (b). Also not as many candidates as anticipated used a graphical approach, preferring to use the calculus with varying degrees of success. In part (c), some candidates calculated the derivatives of inverse trigonometric functions. Some candidates had difficulty with parts (d) and (e). In part (d), some candidates erroneously used their alpha value from part (b). In part (d) many candidates used GDC to calculate decimal values for \(\alpha \) and L. The premature rounding of decimals led sometimes to inaccurate results. Nevertheless many candidates got excellent results in this question.
Part (a) was very well done by most candidates. Parts (b), (c) and (d) required a subtle balance between abstraction, differentiation skills and use of GDC.
In part (b), although candidates were asked to justify their reasoning, very few candidates offered an explanation for the maximum. Therefore most candidates did not earn the R1 mark in part (b). Also not as many candidates as anticipated used a graphical approach, preferring to use the calculus with varying degrees of success. In part (c), some candidates calculated the derivatives of inverse trigonometric functions. Some candidates had difficulty with parts (d) and (e). In part (d), some candidates erroneously used their alpha value from part (b). In part (d) many candidates used GDC to calculate decimal values for \(\alpha \) and L. The premature rounding of decimals led sometimes to inaccurate results. Nevertheless many candidates got excellent results in this question.
Part (a) was very well done by most candidates. Parts (b), (c) and (d) required a subtle balance between abstraction, differentiation skills and use of GDC.
In part (b), although candidates were asked to justify their reasoning, very few candidates offered an explanation for the maximum. Therefore most candidates did not earn the R1 mark in part (b). Also not as many candidates as anticipated used a graphical approach, preferring to use the calculus with varying degrees of success. In part (c), some candidates calculated the derivatives of inverse trigonometric functions. Some candidates had difficulty with parts (d) and (e). In part (d), some candidates erroneously used their alpha value from part (b). In part (d) many candidates used GDC to calculate decimal values for \(\alpha \) and L. The premature rounding of decimals led sometimes to inaccurate results. Nevertheless many candidates got excellent results in this question.
Question
Let \(f(x) = \frac{{{{\text{e}}^{2x}} + 1}}{{{{\text{e}}^x} – 2}}\).
The line \({L_2}\) is parallel to \({L_1}\) and tangent to the curve \(y = f(x)\).
Find the equations of the horizontal and vertical asymptotes of the curve \(y = f(x)\).
(i) Find \(f'(x)\).
(ii) Show that the curve has exactly one point where its tangent is horizontal.
(iii) Find the coordinates of this point.
Find the equation of \({L_1}\), the normal to the curve at the point where it crosses the y-axis.
Find the equation of the line \({L_2}\).
Answer/Explanation
Markscheme
\(x \to – \infty \Rightarrow y \to – \frac{1}{2}\) so \(y = – \frac{1}{2}\) is an asymptote (M1)A1
\({{\text{e}}^x} – 2 = 0 \Rightarrow x = \ln 2\) so \(x = \ln 2{\text{ }}( = 0.693)\) is an asymptote (M1)A1
[4 marks]
(i) \(f'(x) = \frac{{2\left( {{{\text{e}}^x} – 2} \right){{\text{e}}^{2x}} – \left( {{{\text{e}}^{2x}} + 1} \right){{\text{e}}^x}}}{{{{\left( {{{\text{e}}^x} – 2} \right)}^2}}}\) M1A1
\( = \frac{{{{\text{e}}^{3x}} – 4{{\text{e}}^{2x}} – {{\text{e}}^x}}}{{{{\left( {{{\text{e}}^x} – 2} \right)}^2}}}\)
(ii) \(f'(x) = 0\) when \({{\text{e}}^{3x}} – 4{{\text{e}}^{2x}} – {{\text{e}}^x} = 0\) M1
\({{\text{e}}^x}\left( {{{\text{e}}^{2x}} – 4{{\text{e}}^x} – 1} \right) = 0\)
\({{\text{e}}^x} = 0,{\text{ }}{{\text{e}}^x} = – 0.236,{\text{ }}{{\text{e}}^x} = 4.24{\text{ }}({\text{or }}{{\text{e}}^x} = 2 \pm \sqrt 5 )\) A1A1
Note: Award A1 for zero, A1 for other two solutions.
Accept any answers which show a zero, a negative and a positive.
as \({{\text{e}}^x} > 0\) exactly one solution R1
Note: Do not award marks for purely graphical solution.
(iii) (1.44, 8.47) A1A1
[8 marks]
\(f'(0) = – 4\) (A1)
so gradient of normal is \(\frac{1}{4}\) (M1)
\(f(0) = – 2\) (A1)
so equation of \({L_1}\) is \(y = \frac{1}{4}x – 2\) A1
[4 marks]
\(f'(x) = \frac{1}{4}\) M1
so \(x = 1.46\) (M1)A1
\(f(1.46) = 8.47\) (A1)
equation of \({L_2}\) is \(y – 8.47 = \frac{1}{4}(x – 1.46)\) A1
(or \(y = \frac{1}{4}x + 8.11\))
[5 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
Question
The following diagram shows a vertical cross section of a building. The cross section of the roof of the building can be modelled by the curve \(f(x) = 30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\), where \( – 20 \le x \le 20\).
Ground level is represented by the \(x\)-axis.
Find \(f”(x)\).
Show that the gradient of the roof function is greatest when \(x = – \sqrt {200} \).
The cross section of the living space under the roof can be modelled by a rectangle \(CDEF\) with points \({\text{C}}( – a,{\text{ }}0)\) and \({\text{D}}(a,{\text{ }}0)\), where \(0 < a \le 20\).
Show that the maximum area \(A\) of the rectangle \(CDEF\) is \(600\sqrt 2 {{\text{e}}^{ – \frac{1}{2}}}\).
A function \(I\) is known as the Insulation Factor of \(CDEF\). The function is defined as \(I(a) = \frac{{P(a)}}{{A(a)}}\) where \({\text{P}} = {\text{Perimeter}}\) and \({\text{A}} = {\text{Area of the rectangle}}\).
(i) Find an expression for \(P\) in terms of \(a\).
(ii) Find the value of \(a\) which minimizes \(I\).
(iii) Using the value of \(a\) found in part (ii) calculate the percentage of the cross sectional area under the whole roof that is not included in the cross section of the living space.
Answer/Explanation
Markscheme
\(f'(x) = 30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}} \bullet – \frac{{2x}}{{400}}\;\;\;\left( { = – \frac{{3x}}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}} \right)\) M1A1
Note: Award M1 for attempting to use the chain rule.
\(f”(x) = – \frac{3}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}} + \frac{{3{x^2}}}{{4000}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\;\;\;\left( { = \frac{3}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\left( {\frac{{{x^2}}}{{200}} – 1} \right)} \right)\) M1A1
Note: Award M1 for attempting to use the product rule.
[4 marks]
the roof function has maximum gradient when \(f”(x) = 0\) (M1)
Note: Award (M1) for attempting to find \(f”\left( { – \sqrt {200} } \right)\).
EITHER
\( = 0\) A1
OR
\(f”(x) = 0 \Rightarrow x = \pm \sqrt {200} \) A1
THEN
valid argument for maximum such as reference to an appropriate graph or change in the sign of \(f”(x)\) eg \(f”( – 15) = 0.010 \ldots ( > 0)\) and \(f”( – 14) = – 0.001 \ldots ( < 0)\) R1
\( \Rightarrow x = – \sqrt {200} \) AG
[3 marks]
\(A = 2a \bullet 30{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}\;\;\;\left( { = 60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} = – 400g'(a)} \right)\) (M1)(A1)
EITHER
\(\frac{{{\text{d}}A}}{{{\text{d}}a}} = 60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} \bullet – \frac{a}{{200}} + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} = 0 \Rightarrow a = \sqrt {200} {\text{ }}\left( { – 400f”(a) = 0 \Rightarrow a = \sqrt {200} } \right)\) M1A1
OR
by symmetry eg \(a = – \sqrt {200} \) found in (b) or \({A_{{\text{max}}}}\) coincides with \(f”(a) = 0\) R1
\( \Rightarrow a = \sqrt {200} \) A1
Note: Award A0(M1)(A1)M0M1 for candidates who start with \(a = \sqrt {200} \) and do not provide any justification for the maximum area. Condone use of \(x\).
THEN
\({A_{{\text{max}}}} = 60 \bullet \sqrt {200} {{\text{e}}^{ – \frac{{200}}{{400}}}}\) M1
\( = 600\sqrt 2 {{\text{e}}^{ – \frac{1}{2}}}\) AG
[5 marks]
(i) perimeter \( = 4a + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}\) A1A1
Note: Condone use of \(x\).
(ii) \(I(a) = \frac{{4a + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}}}{{60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}}}\) (A1)
graphing \(I(a)\) or other valid method to find the minimum (M1)
\(a = 12.6\) A1
(iii) area under roof \( = \int_{ – 20}^{20} {30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}} {\text{d}}x\) M1
\( = 896.18 \ldots \) (A1)
area of living space \( = 60 \cdot (12.6…) \cdot e – {\frac{{(12.6…)}}{{400}}^2} = 508.56…\)
percentage of empty space \( = 43.3\% \) A1
[9 marks]
Total [21 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider the curve, \(C\) defined by the equation \({y^2} – 2xy = 5 – {{\text{e}}^x}\). The point A lies on \(C\) and has coordinates \((0,{\text{ }}a),{\text{ }}a > 0\).
Find the value of \(a\).
Show that \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{2y – {{\text{e}}^x}}}{{2(y – x)}}\).
Find the equation of the normal to \(C\) at the point A.
Find the coordinates of the second point at which the normal found in part (c) intersects \(C\).
Given that \(v = {y^3},{\text{ }}y > 0\), find \(\frac{{{\text{d}}v}}{{{\text{d}}x}}\) at \(x = 0\).
Answer/Explanation
Markscheme
\({a^2} = 5 – 1\) (M1)
\(a = 2\) A1
[2 marks]
\(2y\frac{{{\text{d}}y}}{{{\text{d}}x}} – \left( {2x\frac{{{\text{d}}y}}{{{\text{d}}x}} + 2y} \right) = – {{\text{e}}^x}\) M1A1A1A1
Note: Award M1 for an attempt at implicit differentiation, A1 for each part.
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{2y – {{\text{e}}^x}}}{{2(y – x)}}\) AG
[4 marks]
at \(x = 0,{\text{ }}\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{3}{4}\) (A1)
finding the negative reciprocal of a number (M1)
gradient of normal is \( – \frac{4}{3}\)
\(y = – \frac{4}{3}x + 2\) A1
[3 marks]
substituting linear expression (M1)
\({\left( { – \frac{4}{3}x + 2} \right)^2} – 2x\left( { – \frac{4}{3}x + 2} \right) + {{\text{e}}^x} – 5 = 0\) or equivalent
\(x = 1.56\) (M1)A1
\(y = – 0.0779\) A1
\((1.56,{\text{ }} – 0.0779)\)
[4 marks]
\(\frac{{{\text{d}}v}}{{{\text{d}}x}} = 3{y^2}\frac{{{\text{d}}y}}{{{\text{d}}x}}\) M1A1
\(\frac{{{\text{d}}v}}{{{\text{d}}x}} = 3 \times 4 \times \frac{3}{4} = 9\) A1
[3 marks]
Examiners report
Parts (a) to (c) were generally well done.
Parts (a) to (c) were generally well done.
Parts (a) to (c) were generally well done although a significant number of students found the equation of the tangent rather than the normal in part (c).
Whilst many were able to make a start on part (d), fewer students had the necessary calculator skills to work it though correctly.
There were many overly complicated solutions to part (e), some of which were successful.
Question
The functions \(f\) and \(g\) are defined by
\[f(x) = \frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2},{\text{ }}x \in \mathbb{R}\]
\[g(x) = \frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{2},{\text{ }}x \in \mathbb{R}\]
Let \(h(x) = nf(x) + g(x)\) where \(n \in \mathbb{R},{\text{ }}n > 1\).
Let \(t(x) = \frac{{g(x)}}{{f(x)}}\).
(i) Show that \(\frac{1}{{4f(x) – 2g(x)}} = \frac{{{{\text{e}}^x}}}{{{{\text{e}}^{2x}} + 3}}\).
(ii) Use the substitution \(u = {{\text{e}}^x}\) to find \(\int_0^{\ln 3} {\frac{1}{{4f(x) – 2g(x)}}} {\text{d}}x\). Give your answer in the form \(\frac{{\pi \sqrt a }}{b}\) where \(a,{\text{ }}b \in {\mathbb{Z}^ + }\).
(i) By forming a quadratic equation in \({{\text{e}}^x}\), solve the equation \(h(x) = k\), where \(k \in {\mathbb{R}^ + }\).
(ii) Hence or otherwise show that the equation \(h(x) = k\) has two real solutions provided that \(k > \sqrt {{n^2} – 1} \) and \(k \in {\mathbb{R}^ + }\).
(i) Show that \(t'(x) = \frac{{{{[f(x)]}^2} – {{[g(x)]}^2}}}{{{{[f(x)]}^2}}}\) for \(x \in \mathbb{R}\).
(ii) Hence show that \(t'(x) > 0\) for \(x \in \mathbb{R}\).
Answer/Explanation
Markscheme
(i) \(\frac{1}{{4\left( {\frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2}} \right) – 2\left( {\frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{2}} \right)}}\) (M1)
\( = \frac{1}{{2({{\text{e}}^x} + {{\text{e}}^{ – x}}) – ({{\text{e}}^x} – {{\text{e}}^{ – x}})}}\) (A1)
\( = \frac{1}{{{{\text{e}}^x} + 3{{\text{e}}^{ – x}}}}\) A1
\( = \frac{{{{\text{e}}^x}}}{{{{\text{e}}^{2x}} + 3}}\) AG
(ii) \(u = {{\text{e}}^x} \Rightarrow {\text{d}}u = {{\text{e}}^x}{\text{d}}x\) A1
\(\int {\frac{{{{\text{e}}^x}}}{{{{\text{e}}^{2x}} + 3}}{\text{d}}x = \int {\frac{1}{{{u^2} + 3}}{\text{d}}u} } \) M1
(when \(x = 0,{\text{ }}u = 1\) and when \(x = \ln 3,{\text{ }}u = 3\))
\(\int_1^3 {\frac{1}{{{u^2} + 3}}{\text{d}}u\left[ {\frac{1}{{\sqrt 3 }}\arctan \left( {\frac{u}{{\sqrt 3 }}} \right)} \right]_1^3} \) M1A1
\(\left( { = \left[ {\frac{1}{{\sqrt 3 }}\arctan \left( {\frac{{{{\text{e}}^x}}}{{\sqrt 3 }}} \right)} \right]_0^{\ln 3}} \right)\)
\( = \frac{{\pi \sqrt 3 }}{9} – \frac{{\pi \sqrt 3 }}{{18}}\) (M1)
\( = \frac{{\pi \sqrt 3 }}{{18}}\) A1
[9 marks]
(i) \((n + 1){{\text{e}}^{2x}} – 2k{{\text{e}}^x} + (n – 1) = 0\) M1A1
\({{\text{e}}^x} = \frac{{2k \pm \sqrt {4{k^2} – 4({n^2} – 1)} }}{{2(n + 1)}}\) M1
\(x = \ln \left( {\frac{{k \pm \sqrt {{k^2} – {n^2} + 1} }}{{n + 1}}} \right)\) M1A1
(ii) for two real solutions, we require \(k > \sqrt {{k^2} – {n^2} + 1} \) R1
and we also require \({k^2} – {n^2} + 1 > 0\) R1
\({k^2} > {n^2} – 1\) A1
\( \Rightarrow k > \sqrt {{n^2} – 1} {\text{ }}({\text{ }}k \in {\mathbb{R}^ + })\) AG
[8 marks]
METHOD 1
\(t(x) = \frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}\)
\(t'(x) = \frac{{{{({{\text{e}}^x} + {{\text{e}}^{ – x}})}^2} – {{({{\text{e}}^x} – {{\text{e}}^{ – x}})}^2}}}{{{{({{\text{e}}^x} + {{\text{e}}^{ – x}})}^2}}}\) M1A1
\(t'(x) = \frac{{{{\left( {\frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2}} \right)}^2} – {{\left( {\frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{2}} \right)}^2}}}{{{{\left( {\frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2}} \right)}^2}}}\) A1
\( = \frac{{{{\left[ {f(x)} \right]}^2} – {{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) AG
METHOD 2
\(t'(x) = \frac{{f(x)g'(x) = g(x)f'(x)}}{{{{\left[ {f(x)} \right]}^2}}}\) M1A1
\(g'(x) = f(x)\) and \(f'(x) = g(x)\) A1
\( = \frac{{{{\left[ {f(x)} \right]}^2} – {{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) AG
METHOD 3
\(t(x) = ({{\text{e}}^x} – {{\text{e}}^{ – x}}){({{\text{e}}^x} + {{\text{e}}^{ – x}})^{ – 1}}\)
\(t'(x) = 1 – \frac{{{{({{\text{e}}^x} – {{\text{e}}^{ – x}})}^2}}}{{{{({{\text{e}}^x} + {{\text{e}}^{ – x}})}^2}}}\) M1A1
\( = 1 – \frac{{{{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) A1
\( = \frac{{{{\left[ {f(x)} \right]}^2} – {{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) AG
METHOD 4
\(t'(x) = \frac{{g'(x)}}{{f(x)}} – \frac{{g(x)f'(x)}}{{{{\left[ {f(x)} \right]}^2}}}\) M1A1
\(g'(x) = f(x)\) and \(f'(x) = g(x)\) gives \(t'(x) = 1 – \frac{{{{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) A1
\( = \frac{{{{\left[ {f(x)} \right]}^2} – {{\left[ {g(x)} \right]}^2}}}{{{{\left[ {f(x)} \right]}^2}}}\) AG
(ii) METHOD 1
\({\left[ {f(x)} \right]^2} > {\left[ {g(x)} \right]^2}\) (or equivalent) M1A1
\({\left[ {f(x)} \right]^2} > 0\) R1
hence \(t'(x) > 0,{\text{ }}x \in \mathbb{R}\) AG
Note: Award as above for use of either \(f(x) = \frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2}\) and \(g(x) = \frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{2}\) or \({{\text{e}}^x} + {{\text{e}}^{ – x}}\) and \({{\text{e}}^x} – {{\text{e}}^{ – x}}\).
METHOD 2
\({\left[ {f(x)} \right]^2} – {\left[ {g(x)} \right]^2} = 1\) (or equivalent) M1A1
\({\left[ {f(x)} \right]^2} > 0\) R1
hence \(t'(x) > 0,{\text{ }}x \in \mathbb{R}\) AG
Note: Award as above for use of either \(f(x) = \frac{{{{\text{e}}^x} + {{\text{e}}^{ – x}}}}{2}\) and \(g(x) = \frac{{{{\text{e}}^x} – {{\text{e}}^{ – x}}}}{2}\) or \({{\text{e}}^x} + {{\text{e}}^{ – x}}\) and \({{\text{e}}^x} – {{\text{e}}^{ – x}}\).
METHOD 3
\(t'(x) = \frac{4}{{{{({{\text{e}}^x} + {{\text{e}}^{ – x}})}^2}}}\)
\({\left( {{{\text{e}}^x} + {{\text{e}}^{ – x}}} \right)^2} > 0\) M1A1
\(\frac{4}{{{{\left( {{{\text{e}}^x} + {{\text{e}}^{ – x}}} \right)}^2}}} > 0\) R1
hence \(t'(x) > 0,{\text{ }}x \in \mathbb{R}\) AG
[6 marks]
Examiners report
Parts (a) and (c) were accessible to the large majority of candidates. Candidates found part (b) considerably more challenging.
Part (a)(i) was reasonably well done with most candidates able to show that \(\frac{1}{{4f(x) – 2g(x)}} = \frac{{{{\text{e}}^x}}}{{{{\text{e}}^{2x}} + 3}}\). In part (a)(ii), a number of candidates correctly used the required substitution to obtain \(\int {\frac{{{{\text{e}}^x}}}{{{{\text{e}}^{2x}} + 3}}{\text{d}}x = \int {\frac{1}{{{u^2} + 3}}{\text{d}}u} } \) but then thought that the antiderivative involved natural log rather than arctan.
Parts (a) and (c) were accessible to the large majority of candidates. Candidates found part (b) considerably more challenging.
In part (b)(i), a reasonable number of candidates were able to form a quadratic in \({{\text{e}}^x}\) (involving parameters \(n\) and \(k\)) and then make some progress towards solving for \({{\text{e}}^x}\) in terms of \(n\) and \(k\). Having got that far, a small number of candidates recognised to then take the natural logarithm of both sides and hence solve \(h(x) = k\) for \(\chi \). In part (b)(ii), a small number of candidates were able to show from their solutions to part (b)(i) or through the use of the discriminant that the equation \(h(x) = k\) has two real solutions provided that \(k > \sqrt {{k^2} – {n^2} + 1} \) and \(k > \sqrt {{n^2} – 1} \).
Parts (a) and (c) were accessible to the large majority of candidates. Candidates found part (b) considerably more challenging.
It was pleasing to see the number of candidates who attempted part (c). In part (c)(i), a large number of candidates were able to correctly apply either the quotient rule or the product rule to find \(t'(x)\). A smaller number of candidates were then able to show equivalence between the form of \(t'(x)\) they had obtained and the form of \(t'(x)\) required in the question. A pleasing number of candidates were able to exploit the property that \(f'(x) = g(x)\) and \(g'(x) = f(x)\). As with part (c)(i), part (c)(ii) could be successfully tackled in a number of ways. The best candidates offered concise logical reasoning to show that \(t'(x) > 0\) for \(x \in \mathbb{R}\).
Question
An earth satellite moves in a path that can be described by the curve \(72.5{x^2} + 71.5{y^2} = 1\) where \(x = x(t)\) and \(y = y(t)\) are in thousands of kilometres and \(t\) is time in seconds.
Given that \(\frac{{{\text{d}}x}}{{{\text{d}}t}} = 7.75 \times {10^{ – 5}}\) when \(x = 3.2 \times {10^{ – 3}}\), find the possible values of \(\frac{{{\text{d}}y}}{{{\text{d}}t}}\).
Give your answers in standard form.
Answer/Explanation
Markscheme
METHOD 1
substituting for \(x\) and attempting to solve for \(y\) (or vice versa) (M1)
\(y = ( \pm )0.11821 \ldots \) (A1)
EITHER
\(145x + 143y\frac{{{\text{d}}y}}{{{\text{d}}x}} = 0{\text{ }}\left( {\frac{{{\text{d}}y}}{{{\text{d}}x}} = – \frac{{145x}}{{143y}}} \right)\) M1A1
OR
\(145x\frac{{{\text{d}}x}}{{{\text{d}}t}} + 143y\frac{{{\text{d}}y}}{{{\text{d}}t}} = 0\) M1A1
THEN
attempting to find \(\frac{{{\text{d}}x}}{{{\text{d}}t}}{\text{ }}\left( {\frac{{{\text{d}}y}}{{{\text{d}}t}} = – \frac{{145(3.2 \times {{10}^{ – 3}})}}{{143\left( {( \pm )0.11821 \ldots } \right)}} \times (7.75 \times {{10}^{ – 5}})} \right)\) (M1)
\(\frac{{{\text{d}}y}}{{{\text{d}}t}} = \pm 2.13 \times {10^{ – 6}}\) A1
Note: Award all marks except the final A1 to candidates who do not consider ±.
METHOD 2
\(y = ( \pm )\sqrt {\frac{{1 – 72.5{x^2}}}{{71.5}}} \) M1A1
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = ( \pm )0.0274 \ldots \) (M1)(A1)
\(\frac{{{\text{d}}y}}{{{\text{d}}t}} = ( \pm )0.0274 \ldots \times 7.75 \times {10^{ – 5}}\) (M1)
\(\frac{{{\text{d}}y}}{{{\text{d}}t}} = \pm 2.13 \times {10^{ – 6}}\) A1
Note: Award all marks except the final A1 to candidates who do not consider ±.
[6 marks]
Examiners report
Question
Let the function \(f\) be defined by \(f(x) = \frac{{2 – {{\text{e}}^x}}}{{2{{\text{e}}^x} – 1}},{\text{ }}x \in D\).
Determine \(D\), the largest possible domain of \(f\).
Show that the graph of \(f\) has three asymptotes and state their equations.
Show that \(f'(x) = – \frac{{3{{\text{e}}^x}}}{{{{(2{{\text{e}}^x} – 1)}^2}}}\).
Use your answers from parts (b) and (c) to justify that \(f\) has an inverse and state its domain.
Find an expression for \({f^{ – 1}}(x)\).
Consider the region \(R\) enclosed by the graph of \(y = f(x)\) and the axes.
Find the volume of the solid obtained when \(R\) is rotated through \(2\pi \) about the \(y\)-axis.
Answer/Explanation
Markscheme
attempting to solve either \(2{{\text{e}}^x} – 1 = 0\) or \(2{{\text{e}}^x} – 1 \ne 0\) for \(x\) (M1)
\(D = \mathbb{R}\backslash \left\{ { – \ln 2} \right\}\) (or equivalent eg \(x \ne – \ln 2\)) A1
Note: Accept \(D = \mathbb{R}\backslash \left\{ { – 0.693} \right\}\) or equivalent eg \(x \ne – 0.693\).
[2 marks]
considering \(\mathop {\lim }\limits_{x \to – \ln 2} f(x)\) (M1)
\(x = – \ln 2{\text{ }}(x = – 0.693)\) A1
considering one of \(\mathop {\lim }\limits_{x \to – \infty } f(x)\) or \(\mathop {\lim }\limits_{x \to + \infty } f(x)\) M1
\(\mathop {\lim }\limits_{x \to – \infty } f(x) = – 2 \Rightarrow y = – 2\) A1
\(\mathop {\lim }\limits_{x \to + \infty } f(x) = – \frac{1}{2} \Rightarrow y = – \frac{1}{2}\) A1
Note: Award A0A0 for \(y = – 2\) and \(y = – \frac{1}{2}\) stated without any justification.
[5 marks]
\(f'(x) = \frac{{ – {{\text{e}}^x}(2{{\text{e}}^x} – 1) – 2{{\text{e}}^x}(2 – {{\text{e}}^x})}}{{{{(2{{\text{e}}^x} – 1)}^2}}}\) M1A1A1
\( = – \frac{{3{{\text{e}}^x}}}{{{{(2{{\text{e}}^x} – 1)}^2}}}\) AG
[3 marks]
\(f'(x) < 0{\text{ (for all }}x \in D) \Rightarrow f\) is (strictly) decreasing R1
Note: Award R1 for a statement such as \(f'(x) \ne 0\) and so the graph of \(f\) has no turning points.
one branch is above the upper horizontal asymptote and the other branch is below the lower horizontal asymptote R1
\(f\) has an inverse AG
\( – \infty < x < – 2 \cup – \frac{1}{2} < x < \infty \) A2
Note: Award A2 if the domain of the inverse is seen in either part (d) or in part (e).
[4 marks]
\(x = \frac{{2 – {{\text{e}}^y}}}{{2{{\text{e}}^y} – 1}}\) M1
Note: Award M1 for interchanging \(x\) and \(y\) (can be done at a later stage).
\(2x{{\text{e}}^y} – x = 2 – {{\text{e}}^y}\) M1
\({{\text{e}}^y}(2x + 1) = x + 2\) A1
\({f^{ – 1}}(x) = \ln \left( {\frac{{x + 2}}{{2x + 1}}} \right){\text{ }}\left( {{f^{ – 1}}(x) = \ln (x + 2) – \ln (2x + 1)} \right)\) A1
[4 marks]
use of \(V = \pi \int_a^b {{x^2}{\text{d}}y} \) (M1)
\( = \pi \int_0^1 {{{\left( {\ln \left( {\frac{{y + 2}}{{2y + 1}}} \right)} \right)}^2}{\text{d}}y} \) (A1)(A1)
Note: Award (A1) for the correct integrand and (A1) for the limits.
\( = 0.331\) A1
[4 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
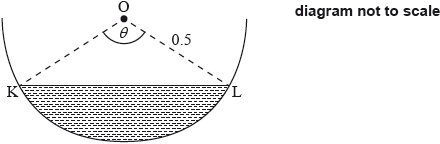
A water trough which is 10 metres long has a uniform cross-section in the shape of a semicircle with radius 0.5 metres. It is partly filled with water as shown in the following diagram of the cross-section. The centre of the circle is O and the angle KOL is \(\theta \) radians.
The volume of water is increasing at a constant rate of \(0.0008{\text{ }}{{\text{m}}^3}{{\text{s}}^{ – 1}}\).
Find an expression for the volume of water \(V{\text{ }}({{\text{m}}^3})\) in the trough in terms of \(\theta \).
Calculate \(\frac{{{\text{d}}\theta }}{{{\text{d}}t}}\) when \(\theta = \frac{\pi }{3}\).
Answer/Explanation
Markscheme
area of segment \( = \frac{1}{2} \times {0.5^2} \times (\theta – \sin \theta )\) M1A1
\(V = {\text{area of segment}} \times 10\)
\(V = \frac{5}{4}(\theta – \sin \theta )\) A1
[3 marks]
METHOD 1
\(\frac{{{\text{d}}V}}{{{\text{d}}t}} = \frac{5}{4}(1 – \cos \theta )\frac{{{\text{d}}\theta }}{{{\text{d}}t}}\) M1A1
\(0.0008 = \frac{5}{4}\left( {1 – \cos \frac{\pi }{3}} \right)\frac{{{\text{d}}\theta }}{{{\text{d}}t}}\) (M1)
\(\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = 0.00128{\text{ }}({\text{rad}}\,{s^{ – 1}})\) A1
METHOD 2
\(\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = \frac{{{\text{d}}\theta }}{{{\text{d}}V}} \times \frac{{{\text{d}}V}}{{{\text{d}}t}}\) (M1)
\(\frac{{{\text{d}}V}}{{{\text{d}}\theta }} = \frac{5}{4}(1 – \cos \theta )\) A1
\(\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = \frac{{4 \times 0.0008}}{{5\left( {1 – \cos \frac{\pi }{3}} \right)}}\) (M1)
\(\frac{{{\text{d}}\theta }}{{{\text{d}}t}} = 0.00128\left( {\frac{4}{{3125}}} \right)({\text{rad }}{s^{ – 1}})\) A1
[4 marks]
Examiners report
[N/A]
[N/A]
Question
Consider \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right)\)
The function \(f\) is defined by \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in D\)
The function \(g\) is defined by \(g(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in \left] {1,{\text{ }}\infty } \right[\).
Find the largest possible domain \(D\) for \(f\) to be a function.
Sketch the graph of \(y = f(x)\) showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
Explain why \(f\) is an even function.
Explain why the inverse function \({f^{ – 1}}\) does not exist.
Find the inverse function \({g^{ – 1}}\) and state its domain.
Find \(g'(x)\).
Hence, show that there are no solutions to \(g'(x) = 0\);
Hence, show that there are no solutions to \(({g^{ – 1}})'(x) = 0\).
Answer/Explanation
Markscheme
\({x^2} – 1 > 0\) (M1)
\(x < – 1\) or \(x > 1\) A1
[2 marks]
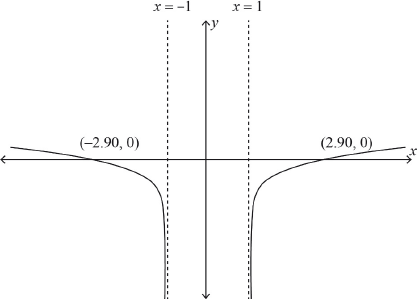
shape A1
\(x = 1\) and \(x = – 1\) A1
\(x\)-intercepts A1
[3 marks]
EITHER
\(f\) is symmetrical about the \(y\)-axis R1
OR
\(f( – x) = f(x)\) R1
[1 mark]
EITHER
\(f\) is not one-to-one function R1
OR
horizontal line cuts twice R1
Note: Accept any equivalent correct statement.
[1 mark]
\(x = – 1 + \ln \left( {\sqrt {{y^2} – 1} } \right)\) M1
\({{\text{e}}^{2x + 2}} = {y^2} – 1\) M1
\({g^{ – 1}}(x) = \sqrt {{{\text{e}}^{2x + 2}} + 1} ,{\text{ }}x \in \mathbb{R}\) A1A1
[4 marks]
\(g'(x) = \frac{1}{{\sqrt {{x^2} – 1} }} \times \frac{{2x}}{{2\sqrt {{x^2} – 1} }}\) M1A1
\(g'(x) = \frac{x}{{{x^2} – 1}}\) A1
[3 marks]
\(g'(x) = \frac{x}{{{x^2} – 1}} = 0 \Rightarrow x = 0\) M1
which is not in the domain of \(g\) (hence no solutions to \(g'(x) = 0\)) R1
[2 marks]
\(({g^{ – 1}})'(x) = \frac{{{{\text{e}}^{2x + 2}}}}{{\sqrt {{{\text{e}}^{2x + 2}} + 1} }}\) M1
as \({{\text{e}}^{2x + 2}} > 0 \Rightarrow ({g^{ – 1}})'(x) > 0\) so no solutions to \(({g^{ – 1}})'(x) = 0\) R1
Note: Accept: equation \({{\text{e}}^{2x + 2}} = 0\) has no solutions.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right)\)
The function \(f\) is defined by \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in D\)
The function \(g\) is defined by \(g(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in \left] {1,{\text{ }}\infty } \right[\).
Find the largest possible domain \(D\) for \(f\) to be a function.
Sketch the graph of \(y = f(x)\) showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
Explain why \(f\) is an even function.
Explain why the inverse function \({f^{ – 1}}\) does not exist.
Find the inverse function \({g^{ – 1}}\) and state its domain.
Find \(g'(x)\).
Hence, show that there are no solutions to \(g'(x) = 0\);
Hence, show that there are no solutions to \(({g^{ – 1}})'(x) = 0\).
Answer/Explanation
Markscheme
\({x^2} – 1 > 0\) (M1)
\(x < – 1\) or \(x > 1\) A1
[2 marks]

shape A1
\(x = 1\) and \(x = – 1\) A1
\(x\)-intercepts A1
[3 marks]
EITHER
\(f\) is symmetrical about the \(y\)-axis R1
OR
\(f( – x) = f(x)\) R1
[1 mark]
EITHER
\(f\) is not one-to-one function R1
OR
horizontal line cuts twice R1
Note: Accept any equivalent correct statement.
[1 mark]
\(x = – 1 + \ln \left( {\sqrt {{y^2} – 1} } \right)\) M1
\({{\text{e}}^{2x + 2}} = {y^2} – 1\) M1
\({g^{ – 1}}(x) = \sqrt {{{\text{e}}^{2x + 2}} + 1} ,{\text{ }}x \in \mathbb{R}\) A1A1
[4 marks]
\(g'(x) = \frac{1}{{\sqrt {{x^2} – 1} }} \times \frac{{2x}}{{2\sqrt {{x^2} – 1} }}\) M1A1
\(g'(x) = \frac{x}{{{x^2} – 1}}\) A1
[3 marks]
\(g'(x) = \frac{x}{{{x^2} – 1}} = 0 \Rightarrow x = 0\) M1
which is not in the domain of \(g\) (hence no solutions to \(g'(x) = 0\)) R1
[2 marks]
\(({g^{ – 1}})'(x) = \frac{{{{\text{e}}^{2x + 2}}}}{{\sqrt {{{\text{e}}^{2x + 2}} + 1} }}\) M1
as \({{\text{e}}^{2x + 2}} > 0 \Rightarrow ({g^{ – 1}})'(x) > 0\) so no solutions to \(({g^{ – 1}})'(x) = 0\) R1
Note: Accept: equation \({{\text{e}}^{2x + 2}} = 0\) has no solutions.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right)\)
The function \(f\) is defined by \(f(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in D\)
The function \(g\) is defined by \(g(x) = – 1 + \ln \left( {\sqrt {{x^2} – 1} } \right),{\text{ }}x \in \left] {1,{\text{ }}\infty } \right[\).
Find the largest possible domain \(D\) for \(f\) to be a function.
Sketch the graph of \(y = f(x)\) showing clearly the equations of asymptotes and the coordinates of any intercepts with the axes.
Explain why \(f\) is an even function.
Explain why the inverse function \({f^{ – 1}}\) does not exist.
Find the inverse function \({g^{ – 1}}\) and state its domain.
Find \(g'(x)\).
Hence, show that there are no solutions to \(g'(x) = 0\);
Hence, show that there are no solutions to \(({g^{ – 1}})'(x) = 0\).
Answer/Explanation
Markscheme
\({x^2} – 1 > 0\) (M1)
\(x < – 1\) or \(x > 1\) A1
[2 marks]

shape A1
\(x = 1\) and \(x = – 1\) A1
\(x\)-intercepts A1
[3 marks]
EITHER
\(f\) is symmetrical about the \(y\)-axis R1
OR
\(f( – x) = f(x)\) R1
[1 mark]
EITHER
\(f\) is not one-to-one function R1
OR
horizontal line cuts twice R1
Note: Accept any equivalent correct statement.
[1 mark]
\(x = – 1 + \ln \left( {\sqrt {{y^2} – 1} } \right)\) M1
\({{\text{e}}^{2x + 2}} = {y^2} – 1\) M1
\({g^{ – 1}}(x) = \sqrt {{{\text{e}}^{2x + 2}} + 1} ,{\text{ }}x \in \mathbb{R}\) A1A1
[4 marks]
\(g'(x) = \frac{1}{{\sqrt {{x^2} – 1} }} \times \frac{{2x}}{{2\sqrt {{x^2} – 1} }}\) M1A1
\(g'(x) = \frac{x}{{{x^2} – 1}}\) A1
[3 marks]
\(g'(x) = \frac{x}{{{x^2} – 1}} = 0 \Rightarrow x = 0\) M1
which is not in the domain of \(g\) (hence no solutions to \(g'(x) = 0\)) R1
[2 marks]
\(({g^{ – 1}})'(x) = \frac{{{{\text{e}}^{2x + 2}}}}{{\sqrt {{{\text{e}}^{2x + 2}} + 1} }}\) M1
as \({{\text{e}}^{2x + 2}} > 0 \Rightarrow ({g^{ – 1}})'(x) > 0\) so no solutions to \(({g^{ – 1}})'(x) = 0\) R1
Note: Accept: equation \({{\text{e}}^{2x + 2}} = 0\) has no solutions.
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
