Focus Question: How is electric potential different from electric field strength?
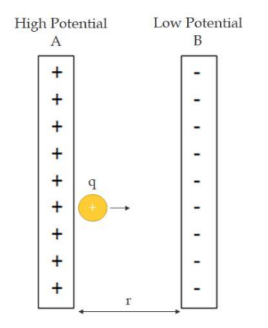
- The change in electric potential is the change in electric potential energy when a charge, q, moves from one point to another. The change in electric potential is also equal to the amount of work the electric field would have to do to move the charge.
- Charges tend to move away from like charges and towards opposite charges. When charges move in a field, there is a change in electrical potential energy.
*The change in electric potential energy is also equal to the amount of work the electric field would have to do to move the charge.
$
\begin{aligned}
& U_A-U_B=\text { Work }=\mathrm{Fr}=\frac{K Q q}{r^2} r=\frac{K Q q}{r} \\
& U_A-U_B=\text { Eqr }
\end{aligned}
$
*Units of electric potential energy: Joules (J)
- Electric Potential is the measure of the amount of work a charge can do on another.
$
\mathrm{V}=\frac{\text { Potential Energy }}{\text { charge }}=\frac{- \text { Work required to move charge }}{q}=\frac{K Q}{r}
$
Units: Volts (V)
Potential $=$ electric field $x$ distance $->V=E d$
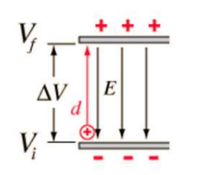
- Work done by a field on a charge:
$
\mathrm{V}=-\frac{W}{q} \rightarrow W=V q
$
Electric potential from point charges.
Example A: A particle of mass $6.3 \times 10^{-3} \mathrm{~kg}$ and charge $9.0 \times 10^{-4} \mathrm{C}$ floats between two horizontal plates that are $25 \mathrm{~cm}$ apart. What is the potential difference between the plates?
Answer/Explanation
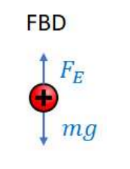
Since the charge floats, the electrostatic force cancels out the force of gravity.
$
\begin{aligned}
& \sum F_y=0 \rightarrow F_E-m g=0 \rightarrow F_E=m g \\
& \rightarrow F_E=E q=\frac{V}{d} q \rightarrow \frac{V q}{d}=m g \rightarrow V=\frac{m g d}{q} \\
& \rightarrow V=\frac{\left(6.3 \times 10^{-3} \mathrm{~kg}\right)\left(10 \frac{\mathrm{m}}{\mathrm{s}^2}\right) .25 \mathrm{~m}}{9.4 \times 10^{-4} \mathrm{C}}=\mathbf{1 6 . 8} \mathrm{V}
\end{aligned}
$
Example B: Two charges are located $6 \mathrm{~m}$ from each other as shown. Point $P$ is located $6 \mathrm{~m}$ from each of the two charges. $q_1=-4 \mu \mathrm{C}, q_2=1 \mu \mathrm{C}$
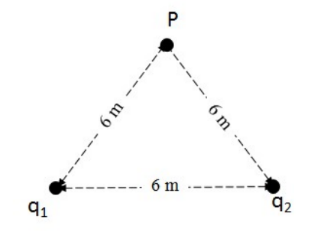
*the superposition principle still applies for potential*
a) Calculate the electric potential at point $P$.
b) Calculate the work required to bring a charge of magnitude of $20 n C$ from infinity to point $\mathrm{P}$.
Answer/Explanation
a) $V=V_1+V_2=\frac{k q_1}{r}+\frac{k q_2}{r}=\frac{\left(9 \times 10^9 \frac{\mathrm{Nm}^2}{C^2}\right)\left(-4 \times 10^{-6} \mathrm{C}\right)}{6 \mathrm{~m}}+\frac{\left(9 \times 1 \frac{9 \mathrm{Nm}^2}{C^2}\right)\left(1 \times 10^{-6} C\right.}{6 m}=$ $-4500 V$
b) $W=V q=(-4500 \mathrm{~V})\left(20 \times 10^{-9} \mathrm{C}\right)=-9 \times 10^{-5} \mathrm{~J}$
*the work in this case is negative. The potential at the point is negative so a positive charge would be attracted, but it takes negative work to bring it here, similar to the way negative work is done when an object is lowered in a gravitational field.
Equipotential lines.
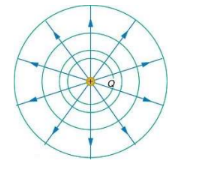
- Equipotential lines – Lines that indicate equal potential (every point on a given equipotential line has the same electric potential as every other point on the line.
*No work is required to move from point to point along an equipotential line. - Equipotential lines are drawn perpendicular to the electric field at any point.
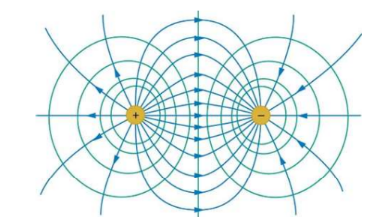
Example C: Draw electric field lines and equipotential lines for the following two oppositely charge point charges:Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
Answer/Explanation
The Electron Volt,
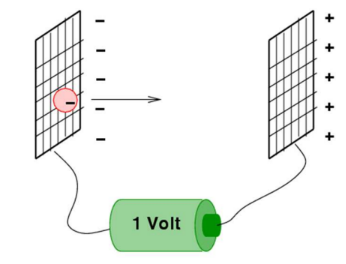
- An electron volt is a common unit of energy.
- The joule is a large unit for measuring energies of many charge particles. Thus, the electron volt is used.
- An electron volt is the energy acquired by an electron as a result of moving through a potential difference of $1 \mathrm{~V}$.
$
1 \mathrm{eV}=W=V q=(1 \mathrm{~V})\left(1.6 \times 10^{-19} \mathrm{C}\right)=1.6 \times 10^{-19} \mathrm{~J}
$
$1 \mathrm{eV}=1.6 \times 10^{-19} \mathrm{~J}$
Example D: What is the speed of an electron that has been accelerated through a potential difference of $4 \mathrm{~V}$ ? Calculate its final kinetic energy in $\mathrm{eV}$. (an electron has a mass of $9.11 \times 10^{-31} \mathrm{~kg}$ and a charge of $1.6 \times 10^{-19} \mathrm{C}$ ).
Answer/Explanation
$
\begin{aligned}
& W=\Delta K \rightarrow V q=\frac{1}{2} m v_f^2-\frac{1}{z} m v_t^{\frac{z}{z}} \rightarrow v_f=\sqrt{\frac{2 V q}{m}} \\
& \rightarrow v_f=\sqrt{\frac{2(4 V)\left(1.6 \times 10^{-1} \mathrm{C}\right)}{9.11}}=1.2 \times 10^6 \mathrm{~m} / \mathrm{s} \\
& K=\frac{1}{2} m v^2=\frac{1}{2}\left(9.11 \times 10^{-31} \mathrm{~kg}\right)\left(1.2 \times 10^6 \frac{\mathrm{m}}{\mathrm{s}}\right)^2=6.4 \times 10^{-1} \mathrm{~J} x \frac{1 \mathrm{eV}}{1.6 \times 10^{-19} \mathrm{~J}}=4 \mathrm{eV}, \text { as expected }
\end{aligned}
$
Electric potential due to a continuous charge distribution.
- Consider a continuous charge distribution to be made of up an infinite number of point charges with charge dq. Superposition can be used to add up all the dV from each contributor, dq.
$V=\int \frac{k d q}{r}$
*or* The electric potential is the opposite of the integral of field over distance.
$\begin{aligned} & W=\int F d x=\int q E d x \\ & V=-\frac{W}{q}=-\frac{\int q E d x}{q} \rightarrow V=-\int E d r\end{aligned}$

Example E: Find an integral for the potential at point A due to the uniform line of charge as shown The charge density is λ C/m.
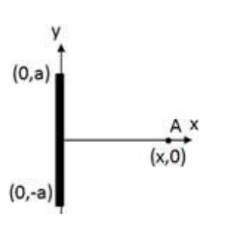
*x is coordinate of A and is constant. y is a variable that gives the location of a typical element of charge dq.
Answer/Explanation
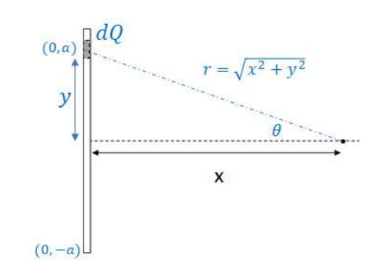
$d V=\frac{k d Q}{r}$
$\begin{aligned} & d Q=\lambda d y=\frac{Q}{2 a} d y \\ & V=\int_{-a}^a \frac{k \frac{Q}{2 a} d y}{\sqrt{x^2+y^2}}=\frac{k Q}{2 a} \int_{-a}^a \frac{d y}{\sqrt{x^2+y^2}}\end{aligned}$
$\begin{aligned} & V=\frac{k Q}{2 a} \ln \left|y+\sqrt{y^2+x^2}\right|_{-a}^a \rightarrow V=\frac{k Q}{2 a}\left(\ln \left|a+\sqrt{a^2+x^2}\right|-\ln \left|-a+\sqrt{a^2+x^2}\right|\right. \\ & \rightarrow \frac{k Q}{2 a} \ln \left|\frac{a+\sqrt{a^2+x^2}}{\sqrt{a^2+x^2}-a}\right|\end{aligned}$
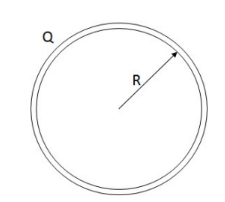
Example F: A conducting shell has a total charge of Q and radius R.
a) Find the electric potential outside the shell
b) Find the electric potential inside the shell.Lorem ipsum dolor sit amet, consectetur adipiscing elit. Ut elit tellus, luctus nec ullamcorper mattis, pulvinar dapibus leo.
In cases where Gauss’s Law can be readily used, the potential can be found by first founding the field using Gauss’s Law, and then finding potential by $V=-\int E \cdot d r$ from infinity to the point where potential is being calculated.
Answer/Explanation
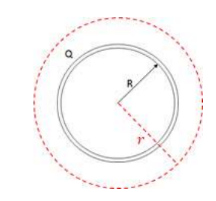
a) Step 1: Find the field –
$
\begin{aligned}
& \varnothing=\oint \vec{E} \cdot \overrightarrow{d A}=\frac{Q_{e n c}}{\varepsilon_0} \rightarrow E\left(4 \pi r^2\right)=\frac{Q}{\varepsilon_0} \\
& \rightarrow E=\frac{Q}{4 \pi r^2 \varepsilon_0}=\frac{k Q}{r^2}
\end{aligned}
$
Step 2: Find potential by integrating from infinity to $r$ :
$
\begin{aligned}
& V=-\int_{\infty}^r \frac{k Q}{r^2} d r=-\left.\left(-\frac{K Q}{r}\right)\right|_{\infty} ^r=\frac{k Q}{r}-0 \\
& \rightarrow \boldsymbol{V}=\frac{\boldsymbol{k} \boldsymbol{Q}}{\boldsymbol{r}}
\end{aligned}
$
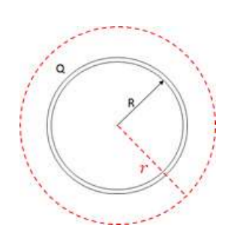
b) Step 1: Find the field with Gauss’s Law-
$
\begin{aligned}
& \emptyset=\oint_E \cdot \overrightarrow{d A}=\frac{Q_{e n c}}{\varepsilon_0} \rightarrow Q_{e n c}=0 \\
& \rightarrow E=0
\end{aligned}
$
Step 2: Find potential by integrating from infinity to $r$ :
Coming from infinity, there are two regions that need to be consider, infinity to the surface of the sphere, and then going in the surface of the sphere to r. The two regions have different fields to integrate:
$\begin{aligned} & V=-\int_{\infty}^R \frac{k Q}{r^2} d r-\int_R^r 0 d r \\ & \rightarrow V=-\left.\left(-\frac{K Q}{r}\right)\right|_{\infty} ^R=\frac{K Q}{R}\end{aligned}$
The potential inside the conducting shell is always $\frac{K Q}{R}$. The potential inside of a conductor is constant since the field inside a conductor is zero.
Example G: Find the electric potential inside a uniformly charged insulating sphere of radius R and total charge Q.
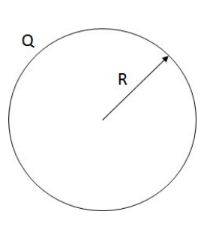
Answer/Explanation
Field Inside:
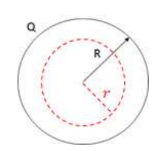
$\begin{aligned} & \emptyset=\oint \vec{E} \cdot \overrightarrow{d A}=\frac{Q_{\text {enc }}}{\varepsilon_0} \rightarrow E\left(4 \pi \digamma^Z\right)=\frac{\frac{Q 4}{4} \pi \frac{4}{3} \pi R^{33} r^3}{\varepsilon_0} \\ & \rightarrow E=\frac{Q r}{4 \pi \varepsilon_0 R^3}\end{aligned}$
Field Outside:
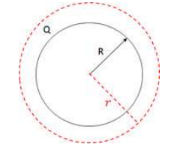
$\begin{aligned} & \emptyset=\oint \vec{E} \cdot \overrightarrow{d A}=\frac{Q_{\text {enc }}}{\varepsilon_0} \rightarrow E\left(4 \pi r^2\right)=\frac{Q}{\varepsilon_0} \\ & \rightarrow E=\frac{Q}{4 \pi r^2 \varepsilon_0}\end{aligned}$
Potential:
$
\begin{aligned}
& V=-\int_{\infty}^R \frac{k Q}{r^2} d r-\int_R^r \frac{Q r}{4 \pi \varepsilon_0 R^3} d r \\
& \rightarrow V=-\left.\left(-\frac{K Q}{r}\right)\right|_{\infty} ^R-\left(\frac{Q r^2}{8 \pi \varepsilon_0 R^3}\right) \mid \frac{r}{R}=\frac{K Q}{R}-\frac{Q}{8 \pi \varepsilon_0 R^3}\left(r^2-R^2\right) \\
& \rightarrow V=\frac{k Q}{R}+\frac{Q R^2-Q r^2}{8 \pi \varepsilon_0 R^3}
\end{aligned}
$
Rate your understanding: Electric Potential