A group of 12 people consists of 3 boys, 4 girls and 5 adults.
(a) Question
In how many ways can a team of 5 people be chosen from the group if exactly one adult is included?
Answer/Explanation
Ans:
5C1 × 7C4
175
(b) Question
In how many ways can a team of 5 people be chosen from the group if the team includes at least 2 boys and at least 1 girl?
The same group of 12 people stand in a line.
Answer/Explanation
Ans:
2B 1G 2A 3C2 × 4C1 × 5C2 = 120
2B 2G 1A 3C2 × 4C2 × 5C1 = 90
2B 3G 3C2 × 4C3 = 12
3B 1G 1A 3C3 × 4C1 × 5C1 = 20
3B 2G 3C3 × 4C2 = 6
[Total =] 248
(c) Question
How many different arrangements are there in which the 3 boys stand together and an adult is at each end of the line?
Answer/Explanation
Ans:
8! × 3! × 5P2
4 838 400
Question
The weights of apples of a certain variety are normally distributed with mean 82 grams. 22% of these apples have a weight greater than 87 grams.
(a) Find the standard deviation of the weights of these apples.
(b) Find the probability that the weight of a randomly chosen apple of this variety differs from the mean weight by less than 4 grams.
Answer/Explanation
Ans:
(a) \(P(X>87)=P(Z>\frac{87-82}{\sigma})=0.22\)
\(P(Z<\frac{5}{\sigma})=0.78\)
\((\frac{5}{\sigma}=)0.772\)
\(\sigma=6.48\)
(b) \(P(-\frac{4}{\sigma}<Z<\frac{4}{\sigma})=P(-0.6176<Z<0.6176)\)
\(\Phi=0.7317\)
Prob = \(2\Phi-1=2(0.7317)-1\)
= 0.463
Question
The score when two fair six-sided dice are thrown is the sum of the two numbers on the upper faces.
(a) Show that the probability that the score is 4 is \(\frac{1}{12}\) [1]
The two dice are thrown repeatedly until a score of 4 is obtained. The number of throws taken is
denoted by the random variable X.
(b) Find the mean of X. [1]
(c) Find the probability that a score of 4 is first obtained on the 6th throw. [1]
(d) Find P(X < 8). [2]
Answer/Explanation
Ans
1 (a) Prob of 4 (from 1,3, 3,1 or 2,2) \(=\frac{3}{36}=\frac{1}{12} \ AG\)
1 (b) \(Mean=\frac{1}{\frac{1}{12}}=12\)
1 (c) \(\left ( \frac{11}{12} \right )\times \frac{1}{12}=0.0539\ or \frac{161051}{2985984}\)
1 (d) \(1-\left ( \frac{11}{12} \right )^{7}\)
\(0.456\ or \ \frac{16344637}{35831808}\ )
Question
In a music competition, there are 8 pianists, 4 guitarists and 6 violinists. 7 of these musicians will be
selected to go through to the final.
How many different selections of 7 finalists can be made if there must be at least 2 pianists, at least
1 guitarist and more violinists than guitarists? [4]
Answer/Explanation
Ans
4 Scenarios:
2P 3V 2G 8C2 × 4C2× 6C3 = 28 × 6 × 20 = 3360
2P 4V 1G 8C2 × 4C1 × 6C4 = 28 × 4 × 15 = 1680
3P 3V 1G 8C3 × 4C1× 6C3 = 56 × 4 × 20 = 4480
4P 2V 1G 8C4 × 4C1 × 6C2 = 70 × 4 × 15 = 4200
(M1 for 8Cr × 4Cr × 6Cr with Σr = 7
Two unsimplified products correct
Summing the number of ways for 3 or 4 correct scenarios
Total: 13 720
Question
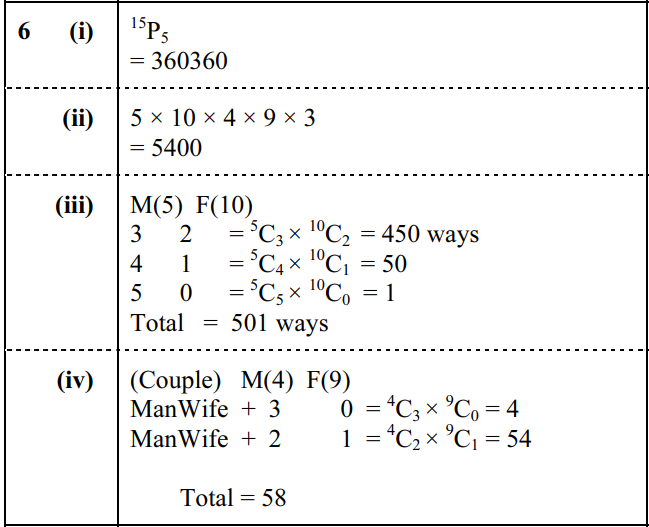
Hannah chooses 5 singers from 15 applicants to appear in a concert. She lists the 5 singers in the order in which they will perform.
(i) How many different lists can Hannah make? [2]
Of the 15 applicants, 10 are female and 5 are male.
(ii) Find the number of lists in which the first performer is male, the second is female, the third is male, the fourth is female and the fifth is male. [2]
Hannah’s friend Ami would like the group of 5 performers to include more males than females. The order in which they perform is no longer relevant.
(iii) Find the number of different selections of 5 performers with more males than females. [3]
(iv) Two of the applicants are Mr and Mrs Blake. Find the number of different selections that include Mr and Mrs Blake and also fulfil Ami’s requirement. [3]
Answer/Explanation
Ans: