Question: [Maximum mark: 4]
Consider the equation kx2 – (k + 3) x + 2k + 9 = 0, where k ∈ R.
(a) Write down an expression for the product of the roots, in terms of k .
(b) Hence or otherwise, determine the values of k such that the equation has one positive and one negative real root.
Answer/Explanation
Ans:
(a) product of roots \(=\frac{2k+9}{k}\)
(b) recognition that the product of the roots will be negative
\(=\frac{2k+9}{k}\) < 0
critical values \(k = 0, -\frac{9}{2}\)
\(-\frac{9}{2}<k<0\)
Question
The cubic equation x3 – kx2 + 3k = 0 where k > 0 has roots α , β and α + β .
Given that α.β =\(\frac{-k^2}{4}\) , find the value of k .[Maximum mark: 5]
Answer/Explanation
Ans:
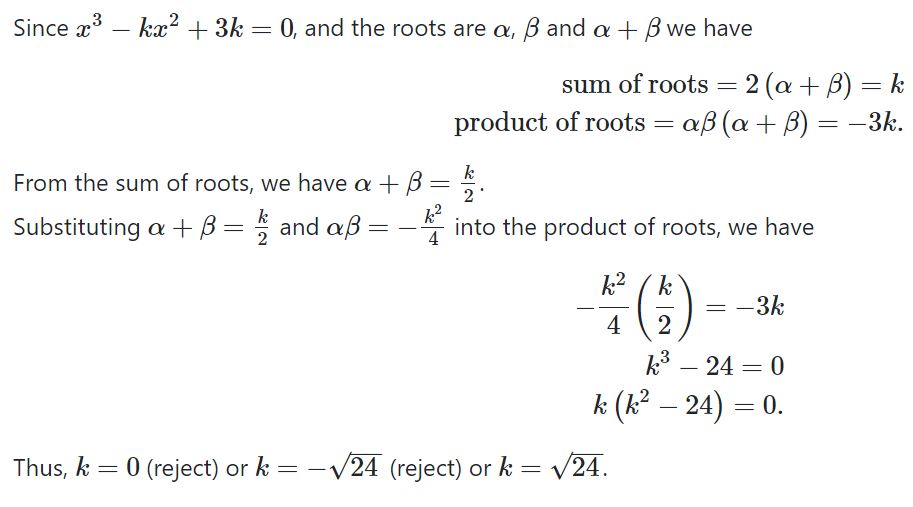
Question
The equation \(5{x^3} + 48{x^2} + 100x + 2 = a\) has roots \({r_1}\), \({r_2}\) and \({r_3}\).
Given that \({r_1} + {r_2} + {r_3} + {r_1}{r_2}{r_3} = 0\), find the value of a.
Answer/Explanation
Markscheme
\({r_1} + {r_2} + {r_3} = \frac{{ – 48}}{5}\) (M1)(A1)
\({r_1}{r_2}{r_3} = \frac{{a – 2}}{5}\) (M1)(A1)
\(\frac{{ – 48}}{5} + \frac{{a – 2}}{5} = 0\) M1
\(a = 50\) A1
Note: Award M1A0M1A0M1A1 if answer of 50 is found using \(\frac{{48}}{5}\) and \(\frac{{2 – a}}{5}\).
[6 marks]
Question
The roots of a quadratic equation \(2{x^2} + 4x – 1 = 0\) are \(\alpha \) and \(\beta \).
Without solving the equation,
(a) find the value of \({\alpha ^2} + {\beta ^2}\);
(b) find a quadratic equation with roots \({\alpha ^2}\) and \({\beta ^2}\).
Answer/Explanation
Markscheme
(a) using the formulae for the sum and product of roots:
\(\alpha + \beta = – 2\) A1
\(\alpha \beta = – \frac{1}{2}\) A1
\({\alpha ^2} + {\beta ^2} = {(\alpha + \beta )^2} – 2\alpha \beta \) M1
\( = {( – 2)^2} – 2\left( { – \frac{1}{2}} \right)\)
\( = 5\) A1
Note: Award M0 for attempt to solve quadratic equation.
[4 marks]
(b) \((x – {\alpha ^2})(x – {\beta ^2}) = {x^2} – ({\alpha ^2} + {\beta ^2})x + {\alpha ^2}{\beta ^2}\) M1
\({x^2} – 5x + {\left( { – \frac{1}{2}} \right)^2} = 0\) A1
\({x^2} – 5x + \frac{1}{4} = 0\)
Note: Final answer must be an equation. Accept alternative correct forms.
[2 marks]
Total [6 marks]
Question
The quadratic equation \(2{x^2} – 8x + 1 = 0\) has roots \(\alpha \) and \(\beta \).
Without solving the equation, find the value of
(i) \(\alpha + \beta \);
(ii) \(\alpha \beta \).[2]
Another quadratic equation \({x^2} + px + q = 0,{\text{ }}p,{\text{ }}q \in \mathbb{Z}\) has roots \(\frac{2}{\alpha }\) and \(\frac{2}{\beta }\).
Find the value of \(p\) and the value of \(q\).[4]
Answer/Explanation
Markscheme
using the formulae for the sum and product of roots:
(i) \(\alpha + \beta = 4\) A1
(ii) \(\alpha \beta = \frac{1}{2}\) A1
Note: Award A0A0 if the above results are obtained by solving the original equation (except for the purpose of checking).
[2 marks]
METHOD 1
required quadratic is of the form \({x^2} – \left( {\frac{2}{\alpha } + \frac{2}{\beta }} \right)x + \left( {\frac{2}{\alpha }} \right)\left( {\frac{2}{\beta }} \right)\) (M1)
\(q = \frac{4}{{\alpha \beta }}\)
\(q = 8\) A1
\(p = – \left( {\frac{2}{\alpha } + \frac{2}{\beta }} \right)\)
\( = – \frac{{2(\alpha + \beta )}}{{\alpha \beta }}\) M1
\( = – \frac{{2 \times 4}}{{\frac{1}{2}}}\)
\(p = – 16\) A1
Note: Accept the use of exact roots
METHOD 2
replacing \(x\) with \(\frac{2}{x}\) M1
\(2{\left( {\frac{2}{x}} \right)^2} – 8\left( {\frac{2}{x}} \right) + 1 = 0\)
\(\frac{8}{{{x^2}}} – \frac{{16}}{x} + 1 = 0\) (A1)
\({x^2} – 16x + 8 = 0\)
\(p = – 16\) and \(q = 8\) A1A1
Note: Award A1A0 for \({x^2} – 16x + 8 = 0\) ie, if \(p = – 16\) and \(q = 8\) are not explicitly stated.
[4 marks]
Total [6 marks]
Question
The quadratic equation \(2{x^2} – 8x + 1 = 0\) has roots \(\alpha \) and \(\beta \).
Without solving the equation, find the value of
(i) \(\alpha + \beta \);
(ii) \(\alpha \beta \).[2]
Another quadratic equation \({x^2} + px + q = 0,{\text{ }}p,{\text{ }}q \in \mathbb{Z}\) has roots \(\frac{2}{\alpha }\) and \(\frac{2}{\beta }\).
Find the value of \(p\) and the value of \(q\).[4]
Answer/Explanation
Markscheme
using the formulae for the sum and product of roots:
(i) \(\alpha + \beta = 4\) A1
(ii) \(\alpha \beta = \frac{1}{2}\) A1
Note: Award A0A0 if the above results are obtained by solving the original equation (except for the purpose of checking).
[2 marks]
METHOD 1
required quadratic is of the form \({x^2} – \left( {\frac{2}{\alpha } + \frac{2}{\beta }} \right)x + \left( {\frac{2}{\alpha }} \right)\left( {\frac{2}{\beta }} \right)\) (M1)
\(q = \frac{4}{{\alpha \beta }}\)
\(q = 8\) A1
\(p = – \left( {\frac{2}{\alpha } + \frac{2}{\beta }} \right)\)
\( = – \frac{{2(\alpha + \beta )}}{{\alpha \beta }}\) M1
\( = – \frac{{2 \times 4}}{{\frac{1}{2}}}\)
\(p = – 16\) A1
Note: Accept the use of exact roots
METHOD 2
replacing \(x\) with \(\frac{2}{x}\) M1
\(2{\left( {\frac{2}{x}} \right)^2} – 8\left( {\frac{2}{x}} \right) + 1 = 0\)
\(\frac{8}{{{x^2}}} – \frac{{16}}{x} + 1 = 0\) (A1)
\({x^2} – 16x + 8 = 0\)
\(p = – 16\) and \(q = 8\) A1A1
Note: Award A1A0 for \({x^2} – 16x + 8 = 0\) ie, if \(p = – 16\) and \(q = 8\) are not explicitly stated.
[4 marks]
Total [6 marks]