Question
A toy rocket is made from a plastic bottle that contains some water.
Air is pumped into the vertical bottle until the pressure inside forces water and air out of the bottle. The bottle then travels vertically upwards.
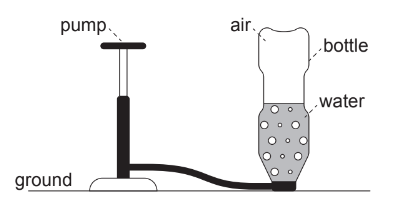
The air–water mixture is called the propellant.
The variation with time of the vertical velocity of the bottle is shown.
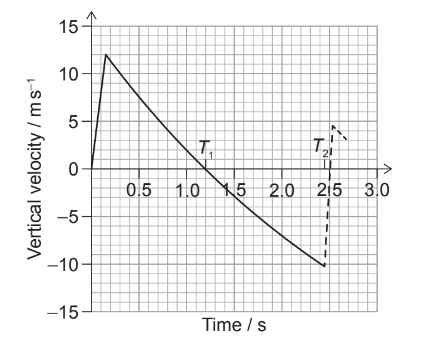
The bottle reaches its highest point at time \(T_1\) on the graph and returns to the ground at time \(T_2\). The bottle then bounces. The motion of the bottle after the bounce is shown as a dashed line.
(a) Estimate, using the graph, the maximum height of the bottle.[3]
(b) Estimate the acceleration of the bottle when it is at its maximum height. [2]
(c) The bottle bounces when it returns to the ground.
(i) Calculate the fraction of the kinetic energy of the bottle that remains after the bounce.
(ii) The mass of the bottle is 27g and it is in contact with the ground for 85ms. Determine the average force exerted by the ground on the bottle. Give your answer to an appropriate number of significant figures. [3]
(d) The maximum height reached by the bottle is greater with an air–water mixture than with only high-pressure air in the bottle.
Assume that the speed at which the propellant leaves the bottle is the same in both cases Explain why the bottle reaches a greater maximum height with an air–water mixture. [2]
▶️Answer/Explanation
Ans:
a. ALTERNATIVE 1
Attempt to count squares \(\checkmark\)
Area of one square found \(\checkmark\)
7.2 «m» (accept \(6.4-7.4 \mathrm{~m}\) )
ALTERNATIVE 2
Uses area equation for either triangle \(\checkmark\)
Correct read offs for estimate of area of triangle
\(7.2 \approx \mathrm{m} »\) (accept \(6.4-7.4\) )
b Attempt to calculate gradient of line at \(t=1.2 \mathrm{~s} \checkmark\)
$
\left.\alpha \rightarrow 9.8 \ll \mathrm{m} \mathrm{s}^{-2} \rightsquigarrow \text { (accept } 9.6-10.0\right) \checkmark
$
c i Attempt to evaluate KE ratio as \(\left(\frac{v_{\text {final }}}{v_{\text {initial }}}\right)^2 \checkmark\)
$
\left(\frac{4.5}{10}\right)^2 \Rightarrow 0.20 \text { OR } 20 \% \text { OR } \frac{1}{5}
$
ii Attempt to use force \(=\) momentum change \(\div\) time \(\checkmark\)
$
\begin{aligned}
& \alpha=\frac{(4.5+10) \times 0.027}{85 \times 10^{-3}}=4.6 » \\
& \text { Force }=\ll 4.6+0.3 » 4.9 \ll \mathrm{N} »
\end{aligned}
$
Any answer to 2 sf \(\checkmark\)
Mass «leaving the bottle per second» will be larger for air-water \(\checkmark\) the momentum change/force is greater \(\checkmark\)
Question
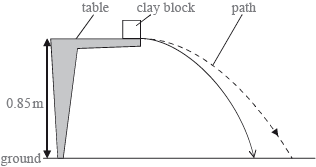
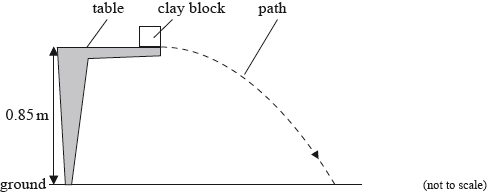
The experiment is repeated with the clay block placed at the edge of the table so that it is fired away from the table. The initial speed of the clay block is \({\text{4.3 m}}\,{{\text{s}}^{ – 1}}\) horizontally. The table surface is 0.85 m above the ground.
The graph shows the variation with distance \(r\) from the centre of Mars of the gravitational potential \(V\). \(R\) is the radius of Mars which is 3.3 Mm. (Values of \(V\) for \(r < R\) are not shown.)
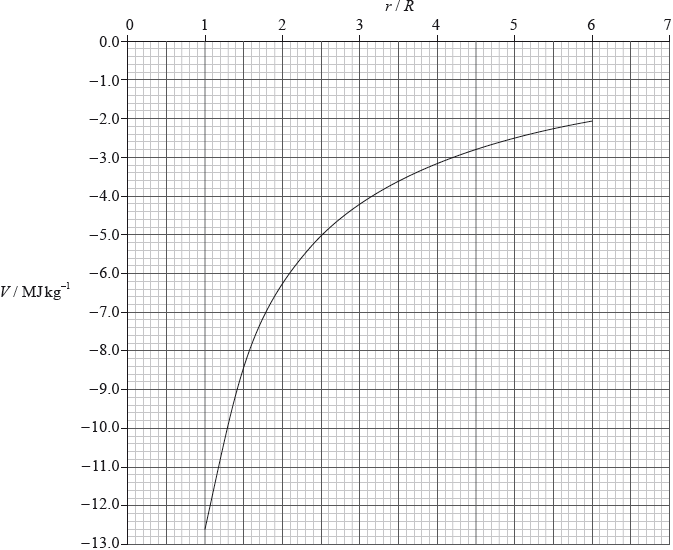
A rocket of mass \(1.2 \times {10^4}{\text{ kg}}\) lifts off from the surface of Mars. Use the graph to
(i) Ignoring air resistance, calculate the horizontal distance travelled by the clay block before it strikes the ground.
(ii) The diagram above shows the path of the clay block neglecting air resistance. On the diagram, draw the approximate shape of the path that the clay block will take assuming that air resistance acts on the clay block.[7]
Answer/Explanation
Ans
(i) use of kinematic equation to yield time;
\(t = \sqrt {\frac{{2s}}{g}} {\text{ (}} = 0.42{\text{ s)}}\);
\(s = {\text{horizontal speed\( \times \)time}}\);
\( = 1.8{\text{ m}}\);
Accept g = 10 m\(\,\)s\(^{ – 2}\) equivalent answers 1.79 from 9.8, 1.77 from 10.
(ii) initial drawn velocity horizontal; (judge by eye)
reasonable shape; (i.e. quasi-parabolic)
horizontal distance moved always decreasing when compared to given path / range less than original;