Question
The probability that it rains on Monday \(\frac{3}{5}\)
If it rains on Monday, the probability that it rains on Tuesday is \(\frac{4}{7}\)
If it does not rain on Monday, the probability that it rains on Tuesday is \(\frac{5}{7}\)
(a) Complete the tree diagram.

(b) Find the probability that it rains
(i) on both days,
(ii) on Monday but not on Tuesday,
(iii) on only one of the two days.
(c) If it does not rain on Monday and it does not rain on Tuesday, the probability that it does not
rain on Wednesday is \(\frac{1}{4}\)
Calculate the probability that it rains on at least one of the three days.
Answer/Explanation
(a)

(b)(i) The probability that it rains on both days is:
Product of probabilities when it rained on monday and tuesday:
\(\frac{3}{5}\).\(\frac{4}{7}\)
= \(\frac{12}{35}\)
(ii) The probability that it rains on Monday but not on tuesday:
Product of probabilities when it rained on monday but not on tuesday:
\(\frac{3}{5}\).\(\frac{3}{7}\)
= \(\frac{9}{35}\)
(iii) The probabilities that it rains on only one of the two days:
Addition of the probability of( no rain on Monday and rain on Tuesday), and the( probability of rain on Monday and no rain on Tuesday):
P( No rain on Monday) .P( Rain on Tuesday)+P(Rain on Monday) .P(No rain on Tuesday)
\(\frac{2}{5}\).\(\frac{5}{7}\)+\(\frac{3}{5}\).\(\frac{3}{7}\)
=\(\frac{19}{35}\)
(c) Probability that it rains on at least one of the three days=P(rain on monday).P(no rain on tuesday).P(no rain on wednesday)+P( no rain on monday).P(rain on tuesday).P(no rain on wednesday)+P(no rain on monday).P(no rain on tuesday).P(rain on wednesday)
=\(\frac{34}{35}\)
Question
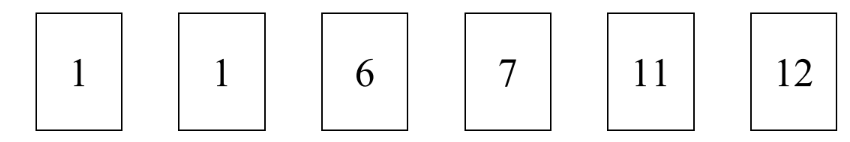
Six cards are numbered $1,1,6,7,11$ and 12 .
In this question, give all probabilities as fractions.
(a) One of the six cards is chosen at random.
(i) Which number has a probability of being chosen of $\frac{1}{3}$ ?
(ii) What is the probability of choosing a card with a number which is
smaller than at least three of the other numbers?
(b) Two of the six cards are chosen at random, without replacement.
Find the probability that
(i) they are both numbered 1,
(ii) the total of the two numbers is 18,
(iii) the first number is not a 1 and the second number is a 1.
(c) Cards are chosen, without replacement, until a card numbered 1 is chosen.
Find the probability that this happens before the third card is chosen.
(d) A seventh card is added to the six cards shown in the diagram. The mean value of the seven numbers on the cards is 6. Find the number on the seventh card.
▶️Answer/Explanation
(a) (i) 1
(ii) $3 / 6$ oe
(b) (i) $\frac{2}{30}$ oe www2
(ii) $6-12$ and $12-6$ and 7-11 and $11-7$ soi
$k \times \frac{1}{6} \times \frac{1}{5}$ for $k=$ integer
$\frac{4}{30}$ oe www 3
(iii) $\begin{aligned} & \frac{4}{6} \times \frac{2}{5} \\ & \frac{8}{30} \text { oe } \quad \text { Www } 2\end{aligned}$
(c) $
\frac{2}{6}+\frac{4}{6} \times \frac{2}{5} \quad \text { oe }
$
$\frac{18}{30}$ oe cao wWw2
(d) 4