Questions
\(Ag^{+}(aq)+NH_{3}(aq)\leftrightharpoons Ag(NH_{3})^{+}(aq)\) \(K_{eq(1)}=2.0\times 10^{3}\)
\(Ag(NH_{3})^{+}(aq)+NH_{3}(aq)\leftrightharpoons Ag(NH_{3})_{2}^{+}(aq) \) \(K_{eq(1)}=8.0\times 10^{3}\)
Equal volumes of 0.1 M \(AgNO_{3}(aq) \) and 2.0 M \(NH_{3}(aq)\) are mixed and the reactions represented above occur. Which Ag species will have the highest concentration in the equilibrium system shown below, and why?
\(Ag^{+}(aq)+2NH_{3}(aq)\leftrightharpoons Ag(NH_{3})_{2}^{+}(aq)\) \(K_{eq(3)}=?\)
(A) \(Ag^{+}(aq)\), because \(K_{eq(3)}=4\)
(B) \(Ag^{+}(aq)\), because \(K_{eq(1)}\) < \(K_{eq(2)}\)
(C) \(Ag(NH_{3})_{2}^{+}(aq)\), because \(K_{eq(3)}=1.6\times 10^{7}\)
(D) \(Ag(NH_{3})_{2}^{+}(aq)\), because \(K_{eq(1)}\) < \(K_{eq(2)}\)
▶️Answer/Explanation
Ans: C
To determine which \( \text{Ag} \) species will have the highest concentration in the equilibrium system, we need to find the equilibrium constant (\( K_{\text{eq}(3)} \)) for the overall reaction:
\[ \text{Ag}^+(aq) + 2\text{NH}_3(aq) \rightleftharpoons \text{Ag(NH}_3)_2^+(aq) \]
We can obtain \( K_{\text{eq}(3)} \) by multiplying the equilibrium constants of the two given steps:
Step 1: \( \text{Ag}^+(aq) + \text{NH}_3(aq) \rightleftharpoons \text{Ag(NH}_3)^+(aq) \) \( K_{\text{eq}(1)} = 2.0 \times 10^3 \)
Step 2: \( \text{Ag(NH}_3)^+(aq) + \text{NH}_3(aq) \rightleftharpoons \text{Ag(NH}_3)_2^+(aq) \) \( K_{\text{eq}(2)} = 8.0 \times 10^3 \)
\[ K_{\text{eq}(3)} = K_{\text{eq}(1)} \times K_{\text{eq}(2)} \]
\[ K_{\text{eq}(3)} = (2.0 \times 10^3) \times (8.0 \times 10^3) \]
\[ K_{\text{eq}(3)} = 1.6 \times 10^7 \]
The larger the equilibrium constant, the more the equilibrium favors the products. Since \( K_{\text{eq}(3)} = 1.6 \times 10^7 \) is a large value, the equilibrium will favor the formation of \( \text{Ag(NH}_3)_2^+(aq) \).
Therefore, the correct answer is (C) \( \text{Ag(NH}_3)_2^+(aq) \), because \( K_{\text{eq}(3)} = 1.6 \times 10^7 \).
Question
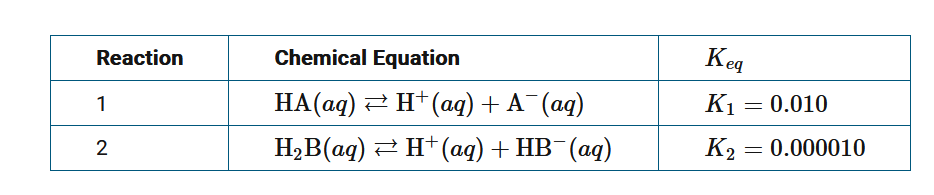
The table above provides the \(K_{eq}\) values for two reactions. Which of the following is the correct mathematical expression needed to determine the equilibrium constant of the reaction shown below?
Reaction 3: \(HA(aq)+HB^−(aq)\)⇄\(H_2B(aq)+A^−(aq)\)
\(K_{eq}=K_3\)
A \(K_3=K_1\times \frac{1}{K_2}\)
B \(K_3=\frac{1}{K_1}×K_2\)
C \(K_3=K_1−K_2\)
D \(K_3=−K_1+K_2\)
▶️Answer/Explanation
Ans:A
Reaction 2 needs to be reversed to obtain reaction 3. When a reaction is reversed, the equilibrium constant for the reverse reaction is the reciprocal of the equilibrium constant of the original reaction. When reactions are added together, the K of the resulting overall reaction is the product of the K’s for the reactions that were summed. Therefore, \(K_3=K1×\frac{1}{K_2}\).
Question
Reaction 1: \(PbCl_2(s)\)⇄\(Pb_2^+(aq)+2Cl^−(aq)\) \(K_{sp}=K_1\)
Reaction 2: \(AgCl(s)\)⇄\(Ag^+(aq)+Cl^−(aq)\) \(K_{sp}=K_2\)
Based on the information given above, which of the following is the expression for \(K_{eq}\) for the reaction that occurs when a 0.1M \(AgNO_3(aq)\) is added to a saturated solution of \(PbCl_2(aq)\) , as represented by the following chemical equation?
\(PbCl_2(s)+2Ag^+(aq)⇄Pb^{2+}(aq)+2AgCl(s)\)
A \(K_{eq}=K_1+(2×K_2)\)
B \(K_{eq}=K_1−(2×K_2)\)
C \(K_{eq}=K_1×(K_2)^2\)
D \(K_{eq}=\frac{K_1}{(K_2)^2}\)
▶️Answer/Explanation
Answer D
To obtain the net equation, the equation 2 must be reversed and doubled; thus, \(K_{eq}\) is determined by multiplying \(K_1\) by the reciprocal of \(K_2\) squared.
Question
Equation 1: \(2NO_2(g)\)⇄\(N_2O_4(g)\) \(K_1\)
Equation 2: \(N_2O_4(g)\)⇄\(2NO_2(g)\) \(K_2\)
In a large reaction vessel at a constant temperature, nitrogen dioxide and dinitrogen tetroxide are in a state of dynamic equilibrium, as represented by the chemical equations shown above. The equilibrium constants for the reactions are \(K_1\) and \(K_2\). Which of the following quantities can most easily be used to find the value of \(K_2\)?
A The value of ΔH for the reaction
B The temperature of the system
C The volume of the system
D The value of \(K_1\)
▶️Answer/Explanation
Ans:D
If the value of \(K_1\) is known, then the relationship \(K_2=\frac{1}{K_1}\) can be used to determine the value of \(K_2\)
Question
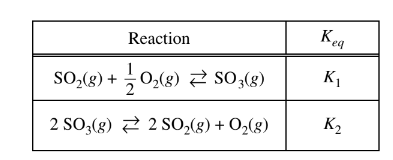
Which of the following shows the relationship between \(K_1\) and\( K_2\) in the reactions represented above?
(A) \(K_{2}\)= \((K_{1})^{2}\)
(B)\( K_{2}\)=\(\sqrt{K_{1}}\)
(C) \( K_{2}\)=\(\frac{1}{(K_{1})^{2}}\)
(D) \(K_{2}\)=\(\frac{1}{K_{1}}\)
▶️Answer/Explanation
Ans:C