IB DP PHYSICS 2025 SL&HL – Study notes- All Topics
Topic 4.1 Kinematics of rotational motion
Topic 1 Weightage : XX
Notes for 4.1 Kinematics of rotational motion – Only for Higher Level (HL)
torque τ of a force about an axis as given by τ = Fr sin θ, rotational equilibrium, unbalanced torque, angular acceleration, angular displacement, angular velocity, equations of motion for uniform angular acceleration, moment of inertia, Newton’s second law for rotation, angular momentum, resultant torque, kinetic energy of rotational motion
- IB DP Physics 2025 SL- IB Style Practice Questions with Answer-Topic Wise-Paper 1
- IB DP Physics 2025 HL- IB Style Practice Questions with Answer-Topic Wise-Paper 1
- IB DP Physics 2025 SL- IB Style Practice Questions with Answer-Topic Wise-Paper 2
- IB DP Physics 2025 HL- IB Style Practice Questions with Answer-Topic Wise-Paper 2
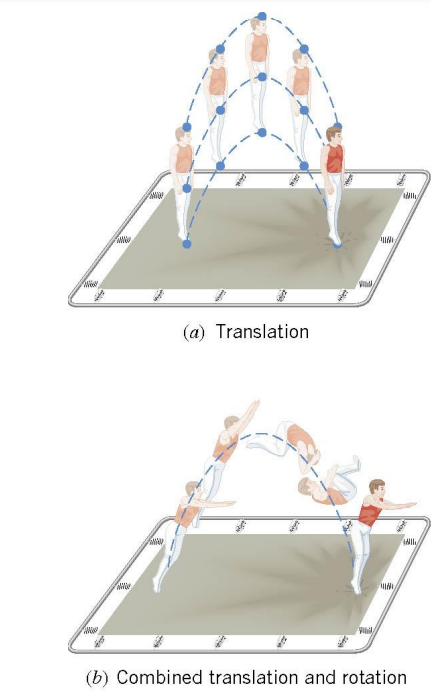
In pure translational motion, all points on an object travel on parallel paths.
The most general motion is a combination of translation and rotation.
According to Newton’s second law, a net force causes an object to have an acceleration.
What causes an object to have an angular acceleration?
TORQUE
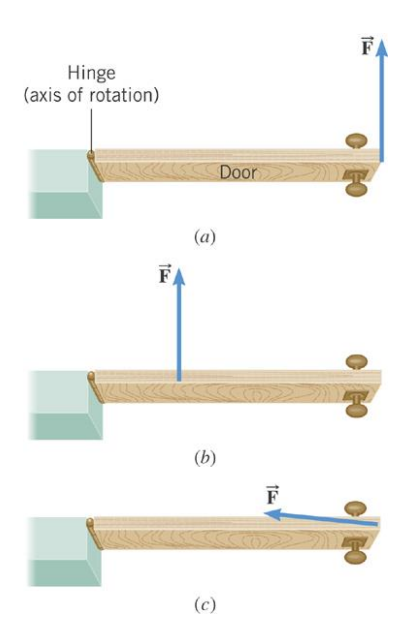
The amount of torque depends on where and in what direction the force is applied, as well as the location of the axis of rotation.
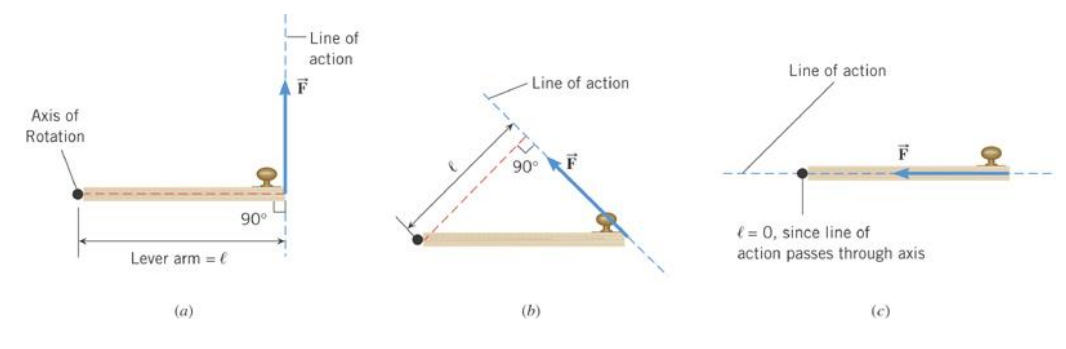
DEFINITION OF TORQUE
Magnitude of Torque \(=(\) Magnitude of the force \() \times(\) Lever arm \()\)
$
\tau=F \ell
$
Direction: The torque is positive when the force tends to produce a counterclockwise rotation about the axis.
SI Unit of Torque: newton \(\mathrm{x}\) meter \((\mathrm{N} \cdot \mathrm{m})\)
Example 2 The Achilles Tendon
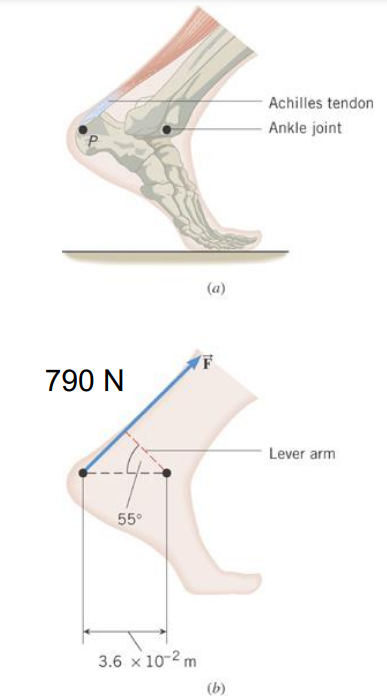
The tendon exerts a force of magnitude \(790 \mathrm{~N}\). Determine the torque (magnitude and direction) of this force about the ankle joint.
▶️Answer/Explanation
Ans:

\(\begin{aligned} & \tau=F \ell \\ & \cos 55^{\circ}=\frac{\ell}{3.6 \times 10^{-2} \mathrm{~m}} \\ & \tau=(720 \mathrm{~N})\left(3.6 \times 10^{-2} \mathrm{~m}\right) \cos 55^{\circ} \\ & =15 \mathrm{~N} \cdot \mathrm{m}\end{aligned}\)
9.2 Rigid Objects in Equilibrium
If a rigid body is in equilibrium, neither its linear motion nor its rotational motion changes.
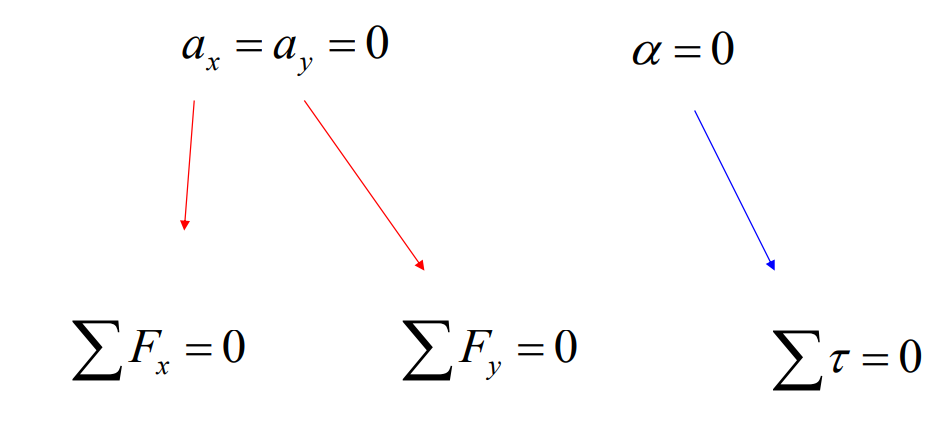
EQUILIBRIUM OF A RIGID BODY
A rigid body is in equilibrium if it has zero translational acceleration and zero angular acceleration. In equilibrium, the sum of the externally applied forces is zero, and the sum of the externally applied torques is zero.
$
\sum F_x=0 \quad \sum F_y=0 \quad \sum \tau=0
$
Reasoning Strategy
1. Select the object to which the equations for equilibrium are to be applied.
2. Draw a free-body diagram that shows all of the external forces acting on the object.
3. Choose a convenient set of \(x, y\) axes and resolve all forces into components that lie along these axes.
4. Apply the equations that specify the balance of forces at equilibrium. (Set the net force in the \(x\) and \(y\) directions equal to zero.)
5. Select a convenient axis of rotation. Set the sum of the torques about this axis equal to zero.
6. Solve the equations for the desired unknown quantities.
Example 3 A Diving Board
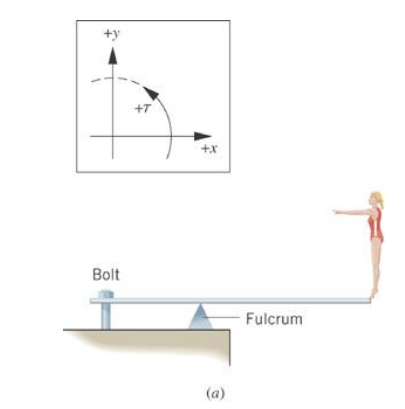
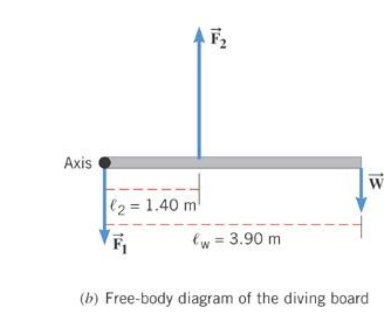
A woman whose weight is \(530 \mathrm{~N}\) is poised at the right end of a diving board with length \(3.90 \mathrm{~m}\). The board has negligible weight and is supported by a fulcrum \(1.40 \mathrm{~m}\) away from the left end.
Find the forces that the bolt and the fulcrum exert on the board.


\(\begin{gathered}\sum \tau=F_2 \ell_2-W \ell_W=0 \\ F_2=\frac{W \ell_W}{\ell_2} \\ F_2=\frac{(530 \mathrm{~N})(3.90 \mathrm{~m})}{1.40 \mathrm{~m}}=1480 \mathrm{~N}\end{gathered}\)


\(\begin{gathered}\sum F_y=-F_1+F_2-W=0 \\ -F_1+1480 \mathrm{~N}-530 \mathrm{~N}=0 \\ F_1=950 \mathrm{~N}\end{gathered}\)
Example 5 Bodybuilding

The arm is horizontal and weighs \(31.0 \mathrm{~N}\). The deltoid muscle can supply \(1840 \mathrm{~N}\) of force. What is the weight of the heaviest dumbell he can hold?

\(\sum \tau=-W_a \ell_a-W_d \ell_d+M \ell_M=0\)
\(\ell_M=(0.150 \mathrm{~m}) \sin 13.0^{\circ}\)

\(\begin{aligned} & W_d=\frac{-W_a \ell_a+M \ell_M}{\ell_d} \\ & =\frac{-(31.0 \mathrm{~N})(0.280 \mathrm{~m})+(1840 \mathrm{~N})(0.150 \mathrm{~m}) \sin 13.0^{\circ}}{0.620 \mathrm{~m}}=86.1 \mathrm{~N}\end{aligned}\)
9.3 Center of Gravity
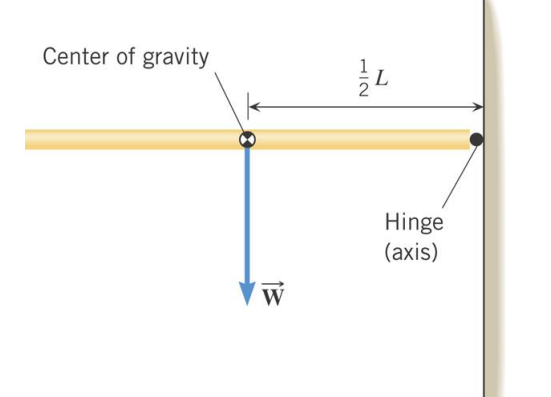
DEFINITION OF CENTER OF GRAVITY
The center of gravity of a rigid body is the point at which its weight can be considered to act when the torque due to the weight is being calculated.
When an object has a symmetrical shape and its weight is distributed uniformly, the center of gravity lies at its geometrical center.
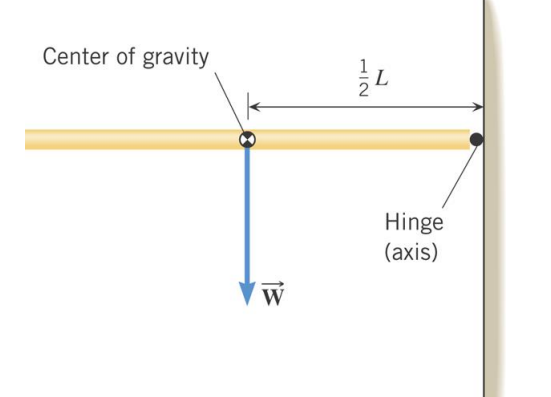
\(x_{c g}=\frac{W_1 x_1+W_2 x_2+\cdots}{W_1+W_2+\cdots}\)
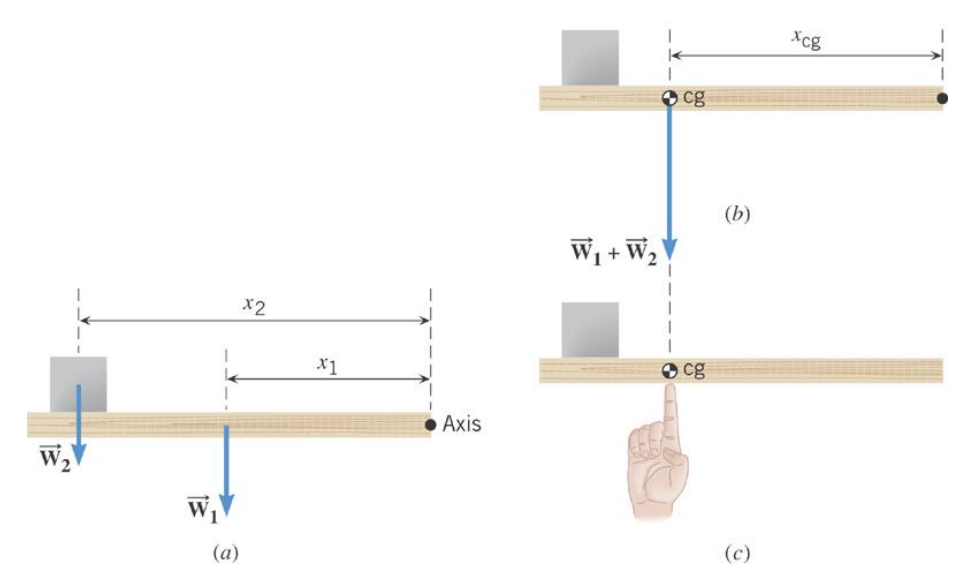
Example 6 The Center of Gravity of an Arm
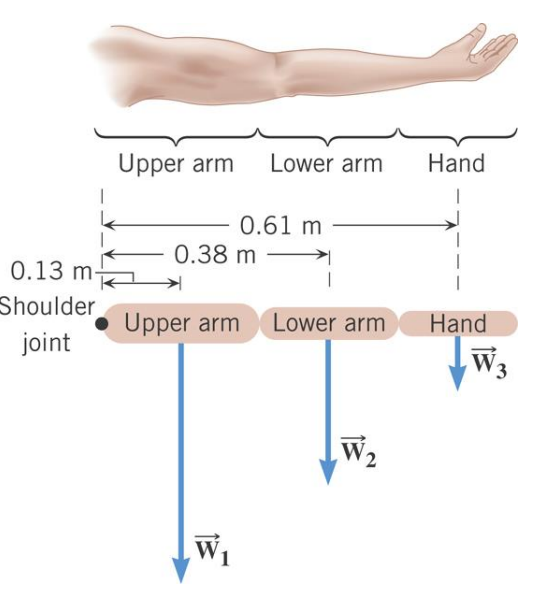
The horizontal arm is composed of three parts: the upper arm (17 N), the lower arm (11 \(\mathrm{N}\) ), and the hand (4.2 N).
Find the center of gravity of the arm relative to the shoulder joint.
▶️Answer/Explanation
Ans:

\(x_{c g}=\frac{W_1 x_1+W_2 x_2+\cdots}{W_1+W_2+\cdots}\)
\(x_{c g}=\frac{(17 \mathrm{~N})(0.13 \mathrm{~m})+(11 \mathrm{~N})(0.38 \mathrm{~m})+(4.2 \mathrm{~N})(0.61 \mathrm{~m})}{17 \mathrm{~N}+11 \mathrm{~N}+4.2 \mathrm{~N}}=0.28 \mathrm{n}\)
Conceptual Example 7 Overloading a Cargo Plane
This accident occurred because the plane was overloaded toward the rear. How did a shift in the center of gravity of the plane cause the accident?
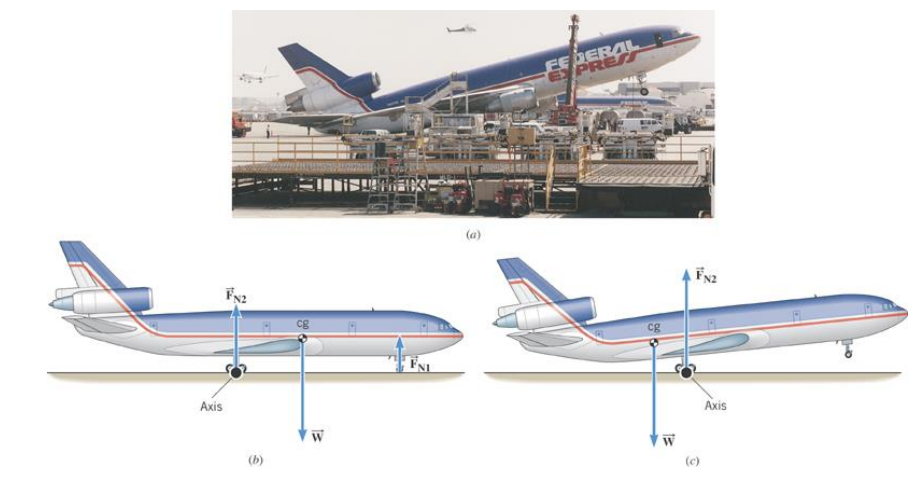
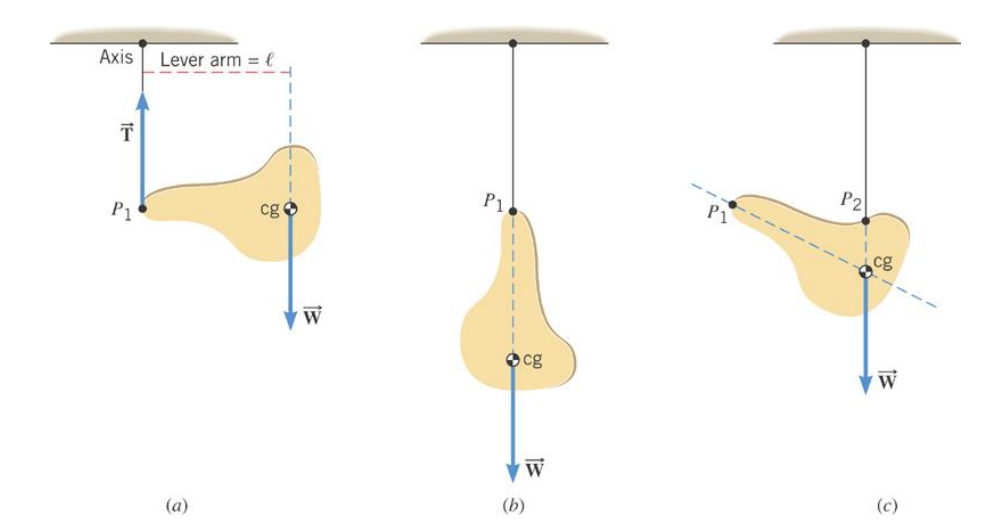
Finding the center of gravity of an irregular shape.
9.4 Newton’s Second Law for Rotational Motion About a Fixed Axis

\(\tau=\left(m r^2\right) \alpha\)
\(\left(m r^2\right)\) = Moment of Inertia, I
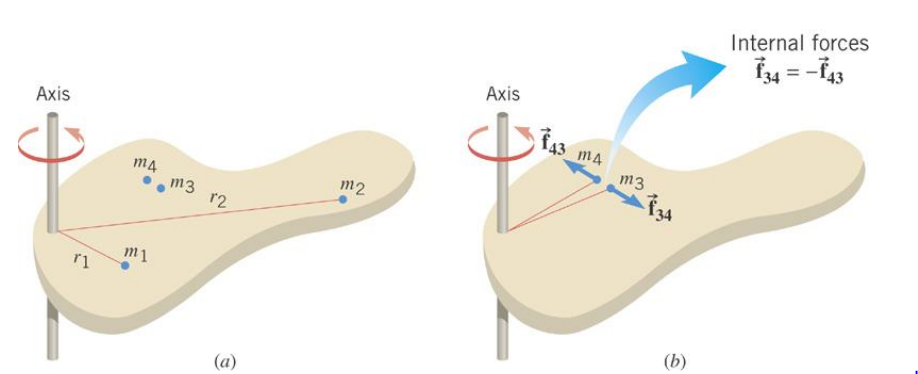
\(\begin{aligned} \tau_1 & =\left(m_1 r_1^2\right) \alpha \\ \tau_2 & =\left(m_2 r_2^2\right) \alpha\end{aligned}\)
\(\tau_N=\left(m_N r_N^2\right) \alpha\)
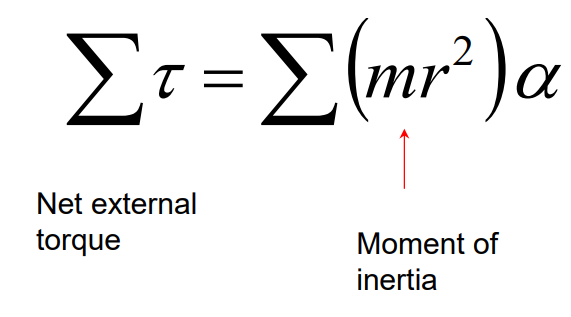
ROTATIONAL ANALOG OF NEWTON’S SECOND LAW FOR A RIGID BODY ROTATING ABOUT A FIXED AXIS
$
\text { Net external torque }=\binom{\text { Moment of }}{\text { inertia }} \times\binom{\text { Angular }}{\text { acceleration }}
$
\(\sum \tau=I \alpha\)
\(I=\sum\left(m r^2\right)\)
Requirement: Angular acceleration must be expressed in radians \(/ \mathrm{s}^2\).
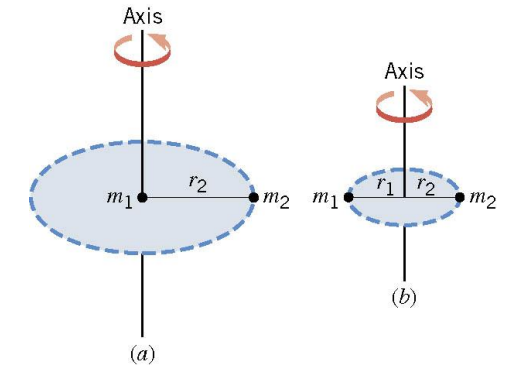
Example 9 The Moment of Inertia Depends on Where the Axis Is.
Two particles each have mass \(m\) and are fixed at the ends of a thin rigid rod. The length of the rod is \(L\). Find the moment of inertia when this object rotates relative to an axis that is perpendicular to the rod at
(a) one end and (b) the center.
▶️Answer/Explanation
Ans:

(a) \(\quad I=\sum\left(m r^2\right)=m_1 r_1^2+m_2 r_2^2=m(0)^2+m(L)^2\)
\(m_1=m_2=m \quad r_1=0 \quad r_2=L\)
\(I=m L^2\)
(b) \(I=\sum\left(m r^2\right)=m_1 r_1^2+m_2 r_2^2=m(L / 2)^2+m(L / 2)^2\)
\(m_1=m_2=m \quad r_1=L / 2 \quad r_2=L / 2\)
\(I=\frac{1}{2} m L^2\)
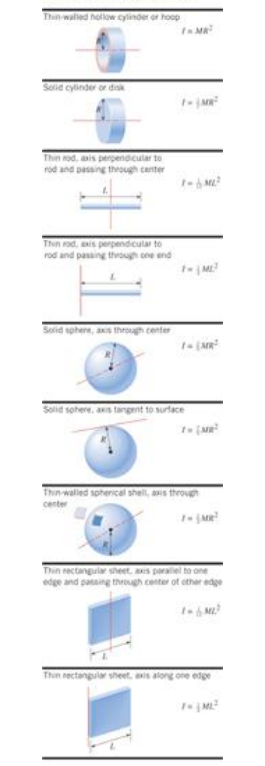
Table 9.1 Moments of inertia / for Various figid Objects of Mass M
Example 12 Hoisting a Crate
The combined moment of inertia of the dual pulley is \(50.0 \mathrm{~kg} \cdot \mathrm{m}^2\). The crate weighs \(4420 \mathrm{~N}\). A tension of \(2150 \mathrm{~N}\) is maintained in the cable attached to the motor. Find the angular acceleration of the dual pulley.

equal in magnitude
\(\sum F_y=T_2^{\prime}-m g=m a_y \quad \sum \tau=T_1 \ell_1-T_2 \ell_2=I \alpha\)
\(T_2=m g+m a_y\)
\(a_y=\ell_2 \alpha\)
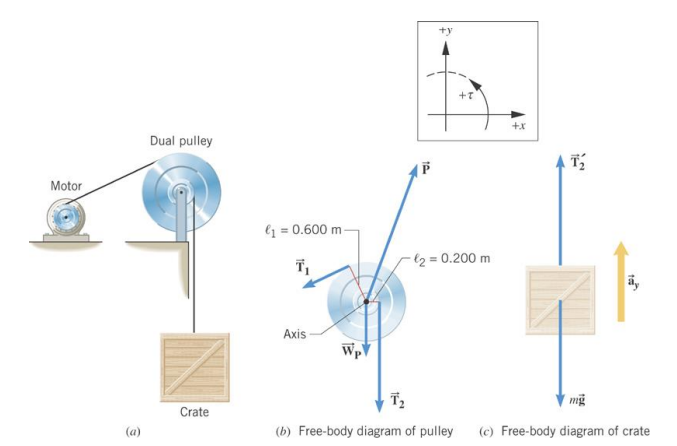
\(T_1 \ell_1-\left(m g+m a_y\right) \ell_2=I \alpha\)
\(a_y=\ell_2 \alpha\)
\(T_1 \ell_1-\left(m g+m \ell_2 \alpha\right) \ell_2=I \alpha\)
\(\begin{aligned} & \alpha=\frac{T_1 \ell_1-m g \ell_2}{I+m \ell_2^2} \\ & =\frac{(2150 \mathrm{~N})(0.600 \mathrm{~m})-(451 \mathrm{~kg})\left(9.80 \mathrm{~m} / \mathrm{s}^2\right)(0.200 \mathrm{~m})}{46.0 \mathrm{~kg} \cdot \mathrm{m}^2+(451 \mathrm{~kg})(0.200 \mathrm{~m})^2}=6.3 \mathrm{rad} / \mathrm{s}^2\end{aligned}\)
9.5 Rotational Work and Energy
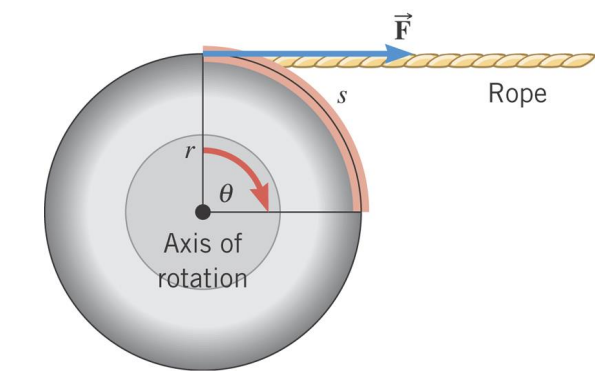
\(s=r \theta\)
\(W=F s=F r \theta\)
\(\tau=F r\)
\(W=\tau \theta\)
DEFINITION OF ROTATIONAL WORK
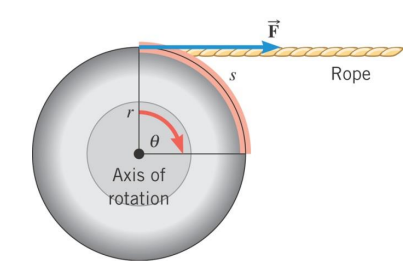
The rotational work done by a constant torque in turning an object through an angle is
$
W_R=\tau \theta
$
Requirement: The angle must be expressed in radians.
SI Unit of Rotational Work: joule (J)
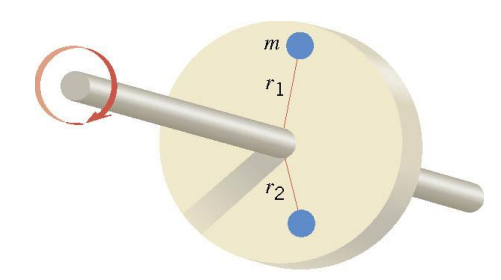
\(K E=\frac{1}{2} m v_T^2=\frac{1}{2} m r^2 \omega^2\)
\(v_T=r \omega\)
\(K E=\sum\left(\frac{1}{2} m r^2 \omega^2\right)=\frac{1}{2}\left(\sum m r^2\right) \omega^2=\frac{1}{2} I \omega^2\)
DEFINITION OF ROTATIONAL KINETIC ENERGY
The rotational kinetic energy of a rigid rotating object is
$
K E_R=\frac{1}{2} I \omega^2
$
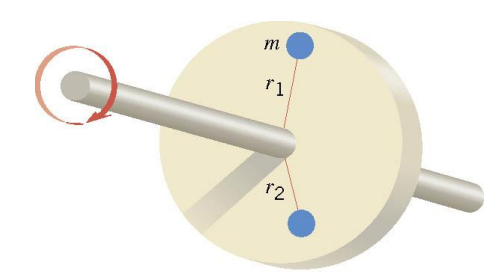
Requirement: The angular speed must be expressed in rad/s.
SI Unit of Rotational Kinetic Energy: joule (J)
Example 13 Rolling Cylinders
A thin-walled hollow cylinder (mass \(=m_{\mathrm{h}}\), radius \(=r_{\mathrm{h}}\) ) and a solid cylinder ( mass \(=m_{\mathrm{s}}\), radius \(=r_{\mathrm{s}}\) ) start from rest at the top of an incline.
▶️Answer/Explanation
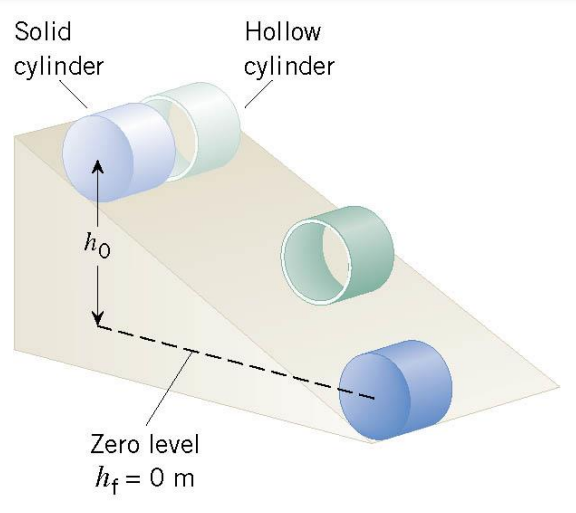
\(E=\frac{1}{2} m v^2+\frac{1}{2} I \omega^2+m g h\)
ENERGY CONSERVATION

$
\frac{1}{2} m v_f^2+\frac{1}{2} I \omega_f^2+m g h_f=\frac{1}{2} m v_i^2+\frac{1}{2} I \omega_i^2+m g h_i
$
\(\frac{1}{2} m v_f^2+\frac{1}{2} I \omega_f^2=m g h_i\)
\(\omega_f=v_f / r\)
\(\begin{gathered}\frac{1}{2} m v_f^2+\frac{1}{2} I v_f^2 / r^2=m g h_i \\ v_f=\sqrt{\frac{2 m g h_o}{m+I / r^2}}\end{gathered}\)

The cylinder with the smaller moment of inertia will have a greater final translational speed.
9.6 Angular Momentum
DEFINITION OF ANGULAR MOMENTUM
The angular momentum \(L\) of a body rotating about a fixed axis is the product of the body’s moment of inertia and its angular velocity with respect to that axis:
$
L=I \omega
$
Requirement: The angular speed must be expressed in rad/s.
SI Unit of Angular Momentum: \(\mathrm{kg} \cdot \mathrm{m}^2 / \mathrm{s}\)
PRINCIPLE OF CONSERVATION OFANGULAR MOMENTUM
The angular momentum of a system remains constant (is conserved) if the net external torque acting on the system is zero.
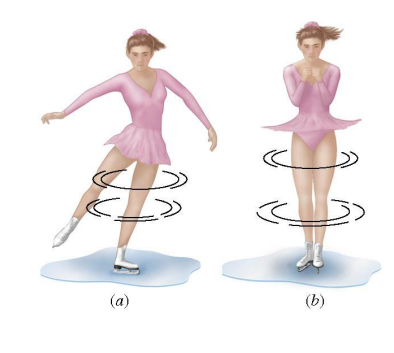
Conceptual Example 14 A Spinning Skater
An ice skater is spinning with both arms and a leg outstretched. She pulls her arms and leg inward and her spinning motion changes dramatically.
Use the principle of conservation of angular momentum to explain how and why her spinning motion changes.

9.6 Angular Momentum
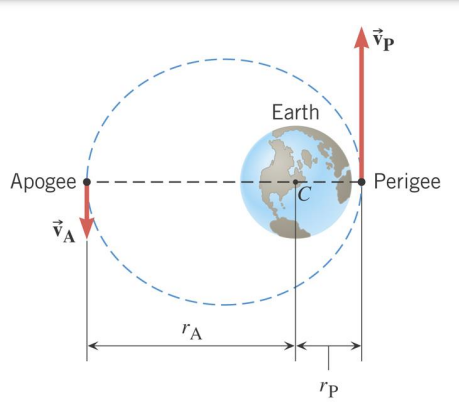
Example 15 A Satellite in an Elliptical Orbit
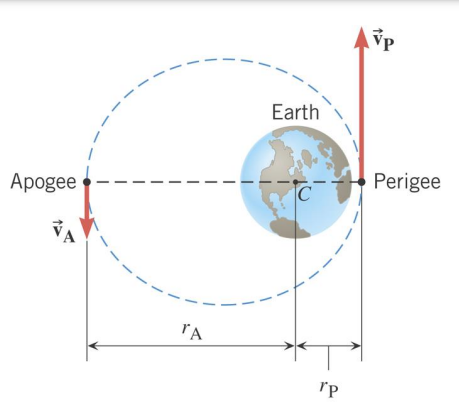
An artificial satellite is placed in an elliptical orbit about the earth. Its point of closest approach is \(8.37 \times 10^6 \mathrm{~m}\) from the center of the earth, and its point of greatest distance is \(25.1 \times 10^6 \mathrm{~m}\) from the center of the earth. The speed of the satellite at the perigee is \(8450 \mathrm{~m} / \mathrm{s}\). Find the speed at the apogee.
▶️Answer/Explanation
Ans:

$
L=I \omega
$
angular momentum conservatio
angular momentum conservation
$
I_A \omega_A=I_P \omega_P
$
\(\begin{aligned} & I=m r^2 \quad \omega=v / r \\ & m r_A^2 \frac{v_A}{r_A}=m r_P^2 \frac{v_P}{r_P}\end{aligned}\)

\(\begin{gathered}m r_A^2 \frac{v_A}{r_A}=m r_P^2 \frac{v_P}{r_P} \\ r_A v_A=r_P v_P\end{gathered}\)
\(r_A v_A=r_P v_P\)
\(v_A=\frac{r_P v_P}{r_A}=\frac{\left(8.37 \times 10^6 \mathrm{~m}\right)(8450 \mathrm{~m} / \mathrm{s})}{25.1 \times 10^6 \mathrm{~m}}=2820 \mathrm{~m} / \mathrm{s}\)