Question
The vector equation of line \(l\) is given as \(\left( {\begin{array}{*{20}{c}}
x \\
y \\
z
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
1 \\
3 \\
6
\end{array}} \right) + \lambda \left( {\begin{array}{*{20}{c}}
{ – 1} \\
2 \\
{ – 1}
\end{array}} \right)\) .
Find the Cartesian equation of the plane containing the line \(l\) and the point A(4, − 2, 5) .
Answer/Explanation
Markscheme
EITHER
\(l\) goes through the point (1, 3, 6) , and the plane contains A(4, –2, 5)
the vector containing these two points is on the plane, i.e.
\(\left( {\begin{array}{*{20}{c}}
1 \\
3 \\
6
\end{array}} \right) – \left( {\begin{array}{*{20}{c}}
4 \\
{ – 2} \\
5
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{ – 3} \\
5 \\
1
\end{array}} \right)\) (M1)A1
\(\left( {\begin{array}{*{20}{c}}
{ – 1} \\
2 \\
{ – 1}
\end{array}} \right) \times \left( {\begin{array}{*{20}{c}}
{ – 3} \\
5 \\
1
\end{array}} \right) = \left| {\begin{array}{*{20}{c}}
{\boldsymbol{i}}&{\boldsymbol{j}}&{\boldsymbol{k}} \\
{ – 1}&2&{ – 1} \\
{ – 3}&5&1
\end{array}} \right| = 7{\boldsymbol{i}} + 4{\boldsymbol{j}} + {\boldsymbol{k}}\) M1A1
\(\left( {\begin{array}{*{20}{c}}
4 \\
{ – 2} \\
5
\end{array}} \right) \cdot \left( {\begin{array}{*{20}{c}}
7 \\
4 \\
1
\end{array}} \right) = 25\) (M1)
hence, Cartesian equation of the plane is \(7x + 4y + z = 25\) A1
OR
finding a third point M1
e.g. (0, 5, 5) A1
three points are (1, 3, 6), (4, –2, 5), (0, 5, 5)
equation is \(ax + by + cz = 1\)
system of equations M1
\(a + 3b + 6c = 1\)
\(4a – 2b + 5c = 1\)
\(5b + 5c = 1\)
\(a = \frac{7}{{25}}\) , \(b = \frac{4}{{25}}\) , \(c = \frac{1}{{25}}\) , from GDC M1A1
so \(\frac{7}{{25}}x + \frac{4}{{25}}y + \frac{1}{{25}}z = 1\) A1
or \(7x + 4y + z = 25\)
[6 marks]
Question
(a) Find the coordinates of the point \(A\) on \({l_1}\) and the point \(B\) on \({l_2}\) such that \(\overrightarrow {{\text{AB}}} \) is perpendicular to both \({l_1}\) and \({l_2}\) .
(b) Find \(\left| {{\text{AB}}} \right|\) .
(c) Find the Cartesian equation of the plane \(\prod \) which contains \({l_1}\) and does not intersect \({l_2}\) .
Answer/Explanation
Markscheme
(a) on \({l_1}\) A(\( – 3 + 3\lambda \), \( – 4 + 2\lambda \), \(6 – 2\lambda \)) A1
on \({l_2}\) \({l_2}:r = \left( {\begin{array}{*{20}{c}}
4 \\
{ – 7} \\
3
\end{array}} \right) + \mu \left( {\begin{array}{*{20}{c}}
{ – 3} \\
4 \\
{ – 1}
\end{array}} \right)\) (M1)
\( \Rightarrow \) B(\(4 – 3\mu \), \( – 7 + 4\mu \), \( – 3 – \mu \)) A1
\(\overrightarrow {{\text{BA}}} = {\boldsymbol{a}} – {\boldsymbol{b}} = \left( {\begin{array}{*{20}{c}}
{3\lambda + 3\mu – 7} \\
{2\lambda – 4\mu + 3} \\
{ – 2\lambda + \mu + 9}
\end{array}} \right)\) (M1)A1
EITHER
\({\text{BA}} \bot {l_1} \Rightarrow {\text{BA}} \cdot \left( {\begin{array}{*{20}{c}}
3 \\
2 \\
{ – 2}
\end{array}} \right) = 0 \Rightarrow 3\left( {3\lambda + 3\mu – 7} \right) + 2\left( {2\lambda – 4\mu + 3} \right) – 2\left( { – 2\lambda + \mu + 9} \right) = 0\) M1
\( \Rightarrow 17\lambda – \mu = 33\) A1
\({\text{BA}} \bot {l_2} \Rightarrow {\text{BA}} \cdot \left( {\begin{array}{*{20}{c}}
{ – 3} \\
4 \\
{ – 1}
\end{array}} \right) = 0 \Rightarrow – 3\left( {3\lambda + 3\mu – 7} \right) + 4\left( {2\lambda – 4\mu + 3} \right) – \left( { – 2\lambda + \mu + 9} \right) = 0\) M1
\( \Rightarrow \lambda – 26\mu = – 24\) A1
solving both equations above simultaneously gives
\(\lambda = 2\); \(\mu = 1 \Rightarrow \) A(3, 0, 2), B(1, –3, –4) A1A1A1A1
OR
\(\left| {\begin{array}{*{20}{c}}
{\boldsymbol{i}}&{\boldsymbol{j}}&{\boldsymbol{k}} \\
3&2&{ – 2} \\
{ – 3}&4&{ – 1}
\end{array}} \right| = 6{\boldsymbol{i}} + 9{\boldsymbol{j}} + 18{\boldsymbol{k}}\) M1A1
so \(\overrightarrow {{\text{AB}}} = p\left( {\begin{array}{*{20}{c}}
2 \\
3 \\
6
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{3\lambda + 3\mu – 7} \\
{2\lambda – 4\mu + 3} \\
{ – 2\lambda + \mu + 9}
\end{array}} \right)\) M1A1
\({3\lambda + 3\mu – 2p = 7}\)
\({2\lambda – 4\mu – 3p = – 3}\)
\({ – 2\lambda + \mu – 6p = – 9}\)
\(\lambda = 2\), \(\mu = 1\), \(p = 1\) A1A1
A(\( – 3 + 6\), \( – 4 + 4\), \(6 – 4\)) \(=\) (\(3\), \(0\), \(2\)) A1
B(\(4 – 3\), \( – 7 + 4\), \( – 3 – 1\)) \(=\) (\(1\), \( – 3\), \( – 4\)) A1
[13 marks]
(b) \({\text{AB}} = \left( {\begin{array}{*{20}{c}}
1 \\
{ – 3} \\
{ – 4}
\end{array}} \right) – \left( {\begin{array}{*{20}{c}}
3 \\
0 \\
2
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{ – 2} \\
{ – 3} \\
{ – 6}
\end{array}} \right)\) (A1)
\(\left| {{\text{AB}}} \right| = \sqrt {{{\left( { – 2} \right)}^2} + {{\left( { – 3} \right)}^2} + {{\left( { – 6} \right)}^2}} = \sqrt {49} = 7\) M1A1
[3 marks]
(c) from (b) \(2{\boldsymbol{i}} + 3{\boldsymbol{j}} + 6{\boldsymbol{k}}\) is normal to both lines
\({l_1}\) goes through (–3, –4, 6) \( \Rightarrow \left( {\begin{array}{*{20}{c}}
{ – 3} \\
{ – 4} \\
6
\end{array}} \right) \cdot \left( {\begin{array}{*{20}{c}}
2 \\
3 \\
6
\end{array}} \right) = 18\) M1A1
hence, the Cartesian equation of the plane through \({l_1}\) , but not \({l_2}\) , is \(2x + 3y + 6z = 18\) A1
[3 marks]
Total [19 marks]
Question
A plane \(\pi \) has vector equation r = (−2i + 3j − 2k) + \(\lambda \)(2i + 3j + 2k) + \(\mu \)(6i − 3j + 2k).
(a) Show that the Cartesian equation of the plane \(\pi \) is 3x + 2y − 6z = 12.
(b) The plane \(\pi \) meets the x, y and z axes at A, B and C respectively. Find the coordinates of A, B and C.
(c) Find the volume of the pyramid OABC.
(d) Find the angle between the plane \(\pi \) and the x-axis.
(e) Hence, or otherwise, find the distance from the origin to the plane \(\pi \).
(f) Using your answers from (c) and (e), find the area of the triangle ABC.
Answer/Explanation
Markscheme
(a) EITHER
normal to plane given by
\(\left| {\begin{array}{*{20}{c}}
i&j&k \\
2&3&2 \\
6&{ – 3}&2
\end{array}} \right|\) M1A1
= 12i + 8j – 24k A1
equation of \(\pi \) is \(3x + 2y – 6z = d\) (M1)
as goes through (–2, 3, –2) so d = 12 M1A1
\(\pi :3x + 2y – 6z = 12\) AG
OR
\(x = – 2 + 2\lambda + 6\mu \)
\(y = 3 + 3\lambda – 3\mu \)
\(z = – 2 + 2\lambda + 2\mu \)
eliminating \(\mu \)
\(x + 2y = 4 + 8\lambda \)
\(2y + 3z = 12\lambda \) M1A1A1
eliminating \(\lambda \)
\(3(x + 2y) – 2(2y + 3z) = 12\) M1A1A1
\(\pi :3x + 2y – 6z = 12\) AG
[6 marks]
(b) therefore A(4, 0, 0), B(0, 6, 0) and C(0, 0, 2) A1A1A1
Note: Award A1A1A0 if position vectors given instead of coordinates.
[3 marks]
(c) area of base \({\text{OAB}} = \frac{1}{2} \times 4 \times 6 = 12\) M1
\(V = \frac{1}{3} \times 12 \times 2 = 8\) M1A1
[3 marks]
(d) \(\left( {\begin{array}{*{20}{c}}
3 \\
2 \\
{ – 6}
\end{array}} \right) \cdot \left( {\begin{array}{*{20}{c}}
1 \\
0 \\
0
\end{array}} \right) = 3 = 7 \times 1 \times \cos \phi \) M1A1
\(\phi = \arccos \frac{3}{7}\)
so \(\theta = 90 – \arccos \frac{3}{7} = 25.4^\circ \,\,\,\,\,\)(accept 0.443 radians) M1A1
[4 marks]
(e) \(d = 4\sin \theta = \frac{{12}}{7}\,\,\,\,\,( = 1.71)\) (M1)A1
[2 marks]
(f) \(8 = \frac{1}{3} \times \frac{{12}}{7} \times {\text{area}} \Rightarrow {\text{area}} = 14\) M1A1
Note: If answer to part (f) is found in an earlier part, award M1A1, regardless of the fact that it has not come from their answers to part (c) and part (e).
[2 marks]
Total [20 marks]
Question
The planes \(2x + 3y – z = 5\) and \(x – y + 2z = k\) intersect in the line \(5x + 1 = 9 – 5y = – 5z\) .
Find the value of k .
Answer/Explanation
Markscheme
point on line is \(x = \frac{{ – 1 – 5\lambda }}{5}{\text{, }}y = \frac{{9 + 5\lambda }}{5}{\text{, }}z = \lambda \) or similar M1A1
Note: Accept use of point on the line or elimination of one of the variables using the equations of the planes
\(\frac{{ – 1 – 5\lambda }}{5} – \frac{{9 + 5\lambda }}{5} + 2\lambda = k\) M1A1
Note: Award M1A1 if coordinates of point and equation of a plane is used to obtain linear equation in k or equations of the line are used in combination with equation obtained by elimination to get linear equation in k.
\(k = – 2\) A1
[5 marks]
Question
The coordinates of points A, B and C are given as \((5,\, – 2,\,5)\) , \((5,\,4,\, – 1)\) and \(( – 1,\, – 2,\, – 1)\) respectively.
Show that AB = AC and that \({\rm{B\hat AC}} = 60^\circ \).
Find the Cartesian equation of \(\Pi \), the plane passing through A, B, and C.
(i) Find the Cartesian equation of \({\Pi _1}\) , the plane perpendicular to (AB) passing through the midpoint of [AB] .
(ii) Find the Cartesian equation of \({\Pi _2}\) , the plane perpendicular to (AC) passing through the midpoint of [AC].
Find the vector equation of L , the line of intersection of \({\Pi _1}\) and \({\Pi _2}\) , and show that it is perpendicular to \(\Pi \) .
A methane molecule consists of a carbon atom with four hydrogen atoms symmetrically placed around it in three dimensions.
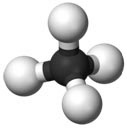
The positions of the centres of three of the hydrogen atoms are A, B and C as given. The position of the centre of the fourth hydrogen atom is D.
Using the fact that \({\text{AB}} = {\text{AD}}\) , show that the coordinates of one of the possible positions of the fourth hydrogen atom is \(( -1,\,4,\,5)\) .
A methane molecule consists of a carbon atom with four hydrogen atoms symmetrically placed around it in three dimensions.

The positions of the centres of three of the hydrogen atoms are A, B and C as given. The position of the centre of the fourth hydrogen atom is D.
Letting D be \(( – 1,\,4,\,5)\) , show that the coordinates of G, the position of the centre of the carbon atom, are \((2,\,1,\,2)\) . Hence calculate \({\rm{D}}\hat {\rm{G}}{\rm{A}}\) , the bonding angle of carbon.
Answer/Explanation
Markscheme
\(\overrightarrow {\text{AB}} = \left( {\begin{array}{*{20}{c}}
0 \\
6 \\
{ – 6}
\end{array}} \right) \Rightarrow {\text{AB}} = \sqrt {72} \) A1
\(\overrightarrow {\text{AC}} = \left( {\begin{array}{*{20}{c}}
{ – 6} \\
0 \\
{ – 6}
\end{array}} \right) \Rightarrow {\text{AC}} = \sqrt {72} \) A1
so they are the same AG
\(\overrightarrow {{\text{AB}}} \cdot \overrightarrow {{\text{AC}}} = 36 = \left( {\sqrt {72} } \right)\left( {\sqrt {72} } \right)\cos \theta \) (M1)
\(\cos \theta = \frac{{36}}{{\left( {\sqrt {72} } \right)\left( {\sqrt {72} } \right)}} = \frac{1}{2} \Rightarrow \theta = 60^\circ \) A1AG
Note: Award M1A1 if candidates find BC and claim that triangle ABC is equilateral.
[4 marks]
METHOD 1
\(\overrightarrow {{\text{AB}}} \times \overrightarrow {{\text{AC}}} = \left| {\begin{array}{*{20}{c}}
\boldsymbol{i}&\boldsymbol{j}&\boldsymbol{k}\\
0&6&{ – 6} \\
{ – 6}&0&{ – 6}
\end{array}} \right| = -36\boldsymbol{i} + 36\boldsymbol{j} + 36\boldsymbol{k}\) (M1)A1
equation of plane is \(x – y – z = k\) (M1)
goes through A, B or C \( \Rightarrow x – y – z = 2\) A1
[4 marks]
METHOD 2
\(x + by + cz = d\) (or similar) M1
\(5 – 2b + 5c = d\)
\(5 + 4b – c = d\) A1
\( -1 – 2b – c = d\)
solving simultaneously M1
\(b = -1,{\text{ }}c = -1,{\text{ }}d = 2\)
so \(x – y – z = 2\) A1
[4 marks]
(i) midpoint is \((5,\,1,\,2)\), so equation of \({\Pi _1}\) is \(y – z = -1\) A1A1
(ii) midpoint is \((2,\, – 2,\,2)\), so equation of \({\Pi _2}\) is \(x + z = 4\) A1A1
Note: In each part, award A1 for midpoint and A1 for the equation of the plane.
[4 marks]
EITHER
solving the two equations above M1
\(L:r = \left( {\begin{array}{*{20}{c}}
4 \\
{ – 1} \\
0
\end{array}} \right) + \lambda \left( {\begin{array}{*{20}{c}}
{ – 1} \\
1 \\
1
\end{array}} \right)\) A1
OR
L has the direction of the vector product of the normal vectors to the planes \({\Pi _1}\) and \({\Pi _2}\) (M1)
\(\left| {\begin{array}{*{20}{c}}
\boldsymbol{i}&\boldsymbol{j}&\boldsymbol{k} \\
0&1&{ – 1} \\
1&0&1
\end{array}} \right| = \boldsymbol{i} – \boldsymbol{j} – \boldsymbol{k}\)
(or its opposite) A1
THEN
direction is \(\left( {\begin{array}{*{20}{c}}
{ – 1} \\
1 \\
1
\end{array}} \right)\)as required R1
[3 marks]
D is of the form \((4 – \lambda ,\, -1 + \lambda ,\,\lambda )\) M1
\({(1 + \lambda )^2} + {( -1 – \lambda )^2} + {(5 – \lambda )^2} = 72\) M1
\(3{\lambda ^2} – 6\lambda – 45 = 0\)
\(\lambda = 5{\text{ or }}\lambda = -3\) A1
\({\text{D}}( -1,\,4,\,5)\) AG
Note: Award M0M0A0 if candidates just show that \({\text{D}}( -1,\,4,\,5)\) satisfies \({\text{AB}} = {\text{AD}}\);
Award M1M1A0 if candidates also show that D is of the form \((4 – \lambda ,\, -1 + \lambda ,\,\lambda )\)
[3 marks]
EITHER
G is of the form \((4 – \lambda ,\, – 1 + \lambda ,\,\lambda )\) and \({\text{DG}} = {\text{AG, BG or CG}}\) M1
e.g. \({(1 + \lambda )^2} + {( – 1 – \lambda )^2} + {(5 – \lambda )^2} = {(5 – \lambda )^2} + {(5 – \lambda )^2} + {(5 – \lambda )^2}\) M1
\({(1 + \lambda )^2} = {(5 – \lambda )^2}\)
\(\lambda = 2\) A1
\({\text{G}}(2,\,1,\,2)\) AG
OR
G is the centre of mass (barycentre) of the regular tetrahedron ABCD (M1)
\({\text{G}}\left( {\frac{{5 + 5 + ( – 1) + ( – 1)}}{4},\frac{{ – 2 + 4 + ( – 2) + 4}}{4},\frac{{5 + ( – 1) + ( – 1) + 5}}{4}} \right)\) M1A1
THEN
Note: the following part is independent of previous work and candidates may use AG to answer it (here it is possible to award M0M0A0A1M1A1)
\(\overrightarrow {GD} = \left( {\begin{array}{*{20}{c}}
{ – 3} \\
3 \\
3
\end{array}} \right)\) and \(\overrightarrow {GA} = \left( {\begin{array}{*{20}{c}}
3 \\
{ – 3} \\
3
\end{array}} \right)\) A1
\(\cos \theta = \frac{{ – 9}}{{\left( {3\sqrt 3 } \right)\left( {3\sqrt 3 } \right)}} = – \frac{1}{3} \Rightarrow \theta = 109^\circ \) (or 1.91 radians) M1A1
[6 marks]
Question
Find the values of k for which the following system of equations has no solutions and the value of k for the system to have an infinite number of solutions.
\[x – 3y + z = 3\]
\[x + 5y – 2z = 1\]
\[16y – 6z = k\]
Given that the system of equations can be solved, find the solutions in the form of a vector equation of a line, r = a + λb , where the components of b are integers.
The plane \( \div \) is parallel to both the line in part (b) and the line \(\frac{{x – 4}}{3} = \frac{{y – 6}}{{ – 2}} = \frac{{z – 2}}{0}\).
Given that \( \div \) contains the point (1, 2, 0) , show that the Cartesian equation of ÷ is 16x + 24y − 11z = 64 .
The z-axis meets the plane \( \div \) at the point P. Find the coordinates of P.
Find the angle between the line \(\frac{{x – 2}}{3} = \frac{{y + 5}}{4} = \frac{z}{2}\) and the plane \( \div \) .
Answer/Explanation
Markscheme
in augmented matrix form \(\left| {\begin{array}{*{20}{c}}
1&{ – 3}&1&3 \\
1&5&{ – 2}&1 \\
0&{16}&{ – 6}&k
\end{array}} \right|\)
attempt to find a line of zeros (M1)
\({r_2} – {r_1}\left| {\begin{array}{*{20}{c}}
1&{ – 3}&1&3 \\
0&8&{ – 3}&{ – 2} \\
0&{16}&{ – 6}&k
\end{array}} \right|\) (A1)
\({r_3} – 2{r_2}\left| {\begin{array}{*{20}{c}}
1&{ – 3}&1&3 \\
0&8&{ – 3}&{ – 2} \\
0&{0}&{0}&{k + 4}
\end{array}} \right|\) (A1)
there is an infinite number of solutions when \(k = – 4\) R1
there is no solution when
\(k \ne – 4,{\text{ }}(k \in \mathbb{R})\) R1
Note: Approaches other than using the augmented matrix are acceptable.
[5 marks]
using \(\left| {\begin{array}{*{20}{c}}
1&{ – 3}&1&3 \\
0&8&{ – 3}&{ – 2} \\
0&{0}&{ 0}&{k + 4}
\end{array}} \right|\) and letting \(\boldsymbol{z} = \lambda \) (M1)
\(8y – 3\lambda = – 2\)
\( \Rightarrow y = \frac{{3\lambda – 2}}{8}\) (A1)
\(x – 3y + z = 3\)
\( \Rightarrow x – \left( {\frac{{9\lambda – 6}}{8}} \right) + \lambda = 3\) (M1)
\( \Rightarrow 8x – 9\lambda + 6 + 8\lambda = 24\)
\( \Rightarrow x = \frac{{18 + \lambda }}{8}\) (A1)
\( \Rightarrow \left( {\begin{array}{*{20}{c}}
x \\
y \\
z
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{\frac{{18}}{8}} \\
{ – \frac{2}{8}} \\
0
\end{array}} \right) + \lambda \left( {\begin{array}{*{20}{c}}
{\frac{1}{8}} \\
{\frac{3}{8}} \\
1
\end{array}} \right)\) (M1)(A1)
\(r = \left( {\begin{array}{*{20}{c}}
{\frac{9}{4}} \\
{ – \frac{1}{4}} \\
0
\end{array}} \right) + \lambda \left( {\begin{array}{*{20}{c}}
1 \\
3 \\
8
\end{array}} \right)\) A1
Note: Accept equivalent answers.
[7 marks]
recognition that \(\left( {\begin{array}{*{20}{c}}
3 \\
{ – 2} \\
0
\end{array}} \right)\) is parallel to the plane (A1)
direction normal of the plane is given by \(\left| {\begin{array}{*{20}{c}}
\boldsymbol{i}&\boldsymbol{j}&\boldsymbol{k} \\
1&3&8 \\
3&{ – 2}&0
\end{array}} \right|\) (M1)
= 16i + 24j – 11k A1
Cartesian equation of the plane is given by 16x + 24y –11z = d and a point which fits this equation is (1, 2, 0) (M1)
\( \Rightarrow 16 + 48 = d\)
d = 64 A1
hence Cartesian equation of plane is 16x + 24y –11z = 64 AG
Note: Accept alternative methods using dot product.
[5 marks]
the plane crosses the z-axis when x = y = 0 (M1)
coordinates of P are \(\left( {0,\,0,\, – \frac{{64}}{{11}}} \right)\) A1
Note: Award A1 for stating \(z = – \frac{{64}}{{11}}\).
Note: Accept. \(\left( {\begin{array}{*{20}{c}}
0 \\
0 \\
{ – \frac{{64}}{{11}}}
\end{array}} \right)\)
[2 marks]
recognition that the angle between the line and the direction normal is given by:
\(\left( {\begin{array}{*{20}{c}}
3 \\
4 \\
2
\end{array}} \right)\left( {\begin{array}{*{20}{c}}
{16} \\
{24} \\
{-11}
\end{array}} \right) = \sqrt {29} \sqrt {953} \cos \theta \) where \(\theta \) is the angle between the line and the normal vector M1A1
\( \Rightarrow 122 = \sqrt {29} \sqrt {953} \cos \theta \) (A1)
\( \Rightarrow \theta = 42.8^\circ {\text{ (0.747 radians)}}\) (A1)
hence the angle between the line and the plane is 90° – 42.8° = 47.2° (0.824 radians) A1
[5 marks]
Note: Accept use of the formula a.b = \(\left| {} \right.\)a\(\left. {} \right|\)\(\left| {} \right.\)b\(\left| {\sin \theta } \right.\) .
Question
Consider the planes \({\pi _1}:x – 2y – 3z = 2{\text{ and }}{\pi _2}:2x – y – z = k\) .
Find the angle between the planes \({\pi _1}\)and \({\pi _2}\) .
The planes \({\pi _1}\) and \({\pi _2}\) intersect in the line \({L_1}\) . Show that the vector equation of
\({L_1}\) is \(r = \left( {\begin{array}{*{20}{c}}
0\\
{2 – 3k}\\
{2k – 2}
\end{array}} \right) + t\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\)
The line \({L_2}\) has Cartesian equation \(5 – x = y + 3 = 2 – 2z\) . The lines \({L_1}\) and \({L_2}\) intersect at a point X. Find the coordinates of X.
Determine a Cartesian equation of the plane \({\pi _3}\) containing both lines \({L_1}\) and \({L_2}\) .
Let Y be a point on \({L_1}\) and Z be a point on \({L_2}\) such that XY is perpendicular to YZ and the area of the triangle XYZ is 3. Find the perimeter of the triangle XYZ.
Answer/Explanation
Markscheme
Note: Accept alternative notation for vectors (eg \(\langle a{\text{, }}b{\text{, }}c\rangle {\text{ or }}\left( {a{\text{, }}b{\text{, }}c} \right)\)).
\(\boldsymbol{n} = \left( {\begin{array}{*{20}{c}}
1 \\
{ – 2} \\
{ – 3}
\end{array}} \right)\) and \(\boldsymbol{m} = \left( {\begin{array}{*{20}{c}}
2 \\
{ – 1} \\
{ – 1}
\end{array}} \right)\) (A1)
\(\cos \theta = \frac{{\boldsymbol{n} \cdot \boldsymbol{m}}}{{\left| \boldsymbol{n} \right|\left| \boldsymbol{m} \right|}}\) (M1)
\(\cos \theta = \frac{{2 + 2 + 3}}{{\sqrt {1 + 4 + 9} \sqrt {4 + 1 + 1} }} = \frac{7}{{\sqrt {14} \sqrt 6 }}\) A1
\(\theta = 40.2^\circ \,\,\,\,\,(0.702{\text{ rad}})\) A1
[4 marks]
Note: Accept alternative notation for vectors (eg \(\langle a{\text{, }}b{\text{, }}c\rangle {\text{ or }}\left( {a{\text{, }}b{\text{, }}c} \right)\)).
METHOD 1
eliminate z from x – 2y – 3z = 2 and 2x – y – z = k
\(5x – y = 3k – 2 \Rightarrow x = \frac{{y – (2 – 3k)}}{5}\) M1A1
eliminate y from x – 2y – 3z = 2 and 2x – y – z = k
\(3x + z = 2k – 2 \Rightarrow x = \frac{{z – (2k – 2)}}{{ – 3}}\) A1
x = t, y = (2 − 3k) + 5t and z = (2k − 2) − 3t A1A1
\(r = \left( {\begin{array}{*{20}{c}}
0\\
{2 – 3k}\\
{2k – 2}
\end{array}} \right) + t\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\) AG
[5 marks]
METHOD 2
\(\left( {\begin{array}{*{20}{c}}
1\\
{ – 2}\\
{ – 3}
\end{array}} \right) \times \left( {\begin{array}{*{20}{c}}
2\\
{ – 1}\\
{ – 1}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
{ – 1}\\
{ – 5}\\
3
\end{array}} \right) \Rightarrow {\text{direction is }}\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\) M1A1
Let x = 0
\(0 – 2y – 3z = 2{\text{ and }}2 \times 0 – y – z = k\) (M1)
solve simultaneously (M1)
\(y = 2 – 3k{\text{ and }}z = 2k – 2\) A1
therefore r \( = \left( {\begin{array}{*{20}{c}}
0\\
{2 – 3k}\\
{2k – 2}
\end{array}} \right) + t\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\) AG
[5 marks]
METHOD 3
substitute \(x = t,{\text{ }}y = (2 – 3k) + 5t{\text{ and }}z = (2k – 2) – 3t{\text{ into }}{\pi _1}{\text{ and }}{\pi _2}\) M1
for \({\pi _1}:t – 2(2 – 3k + 5t) – 3(2k – 2 – 3t) = 2\) A1
for \({\pi _2}:2t – (2 – 3k + 5t) – (2k – 2 – 3t) = k\) A1
the planes have a unique line of intersection R2
therefore the line is \(r = \left( {\begin{array}{*{20}{c}}
0\\
{2 – 3k}\\
{2k – 2}
\end{array}} \right) + t\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\) AG
[5 marks]
Note: Accept alternative notation for vectors (eg \(\langle a{\text{, }}b{\text{, }}c\rangle {\text{ or }}\left( {a{\text{, }}b{\text{, }}c} \right)\)).
\(5 – t = (2 – 3k + 5t) + 3 = 2 – 2(2k – 2 – 3t)\) M1A1
Note: Award M1A1 if candidates use vector or parametric equations of \({L_2}\)
eg \(\left( {\begin{array}{*{20}{c}}
0\\
{2 – 3k}\\
{2k – 2}
\end{array}} \right) + t\left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
5\\
{ – 3}\\
1
\end{array}} \right) + s\left( {\begin{array}{*{20}{c}}
{ – 2}\\
2\\
{ – 1}
\end{array}} \right)\) or \( \Rightarrow \left\{ {\begin{array}{*{20}{l}}
{t = 5 – 2s}\\
{2 – 3k + 5t = – 3 + 2s}\\
{2k – 2 – 3t = 1 + s}
\end{array}} \right.\)
solve simultaneously M1
\(k = 2,{\text{ }}t = 1{\text{ }}(s = 2)\) A1
intersection point (\(1\), \(1\), \( – 1\)) A1
[5 marks]
Note: Accept alternative notation for vectors (eg \(\langle a{\text{, }}b{\text{, }}c\rangle {\text{ or }}\left( {a{\text{, }}b{\text{, }}c} \right)\)).
\({\overrightarrow l _2} = \left( {\begin{array}{*{20}{c}}
2\\
{ – 2}\\
1
\end{array}} \right)\) A1
\({\overrightarrow l _1} \times {\overrightarrow l _2} = \left| {\begin{array}{*{20}{c}}
\boldsymbol{i}&\boldsymbol{j}&\boldsymbol{k}\\
1&5&{ – 3}\\
2&{ – 2}&1
\end{array}} \right| = \left( {\begin{array}{*{20}{c}}
{ – 1}\\
{ – 7}\\
{ – 12}
\end{array}} \right)\) (M1)A1
\(\boldsymbol{r} \cdot \left( {\begin{array}{*{20}{c}}
1\\
7\\
{12}
\end{array}} \right) = \left( {\begin{array}{*{20}{c}}
1\\
1\\
{ – 1}
\end{array}} \right) \cdot \left( {\begin{array}{*{20}{c}}
1\\
7\\
{12}
\end{array}} \right)\) (M1)
\(x + 7y + 12z = – 4\) A1
[5 marks]
Note: Accept alternative notation for vectors (eg \(\langle a{\text{, }}b{\text{, }}c\rangle {\text{ or }}\left( {a{\text{, }}b{\text{, }}c} \right)\)).
Let \(\theta \) be the angle between the lines \({\overrightarrow l _1} = \left( {\begin{array}{*{20}{c}}
1\\
5\\
{ – 3}
\end{array}} \right)\) and \({\overrightarrow l _2} = \left( {\begin{array}{*{20}{c}}
2\\
{ – 2}\\
1
\end{array}} \right)\)
\(\cos \theta = \frac{{\left| {2 – 10 – 3} \right|}}{{\sqrt {35} \sqrt 9 }} \Rightarrow \theta = 0.902334…{\text{ }}51.699…^\circ )\) (M1)
as the triangle XYZ has a right angle at Y,
\({\text{XZ}} = a \Rightarrow {\text{YZ}} = a\sin \theta {\text{ and XY}} = a\cos \theta \) (M1)
\({\text{area = 3}} \Rightarrow \frac{{{a^2}\sin \theta \cos \theta }}{2} = 3\) (M1)
\(a = 3.5122…\) (A1)
perimeter \( = a + a\sin \theta + a\cos \theta = 8.44537… = 8.45\) A1
Note: If candidates attempt to find coordinates of Y and Z award M1 for expression of vector YZ in terms of two parameters, M1 for attempt to use perpendicular condition to determine relation between parameters, M1 for attempt to use the area to find the parameters and A2 for final answer.
[5 marks]
Examiners report
Although this was the last question in part B, it was answered surprisingly well by many candidates, except for part (e). Even those who had not done so well elsewhere often gained a number of marks in some parts of the question. Nevertheless the presence of parameters seemed to have blocked the abilities of weaker candidates to solve situations in which vectors were involved. Mathematical skills for this particular question were sometimes remarkable, however, calculations proved incomplete due to the way that planes were presented. Most candidates found a correct angle in part (a). Occasional arithmetic errors in calculating the magnitude of a vector and dot product occurred. In part (b) the vector product approach was popular. In some case candidates simply verified the result by substitution. There was a lot of simultaneous equation solving, much of which was not very pretty. In part (c), a number of candidates made errors when attempting to solve a system of equations involving parameters. Many of the results for the point were found in terms of k. It was notorious that candidates did not use their GDC to try to find the coordinates of the intersection point between lines. In part (d), a number of candidates used an incorrect point but this part was often done well.
Very few excellent answers to part (e) were seen using an efficient method. Most candidates attempted methods involving heavy algebraic manipulation and had little success in this part of the question.
Although this was the last question in part B, it was answered surprisingly well by many candidates, except for part (e). Even those who had not done so well elsewhere often gained a number of marks in some parts of the question. Nevertheless the presence of parameters seemed to have blocked the abilities of weaker candidates to solve situations in which vectors were involved. Mathematical skills for this particular question were sometimes remarkable, however, calculations proved incomplete due to the way that planes were presented. Most candidates found a correct angle in part (a). Occasional arithmetic errors in calculating the magnitude of a vector and dot product occurred. In part (b) the vector product approach was popular. In some case candidates simply verified the result by substitution. There was a lot of simultaneous equation solving, much of which was not very pretty. In part (c), a number of candidates made errors when attempting to solve a system of equations involving parameters. Many of the results for the point were found in terms of k. It was notorious that candidates did not use their GDC to try to find the coordinates of the intersection point between lines. In part (d), a number of candidates used an incorrect point but this part was often done well.
Very few excellent answers to part (e) were seen using an efficient method. Most candidates attempted methods involving heavy algebraic manipulation and had little success in this part of the question.
Although this was the last question in part B, it was answered surprisingly well by many candidates, except for part (e). Even those who had not done so well elsewhere often gained a number of marks in some parts of the question. Nevertheless the presence of parameters seemed to have blocked the abilities of weaker candidates to solve situations in which vectors were involved. Mathematical skills for this particular question were sometimes remarkable, however, calculations proved incomplete due to the way that planes were presented. Most candidates found a correct angle in part (a). Occasional arithmetic errors in calculating the magnitude of a vector and dot product occurred. In part (b) the vector product approach was popular. In some case candidates simply verified the result by substitution. There was a lot of simultaneous equation solving, much of which was not very pretty. In part (c), a number of candidates made errors when attempting to solve a system of equations involving parameters. Many of the results for the point were found in terms of k. It was notorious that candidates did not use their GDC to try to find the coordinates of the intersection point between lines. In part (d), a number of candidates used an incorrect point but this part was often done well.
Very few excellent answers to part (e) were seen using an efficient method. Most candidates attempted methods involving heavy algebraic manipulation and had little success in this part of the question.
Although this was the last question in part B, it was answered surprisingly well by many candidates, except for part (e). Even those who had not done so well elsewhere often gained a number of marks in some parts of the question. Nevertheless the presence of parameters seemed to have blocked the abilities of weaker candidates to solve situations in which vectors were involved. Mathematical skills for this particular question were sometimes remarkable, however, calculations proved incomplete due to the way that planes were presented. Most candidates found a correct angle in part (a). Occasional arithmetic errors in calculating the magnitude of a vector and dot product occurred. In part (b) the vector product approach was popular. In some case candidates simply verified the result by substitution. There was a lot of simultaneous equation solving, much of which was not very pretty. In part (c), a number of candidates made errors when attempting to solve a system of equations involving parameters. Many of the results for the point were found in terms of k. It was notorious that candidates did not use their GDC to try to find the coordinates of the intersection point between lines. In part (d), a number of candidates used an incorrect point but this part was often done well.
Very few excellent answers to part (e) were seen using an efficient method. Most candidates attempted methods involving heavy algebraic manipulation and had little success in this part of the question.
Although this was the last question in part B, it was answered surprisingly well by many candidates, except for part (e). Even those who had not done so well elsewhere often gained a number of marks in some parts of the question. Nevertheless the presence of parameters seemed to have blocked the abilities of weaker candidates to solve situations in which vectors were involved. Mathematical skills for this particular question were sometimes remarkable, however, calculations proved incomplete due to the way that planes were presented. Most candidates found a correct angle in part (a). Occasional arithmetic errors in calculating the magnitude of a vector and dot product occurred. In part (b) the vector product approach was popular. In some case candidates simply verified the result by substitution. There was a lot of simultaneous equation solving, much of which was not very pretty. In part (c), a number of candidates made errors when attempting to solve a system of equations involving parameters. Many of the results for the point were found in terms of k. It was notorious that candidates did not use their GDC to try to find the coordinates of the intersection point between lines. In part (d), a number of candidates used an incorrect point but this part was often done well.
Very few excellent answers to part (e) were seen using an efficient method. Most candidates attempted methods involving heavy algebraic manipulation and had little success in this part of the question.
Question
The lines \({l_1}\) and \({l_2}\) are defined as
\({l_1}:\frac{{x – 1}}{3} = \frac{{y – 5}}{2} = \frac{{z – 12}}{{ – 2}}\)
\({l_2}:\frac{{x – 1}}{8} = \frac{{y – 5}}{{11}} = \frac{{z – 12}}{6}\).
The plane \(\pi \) contains both \({l_1}\) and \({l_2}\).
Find the Cartesian equation of \(\pi \).
The line \({l_3}\) passing through the point \((4,{\text{ }}0,{\text{ }}8)\) is perpendicular to \(\pi \).
Find the coordinates of the point where \({l_3}\) meets \(\pi \).
Answer/Explanation
Markscheme
attempting to find a normal to \(\pi {\text{ }}eg{\text{ }}\left( {\begin{array}{*{20}{c}} 3 \\ 2 \\ { – 2} \end{array}} \right) \times \left( {\begin{array}{*{20}{c}} 8 \\ {11} \\ 6 \end{array}} \right)\) (M1)
\(\left( {\begin{array}{*{20}{c}} 3 \\ 2 \\ { – 2} \end{array}} \right) \times \left( {\begin{array}{*{20}{c}} 8 \\ {11} \\ 6 \end{array}} \right) = 17\left( {\begin{array}{*{20}{c}} 2 \\ { – 2} \\ 1 \end{array}} \right)\) (A1)
\({{r}} \bullet \left( {\begin{array}{*{20}{c}} 2 \\ { – 2} \\ 1 \end{array}} \right) = \left( {\begin{array}{*{20}{c}} 1 \\ 5 \\ {12} \end{array}} \right) \bullet \left( {\begin{array}{*{20}{c}} 2 \\ { – 2} \\ 1 \end{array}} \right)\) M1
\(2x – 2y + z = 4\) (or equivalent) A1
[4 marks]
\({l_3}:{{r}} = \left( {\begin{array}{*{20}{c}} 4 \\ 0 \\ 8 \end{array}} \right) + t\left( {\begin{array}{*{20}{c}} 2 \\ { – 2} \\ 1 \end{array}} \right),\;\;\;t \in \mathbb{R}\) (A1)
attempting to solve \(\left( {\begin{array}{*{20}{c}} {4 + 2t} \\ { – 2t} \\ {8 + t} \end{array}} \right) \bullet \left( {\begin{array}{*{20}{c}} 2 \\ { – 2} \\ 1 \end{array}} \right) = 4\;\;\;{\text{for }}t\;\;\;ie{\text{ }}9t + 16 = 4\;\;\;{\text{for }}t\) M1
\(t = – \frac{4}{3}\) A1
\(\left( {\frac{4}{3},{\text{ }}\frac{8}{3},{\text{ }}\frac{{20}}{3}} \right)\) A1
[4 marks]
Total [8 marks]
Examiners report
Part (a) was reasonably well done. Some candidates made numerical errors when attempting to find a normal to \(\pi \).
In part (b), a number of candidates were awarded follow through marks from numerical errors committed in part (a).