Question
At a skiing competition the mean time of the first three skiers is 34.1 seconds. The time for the fourth skier is then recorded and the mean time of the first four skiers is 35.0 seconds. Find the time achieved by the fourth skier.
▶️Answer/Explanation
Markscheme
total time of first 3 skiers \( = 34.1 \times 3 = 102.3\) (M1)A1
total time of first 4 skiers \( = 35.0 \times 4 = 140.0\) A1
time taken by fourth skier \( = 140.0 – 102.3 = 37.7{\text{ (seconds)}}\) A1 [4 marks]
Question
The discrete random variable X has the following probability distribution, where p is a constant.
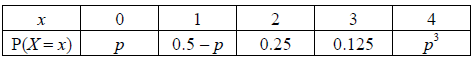
a. Find the value of p.[2]
b.i. Find μ, the expected value of X.[2]
b.ii. Find P(X > μ). [2]
▶️Answer/Explanation
Markscheme
a.equating sum of probabilities to 1 (p + 0.5 − p + 0.25 + 0.125 + p3 = 1) M1
p3 = 0.125 = \(\frac{1}{8}\)
p= 0.5 A1
[2 marks]
μ = 0 × 0.5 + 1 × 0 + 2 × 0.25 + 3 × 0.125 + 4 × 0.125 M1
= 1.375 \(\left( { = \frac{{11}}{8}} \right)\) A1
[2 marks]
P(X > μ) = P(X = 2) + P(X = 3) + P(X = 4) (M1)
= 0.5 A1
Note: Do not award follow through A marks in (b)(i) from an incorrect value of p.
Note: Award M marks in both (b)(i) and (b)(ii) provided no negative probabilities, and provided a numerical value for μ has been found.
[2 marks]
Question
Consider the data \(x_1,x_2,x_3, …, x_n,\) with mean \(\overline{x}\), and standard deviation \(s\).
- If each number is increased by \(k\),
- show that the new mean is \(\overline{x}+k\) (i.e. it is also increased by \(k\))
- show that the new standard deviation is \(s\)(i.e. it remains the same)
- If each number is multiplied by \(k\)
- show that the new mean is \(k\overline{x}\) (i.e. it is also multiplied by \(k\))
- show that the new standard deviation is \(ks\)(i.e. it is also multiplied by \(k\))
- write down the relation between the original and the new variance.
▶️Answer/Explanation
Ans:
- \(\overline{x}_{new}=\frac{\sum_{i=1}^{n}(x_i+k)}{n}=\frac{\sum_{i=1}^{n}x_i+\sum_{i=1}^{n}k}{n}=\frac{\sum_{i=1}^{n}x_i}{n}+\frac{\sum_{i=1}^{n}k}{n}=\overline{x}+\frac{kn}{n}=\overline{x}+k\)
\(s_{new}=\sqrt{\frac{\sum_{i=1}^{n} ((x_i+k)-(\overline{x}-k))^2 }{n}}=\sqrt{\frac{\sum_{i=1}^{n}(x_i-\overline{x})^2)}{n}}=s\) - \(\overline{x}_{new}=\frac{\sum_{i=1}^{n}(kx_i)}{n}=\frac{k\sum_{i=1}^{n}(x_i)}{n}=k\frac{\sum_{i=1}^{n}(x_i)}{n}=k\overline{x}\)
\(s_{new}=\sqrt{\frac{\sum_{i=1}^{n}(kx_i-k\overline{x})^2}{n}}=\sqrt{\frac{k^2\sum_{i=1}^{n}(x_i-\overline{x})^2}{n}}=k\sqrt{\frac{\sum_{i=1}^{n}(x_i-\overline{x})^2}{n}}=ks\)
\(s_{new}=ks\)⇒\(s^2_{new}=k^2s^2\)
so the original variance is multiplied by \(k^2\).
Question
Consider the following data
| x | 1 | 2 | 3 | 4 |
| y | 2 | 3 | 7 | 8 |
- Find the mean and the variance for the values of \(x\).
- Find the mean and the variance for the values of \(y\).
- Find the correlation coefficient \(r\).
- Describe the relation between \(x\) and \(y\).
- Find the equation \(y = ax+b\) of the regression line for \(y\) on \(x\).
- Find the equation \(x = cy+d\) of the regression line for \(x\) on \(y\).
- Find the inverse of the function in question \((e);\) Is it the function in question \((f)\)?
▶️Answer/Explanation
Ans:
- \(\mu_x = 2.5, \sigma_x^2= 1.11803^2= 1.25\)
- \(\mu_y = 5, \sigma_y^2=2.54951^2= 6.5\)
- 0.965
- strong positive
- \(y = 2.2x – 0.5\)
- \(x = 0.423y + 0.385\)
- \(y = 2.2x – 0.5\) ⇔ \(y + 0.5 = 2.2x\) ⇔ \(x = 0.455 y + 0.227\). They are different