Question
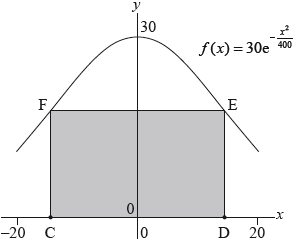
The following diagram shows a vertical cross section of a building. The cross section of the roof of the building can be modelled by the curve \(f(x) = 30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\), where \( – 20 \le x \le 20\).
Ground level is represented by the \(x\)-axis.
a.Find \(f”(x)\).[4]
b.Show that the gradient of the roof function is greatest when \(x = – \sqrt {200} \).[3]
Show that the maximum area \(A\) of the rectangle \(CDEF\) is \(600\sqrt 2 {{\text{e}}^{ – \frac{1}{2}}}\).[5]
(i) Find an expression for \(P\) in terms of \(a\).
(ii) Find the value of \(a\) which minimizes \(I\).
(iii) Using the value of \(a\) found in part (ii) calculate the percentage of the cross sectional area under the whole roof that is not included in the cross section of the living space.[9]
▶️Answer/Explanation
Markscheme
\(f'(x) = 30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}} \bullet – \frac{{2x}}{{400}}\;\;\;\left( { = – \frac{{3x}}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}} \right)\) M1A1
Note: Award M1 for attempting to use the chain rule.
\(f”(x) = – \frac{3}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}} + \frac{{3{x^2}}}{{4000}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\;\;\;\left( { = \frac{3}{{20}}{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}\left( {\frac{{{x^2}}}{{200}} – 1} \right)} \right)\) M1A1
Note: Award M1 for attempting to use the product rule.
[4 marks]
the roof function has maximum gradient when \(f”(x) = 0\) (M1)
Note: Award (M1) for attempting to find \(f”\left( { – \sqrt {200} } \right)\).
EITHER
\( = 0\) A1
OR
\(f”(x) = 0 \Rightarrow x = \pm \sqrt {200} \) A1
THEN
valid argument for maximum such as reference to an appropriate graph or change in the sign of \(f”(x)\) eg \(f”( – 15) = 0.010 \ldots ( > 0)\) and \(f”( – 14) = – 0.001 \ldots ( < 0)\) R1
\( \Rightarrow x = – \sqrt {200} \) AG
[3 marks]
\(A = 2a \bullet 30{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}\;\;\;\left( { = 60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} = – 400g'(a)} \right)\) (M1)(A1)
EITHER
\(\frac{{{\text{d}}A}}{{{\text{d}}a}} = 60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} \bullet – \frac{a}{{200}} + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}} = 0 \Rightarrow a = \sqrt {200} {\text{ }}\left( { – 400f”(a) = 0 \Rightarrow a = \sqrt {200} } \right)\) M1A1
OR
by symmetry eg \(a = – \sqrt {200} \) found in (b) or \({A_{{\text{max}}}}\) coincides with \(f”(a) = 0\) R1
\( \Rightarrow a = \sqrt {200} \) A1
Note: Award A0(M1)(A1)M0M1 for candidates who start with \(a = \sqrt {200} \) and do not provide any justification for the maximum area. Condone use of \(x\).
THEN
\({A_{{\text{max}}}} = 60 \bullet \sqrt {200} {{\text{e}}^{ – \frac{{200}}{{400}}}}\) M1
\( = 600\sqrt 2 {{\text{e}}^{ – \frac{1}{2}}}\) AG
[5 marks]
(i) perimeter \( = 4a + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}\) A1A1
Note: Condone use of \(x\).
(ii) \(I(a) = \frac{{4a + 60{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}}}{{60a{{\text{e}}^{ – \frac{{{a^2}}}{{400}}}}}}\) (A1)
graphing \(I(a)\) or other valid method to find the minimum (M1)
\(a = 12.6\) A1
(iii) area under roof \( = \int_{ – 20}^{20} {30{{\text{e}}^{ – \frac{{{x^2}}}{{400}}}}} {\text{d}}x\) M1
\( = 896.18 \ldots \) (A1)
area of living space \( = 60 \cdot (12.6…) \cdot e – {\frac{{(12.6…)}}{{400}}^2} = 508.56…\)
percentage of empty space \( = 43.3\% \) A1
[9 marks]
Total [21 marks]
Question
Consider the curve defined by the equation \(4{x^2} + {y^2} = 7\).
a.Find the equation of the normal to the curve at the point \(\left( {1,{\text{ }}\sqrt 3 } \right)\).[6]
b.Find the volume of the solid formed when the region bounded by the curve, the \(x\)-axis for \(x \geqslant 0\) and the \(y\)-axis for \(y \geqslant 0\) is rotated through \(2\pi \) about the \(x\)-axis.[3]
▶️Answer/Explanation
Markscheme
METHOD 1
\(4{x^2} + {y^2} = 7\)
\(8x + 2y\frac{{{\text{d}}y}}{{{\text{d}}x}} = 0\) (M1)(A1)
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – \frac{{4x}}{y}\)
Note: Award M1A1 for finding \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – 2.309 \ldots \) using any alternative method.
hence gradient of normal \( = \frac{y}{{4x}}\) (M1)
hence gradient of normal at \(\left( {1,{\text{ }}\sqrt 3 } \right)\) is \(\frac{{\sqrt 3 }}{4}\,\,\,( = 0.433)\) (A1)
hence equation of normal is \(y – \sqrt 3 = \frac{{\sqrt 3 }}{4}(x – 1)\) (M1)A1
\(\left( {y = \frac{{\sqrt 3 }}{4}x + \frac{{3\sqrt 3 }}{4}} \right)\,\,\,(y = 0.433x + 1.30)\)
METHOD 2
\(4{x^2} + {y^2} = 7\)
\(y = \sqrt {7 – 4{x^2}} \) (M1)
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – \frac{{4x}}{{\sqrt {7 – 4{x^2}} }}\) (A1)
Note: Award M1A1 for finding \(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – 2.309 \ldots \) using any alternative method.
hence gradient of normal \( = \frac{{\sqrt {7 – 4{x^2}} }}{{4x}}\) (M1)
hence gradient of normal at \(\left( {1,{\text{ }}\sqrt 3 } \right)\) is \(\frac{{\sqrt 3 }}{4}\,\,\,( = 0.433)\) (A1)
hence equation of normal is \(y – \sqrt 3 = \frac{{\sqrt 3 }}{4}(x – 1)\) (M1)A1
\(\left( {y = \frac{{\sqrt 3 }}{4}x + \frac{{3\sqrt 3 }}{4}} \right)\,\,\,(y = 0.433x + 1.30)\)
[6 marks]
Use of \(V = \pi \int\limits_0^{\frac{{\sqrt 7 }}{2}} {{y^2}{\text{d}}x} \)
\(V = \pi \int\limits_0^{\frac{{\sqrt 7 }}{2}} {\left( {7 – 4{x^2}} \right){\text{d}}x} \) (M1)(A1)
Note: Condone absence of limits or incorrect limits for M mark.
Do not condone absence of or multiples of \(\pi \).
\( = 19.4\,\,\,\left( { = \frac{{7\sqrt 7 \pi }}{3}} \right)\) A1
[3 marks]