Question
A curve has equation \(\arctan {x^2} + \arctan {y^2} = \frac{\pi }{4}\).
(a) Find \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) in terms of x and y.
(b) Find the gradient of the curve at the point where \(x = \frac{1}{{\sqrt 2 }}\) and \(y < 0\).
▶️Answer/Explanation
Markscheme
(a) METHOD 1
\(\frac{{2x}}{{1 + {x^4}}} + \frac{{2y}}{{1 + {y^4}}}\frac{{{\text{d}}y}}{{{\text{d}}x}} = 0\) M1A1A1
Note: Award M1 for implicit differentiation, A1 for LHS and A1 for RHS.
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – \frac{{x\left( {1 + {y^4}} \right)}}{{y\left( {1 + {x^4}} \right)}}\) A1
METHOD 2
\({y^2} = \tan \left( {\frac{\pi }{4} – \arctan {x^2}} \right)\)
\( = \frac{{\tan \frac{\pi }{4} – \tan \left( {\arctan {x^2}} \right)}}{{1 + \left( {\tan \frac{\pi }{4}} \right)\left( {\tan \left( {\arctan {x^2}} \right)} \right)}}\) (M1)
\( = \frac{{1 – {x^2}}}{{1 + {x^2}}}\) A1
\(2y\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{ – 2x\left( {1 + {x^2}} \right) – 2x\left( {1 – {x^2}} \right)}}{{{{\left( {1 + {x^2}} \right)}^2}}}\) M1
\(2y\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{ – 4x}}{{{{\left( {1 + {x^2}} \right)}^2}}}\)
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – \frac{{2x}}{{y{{\left( {1 + {x^2}} \right)}^2}}}\) A1
\(\left( { = \frac{{2x\sqrt {1 + {x^2}} }}{{\sqrt {1 – {x^2}} {{\left( {1 + {x^2}} \right)}^2}}}} \right)\)
[4 marks]
(b) \({y^2} = \tan \left( {\frac{\pi }{4} – \arctan \frac{1}{2}} \right)\) (M1)
\( = \frac{{\tan \frac{\pi }{4} – \tan \left( {\arctan \frac{1}{2}} \right)}}{{1 + \left( {\tan \frac{\pi }{4}} \right)\left( {\tan \left( {\arctan \frac{1}{2}} \right)} \right)}}\) (M1)
Note: The two M1s may be awarded for working in part (a).
\( = \frac{{1 – \frac{1}{2}}}{{1 + \frac{1}{2}}} = \frac{1}{3}\) A1
\(y = – \frac{1}{{\sqrt 3 }}\) A1
substitution into \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\)
\( = \frac{{4\sqrt 6 }}{9}\) A1
Note: Accept \(\frac{{8\sqrt 3 }}{{9\sqrt 2 }}\) etc.
[5 marks]
Total [9 marks]
Examiners report
Question
Consider the function \(f(x) = \frac{{\ln x}}{x}\) , \(0 < x < {{\text{e}}^2}\) .
(i) Solve the equation \(f'(x) = 0\) .
(ii) Hence show the graph of \(f\) has a local maximum.
(iii) Write down the range of the function \(f\) .
Show that there is a point of inflexion on the graph and determine its coordinates.
Sketch the graph of \(y = f(x)\) , indicating clearly the asymptote, x-intercept and the local maximum.
Now consider the functions \(g(x) = \frac{{\ln \left| x \right|}}{x}\) and \(h(x) = \frac{{\ln \left| x \right|}}{{\left| x \right|}}\) , where \(0 < x < {{\text{e}}^2}\) .
(i) Sketch the graph of \(y = g(x)\) .
(ii) Write down the range of \(g\) .
(iii) Find the values of \(x\) such that \(h(x) > g(x)\) .
▶️Answer/Explanation
Markscheme
(i) \(f'(x) = \frac{{x\frac{1}{x} – \ln x}}{{{x^2}}}\) M1A1
\( = \frac{{1 – \ln x}}{{{x^2}}}\)
so \(f'(x) = 0\) when \(\ln x = 1\), i.e. \(x = {\text{e}}\) A1
(ii) \(f'(x) > 0\) when \(x < {\text{e}}\) and \(f'(x) < 0\) when \(x > {\text{e}}\) R1
hence local maximum AG
Note: Accept argument using correct second derivative.
(iii) \(y \leqslant \frac{1}{{\text{e}}}\) A1
[5 marks]
\(f”(x) = \frac{{{x^2}\frac{{ – 1}}{x} – \left( {1 – \ln x} \right)2x}}{{{x^4}}}\) M1
\( = \frac{{ – x – 2x + 2x\ln x}}{{{x^4}}}\)
\( = \frac{{ – 3 + 2\ln x}}{{{x^3}}}\) A1
Note: May be seen in part (a).
\(f”(x) = 0\) (M1)
\({ – 3 + 2\ln x = 0}\)
\(x = {{\text{e}}^{\frac{3}{2}}}\)
since \(f”(x) < 0\) when \(x < {{\text{e}}^{\frac{3}{2}}}\) and \(f”(x) > 0\) when \(x > {{\text{e}}^{\frac{3}{2}}}\) R1
then point of inflexion \(\left( {{{\text{e}}^{\frac{3}{2}}},\frac{3}{{2{{\text{e}}^{\frac{3}{2}}}}}} \right)\) A1
[5 marks]
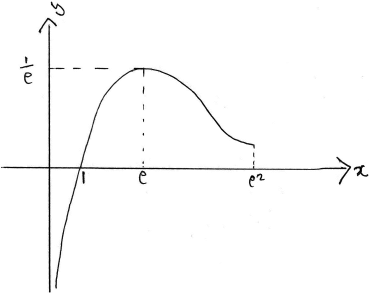 A1A1A1
A1A1A1
Note: Award A1 for the maximum and intercept, A1 for a vertical asymptote and A1 for shape (including turning concave up).
[3 marks]
(i)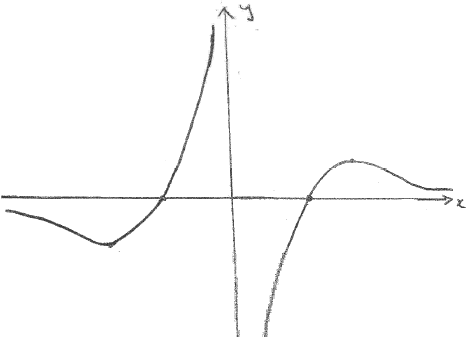 A1A1
A1A1
Note: Award A1 for each correct branch.
(ii) all real values A1
(iii)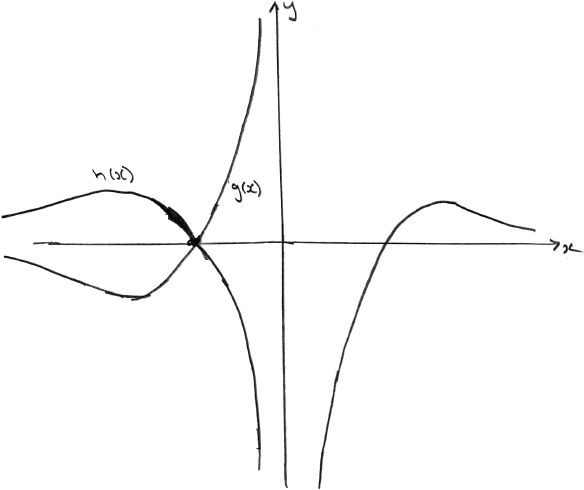 (M1)(A1)
(M1)(A1)
Note: Award (M1)(A1) for sketching the graph of h, ignoring any graph of g.
\( – {{\text{e}}^2} < x < – 1\) (accept \(x < – 1\) ) A1
[6 marks]
Examiners report
Most candidates attempted parts (a), (b) and (c) and scored well, although many did not gain the reasoning marks for the justification of the existence of local maximum and inflexion point. The graph sketching was poorly done. A wide selection of range shapes were seen, in some cases showing little understanding of the relation between the derivatives of the function and its graph and difficulties with transformation of graphs. In some cases candidates sketched graphs consistent with their previous calculations but failed to label them properly.
Most candidates attempted parts (a), (b) and (c) and scored well, although many did not gain the reasoning marks for the justification of the existence of local maximum and inflexion point. The graph sketching was poorly done. A wide selection of range shapes were seen, in some cases showing little understanding of the relation between the derivatives of the function and its graph and difficulties with transformation of graphs. In some cases candidates sketched graphs consistent with their previous calculations but failed to label them properly.
Most candidates attempted parts (a), (b) and (c) and scored well, although many did not gain the reasoning marks for the justification of the existence of local maximum and inflexion point. The graph sketching was poorly done. A wide selection of range shapes were seen, in some cases showing little understanding of the relation between the derivatives of the function and its graph and difficulties with transformation of graphs. In some cases candidates sketched graphs consistent with their previous calculations but failed to label them properly.
Most candidates attempted parts (a), (b) and (c) and scored well, although many did not gain the reasoning marks for the justification of the existence of local maximum and inflexion point. The graph sketching was poorly done. A wide selection of range shapes were seen, in some cases showing little understanding of the relation between the derivatives of the function and its graph and difficulties with transformation of graphs. In some cases candidates sketched graphs consistent with their previous calculations but failed to label them properly.
Question
The diagram below shows a circular lake with centre O, diameter AB and radius 2 km.

Jorg needs to get from A to B as quickly as possible. He considers rowing to point P and then walking to point B. He can row at \(3{\text{ km}}\,{{\text{h}}^{ – 1}}\) and walk at \(6{\text{ km}}\,{{\text{h}}^{ – 1}}\). Let \({\rm{P\hat AB}} = \theta \) radians, and t be the time in hours taken by Jorg to travel from A to B.
Show that \(t = \frac{2}{3}(2\cos \theta + \theta )\).
Find the value of \(\theta \) for which \(\frac{{{\text{d}}t}}{{{\text{d}}\theta }} = 0\).
What route should Jorg take to travel from A to B in the least amount of time?
Give reasons for your answer.
▶️Answer/Explanation
Markscheme
angle APB is a right angle
\( \Rightarrow \cos \theta = \frac{{{\text{AP}}}}{4} \Rightarrow {\text{AP}} = 4\cos \theta \) A1
Note: Allow correct use of cosine rule.
\({\text{arc PB}} = 2 \times 2\theta = 4\theta \) A1
\(t = \frac{{{\text{AP}}}}{3} + \frac{{{\text{PB}}}}{6}\) M1
Note: Allow use of their AP and their PB for the M1.
\( \Rightarrow t = \frac{{4\cos \theta }}{3} + \frac{{4\theta }}{6} = \frac{{4\cos \theta }}{3} + \frac{{2\theta }}{3} = \frac{2}{3}(2\cos \theta + \theta )\) AG
[3 marks]
\(\frac{{{\text{d}}t}}{{{\text{d}}\theta }} = \frac{2}{3}( – 2\sin \theta + 1)\) A1
\(\frac{2}{3}( – 2\sin \theta + 1) = 0 \Rightarrow \sin \theta = \frac{1}{2} \Rightarrow \theta = \frac{\pi }{6}\) (or 30 degrees) A1
[2 marks]
\(\frac{{{{\text{d}}^2}t}}{{{\text{d}}{\theta ^2}}} = – \frac{4}{3}\cos \theta < 0\,\,\,\,\left( {{\text{at }}\theta = \frac{\pi }{6}} \right)\) M1
\( \Rightarrow t\) is maximized at \(\theta = \frac{\pi }{6}\) R1
time needed to walk along arc AB is \(\frac{{2\pi }}{6}{\text{ (}} \approx {\text{1 hour)}}\)
time needed to row from A to B is \(\frac{4}{3}{\text{ (}} \approx {\text{1.33 hour)}}\)
hence, time is minimized in walking from A to B R1
[3 marks]
Examiners report
The fairly easy trigonometry challenged a large number of candidates.
Part (b) was very well done.
Satisfactory answers were very rarely seen for (c). Very few candidates realised that a minimum can occur at the beginning or end of an interval.
Question
Let \(f(x) = \sqrt {\frac{x}{{1 – x}}} ,{\text{ }}0 < x < 1\).
Show that \(f'(x) = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}\) and deduce that f is an increasing function.
Show that the curve \(y = f(x)\) has one point of inflexion, and find its coordinates.
Use the substitution \(x = {\sin ^2}\theta \) to show that \(\int {f(x){\text{d}}x} = \arcsin \sqrt x – \sqrt {x – {x^2}} + c\) .
▶️Answer/Explanation
Markscheme
EITHER
derivative of \(\frac{x}{{1 – x}}\) is \(\frac{{(1 – x) – x( – 1)}}{{{{(1 – x)}^2}}}\) M1A1
\(f'(x) = \frac{1}{2}{\left( {\frac{x}{{1 – x}}} \right)^{ – \frac{1}{2}}}\frac{1}{{{{(1 – x)}^2}}}\) M1A1
\( = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}\) AG
\(f'(x) > 0\) (for all \(0 < x < 1\)) so the function is increasing R1
OR
\(f(x) = \frac{{{x^{\frac{1}{2}}}}}{{{{(1 – x)}^{\frac{1}{2}}}}}\)
\(f'(x) = \frac{{{{(1 – x)}^{\frac{1}{2}}}\left( {\frac{1}{2}{x^{ – \frac{1}{2}}}} \right) – \frac{1}{2}{x^{\frac{1}{2}}}{{(1 – x)}^{ – \frac{1}{2}}}( – 1)}}{{1 – x}}\) M1A1
\( = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{1}{2}}} + \frac{1}{2}{x^{\frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}\) A1
\( = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}[1 – x + x]\) M1
\( = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}\) AG
\(f'(x) > 0\) (for all \(0 < x < 1\)) so the function is increasing R1
[5 marks]
\(f'(x) = \frac{1}{2}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{3}{2}}}\)
\( \Rightarrow f”(x) = -\frac{1}{4}{x^{ – \frac{3}{2}}}{(1 – x)^{ – \frac{3}{2}}} + \frac{3}{4}{x^{ – \frac{1}{2}}}{(1 – x)^{ – \frac{5}{2}}}\) M1A1
\( = -\frac{1}{4}{x^{ – \frac{3}{2}}}{(1 – x)^{ – \frac{5}{2}}}[1 – 4x]\)
\(f”(x) = 0 \Rightarrow x = \frac{1}{4}\) M1A1
\(f”(x)\) changes sign at \(x = \frac{1}{4}\) hence there is a point of inflexion R1
\(x = \frac{1}{4} \Rightarrow y = \frac{1}{{\sqrt 3 }}\) A1
the coordinates are \(\left( {\frac{1}{4},\frac{1}{{\sqrt 3 }}} \right)\)
[6 marks]
\(x = {\sin ^2}\theta \Rightarrow \frac{{{\text{d}}x}}{{{\text{d}}\theta }} = 2\sin \theta \cos \theta \) M1A1
\(\int {\sqrt {\frac{x}{{1 – x}}} {\text{d}}x = \int {\sqrt {\frac{{{{\sin }^2}\theta }}{{1 – {{\sin }^2}\theta }}} 2\sin \theta \cos \theta {\text{d}}\theta } } \) M1A1
\( = \int {2{{\sin }^2}\theta {\text{d}}\theta } \) A1
\( = \int {1 – \cos 2\theta } {\text{d}}\theta \) M1A1
\( = \theta – \frac{1}{2}\sin 2\theta + c\) A1
\(\theta = \arcsin \sqrt x \) A1
\(\frac{1}{2}\sin 2\theta = \sin \theta \cos \theta = \sqrt x \sqrt {1 – x} = \sqrt {x – {x^2}} \) M1A1
hence \(\int {\sqrt {\frac{x}{{1 – x}}} {\text{d}}x = \arcsin \sqrt x } – \sqrt {x – {x^2}} + c\) AG
[11 marks]
Examiners report
Part (a) was generally well done, although few candidates made the final deduction asked for. Those that lost other marks in this part were generally due to mistakes in algebraic manipulation. In part (b) whilst many students found the second derivative and set it equal to zero, few then confirmed that it was a point of inflexion. There were several good attempts for part (c), even though there were various points throughout the question that provided stopping points for other candidates.
Part (a) was generally well done, although few candidates made the final deduction asked for. Those that lost other marks in this part were generally due to mistakes in algebraic manipulation. In part (b) whilst many students found the second derivative and set it equal to zero, few then confirmed that it was a point of inflexion. There were several good attempts for part (c), even though there were various points throughout the question that provided stopping points for other candidates.
Part (a) was generally well done, although few candidates made the final deduction asked for. Those that lost other marks in this part were generally due to mistakes in algebraic manipulation. In part (b) whilst many students found the second derivative and set it equal to zero, few then confirmed that it was a point of inflexion. There were several good attempts for part (c), even though there were various points throughout the question that provided stopping points for other candidates.
Question
The function \(f\) is given by \(f(x) = x{{\text{e}}^{ – x}}{\text{ }}(x \geqslant 0)\).
(i) Find an expression for \(f'(x)\).
(ii) Hence determine the coordinates of the point A, where \(f'(x) = 0\).
Find an expression for \(f”(x)\) and hence show the point A is a maximum.
Find the coordinates of B, the point of inflexion.
The graph of the function \(g\) is obtained from the graph of \(f\) by stretching it in the x-direction by a scale factor 2.
(i) Write down an expression for \(g(x)\).
(ii) State the coordinates of the maximum C of \(g\).
(iii) Determine the x-coordinates of D and E, the two points where \(f(x) = g(x)\).
Sketch the graphs of \(y = f(x)\) and \(y = g(x)\) on the same axes, showing clearly the points A, B, C, D and E.
Find an exact value for the area of the region bounded by the curve \(y = g(x)\), the x-axis and the line \(x = 1\).
▶️Answer/Explanation
Markscheme
(i) \(f'(x) = {{\text{e}}^{ – x}} – x{{\text{e}}^{ – x}}\) M1A1
(ii) \(f'(x) = 0 \Rightarrow x = 1\)
coordinates \(\left( {1,{\text{ }}{{\text{e}}^{ – 1}}} \right)\) A1
[3 marks]
\(f”(x) = – {{\text{e}}^{ – x}} – {{\text{e}}^{ – x}} + x{{\text{e}}^{ – x}}{\text{ }}\left( { = – {{\text{e}}^{ – x}}(2 – x)} \right)\) A1
substituting \(x = 1\) into \(f”(x)\) M1
\(f”(1){\text{ }}\left( { = – {{\text{e}}^{ – 1}}} \right) < 0\) hence maximum R1AG
[3 marks]
\(f”(x) = 0{\text{ (}} \Rightarrow x = 2)\) M1
coordinates \(\left( {2,{\text{ 2}}{{\text{e}}^{ – 2}}} \right)\) A1
[2 marks]
(i) \(g(x) = \frac{x}{2}{{\text{e}}^{ – \frac{x}{2}}}\) A1
(ii) coordinates of maximum \(\left( {2,{\text{ }}{{\text{e}}^{ – 1}}} \right)\) A1
(iii) equating \(f(x) = g(x)\) and attempting to solve \(x{{\text{e}}^{ – x}} = \frac{x}{2}{{\text{e}}^{ – \frac{x}{2}}}\)
\( \Rightarrow x\left( {2{{\text{e}}^{\frac{x}{2}}} – {{\text{e}}^x}} \right) = 0\) (A1)
\( \Rightarrow x = 0\) A1
or \(2{{\text{e}}^{\frac{x}{2}}} = {{\text{e}}^x}\)
\( \Rightarrow {{\text{e}}^{\frac{x}{2}}} = 2\)
\( \Rightarrow x = 2\ln 2\) \((\ln 4)\) A1
Note: Award first (A1) only if factorisation seen or if two correct
solutions are seen.
A4
Note: Award A1 for shape of \(f\), including domain extending beyond \(x = 2\).
Ignore any graph shown for \(x < 0\).
Award A1 for A and B correctly identified.
Award A1 for shape of \(g\), including domain extending beyond \(x = 2\).
Ignore any graph shown for \(x < 0\). Allow follow through from \(f\).
Award A1 for C, D and E correctly identified (D and E are interchangeable).
[4 marks]
\(A = \int_0^1 {\frac{x}{2}{{\text{e}}^{ – \frac{x}{2}}}{\text{d}}x} \) M1
\( = \left[ { – x{{\text{e}}^{ – \frac{x}{2}}}} \right]_0^1 – \int_0^1 { – {{\text{e}}^{ – \frac{x}{2}}}{\text{d}}x} \) A1
Note: Condone absence of limits or incorrect limits.
\( = – {{\text{e}}^{ – \frac{1}{2}}} – \left[ {2{{\text{e}}^{ – \frac{x}{2}}}} \right]_0^1\)
\( = – {{\text{e}}^{ – \frac{1}{2}}} – \left( {2{{\text{e}}^{ – \frac{1}{2}}} – 2} \right) = 2 – 3{{\text{e}}^{ – \frac{1}{2}}}\) A1
[3 marks]
Examiners report
Part a) proved to be an easy start for the vast majority of candidates.
Full marks for part b) were again likewise seen, though a small number shied away from considering the sign of their second derivative, despite the question asking them to do so.
Part c) again proved to be an easily earned 2 marks.
Full marks for part b) were again likewise seen, though a small number shied away from considering the sign of their second derivative, despite the question asking them to do so.
Part c) again proved to be an easily earned 2 marks.
Many candidates lost their way in part d). A variety of possibilities for \(g(x)\) were suggested, commonly \(2x{{\text{e}}^{ – 2x}}\), \(\frac{{x{{\text{e}}^{ – 1}}}}{2}\) or similar variations. Despite section ii) being worth only one mark, (and ‘state’ being present in the question), many laborious attempts at further differentiation were seen. Part diii was usually answered well by those who gave the correct function for \(g(x)\).
Part e) was also answered well by those who had earned full marks up to that point.
While the integration by parts technique was clearly understood, it was somewhat surprising how many careless slips were seen in this part of the question. Only a minority gained full marks for part f).
Question
Consider the following functions:
\(h(x) = \arctan (x),{\text{ }}x \in \mathbb{R}\)
\(g(x) = \frac{1}{x}\), \(x\in \mathbb{R}\), \({\text{ }}x \ne 0\)
Sketch the graph of \(y = h(x)\).
Find an expression for the composite function \(h \circ g(x)\) and state its domain.
Given that \(f(x) = h(x) + h \circ g(x)\),
(i) find \(f'(x)\) in simplified form;
(ii) show that \(f(x) = \frac{\pi }{2}\) for \(x > 0\).
Nigel states that \(f\) is an odd function and Tom argues that \(f\) is an even function.
(i) State who is correct and justify your answer.
(ii) Hence find the value of \(f(x)\) for \(x < 0\).
▶️Answer/Explanation
Markscheme
A1A1
Note: A1 for correct shape, A1 for asymptotic behaviour at \(y = \pm \frac{\pi }{2}\).
[2 marks]
\(h \circ g(x) = \arctan \left( {\frac{1}{x}} \right)\) A1
domain of \(h \circ g\) is equal to the domain of \(g:x \in \circ ,{\text{ }}x \ne 0\) A1
[2 marks]
(i) \(f(x) = \arctan (x) + \arctan \left( {\frac{1}{x}} \right)\)
\(f'(x) = \frac{1}{{1 + {x^2}}} + \frac{1}{{1 + \frac{1}{{{x^2}}}}} \times – \frac{1}{{{x^2}}}\) M1A1
\(f'(x) = \frac{1}{{1 + {x^2}}} + \frac{{ – \frac{1}{{{x^2}}}}}{{\frac{{{x^2} + 1}}{{{x^2}}}}}\) (A1)
\( = \frac{1}{{1 + {x^2}}} – \frac{1}{{1 + {x^2}}}\)
\( = 0\) A1
(ii) METHOD 1
f is a constant R1
when \(x > 0\)
\(f(1) = \frac{\pi }{4} + \frac{\pi }{4}\) M1A1
\( = \frac{\pi }{2}\) AG
METHOD 2
from diagram
\(\theta = \arctan \frac{1}{x}\) A1
\(\alpha = \arctan x\) A1
\(\theta + \alpha = \frac{\pi }{2}\) R1
hence \(f(x) = \frac{\pi }{2}\) AG
METHOD 3
\(\tan \left( {f(x)} \right) = \tan \left( {\arctan (x) + \arctan \left( {\frac{1}{x}} \right)} \right)\) M1
\( = \frac{{x + \frac{1}{x}}}{{1 – x\left( {\frac{1}{x}} \right)}}\) A1
denominator = 0, so \(f(x) = \frac{\pi }{2}{\text{ (for }}x > 0)\) R1
[7 marks]
(i) Nigel is correct. A1
METHOD 1
\(\arctan (x)\) is an odd function and \(\frac{1}{x}\) is an odd function
composition of two odd functions is an odd function and sum of two odd functions is an odd function R1
METHOD 2
\(f( – x) = \arctan ( – x) + \arctan \left( { – \frac{1}{x}} \right) = – \arctan (x) – \arctan \left( {\frac{1}{x}} \right) = – f(x)\)
therefore f is an odd function. R1
(ii) \(f(x) = – \frac{\pi }{2}\) A1
[3 marks]
Question
Let \(y(x) = x{e^{3x}},{\text{ }}x \in \mathbb{R}\).
Find \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\).
Prove by induction that \(\frac{{{{\text{d}}^n}y}}{{{\text{d}}{x^n}}} = n{3^{n – 1}}{{\text{e}}^{3x}} + x{3^n}{{\text{e}}^{3x}}\) for \(n \in {\mathbb{Z}^ + }\).
Find the coordinates of any local maximum and minimum points on the graph of \(y(x)\).
Justify whether any such point is a maximum or a minimum.
Find the coordinates of any points of inflexion on the graph of \(y(x)\). Justify whether any such point is a point of inflexion.
Hence sketch the graph of \(y(x)\), indicating clearly the points found in parts (c) and (d) and any intercepts with the axes.
▶️Answer/Explanation
Markscheme
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = 1 \times {{\text{e}}^{3x}} + x \times 3{{\text{e}}^{3x}} = ({{\text{e}}^{3x}} + 3x{{\text{e}}^{3x}})\) M1A1
[2 marks]
let \(P(n)\) be the statement \(\frac{{{{\text{d}}^n}y}}{{{\text{d}}{x^n}}} = n{3^{n – 1}}{{\text{e}}^{3x}} + x{3^n}{{\text{e}}^{3x}}\)
prove for \(n = 1\) M1
\(LHS\) of \(P(1)\) is \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) which is \(1 \times {{\text{e}}^{3x}} + x \times 3{{\text{e}}^{3x}}\) and \(RHS\) is \({3^0}{{\text{e}}^{3x}} + x{3^1}{{\text{e}}^{3x}}\) R1
as \({\text{LHS}} = {\text{RHS, }}P(1)\) is true
assume \(P(k)\) is true and attempt to prove \(P(k + 1)\) is true M1
assuming \(\frac{{{{\text{d}}^k}y}}{{{\text{d}}{x^k}}} = k{3^{k – 1}}{{\text{e}}^{3x}} + x{3^k}{{\text{e}}^{3x}}\)
\(\frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = \frac{{\text{d}}}{{{\text{d}}x}}\left( {\frac{{{{\text{d}}^k}y}}{{{\text{d}}{x^k}}}} \right)\) (M1)
\( = k{3^{k – 1}} \times 3{{\text{e}}^{3x}} + 1 \times {3^k}{{\text{e}}^{3x}} + x{3^k} \times 3{{\text{e}}^{3x}}\) A1
\( = (k + 1){3^k}{{\text{e}}^{3x}} + x{3^{k + 1}}{{\text{e}}^{3x}}\;\;\;\)(as required) A1
Note: Can award the A marks independent of the M marks
since \(P(1)\) is true and \(P(k)\) is true \( \Rightarrow P(k + 1)\) is true
then (by \(PMI\)), \(P(n)\) is true \((\forall n \in {\mathbb{Z}^ + })\) R1
Note: To gain last R1 at least four of the above marks must have been gained.
[7 marks]
\({{\text{e}}^{3x}} + x \times 3{{\text{e}}^{3x}} = 0 \Rightarrow 1 + 3x = 0 \Rightarrow x = – \frac{1}{3}\) M1A1
point is \(\left( { – \frac{1}{3},{\text{ }} – \frac{1}{{3{\text{e}}}}} \right)\) A1
EITHER
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2 \times 3{{\text{e}}^{3x}} + x \times {3^2}{{\text{e}}^{3x}}\)
when \(x = – \frac{1}{3},{\text{ }}\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} > 0\) therefore the point is a minimum M1A1
OR

nature table shows point is a minimum M1A1
[5 marks]
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2 \times 3{{\text{e}}^{3x}} + x \times {3^2}{{\text{e}}^{3x}}\) A1
\(2 \times 3{{\text{e}}^{3x}} + x \times {3^2}{{\text{e}}^{3x}} = 0 \Rightarrow 2 + 3x = 0 \Rightarrow x = – \frac{2}{3}\) M1A1
point is \(\left( { – \frac{2}{3},{\text{ }} – \frac{2}{{3{{\text{e}}^2}}}} \right)\) A1
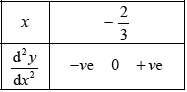
since the curvature does change (concave down to concave up) it is a point of inflection R1
Note: Allow \({3^{{\text{rd}}}}\) derivative is not zero at \( – \frac{2}{3}\)
[5 marks]
(general shape including asymptote and through origin) A1
showing minimum and point of inflection A1
Note: Only indication of position of answers to (c) and (d) required, not coordinates.
[2 marks]
Total [21 marks]
Examiners report
Well done.
The logic of an induction proof was not known well enough. Many candidates used what they had to prove rather than differentiating what they had assumed. They did not have enough experience in doing Induction proofs.
Good, some forgot to test for min/max, some forgot to give the \(y\) value.
Again quite good, some forgot to check for change in curvature and some forgot the \(y\) value.
Some accurate sketches, some had all the information from earlier parts but could not apply it. The asymptote was often missed.
Question
Consider the functions \(f(x) = \tan x,{\text{ }}0 \le \ x < \frac{\pi }{2}\) and \(g(x) = \frac{{x + 1}}{{x – 1}},{\text{ }}x \in \mathbb{R},{\text{ }}x \ne 1\).
Find an expression for \(g \circ f(x)\), stating its domain.
Hence show that \(g \circ f(x) = \frac{{\sin x + \cos x}}{{\sin x – \cos x}}\).
Let \(y = g \circ f(x)\), find an exact value for \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) at the point on the graph of \(y = g \circ f(x)\) where \(x = \frac{\pi }{6}\), expressing your answer in the form \(a + b\sqrt 3 ,{\text{ }}a,{\text{ }}b \in \mathbb{Z}\).
Show that the area bounded by the graph of \(y = g \circ f(x)\), the \(x\)-axis and the lines \(x = 0\) and \(x = \frac{\pi }{6}\) is \(\ln \left( {1 + \sqrt 3 } \right)\).
▶️Answer/Explanation
Markscheme
\(g \circ f(x) = \frac{{\tan x + 1}}{{\tan x – 1}}\) A1
\(x \ne \frac{\pi }{4},{\text{ }}0 \le x < \frac{\pi }{2}\) A1
[2 marks]
\(\frac{{\tan x + 1}}{{\tan x – 1}} = \frac{{\frac{{\sin x}}{{\cos x}} + 1}}{{\frac{{\sin x}}{{\cos x}} – 1}}\) M1A1
\( = \frac{{\sin x + \cos x}}{{\sin x – \cos x}}\) AG
[2 marks]
METHOD 1
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{(\sin x – \cos x)(\cos x – \sin x) – (\sin x + \cos x)(\cos x + \sin x)}}{{{{(\sin x – \cos x)}^2}}}\) M1(A1)
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{(2\sin x\cos x – {{\cos }^2}x – {{\sin }^2}x) – (2\sin x\cos x + {{\cos }^2}x + {{\sin }^2}x)}}{{{{\cos }^2}x + {{\sin }^2}x – 2\sin x\cos x}}\)
\( = \frac{{ – 2}}{{1 – \sin 2x}}\)
Substitute \(\frac{\pi }{6}\) into any formula for \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) M1
\(\frac{{ – 2}}{{1 – \sin \frac{\pi }{3}}}\)
\( = \frac{{ – 2}}{{1 – \frac{{\sqrt 3 }}{2}}}\) A1
\( = \frac{{ – 4}}{{2 – \sqrt 3 }}\)
\( = \frac{{ – 4}}{{2 – \sqrt 3 }}\left( {\frac{{2 + \sqrt 3 }}{{2 + \sqrt 3 }}} \right)\) M1
\( = \frac{{ – 8 – 4\sqrt 3 }}{1} = – 8 – 4\sqrt 3 \) A1
METHOD 2
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{(\tan x – 1){{\sec }^2}x – (\tan x + 1){{\sec }^2}x}}{{{{(\tan x – 1)}^2}}}\) M1A1
\( = \frac{{ – 2{{\sec }^2}x}}{{{{(\tan x – 1)}^2}}}\) A1
\( = \frac{{ – 2{{\sec }^2}\frac{\pi }{6}}}{{{{\left( {\tan \frac{\pi }{6} – 1} \right)}^2}}} = \frac{{ – 2\left( {\frac{4}{3}} \right)}}{{{{\left( {\frac{1}{{\sqrt 3 }} – 1} \right)}^2}}} = \frac{{ – 8}}{{{{\left( {1 – \sqrt 3 } \right)}^2}}}\) M1
Note: Award M1 for substitution \(\frac{\pi }{6}\).
\(\frac{{ – 8}}{{{{\left( {1 – \sqrt 3 } \right)}^2}}} = \frac{{ – 8}}{{\left( {4 – 2\sqrt 3 } \right)}}\frac{{\left( {4 + 2\sqrt 3 } \right)}}{{\left( {4 + 2\sqrt 3 } \right)}} = – 8 – 4\sqrt 3 \) M1A1
[6 marks]
Area \(\left| {\int_0^{\frac{\pi }{6}} {\frac{{\sin x + \cos x}}{{\sin x – \cos x}}{\text{d}}x} } \right|\) M1
\( = \left| {\left[ {\ln \left| {\sin x – \cos x} \right|} \right]_0^{\frac{\pi }{6}}} \right|\) A1
Note: Condone absence of limits and absence of modulus signs at this stage.
\( = \left| {\ln \left| {\sin \frac{\pi }{6} – \cos \frac{\pi }{6}} \right| – \ln \left| {\sin 0 – \cos 0} \right|} \right|\) M1
\( = \left| {\ln \left| {\frac{1}{2} – \frac{{\sqrt 3 }}{2}} \right| – 0} \right|\)
\( = \left| {\ln \left( {\frac{{\sqrt 3 – 1}}{2}} \right)} \right|\) A1
\( = – \ln \left( {\frac{{\sqrt 3 – 1}}{2}} \right) = \ln \left( {\frac{2}{{\sqrt 3 – 1}}} \right)\) A1
\( = \ln \left( {\frac{2}{{\sqrt 3 – 1}} \times \frac{{\sqrt 3 + 1}}{{\sqrt 3 + 1}}} \right)\) M1
\( = \ln \left( {\sqrt 3 + 1} \right)\) AG
[6 marks]
Total [16 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider the curve \(y = \frac{1}{{1 – x}},{\text{ }}x \in \mathbb{R},{\text{ }}x \ne 1\).
Find \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\).
Determine the equation of the normal to the curve at the point \(x = 3\) in the form \(ax + by + c = 0\) where \(a,{\text{ }}b,{\text{ }}c \in \mathbb{Z}\).
▶️Answer/Explanation
Markscheme
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {(1 – x)^{ – 2}}\;\;\;\left( { = \frac{1}{{{{(1 – x)}^2}}}} \right)\) (M1)A1
[2 marks]
gradient of Tangent \( = \frac{1}{4}\) (A1)
gradient of Normal \( = – 4\) (M1)
\(y + \frac{1}{2} = – 4(x – 3)\) or attempt to find \(c\) in \(y = mx + c\) M1
\(8x + 2y – 23 = 0\) A1
[4 marks]
Total [6 marks]
Examiners report
[N/A]
[N/A]
Question
Show that \(\sin \left( {\theta + \frac{\pi }{2}} \right) = \cos \theta \).
Consider \(f(x) = \sin (ax)\) where \(a\) is a constant. Prove by mathematical induction that \({f^{(n)}}(x) = {a^n}\sin \left( {ax + \frac{{n\pi }}{2}} \right)\) where \(n \in {\mathbb{Z}^ + }\) and \({f^{(n)}}(x)\) represents the \({{\text{n}}^{{\text{th}}}}\) derivative of \(f(x)\).
▶️Answer/Explanation
Markscheme
\(\sin \left( {\theta + \frac{\pi }{2}} \right) = \sin \theta \cos \frac{\pi }{2} + \cos \theta \sin \frac{\pi }{2}\) M1
\( = \cos \theta \) AG
Note: Accept a transformation/graphical based approach.
[1 mark]
consider \(n = 1,{\text{ }}f'(x) = a\cos (ax)\) M1
since \(\sin \left( {ax + \frac{\pi }{2}} \right) = \cos ax\) then the proposition is true for \(n = 1\) R1
assume that the proposition is true for \(n = k\) so \({f^{(k)}}(x) = {a^k}\sin \left( {ax + \frac{{k\pi }}{2}} \right)\) M1
\({f^{(k + 1)}}(x) = \frac{{{\text{d}}\left( {{f^{(k)}}(x)} \right)}}{{{\text{d}}x}}\;\;\;\left( { = a\left( {{a^k}\cos \left( {ax + \frac{{k\pi }}{2}} \right)} \right)} \right)\) M1
\( = {a^{k + 1}}\sin \left( {ax + \frac{{k\pi }}{2} + \frac{\pi }{2}} \right)\) (using part (a)) A1
\( = {a^{k + 1}}\sin \left( {ax + \frac{{(k + 1)\pi }}{2}} \right)\) A1
given that the proposition is true for \(n = k\) then we have shown that the proposition is true for \(n = k + 1\). Since we have shown that the proposition is true for \(n = 1\) then the proposition is true for all \(n \in {\mathbb{Z}^ + }\) R1
Note: Award final R1 only if all prior M and R marks have been awarded.
[7 marks]
Total [8 marks]
Examiners report
[N/A]
[N/A]
Question
The function f is defined on the domain \(x \geqslant 0\) by \(f(x) = {{\text{e}}^x} – {x^{\text{e}}}\) .
(i) Find an expression for \(f'(x)\) .
(ii) Given that the equation \(f'(x) = 0\) has two roots, state their values.
Sketch the graph of f , showing clearly the coordinates of the maximum and minimum.
Hence show that \({{\text{e}}^\pi } > {\pi ^{\text{e}}}\) .
▶️Answer/Explanation
Markscheme
(i) \(f'(x) = {{\text{e}}^x} – {\text{e}}{x^{{\text{e}} – 1}}\) A1
(ii) by inspection the two roots are 1, e A1A1
[3 marks]
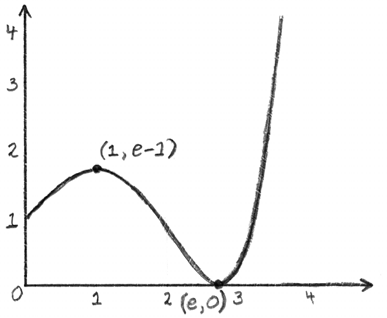 A3
A3
Note: Award A1 for maximum, A1 for minimum and A1 for general shape.
[3 marks]
from the graph: \({{\text{e}}^x} > {x^{\text{e}}}\) for all \(x > 0\) except x = e R1
putting \(x = \pi \) , conclude that \({{\text{e}}^\pi } > {\pi ^{\text{e}}}\) AG
[1 mark]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The function f is defined by \(f(x) = {{\text{e}}^x}\sin x\) .
Show that \(f”(x) = 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right)\) .
Obtain a similar expression for \({f^{(4)}}(x)\) .
Suggest an expression for \({f^{(2n)}}(x)\), \(n \in {\mathbb{Z}^ + }\), and prove your conjecture using mathematical induction.
▶️Answer/Explanation
Markscheme
\(f'(x) = {{\text{e}}^x}\sin x + {{\text{e}}^x}\cos x\) A1
\(f”(x) = {{\text{e}}^x}\sin x + {{\text{e}}^x}\cos x + {{\text{e}}^x}\cos x – {{\text{e}}^x}\sin x\) A1
\( = 2{{\text{e}}^x}\cos x\) A1
\( = 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right)\) AG
[3 marks]
\(f”'(x) = 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right) + 2{{\text{e}}^x}\cos \left( {x + \frac{\pi }{2}} \right)\) A1
\({f^{(4)}}(x) = 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right) + 2{{\text{e}}^x}\cos \left( {x + \frac{\pi }{2}} \right) + 2{{\text{e}}^x}\cos \left( {x + \frac{\pi }{2}} \right) – 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right)\) A1
\( = 4{{\text{e}}^x}\cos \left( {x + \frac{\pi }{2}} \right)\) A1
\( = 4{{\text{e}}^x}\sin (x + \pi )\) A1
[4 marks]
the conjecture is that
\({f^{(2n)}}(x) = {2^n}{{\text{e}}^x}\sin \left( {x + \frac{{n\pi }}{2}} \right)\) A1
for n = 1, this formula gives
\(f”(x) = 2{{\text{e}}^x}\sin \left( {x + \frac{\pi }{2}} \right)\) which is correct A1
let the result be true for n = k , \(\left( {i.e.{\text{ }}{f^{(2k)}}(x) = {2^k}{{\text{e}}^x}\sin \left( {x + \frac{{k\pi }}{2}} \right)} \right)\) M1
consider \({f^{(2k + 1)}}(x) = {2^k}{{\text{e}}^x}\sin \left( {x + \frac{{k\pi }}{2}} \right) + {2^k}{{\text{e}}^x}\cos \left( {x + \frac{{k\pi }}{2}} \right)\) M1
\({f^{\left( {2(k + 1)} \right)}}(x) = {2^k}{{\text{e}}^x}\sin \left( {x + \frac{{k\pi }}{2}} \right) + {2^k}{{\text{e}}^x}\cos \left( {x + \frac{{k\pi }}{2}} \right) + {2^k}{{\text{e}}^x}\cos \left( {x + \frac{{k\pi }}{2}} \right) – {2^k}{{\text{e}}^x}\sin \left( {x + \frac{{k\pi }}{2}} \right)\) A1
\( = {2^{k + 1}}{{\text{e}}^x}\cos \left( {x + \frac{{k\pi }}{2}} \right)\) A1
\( = {2^{k + 1}}{{\text{e}}^x}\sin \left( {x + \frac{{(k + 1)\pi }}{2}} \right)\) A1
therefore true for \(n = k \Rightarrow \) true for \(n = k + 1\) and since true for \(n = 1\)
the result is proved by induction. R1
Note: Award the final R1 only if the two M marks have been awarded.
[8 marks]
Examiners report
[N/A]
[N/A]
[N/A]
Question
The function f is defined by
\[f(x) = \left\{ {\begin{array}{*{20}{c}}
{2x – 1,}&{x \leqslant 2} \\
{a{x^2} + bx – 5,}&{2 < x < 3}
\end{array}} \right.\]
where a , \(b \in \mathbb{R}\) .
Given that f and its derivative, \(f’\) , are continuous for all values in the domain of f , find the values of a and b .
Show that f is a one-to-one function.
Obtain expressions for the inverse function \({f^{ – 1}}\) and state their domains.
▶️Answer/Explanation
Markscheme
f continuous \( \Rightarrow \mathop {\lim }\limits_{x \to {2^ – }} f(x) = \mathop {\lim }\limits_{x \to {2^ \div }} f(x)\) M1
\(4a + 2b = 8\) A1
\(f'(x) = \left\{ {\begin{array}{*{20}{c}}
{2,}&{x < 2} \\
{2ax + b,}&{2 < x < 3}
\end{array}} \right.\) A1
\(f'{\text{ continuous}} \Rightarrow \mathop {\lim }\limits_{x \to {2^ – }} f'(x) = \mathop {\lim }\limits_{x \to {2^ \div }} f'(x)\)
\(4a + b = 2\) A1
solve simultaneously M1
to obtain a = –1 and b = 6 A1
[6 marks]
for \(x \leqslant 2,{\text{ }}f'(x) = 2 > 0\) A1
for \(2 < x < 3,{\text{ }}f'(x) = – 2x + 6 > 0\) A1
since \(f'(x) > 0\) for all values in the domain of f , f is increasing R1
therefore one-to-one AG
[3 marks]
\(x = 2y – 1 \Rightarrow y = \frac{{x + 1}}{2}\) M1
\(x = – {y^2} + 6y – 5 \Rightarrow {y^2} – 6y + x + 5 = 0\) M1
\(y = 3 \pm \sqrt {4 – x} \)
therefore
\({f^{ – 1}}(x) = \left\{ {\begin{array}{*{20}{c}}
{\frac{{x + 1}}{2},}&{x \leqslant 3} \\
{3 – \sqrt {4 – x} ,}&{3 < x < 4}
\end{array}} \right.\) A1A1A1
Note: Award A1 for the first line and A1A1 for the second line.
[5 marks]
Examiners report
[N/A]
[N/A]
[N/A]
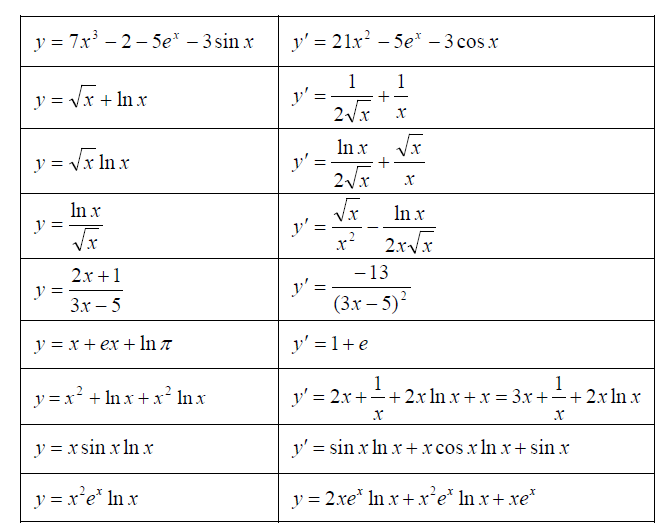
[MAI 5.6-5.7] RULES OF DIFFERENTIATION-lala
Question
[Maximum mark: 3 per function]
Differentiate the following functions:

▶️Answer/Explanation
Ans.
Question
[Maximum mark: 4]
Let \(f(x)=2x^{3}+ln\;x\)
(a) Find \({f}'(x)\)
(b) Find the gradient of the curve \(y = f (x)\) at \(x = 1\).
▶️Answer/Explanation
Ans.
(a)\({f}'(x)=6x^{2}+\frac{1}{x}\) (b)\({f}'(1)=6+1=7\)
Question
[Maximum mark: 6]
Let \(f(x)=\frac{x^{3}+1}{sin\;x}\)
(a) Find \({f}'(x)\)
(b) Find the gradient of the curve \(y=f(x)\)
(i) at \(x=\frac{\pi }{4}\) (ii) at \(x = 1\) rad.
▶️Answer/Explanation
Ans.
(a) \({f}'(x)=\frac{3x^{2}\;sin\;x-(x^{3}+1)cos\;x}{sin^{2}x}\)
(b) Directly by GDC (i) \({f}'(\frac{\pi }{4})\cong 0.518\) (ii)\({f}'(1)\cong 2.04\)
[Notice: the exact value for (i) is \({f}'(\frac{\pi }{4})=\frac{3\pi ^{2}}{16}\sqrt{2}-\frac{\pi ^{3}+64}{64}\sqrt{2}\)]
Question
[Maximum mark: 12]
Given the following values at \(x = 1\)

Calculate the derivatives of the following functions at \(x = 1\)
(i) \(y=3f(x)-2g(x)\) (ii) \(y = f (x)g(x)\)
(iii) \(y=\frac{f(x)}{g(x)}\) (iv) \(y=2x^{3}+1+5f(x)\)
▶️Answer/Explanation
Ans.
(a) \(\frac{dy}{dx}=2{f}'(x)-3{g}'(x)\), at \(x=1\) the value is -7
(b) \(\frac{dy}{dx}={f}'(x)g(x)+f(x){g}'(x)\), at \(x=1\) the value is 22
(c)\(\frac{dy}{dx}=\frac{{f}'(x)g(x)-f(x){g}'(x)}{g(x)^{2}}\),at \(x=1\) the value is \(\frac{2}{9}\)
(d)\(\frac{dy}{dx}=6x^{2}+5{f}'(x)\), at \(x=1\) the value is 26
Question
[Maximum mark: 4]
Let \(f(x)=6\sqrt[3]{x^{2}}\). Find \({f}'(x)\).
▶️Answer/Explanation
Ans.
\(f(x)=6x^{\frac{2}{3}}, {f}'(x)=4x^{-\frac{1}{3}}\left ( =\frac{4}{x^{\frac{1}{3}}}=\frac{4}{\sqrt[3]{x}} \right )\)
Question
[Maximum mark: 5]
Let \(g(x)=2x\) sin \(x\).
(a) Find g′(x).
(b) Find the exact value of the gradient of the graph of \(g\) at \(x\) = π.
▶️Answer/Explanation
Ans.
(a)\({g}'(x)=2\;sin\;x+2\;x\;cos\;x\)
(b)\({g}'(\pi )=2\;sin\;\pi +2\pi\;cos\;\pi =-2\pi\)
Question
[Maximum mark: 4]
Consider the function\(f(x)=k\;sin\;x+3x\), where \(k\) is a constant.
(a) Find \({f}'(x)\).
(b) When \(x=\frac{\pi }{3}\), the gradient of the curve of \(f (x)\) is 8. Find the value of \(k\) .
▶️Answer/Explanation
Ans.
(a)\({f}'(x)=k\;cos\;x+3\)
(b)\(k\;cos\;\left ( \frac{\pi }{3} \right )+3=8 \Rightarrow k\left ( \frac{1}{2} \right )+3=8\Rightarrow k=10\)
Question
[Maximum mark: 5]
Let \(f(x)=\frac{3x^{2}}{5x-1}\).
(a) Write down the equation of the vertical asymptote of \(y = f (x)\) .
(b) Find \({f}'(x)\) . Give your answer in the form \(\frac{ax^{2}+bx}{(5x-1)^{2}}\) where \(a\) and \(b\in \mathbb{Z}\) .
▶️Answer/Explanation
Ans.
(a)\(x=\frac{1}{5}\)
(b)\({f}'(x)=\frac{(5x-1)(6x)-(3x^{2})(5)}{(5x-1)^{2}}=\frac{30x^{2}-6x-15x^{2}}{(5x-1)^{2}}=\frac{15x^{2}-6x}{(5x-1)^{2}}\)
Question
[Maximum mark: 8]
Let \(f(x)=x\;cos\;x\), for 0 ≤ x ≤ 6.
(a) Find \({f}'(x)\).
(b) On the grid below, sketch the graph of \(y={f}'(x)\).
(c) Write down the range of the function \(y={f}'(x)\) , for 0 ≤ x ≤ 6
▶️Answer/Explanation
Ans.
(a)\({f}'(x)=cos\;x-x\;sin\;x\)
(b)
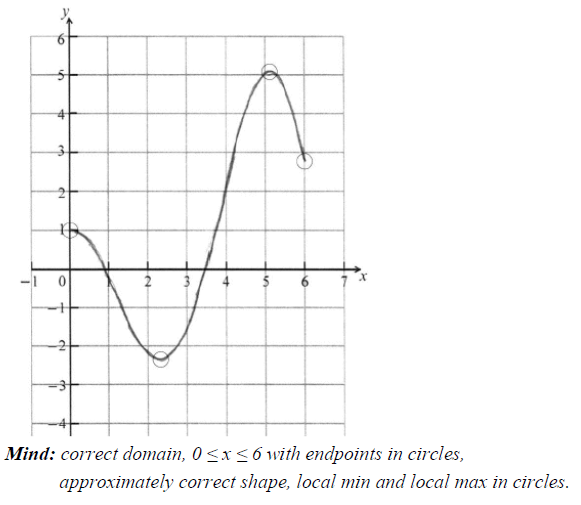
(c) y ∈ [-2.38 , 5.10]
Question
[Maximum mark: 7]
Let \(f(x)=e^{x}\;cos\;x\).
(a) Find \({f}'(x)\).
(b) Find the gradient of the normal to the curve of \(f\) at \(x=\pi \).
(c) Find the gradient of the tangent to the curve of \(f\) at \(x=\frac{\pi }{4}\).
▶️Answer/Explanation
Ans.
(a)\({f}'(x)=e^{x}\) x \((-sin\;x)+cos\;x \) x \(e^{x}=e^{x}\;cos\;x-e^{x}\;sin\;x\)
\({f}'(\pi)=e^{\pi }\;cos\;\pi -e^{\pi }\;sin\;\pi =-e^{\pi }\)
gradient of normal = \(\frac{1}{e^{\pi }}\)
(b)\({f}’\left ( \frac{\pi }{4} \right )=0\)
Question
[Maximum mark: 7]
Let \(f(x)=xe^{x}\).
(a) Find the equation of the tangent line at \(x = 0\).
(b) Find the equation of the normal line at \(x = 0\).
(c) Solve the equation \({f}'(x)=0\).
▶️Answer/Explanation
Ans.
(a) Point (0,0), \({f}'(x)=e^{x}+xe^{x}\), \(m_{T}=1\), Tangent line \(y=x\)
(b)\(m_{N}=-1\), Normal line \(y=-x\)
(c)\(e^{x}+xe^{x}=0\Leftrightarrow e^{x}(1+x)=0\Leftrightarrow x=-1\)
Question
[Maximum mark: 3 per function]
Find the derivative of each function below. [cover 3rd column; then compare with your answer]

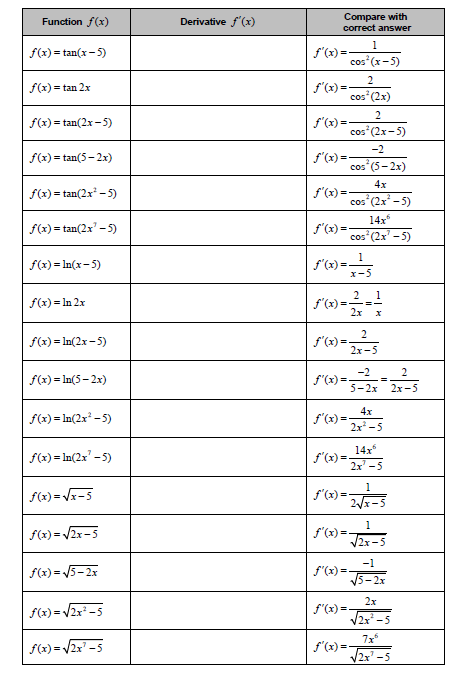

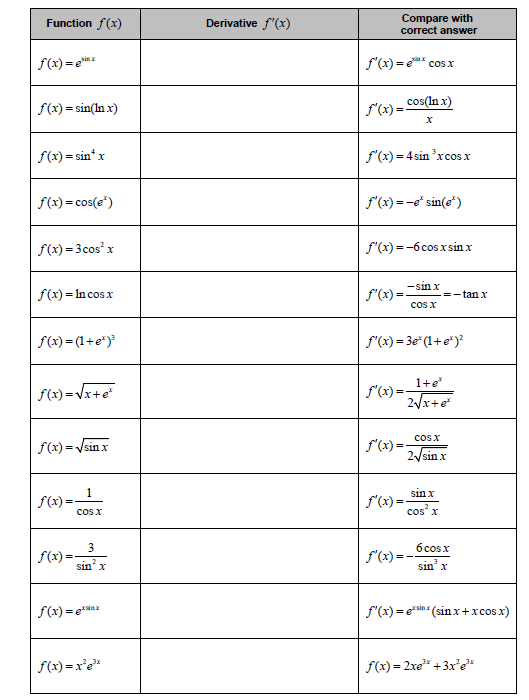
▶️Answer/Explanation
Ans.
Solutions are shown in the question.
Question
[Maximum mark: 3 per function]
Differentiate the following functions:

▶️Answer/Explanation
Ans.

Question
[Maximum mark: 10]
Find \(\frac{ds}{dt}\) for each of the following functions
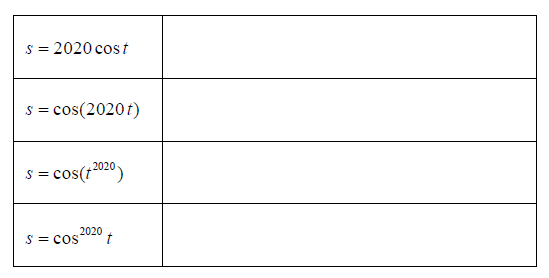
▶️Answer/Explanation
Ans.
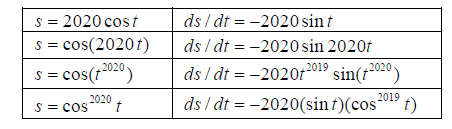
Question
[Maximum mark: 18]
Given that \(f(1)=2\) and \({f}'(1)=4\) , find the derivatives of the following functions at \(x =1\)
(i) \(y=f(x)^{2}\) (ii) \(y=f(x)^{3}\) (iii) \(y=ln\;f(x)\)
(iv) \(y=f(x^{2})\) (v) \(y=f(x^{3})\) (vi) \(y=\sqrt{f(x)}\)
▶️Answer/Explanation
Ans.
(i)\(y=f(x)^{2}\Rightarrow \frac{dy}{dx}=2f(x){f}'(x)\). At \(x=1,\frac{dy}{dx}=2x2x4=16\)
(ii)\(y=f(x)^{3}\Rightarrow \frac{dy}{dx}=3f(x)^{2}{f}'(x)\). At \(x=1,\frac{dy}{dx}=3×2^{2}x4=48\)
(iii)\(y=ln\;f(x)\Rightarrow \frac{dy}{dx}=\frac{{f}'(x)}{f(x)}\). At \(x=1,\frac{dy}{dx}=\frac{4}{2}=2\)
(iv)\(y=f(x^{2})\Rightarrow \frac{dy}{dx}={f}'(x^{2})x2x\). At \(x=1, \frac{dy}{dx}=2x2x1=4\)
(v)\(y=f(x^{3})\Rightarrow \frac{dy}{dx}={f}'(x^{3})x3x^{2}\). At\(x=1, \frac{dy}{dx}=2x3x1^{2}=6\)
(vi)\(y=\sqrt{f(x)}\Rightarrow \frac{dy}{dx}=\frac{1}{2\sqrt{f(x)}}{f}'(x)\). At \(x=1, \frac{dy}{dx}=\frac{1}{2\sqrt{2}}x4=\frac{2}{\sqrt{2}}=\sqrt{2}\)
Question
[Maximum mark: 10]
(a) Show that the derivative of \(y\) = tan \(x\) is \(\frac{1}{cos^{2}x}\).
(b) Hence, differentiate the functions
(i) \(y = x\; tan\; x\) (ii) \(y = tan\; 3x\)
(iii) \(y=tan^{2}x\) (iv) \(y=tan^{3}x\)
▶️Answer/Explanation
Ans.
(a) \(y=tan\;x=\frac{sin\;x}{cos\;x}\)
\(\frac{dy}{dx}=\frac{sin\;x\;sin\;x-cos\;x(-cos\;x)}{cos^{2}x}=\frac{sin^{2}x+cos^{2}x}{cos^{2}x}=\frac{1}{cos^{2}x}\)
(b) (i)\(\frac{dy}{dx}=\frac{x}{cos^{2}x}+tan\;x\)
(ii)\(\frac{dy}{dx}=\frac{3}{cos^{2}3x}\)
(iii)\(\frac{dy}{dx}=2\;tan\;x\frac{1}{cos^{2}x}\left ( =\frac{2\;sin\;x}{cos^{3}x} \right)\)
(iv)\(\frac{dy}{dx}=3\;tan^{2}x\frac{1}{cos^{2}x}\left ( =\frac{3\;sin^{2}x}{cos^{4}x} \right)\)
Question
[Maximum mark: 4]
Differentiate each of the following with respect to \(x\).
(a) \(y=x\;sin\;3x\)
(b) \(y=\frac{ln\;x}{x}\)
▶️Answer/Explanation
Ans.
(a) \(\frac{dy}{dx}=sin\;3x+3x\;cos\;3x\)
(b)\(\frac{dy}{dx}=\frac{xx\frac{1}{x}-ln\;x}{x^{2}}=\frac{1-ln\;x}{x^{2}}\)
Question
[Maximum mark: 4]
The point P \(\left ( \frac{1}{2},0 \right )\) lies on the graph of the curve of \(y=sin(2x-1)\).
Find the gradient of the tangent to the curve at P.
▶️Answer/Explanation
Ans.
\(y = sin\; (2x – 1)\) \(\frac{dy}{dx}=2\;cos(2x-1)\)
At \(\left ( \frac{1}{2},0 \right )\), the gradient of the tangent = 2 cos 0 = 2
Question
[Maximum mark: 4]
Differentiate with respect to \(x\): (i) \(y=(x^{2}+1)^{2}\). (ii) \(y=ln(3x-1)\)
▶️Answer/Explanation
Ans.
(i)\(\frac{d}{dx}(x^{2}+1)^{2}=2(x^{2}+1)x(2x)=4x(x^{2}+1)\)
(ii)\(\frac{d}{dx}(ln(3x-1))=\frac{1}{3x-1}x(3)=\frac{3}{3x-1}\)
Question
[Maximum mark: 4]
Differentiate with respect to \(x\) (i) \(\sqrt{3-4x}\) (ii) \(e^{sin\;x}\)
▶️Answer/Explanation
Ans.
(i)\(\frac{dy}{dx}=\frac{-4}{2\sqrt{3-4x}}=\frac{-2}{\sqrt{3-4x}}\)
OR \(y=\sqrt{3-4x}=(3-4x)^{\frac{1}{2}}\) \(\frac{dy}{dx}=\frac{1}{2}(3-4x)^{-\frac{1}{2}}(-4)\)
(ii) \(y=e^{sin\;x}\) \( \frac{dy}{dx}=(cos\;x)(e^{sin\;x})\)
Question
[Maximum mark: 5]
Let \(f(x)=e^{\frac{x}{3}}+5\;cos^{2}\;x\). Find \({f}'(x)\).
▶️Answer/Explanation
Ans.
\({f}'(x)=\frac{1}{3}e^{\frac{x}{3}}-10\;cos\;x\;sin\;x\)
Question
[Maximum mark: 6]
Let \(f(x) = cos\;2x\) and \(g(x) = ln(3x – 5)\).
(a) Find \({f}'(x)\).
(b) Find \({g}'(x)\).
(c) Let \(h(x) = f(x) × g(x)\). Find \({h}'(x)\).
▶️Answer/Explanation
Ans.
(a) \({f}'(x)\)=\(-sin\;2x\) x 2(=\(-2\;sin\;2x\))
(b) \({g}'(x)=3x\frac{1}{3x-5}\left ( =\frac{3}{3x-5} \right )\)
(c) product rule: \({h}'(x)=(cos\;2x)\left ( \frac{3}{3x-5} \right )+ln(3x-5)(-2\;sin\;2x)\)
Question
[Maximum mark: 6]
(a) Let \(f(x)=e^{5x}\). Write down \({f}'(x)\).
(b) Let \(g(x)=sin\;2x\). Write down \({g}'(x)\).
(c) Let \(h(x)=e^{5x}\;sin\;2x\). Find \({h}'(x)\).
▶️Answer/Explanation
Ans.
(a) \({f}'(x)=5e^{5x}\)
(b) \({g}'(x)=2\;cos\;2x\)
(c) \({h}’={fg}’+{gf}’=e^{5x}(2\;cos\;2x)+sin\;2x(5e^{5x})\)
Question
[Maximum mark: 6]
Let \(f(x)=e^{-3x}\) and \(g(x)=sin\left ( x-\frac{\pi }{3} \right )\).
(a) Write down (i) \({f}'(x)\); (ii)\({g}'(x)\).
(b) Let \(h(x)=e^{-3x}\;sin\left ( x-\frac{\pi }{3} \right )\). Find the exact value of \({h}’\left ( \frac{\pi }{3} \right )\).
▶️Answer/Explanation
Ans.
(a) (i) \(-3e^{-3x}\) (ii) \(cos\left ( x-\frac{\pi }{3} \right )\)
(b)\({h}'(x)=-3e^{-3x}sin\left ( x-\frac{\pi }{3} \right )+e^{-3x}cos\left ( x-\frac{\pi }{3} \right )\)
\({h}'(\frac{\pi }{3})=-3e^{-3\frac{\pi }{3}}sin\left ( \frac{\pi }{3}-\frac{\pi }{3} \right )+e^{-3\frac{\pi }{3}}cos\left ( \frac{\pi }{3}-\frac{\pi }{3} \right )=e^{-\pi }\)
Question
[Maximum mark: 6]
Let \(f(x)=(2x+7)^{3}\) and \(g(x)=cos^{2}(4x)\). Find (i) \({f}'(x)\); (ii)\({g}'(x)\)
▶️Answer/Explanation
Ans.
(i) \({f}'(x)=3(2x+7)^{2}x2=6(2x+7)^{2} (=24x^{2}+168x+294)\)
(ii)\({g}'(x)=2\;cos(4x)(-sin(4x))(4)=-8\;cos(4x)sin(4x) (=-4sin(8x))\)
Question
[Maximum mark: 6]
The population \(p\) of bacteria at time \(t\) is given by \(p\) = 100e0.05t. Calculate
(a) the value of \(p\) when \(t\) = 0;
(b) the rate of increase of the population when \(t\) = 10.
▶️Answer/Explanation
Ans.
(a) p = 100e0 = 100
(b) Rate of increase is \(\frac{dp}{dt}=0.05x100e^{0.05t}=5e^{0.05t}\)
When \(t=10\) \(\frac{dp}{dt}=5e^{0.05(10)}=5e^{0.5}\) \((=8.24=5\sqrt{e})\)
Question
[Maximum mark: 8]
The number of bacteria, \(n\), in a dish, after \(t\) minutes is given by \(n\) = 800e013t.
(a) Find the value of \(n\) when \(t\) = 0.
(b) Find the rate at which \(n\) is increasing when \(t\) = 15.
(c) After \(k\) minutes, the rate of increase in \(n\) is greater than 10 000 bacteria per
minute. Find the least value of \(k\), where \(k\in \mathbb{Z}\).
▶️Answer/Explanation
Ans.
(a) \(n\) = 800e0 = 800
(b) derivative: n′(15) = 731
(c) METHOD 1
setting up inequality. n′(t) > 10 000
\(k\) = 35.1226…, least value of \(k\) is 36
METHOD 2
n′(35) = 9842, and n′(36) = 11208
least value of \(k\) is 36
Question
[Maximum mark: 7]
Let \(f(x)=3x-e^{x-2}-4\), for \(-1\leq x\leq 5\).
(a) Find the x -intercepts of the graph of \(f\) .
(b) On the grid below, sketch the graph of \(f\) .
(c) Write down the gradient of the graph of \(f\) at \(x = 2\).
▶️Answer/Explanation
Ans.
(a) (1.54, 0) (4.13, 0) (accept x = 1.54 x = 4.13)
(b)

(c) gradient is 2
Question
[Maximum mark: 7]
Let \(f(x)=3x-e^{x-2}-4\), for \(-1\leq x\leq 5\).
(a) Find the x -intercepts of the graph of \(f\) .
(b) On the grid below, sketch the graph of \(f\) .
(c) Write down the gradient of the graph of \(f\) at \(x = 2\).

▶️Answer/Explanation
Ans.
(a) (1.54, 0) (4.13, 0) (accept x = 1.54 x = 4.13)
(b)

(c) gradient is 2
Question
[Maximum mark: 6]
If \(y=ln(2x-1)\) find \(\frac{d^{2}y}{dx^{2}}\).
▶️Answer/Explanation
Ans.
\(y-ln(2x-1)\Rightarrow \frac{dy}{dx}=\frac{2}{2x-1}\Rightarrow \frac{dy}{dx}=2(2x-1)^{-1}\)
\(\Rightarrow \frac{d^{2}y}{dx^{2}}=-2(2x-1)^{-2}(2)\)
\(\Rightarrow \frac{d^{2}y}{dx^{2}}=\frac{-4}{(2x-1)^{-2}}\)
Question
[Maximum mark: 6]
Consider the function \(y\) = tan \(x\) – 8sin \(x\) .
(a) Find \(\frac{dy}{dx}\).
(b) Find the value of cos \(x\) for which \(\frac{dy}{dx}=0\).
(c) Solve the equation \(\frac{dy}{dx}=0\). for \(-\pi \leq x\leq 2\pi\).
▶️Answer/Explanation
Ans.
(a) \(\frac{dy}{dx}=\frac{1}{cos^{2}x}-8cosx\)
(b) \(\frac{dy}{dx}=\frac{1-8cos^{3}x}{cos^{2}x}=0\Rightarrow cosx=\frac{1}{2}\)
(c) \(x=-\frac{\pi }{3},x=\frac{\pi }{3},x=\frac{5\pi }{3}\)
Question
[Maximum mark: 6]
Let \(y=e^{3x}\;sin(\pi x)\). Find
(a) \(\frac{dy}{dx}\).
(b) the smallest positive value of x for which \(\frac{dy}{dx}=0\).
▶️Answer/Explanation
Ans.
y = e3x sin(πx)
(a)\(\frac{dy}{dx}=3e^{3x}sin(\pi x)+\pi e^{3x}cos(\pi x)\)
(b) x = 0.7426… (0.743 to 3 s.f.)
Question
[Maximum mark: 6]
Let \(f(x)=cos^{3}(4x+1), 0\leq x\geq 1\). Find
(a) \({f}'(x)\)
(b) the exact values of the three roots of \({f}'(x)=0\).
▶️Answer/Explanation
Ans.
(a) \(-12cos^{2}(4x+1)sin(4x+1)\)
(b) \(x=\frac{\pi }{8}-\frac{1}{4},x=\frac{3\pi }{8}-\frac{1}{4},x=\frac{\pi-1 }{4}\)
Question
[Maximum mark: 6]
Let \(f\) be a cubic polynomial function. Given that \(f (0) = 2\), \({f}'(0)=-3\), \(f (1)\) = \({f}'(1)\)
and \({f}”(-1)=6\) , find \(f (x)\) .
▶️Answer/Explanation
Ans.
\(f(x)=ax^{3}+bx^{2}+cx+d\)
\({f}'(x)=3ax^{2}+2bx+c\)
\({f}”(x)=6ax+2b\)
\(f(0)=2=d\)
\({f}'(1)=f(1)\rightarrow a+b+c+2=3a+2b+c\)
\(2=2a+b\)
\({f}'(0)=-3=c\)
\({f}”(-1)=6=-6a+2b\)
\(b=\frac{12}{5},a=-\frac{1}{5}\)
\(f(x)=-\frac{1}{5}x^{3}+\frac{12}{5}x^{2}-3x+2 (Accept \;a=-\frac{1}{5},b=\frac{12}{5},c=-3,d=2)\)
Question
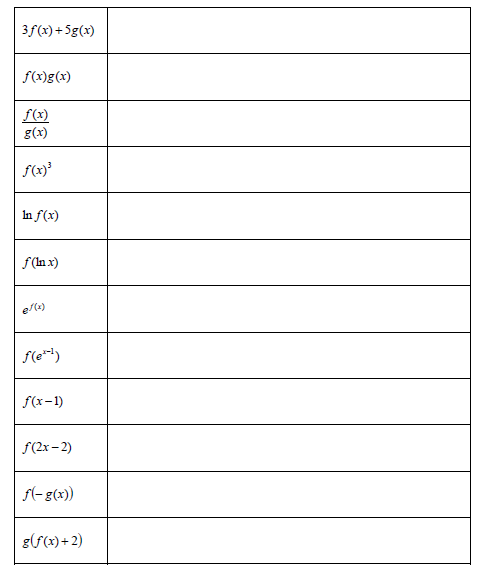
[Maximum mark: 3 per function]
The following table shows the values of two functions \(f\) and \(g\) and their derivatives
when \(x = 1\) and \(x = 0\) .
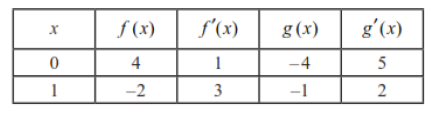
Find the derivatives of the following functions when \(x = 1\).
▶️Answer/Explanation
Ans.
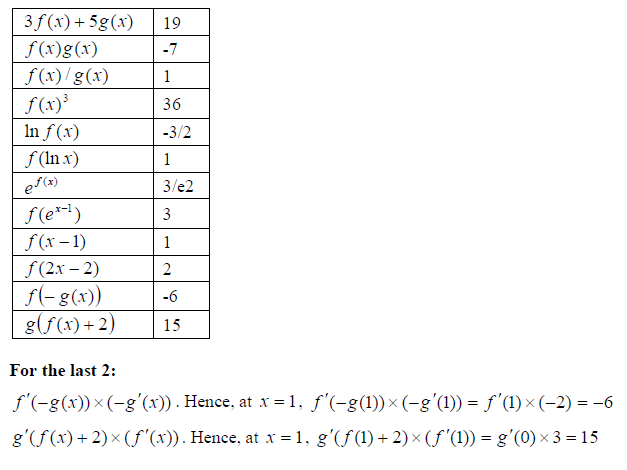
Question
[Maximum mark: 10]
Let \(f(x)=1+3\;cos(2x)\) for \(0\leq x\leq \pi \), and \(x\) is in radians.
(a) (i) Find \({f}'(x)\).
(ii) Find the values for \(x\) for which \({f}'(x)=0\) ; Give your answers in terms of π .
The function \(g(x)\) is defined as \(g(x)=f(2x)-1, 0\leq x\leq \frac{\pi }{2}\).
(b) (i) The graph of \(f\) may be transformed to the graph of \(g\) by a stretch in the
x -direction with scale factor \(\frac{1}{2}\) followed by another transformation.
Describe fully this other transformation.
(ii) Find the solution to the equation \(g(x) = f (x)\) .
▶️Answer/Explanation
Ans.
(a) (i)\({f}'(x)=-6\;sin\;2x\)
(ii) EITHER \({f}'(x)=-12sin\;x\;cos\;x=0\Rightarrow sin\;x=0\) or \(cos\;x=0\)
OR \(sin\;2x=0\), for \(0\leq 2x\leq 2\pi\)
THEN
\(x=0,\frac{\pi }{2},\pi \)
(b) (i) translation in the y-direction of –1
(ii) 1.11 (1.10 from TRACE is subject to AP)
Question
[Maximum mark: 20]
The diagram shows the graph of the function \(f\) given by \(f(x)=A\;sin\left ( \frac{\pi }{2}x \right )+B\), for
0 ≤ x ≤ 5 , where A and B are constants, and \(x\) is measured in radians.
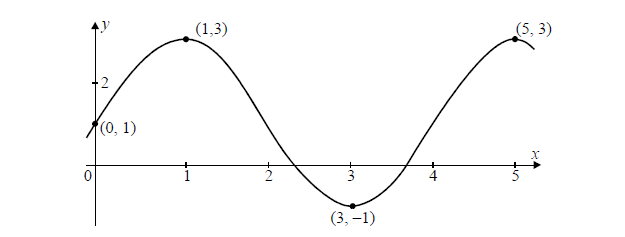
The graph includes the points (1, 3) and (5, 3), which are maximum points of the graph.
(a) Show that A = 2 , and find the value of B .
(b) Show that \({f}'(x)=\pi cos\left ( \frac{\pi }{2}x \right )\).
The line \(y=k-\pi x\) is a tangent line to the graph for 0 ≤ x ≤ 5 .
(c) Find
(i) the point where this tangent meets the curve;
(ii) the value of \(k\) .
(d) Solve the equation \(f (x) = 2\) for 0 ≤ x ≤ 5 .
▶️Answer/Explanation
Ans.
(a) EITHER \(A\;sin\left ( \frac{\pi }{2} \right )+B=3\) and \(A\;sin\left ( \frac{3\pi }{2} \right )+B=-1\)
\(\Leftrightarrow A+B=3,-A+B=-1\)
\(\Leftrightarrow A=2,B=1\)
OR
Amplitude = \(A =\frac{3-(-1)}{2}=\frac{4}{2}=2\)
Midpoint value = \(B =\frac{3+(-1)}{2}=\frac{2}{2}=1\)
(b) \(f(x)=2\;sin\left ( \frac{\pi }{2}x \right )+1\)
\({f}'(x)=\left ( \frac{\pi }{2} \right )2\;cos\left ( \frac{\pi }{2}x \right )+0=\pi \;cos\left ( \frac{\pi }{2}x \right )\)
(c) (i) y = k – πx is a tangent \(\Rightarrow -\pi =\pi \;cos\left ( \frac{\pi }{2}x\right )\)
\(\Rightarrow -1=cos\left ( \frac{\pi }{2}x\right )\)
\(\Rightarrow \frac{\pi }{2}x=\pi\) or \(3\pi\) or…
\(\Rightarrow x=2\) or 6…
Since 0 ≤ x ≤ 5, we take x = 2, so the point is (2, 1)
(ii) Tangent line is: y = –π(x – 2) + 1
y = (2π + 1) – πx
k = 2π + 1
(d) \(f(x)=2\Rightarrow 2\;sin\left ( \frac{\pi }{2}x \right )+1=2\)
\(\Rightarrow sin\left ( \frac{\pi }{2}x \right )=\frac{1}{2}\)
\(\Rightarrow \frac{\pi }{2}x=\frac{\pi }{6}or\frac{5\pi }{6}or\frac{13\pi }{6}\)
\(x=\frac{1}{3}or\frac{5}{3}\) or \(\frac{13}{3}\)
Question
[Maximum mark: 14]
The following diagram shows a waterwheel with a bucket. The wheel rotates at a
constant rate in an anticlockwise (counterclockwise) direction.
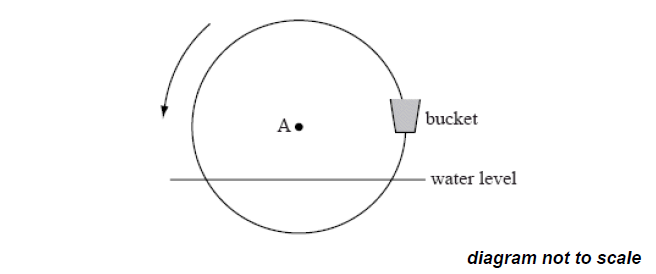
The diameter of the wheel is 8 metres. The centre of the wheel, A, is 2 metres above
the water level. After \(t\) seconds, the height of the bucket above the water level is given
by \(h = a\) sin \(bt + 2\).
(a) Show that \(a = 4\).
The wheel turns at a rate of one rotation every 30 seconds.
(b) Show that \(b=\frac{\pi }{15}\).
In the first rotation, there are two values of \(t\) when the bucket is descending at a rate of
0.5 m s–1.
(c) Find these values of \(t\).
(d) Determine whether the bucket is underwater at the second value of \(t\).
▶️Answer/Explanation
Ans.
(a) recognizing the amplitude is the radius: \(a=\frac{8}{2}\Rightarrow a=4\)
(b) period = 30: \(b=\frac{2\pi }{30}=\frac{\pi }{15}\)
(c) recognizing \({h}'(t)=-0.5\)
\(-0.5=\frac{4\pi }{15}cos\left ( \frac{\pi }{15}t \right )\Rightarrow t-10.6,t=19.4\)
(d) h(t) < 0 so underwater; h(t) > 0 so not underwater
h(19.4) = \(4\;sin\frac{19.4\pi }{15}+2=-1.19\)
OR
solving h(t) = 0, graph showing region below x-axis, roots 17.5, 27.5
Hence, the bucket is underwater, yes
Question
[Maximum mark: 18]
Let \(f (x) = 3sinx + 4\; cos\; x\), for –2π ≤ x ≤ 2π.
(a) Sketch the graph of \(f\).
(b) Write down
(i) the amplitude; (ii) the period; (iii) the x-intercept between \(-\frac{\pi }{2}\) and 0.
(c) Hence write \(f (x)\) in the form \(p\) sin \((qx + r)\).
(d) Write down one value of \(x\) such that \({f}'(x)=0\).
(e) Write down the two values of \(k\) for which the equation \(f (x) = k\) has exactly two
solutions.
(f) Let \(g(x) = ln(x + 1)\), for 0 ≤ x ≤ π. There is a value of \(x\), between 0 and 1, for which
the gradient of \(f\) is equal to the gradient of \(g\). Find this value of \(x\).
▶️Answer/Explanation
Ans.
(a)
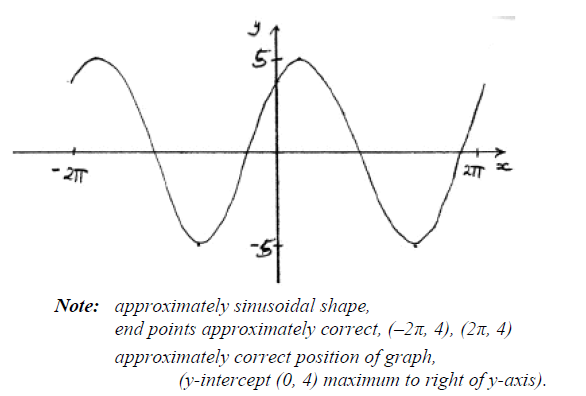
(b) (i) 5 (ii) 2π (6.28) (iii) –0.927
(c) \(f(x)\) = 5 sin (x + 0.927) (accept \(p\) = 5, \(q\) = 1, \(r\) = 0.927)
(d) (man or min)
one 3 s.f. value which rounds to one of –5.6, –2.5, 0.64, 3.8
(e) \(k\) = –5, \(k\) = 5
(f) \({g}'(x)=\frac{1}{x+1}\)
\({f}'(x)=3\;cos\;x-4\;sin\;x\) \((5\;cos(x+0.927))\)
\({g}'(x)={f}'(x)\)
\(x=0.511\)
Question
[Maximum mark: 15]
(a) The function \(g\) is defined by \(g(x)=\frac{e^{x}}{\sqrt{x}}\), for 0 < x ≤ 3 .
(i) Sketch the graph of \(g\) .
(ii) Find \({g}'(x)\) .
(iii) Write down an expression representing the gradient of the normal to the
curve at any point.
(b) Let P be the point \((x, y)\) on the graph of \(g\) , and Q the point (1,0).
(i) Find the gradient of (PQ) in terms of \(x\) .
(ii) Given that the line (PQ) is a normal to the graph of \(g\) at the point P, find the
minimum distance from the point Q to the graph of \(g\) .
▶️Answer/Explanation
Ans.
(a)
(ii) \(g(x)=\frac{e^{x}}{\sqrt{x}}\)
\({g}'(x)=\frac{e^{x}\sqrt{x}-\frac{e^{x}}{2\sqrt{x}}}{x}\)
\(=\frac{(2x-1)e^{x}}{2x\sqrt{x}}\)
(iii) gradient is \(-\frac{1}{{g}'(x)}\)
\(=\frac{2x\sqrt{x}}{(1-2x)e^{x}}\)
(b) (i) \(\frac{y-0}{x-1}=\frac{e^{x}}{\sqrt{x}(x-1)}\)
(ii) EITHER
\(\frac{e^{x}}{\sqrt{x}(x-1)}=\frac{2x\sqrt{x}}{(1-2x)e^{x}}\)
\(x=0.5454…\)
OR
\(D^{2}=(x-1)^{2}+y^{2}=(x-1)^{2}+\frac{e^{2x}}{x}\)
\(\frac{dD^{2}}{dx^{2}}=2(x-1)+\frac{2e^{2x}x-e^{2x}}{x^{2}}=0\)
\(x=0.5454…\)
THEN
distance = \(\sqrt{(1-0.5454)^{2}+\left ( \frac{e^{0.5454}}{\sqrt{0.5454}} \right )^{2}}\)
=2.38
[MAI 5.9] RELATED RATES-lala
Question
[Maximum mark: 8]
The quantity A increases at a constant rate \(\frac{dA}{dt}=3\).
(a) Given that \(C=2A^{3}+1\), find the rate of change of C , at the instant when A = 2 ;
(b) Given that \(ln\;D=\frac{3}{A}\) , find the rate of change of D , at the instant when D = e ;
▶️Answer/Explanation
Ans.
(a) \(C=2A^{3}+1\Rightarrow \frac{dC}{dt}=6A^{2}\frac{dA}{dt}\)
\(\Rightarrow \frac{dC}{dt}=18A^{2}\).
When \(A=2\),then \(\frac{dC}{dt}=72\).
(b) \(ln\;D=\frac{3}{B}\Rightarrow \frac{1}{D}\frac{dD}{dt}=-\frac{3}{B^{2}}\frac{dB}{dt}\)
\(\Rightarrow \frac{dD}{dt}=-\frac{6D}{B^{2}}\).
When \(D=e, B=3\) then \(\frac{dD}{dt}=-\frac{2e}{3}\)
Question
[Maximum mark: 5]
The quantities A and B increase at constant rates \(\frac{dA}{dt}=3\) and \(\frac{dB}{dt}=2\) respectively.
Given that \(F=2A^{2}B+2B^{3}\), find the rate of change of F , when A = B = 1.
▶️Answer/Explanation
Ans.
\(F=2A^{2}B+2B^{3}\Rightarrow \frac{dF}{dt}=4AB\frac{dA}{dt}+2A^{2}\frac{dB}{dt}+6B^{2}\frac{dB}{dt}\)
\(\frac{dF}{dt}=12AB+4A^{2}+12B^{2}\).
When \(A=B=1\) then \(\frac{dF}{dt}=28\)
Question
[Maximum mark: 6]
The quantities A and B increase at constant rates \(\frac{dA}{dt}=3\) and \(\frac{dB}{dt}=2\) respectively.
Given that \(F^{4}=2A^{2}B+2B^{3}\), find the rate of change of F , when A = B = 1.
▶️Answer/Explanation
Ans.
\(F^{4}=2A^{2}B+2B^{3}\Rightarrow 4F^{3}\frac{dF}{dt}=4AB\frac{dA}{dt}+2A^{2}\frac{dB}{dt}+6B^{2}\frac{dB}{dt}\)
\(\Rightarrow 2F^{3}\frac{dF}{dt}=2AB\frac{dA}{dt}+A^{2}\frac{dB}{dt}+3B^{2}\frac{dB}{dt}\)
Thus, \(\Rightarrow 2F^{3}\frac{dF}{dt}=6AB+2A^{2}+6B^{2}\)
When \(A=B=1\) then \(F^{4}=4\Rightarrow F^{2}=2\Rightarrow F=\sqrt{2}\)
Finally,
\(\Rightarrow 2\sqrt{2}^{3}\frac{dF}{dt}=6+2+6\Rightarrow 4\sqrt{2}\frac{dF}{dt}=14\Rightarrow 4\sqrt{2}\frac{dF}{dt}=\frac{7}{2\sqrt{2}}\)
Question
[Maximum mark: 6]
Air is pumped into a spherical ball which expands at a rate of 8 cm3 per second (8 cm3s–1).
Find the exact rate of increase of the radius of the ball when the radius is 2 cm.
▶️Answer/Explanation
Ans.
\(\frac{dV}{dt}=8(cm^{3}s^{-1}),\)
\(V=\frac{4}{3}\pi r^{3}\Rightarrow \frac{dV}{dt}=4\pi r^{2}x\frac{dr}{dt}\Rightarrow \frac{dr}{dt}=\left ( \frac{dV}{dt} \right )\div \left ( \frac{dV}{dr} \right )\)
When \(r=2\), \(\frac{dr}{dt}=8\div (4\pi x2^{2})=\frac{1}{2\pi }(cm\;s^{-1})\) \((i.e.\cong 0.159)\)
Question
[Maximum mark: 6]
The following diagram shows an isosceles triangle ABC with AB = 10 cm and AC = BC. The
vertex C is moving in a direction perpendicular to (AB) with speed 2 cm per second.

Calculate the rate of increase of the angle CAB at the moment the triangle is equilateral.
▶️Answer/Explanation
Ans.
Let h = height of triangle and θ = \(\hat{CAB}\).
\(h=5tan\Theta \Rightarrow \frac{dh}{dt}=\frac{5}{cos^{2}x}\frac{d\Theta }{dt}\)
Put \(\Theta =\frac{\pi }{3}\). 2=5x4x\(\frac{d\Theta }{dt}\)
\(\frac{d\Theta }{dt}=\frac{1}{10}\) rad per sec ( Accept\(\frac{18^{\circ}}{\pi }\) per second or \(5.73^{\circ}\)per second )
Question
[Maximum mark: 6]
An airplane is flying at a constant speed at a constant altitude of 3 km in a straight line
that will take it directly over an observer at ground level. At a given instant the observer
notes that the angle θ is \(\frac{1}{3}\)π radians and is increasing at \(\frac{1}{60}\) radians per second.
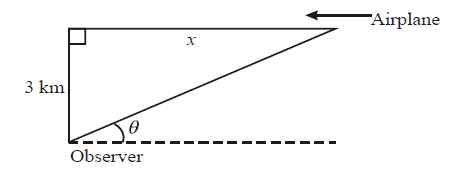
Find the speed, in kilometres per hour, at which the airplane is moving towards the observer.
▶️Answer/Explanation
Ans.
\(tan\;\Theta =\frac{3}{x}\Rightarrow \frac{1}{cos^{2}\Theta }\frac{d\Theta }{dt}=\frac{-3}{x^{2}}\frac{dx}{dt}\)
When \(\Theta =\frac{\pi }{3},x^{2}=3\) and \(cos^{2}\Theta =\frac{1}{4}\)
Hence, \(4\frac{1}{60}=-\frac{dx}{dt}\Rightarrow \frac{dx}{dt}=-\frac{1}{15}km\;s^{-1}=-240\;km\;h^{-1}\)
The aeroplane is moving towards him at 240 km \(h^{-1}\)
Question
[Maximum mark: 5]
In the previous problem, find the rate of change of the distance between the observer
and the airplane, at the instant when the angle θ is \(\frac{1}{3}\)π radians and is increasing at \(\frac{1}{60}\) radians per second.
▶️Answer/Explanation
Ans.
If \(z\) is the distance then \(z^{2}=3^{2}+x^{2}\Rightarrow z^{2}=9+x^{2}\)
Then \(2z\frac{dz}{dt}=2x\frac{dx}{dt}\Rightarrow z\frac{dz}{dt}=x\frac{dx}{dt}\)
When \(\Theta =\frac{\pi }{3}, x=\sqrt{3},z\sqrt{12}=2\sqrt{3}\) and \(\frac{dx}{dt}\)=-240 km \(h^{-1}\)
Hence,
\(\Rightarrow 2\sqrt{3}\frac{dz}{dt}=-240\sqrt{3}\Rightarrow \frac{dz}{dt}\)=-240 km \(h^{-1}\)
Question
[Maximum mark: 6]
A conical tank with vertex down is 8 metres in diamater and 12 meters deep. Water
flows into the tank at 10 m3 per minute. Find the rate of change of the depth of the
water at the instant when the water is 6 meters deep.
▶️Answer/Explanation
Ans.
\(\frac{r}{h}=\frac{4}{12}\Rightarrow r=\frac{h}{3}\)
\(V=\frac{\pi }{3}\left (\frac{h}{3} \right )^{2}(h)\)
\(V=\frac{\pi }{27}h^{3}\)
\(\frac{dV}{dt}=\frac{\pi }{9}h^{2}\frac{dh}{dt}\)
\(10=\frac{\pi }{9}(6)^{2}\frac{dh}{dt}\)
\(\frac{dh}{dt}=\frac{90}{36\pi }\left ( =\frac{5}{2\pi }=0.796 \right )\) metres per min
Question
[Maximum mark: 6]
Car A is travelling on a straight east-west road in a westernly direction at 60 km h-1.
Car B is travelling on a straight north-soutg road in a northernly direction at 70 km h-1.
The roads intersect at the point O. When Car A is x km east of O, and Car B is y km
south of O, the distance between the cars is z km.
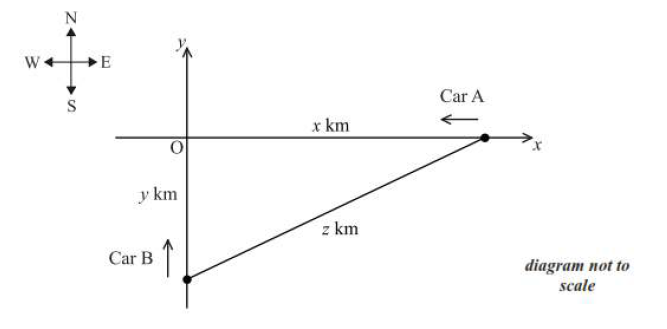
Find the rate of change of z when Car A is 0.8 km east of O and Car B is 0.6 km south
of O.
▶️Answer/Explanation
Ans.
\(z^{2}=x^{2}+y^{2}\) (or equivalent)
\(z=\sqrt{0.8^{2}+0.6^{2}}\) (=1,initially)
Attempting to differentiate implicitly with respect to \(t\)
\(2z\frac{dz}{dt}=2x\frac{dx}{dt}+2y\frac{dy}{dt}\)
\(\frac{dz}{dt}\)=-(0.8×60)-(0.6×70)
Rate is -90 (km h-1)
Question
[Maximum mark: 5]
In problem 9, answer the same question if Car A was travelling in an easternly direction.
▶️Answer/Explanation
Ans.
Then \(\frac{dx}{dt}=60\) (positive) , \(\frac{dz}{dt}\) =+0.8 x 60 – 0.6 x 70 = 6 km h-1
Question
[Maximum mark: 6]
The volume of a solid is given by \(V=\frac{4}{3}\pi r^{3}+\pi r^{2}h\).
At the time when the radius is 3 cm, the volume is 81π cm3, the radius is changing at a
rate of 2 cm/min and the volume is changing at a rate of 204π cm3/min. Find the rate of
change of the height at this time.
▶️Answer/Explanation
Ans.
\(81\pi =\frac{4}{3}\pi (3)^{3}+\pi (3)^{2}h\)
\(81\pi =36\pi +9\pi h\)
\(h=5\) (cm)
\(V=\frac{4}{3}\pi r^{3}+\pi r^{2}h\)
Attempt to differentiate with respect to time.
\(\frac{dV}{dt}=4\pi r^{2}\frac{dr}{dt}+2\pi rh\frac{dr}{dt}+\pi r^{2}\frac{dh}{dt}\)
\(204\pi=4\pi (3)^{2}(2)+2\pi (3)(5)(2)+\pi (3)^{2}\frac{dh}{dt}\)
\(204\pi=72\pi+60\pi+9\pi\frac{dh}{dt}\)
\(72\pi=9\pi\frac{dh}{dt}\)
\(\frac{dh}{dt}\)=8 (cm/min)
Question
[Maximum mark: 24]
The diagram shows a right-angled triangle PQR, with \(\hat{Q}=\frac{\pi }{2}\) and QR = 5 m (constant).
The sides PQ = x and PR = y are variable, as P can be moved horizontally on the line (PQ).
Hence the angle θ =\(\hat{RPQ}\), the area A and the perimeter P of the triangle are also variable.
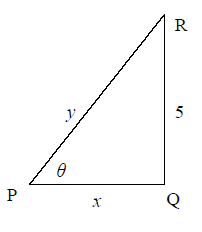
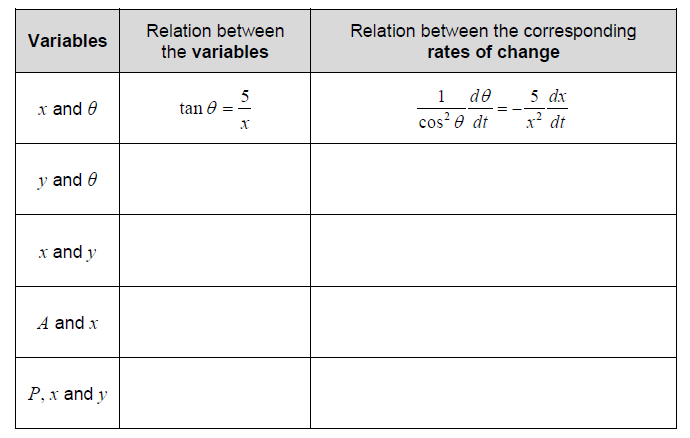
(a) Complete the following table
(b) At the instant when x = 5m, write down the values of
(i) θ (ii) y (iii) A (iv) P
(c) Given that P is moving to the left by 0.5 m per second, find the rate of change of the
following at the instant when x = 5m
(i) x (ii) y (iii) A (iv) P
▶️Answer/Explanation
Ans.
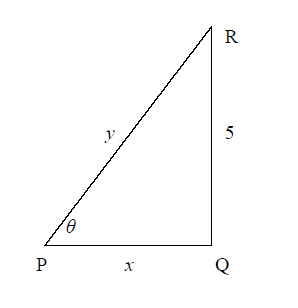
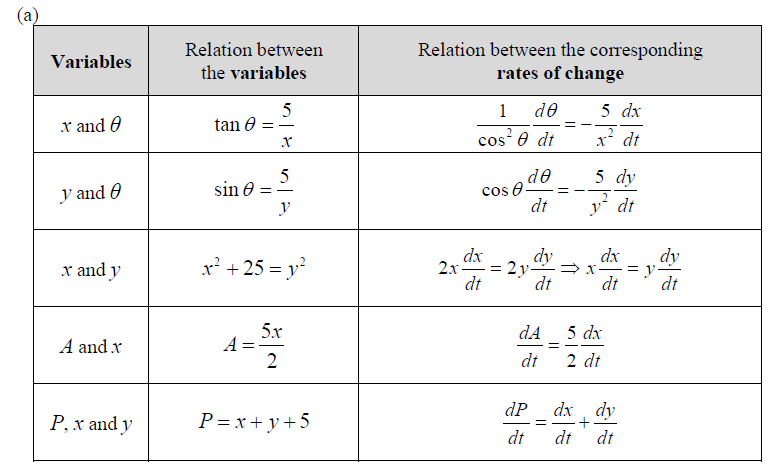
(b) When \(x=5\) : (i) \(\Theta =\frac{\pi }{4}\) (ii) \(y=5\sqrt{2}\) (iii) \(A=\frac{25}{2}\) (iv) \(P=10+5\sqrt{2}\)
(c) \(\frac{dx}{dt}=0.5\)
(i) \(\frac{1}{cos^{2}\Theta }\frac{d\Theta }{dt}=-\frac{5}{x^{2}}\frac{dx}{dt}\Rightarrow 4x\frac{d\Theta }{dt}=-\frac{5}{5^{2}}0.5\Rightarrow \frac{d\Theta }{dt}=-\frac{1}{40}\)
(ii) \(x\frac{dx}{dt}=y\frac{dy}{dt}\Rightarrow 5×0.5=5\sqrt{2}\frac{dy}{dt}\Rightarrow \frac{dy}{dt}=\frac{1}{2\sqrt{2}}\)
(iii) \(\frac{dA}{dt}=\frac{5}{2}\frac{dx}{dt}\Rightarrow \frac{dA}{dt}=\frac{5}{2}0.5\Rightarrow \frac{dA}{dt}=\frac{5}{4}\)
(iv) \(\frac{dP}{dt}=\frac{dx}{dt}+\frac{dy}{dt}\Rightarrow \frac{dP}{dt}=\frac{1}{2}+\frac{1}{2\sqrt{2}}=\frac{2+\sqrt{2}}{4}\)
Question
[Maximum mark: 12]
The height and the diameter of the base of a cylinder are equal.
(a) Express the volume V of the cylinder in terms of the radius r .
(b) Show that the surface area S of the cylinder is given by \(S=6\pi r^{2}\).
(c) Find the relations between
(i) the rates \(\frac{dV}{dt}\) and \(\frac{dr}{dt}\). (ii) the rates \(\frac{dS}{dt}\) and \(\frac{dr}{dt}\).
(d) Hence, find the relation between the rates \(\frac{dV}{dt}\) and \(\frac{dS}{dt}\).
The volume of the cylinder increases at a constant rate of 6 cm3 min-1.
(e) Find the rate of change of the surface area at the instant when r = 12 cm.
▶️Answer/Explanation
Ans.
It is given that \(h = 2r\)
(a) \(V=\pi r^{2}h=2\pi r^{3}\)
(b) \(S=2\pi r^{2}+2\pi rh=2\pi r^{2}+4\pi r^{2}=6\pi r^{2}\)
(c) (i) \(\frac{dV}{dt}=6\pi r^{2}\frac{dr}{dt}\) (ii) \(\frac{dS}{dt}=12\pi r\frac{dr}{dt}\)
(d) Divide (c)(i) by (c)(ii): \(\frac{\frac{dV}{dt}}{\frac{dS}{dt}}=\frac{6\pi r^{2}\frac{dr}{dt}}{12\pi r\frac{dr}{dt}}=\frac{r}{2}\Rightarrow \frac{dV}{dt}=\frac{r}{2}\frac{dS}{dt}\)
(e) \(\frac{dV}{dt}=\frac{r}{2}\frac{dS}{dt}\Rightarrow 6=\frac{12}{2}\frac{dS}{dt}\Rightarrow \frac{dS}{dt}=1\)