Question
Topic: Measurements
Discuss: quantity has the fundamental SI units of $\mathrm{kg} \mathrm{m}^{-1} \mathrm{~s}^{-2} ?$
A. Energy
B. Force
C. Momentum
D. Pressure
▶️Answer/Explanation
Ans:D
Solution:
The quantity that has the fundamental SI units of $\mathrm{kg} \mathrm{m}^{-1} \mathrm{~s}^{-2}$ is pressure.
Therefore, the answer is D. Pressure.
Pressure is defined as force per unit area and its SI unit is Pascal (Pa). In terms of fundamental SI units, 1 Pa can be expressed as $\mathrm{kg} \mathrm{m}^{-1} \mathrm{~s}^{-2}$, which matches the given units.
Question
Topic: Forces
Given: Two forces act along a straight line on an object that is initially at rest. One force is constant; the second force is in the opposite direction and proportional to the velocity of the object.

Discuss: correct about the motion of the object?
A. The acceleration increases from zero to a maximum.
B. The acceleration increases from zero to a maximum and then decreases.
C. The velocity increases from zero to a maximum.
D. The velocity increases from zero to a maximum and then decreases.
▶️Answer/Explanation
Ans:C
Solution:
Since one force is constant and the other force is proportional to the velocity and in the opposite direction, the net force acting on the object decreases as the object’s velocity increases. At some point, the opposing force becomes equal in magnitude to the constant force, resulting in zero net force and a constant velocity.
Initially, when the object is at rest, the net force is equal to the constant force. Therefore, the object accelerates in the direction of the constant force. As the velocity increases, the opposing force also increases proportionally, slowing down the acceleration. Eventually, the opposing force becomes equal to the constant force, and the net force acting on the object becomes zero. At this point, the object moves with a constant velocity, which is the maximum velocity attained during the motion. Therefore, the correct option is C. The velocity increases from zero to a maximum.
Question
Topic: Motion
Given: A ball falls from rest in the absence of air resistance. The position of the centre of the ball is determined at one-second intervals from the instant at which it is released.
Discuss: What are the distances, in metres, travelled by the centre of the ball during each second for the first $4.0 \mathrm{~s}$ of the motion?
A. $5,10,15,20$
B. $5,15,25,35$
C. $5,20,45,80$
D. $5,25,70,150$
▶️Answer/Explanation
Ans: B
Solution:
If we use $g = 10 \mathrm{~m/s^2}$, then the distances travelled by the centre of the ball during each second for the first $4.0\mathrm{~s}$ of the motion are:
- For the first second: $d = \frac{1}{2}(10\mathrm{~m/s^2})(1\mathrm{~s})^2 = 5\mathrm{~m}$
- For the second second: $d = \frac{1}{2}(10\mathrm{~m/s^2})(2\mathrm{~s})^2 – \frac{1}{2}(10\mathrm{~m/s^2})(1\mathrm{~s})^2 = 20\mathrm{~m} – 5\mathrm{~m} = 15\mathrm{~m}$
- For the third second: $d = \frac{1}{2}(10\mathrm{~m/s^2})(3\mathrm{~s})^2 – \frac{1}{2}(10\mathrm{~m/s^2})(2\mathrm{~s})^2 = 45\mathrm{~m} – 20\mathrm{~m} = 25\mathrm{~m}$
- For the fourth second: $d = \frac{1}{2}(10\mathrm{~m/s^2})(4\mathrm{~s})^2 – \frac{1}{2}(10\mathrm{~m/s^2})(3\mathrm{~s})^2 = 80\mathrm{~m} – 45\mathrm{~m} = 35\mathrm{~m}$
Therefore, the distances travelled by the centre of the ball during each second for the first $4.0\mathrm{~s}$ of the motion, using $g = 10 \mathrm{~m/s^2}$, are $5\mathrm{~m}$, $15\mathrm{~m}$, $25\mathrm{~m}$, and $35\mathrm{~m}$. The correct option is B. $5,15,25,35$.
Question
Topic: Motion
Given: An object is thrown from a cliff at an angle to the horizontal. The ground below the cliff is horizontal.
Three quantities are known about this motion.
- The horizontal component of the initial velocity of the object
- The initial angle between the velocity of the object and the horizontal
- The height of the cliff
Discuss: the quantities that must be known in order to determine the horizontal distance from the point of projection to the point at which the object hits the ground?
A. I and II only
B. I and III only
C. II and III only
D. I, II and III
▶️Answer/Explanation
Ans:D
Solution:
To determine the horizontal distance from the point of projection to the point at which the object hits the ground, we need to know the initial horizontal velocity and the time of flight. The initial horizontal velocity is the horizontal component of the initial velocity of the object, which is given in I. The time of flight can be determined using the initial vertical velocity, the acceleration due to gravity, and the height of the cliff, which is given in III.
The initial vertical velocity can be found using the initial velocity and the initial angle of projection, which is given in II. Once the initial vertical velocity is known, the time of flight can be found using the formula for the time taken for an object to reach maximum height and the formula for the time taken for an object to fall from a given height.
Therefore, all three quantities I, II and III are needed to determine the horizontal distance from the point of projection to the point at which the object hits the ground. Hence, the correct answer is option D.
Question
Topic: Momentum and impulse
Given: The variation with time $t$ of the acceleration a of an object is shown.
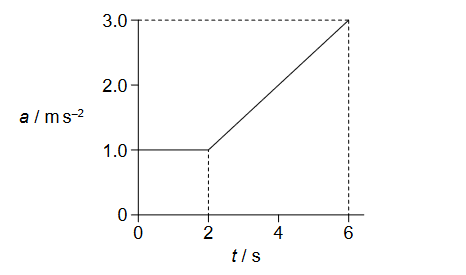
Calculate: the change in velocity of the object from $t=0$ to $t=6 \mathrm{~s}$ ?
A. $6 \mathrm{~ms}^{-1}$
B. $8 \mathrm{~ms}^{-1}$
C. $10 \mathrm{~m} \mathrm{~s}^{-1}$
D. $14 \mathrm{~m} \mathrm{~s}^{-1}$
▶️Answer/Explanation
Ans:C
Solution:
The change in velocity of the object from $t=0$ to $t=6 \mathrm{~s}$ can be determined by finding the area under the acceleration-time graph between these two times. This area represents the change in velocity of the object during this time interval, according to the kinematic equation:
$\Delta v = \int_{t_1}^{t_2} a(t) dt$
Using the graph, we can see that the acceleration of the object is constant at $1 \mathrm{~ms}^{-2}$ between $t=0$ and $t=2 \mathrm{~s}$. Therefore, we can calculate the change in velocity during this time interval as:
$\Delta v_1 = a_1 \Delta t = 1 \mathrm{~ms}^{-2} \times 2 \mathrm{~s} = 2 \mathrm{~ms}^{-1}$
Between $t=2 \mathrm{~s}$ and $t=6 \mathrm{~s}$, the acceleration of the object varies linearly from $1 \mathrm{~ms}^{-2}$ to $3 \mathrm{~ms}^{-2}$. Therefore, we can calculate the change in velocity during this time interval as the area of a trapezium:
$\Delta v_2 = \frac{1}{2} (a_1 + a_2) \Delta t = \frac{1}{2} (1 \mathrm{~ms}^{-2} + 3 \mathrm{~ms}^{-2}) \times 4 \mathrm{~s} = 8 \mathrm{~ms}^{-1}$
The total change in velocity of the object from $t=0$ to $t=6 \mathrm{~s}$ is therefore:
$\Delta v = \Delta v_1 + \Delta v_2 = 2 \mathrm{~ms}^{-1} + 8 \mathrm{~ms}^{-1} = 10 \mathrm{~ms}^{-1}$
Therefore, the correct answer is the option C.
