Question
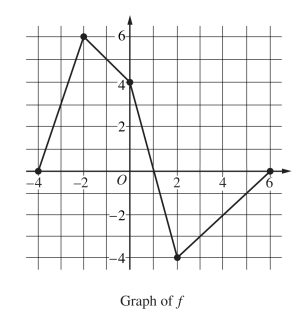
Let f be a continuous function defined on the closed interval −4 ≤ x ≤ 6. The graph of f , consisting of four line segments, is shown above. Let G be the function defined by \(G(x)=\int_{0}^{x}f(t)dt.\)
(a) On what open intervals is the graph of G concave up? Give a reason for your answer.
(b) Let P be the function defined by P (x) = G (x)• f(x). Find P'(3 ).
(c) Find \(\lim_{x\rightarrow 2}\frac{G(x)}{x^{2}-2x}.\)
(d) Find the average rate of change of G on the interval [−4, 2]. Does the Mean Value Theorem guarantee a value c, −4 < c < 2, for which G'(c) is equal to this average rate of change? Justify your answer.
Answer/Explanation
Ans:
(a)
G'(x) = f(x)
G”(x) = f'(x)
On (-4, -2) and (2, 6), G(x) is concave up because f(x) (which is equal to G'(x)) has a positive slope / is increasing.
(b)
p'(x) = G'(x) f(x) + f'(x) G(x)
p'(3) = G'(3) f(3) + f'(3) G(3)
G'(x) = f(x) \(G(3)=\int_{0}^{3}f(t)dt=-\frac{7}{2}\)
\(p'(3)=(-3)(-3)+(1)\left (- \frac{7}{2} \right )\)
(c)
\(\lim_{x\rightarrow 2}G(x)=\lim_{x\rightarrow 2}(x^{2}-2x)=0\) Must be I’hopital’s rule
\(\int_{0}^{2}f(t)dt=0\)
\(\lim_{x\rightarrow 2}\frac{G'(x)}{2x-2}=\frac{f(2)}{4-2}=\frac{-4}{2}\)
(d)
\(\int_{0}^{2}f(t)dt\) \(\int_{0}^{-4}f(t)dt= -(3+9+3+1)\)
= -16
AROC of \(G = \frac{G(2)-G(-4)}{2-(-4)}=\frac{0-(16)}{2+4}=\frac{16}{6}=\frac{8}{3}\)
The meanvalue theorem does guarantee a value of -4<c<2, for which G'(c) is equal to average rate of chang. This is because G'(x) = f(t) and x = t exists for all values, -4<x=+t<2, meaning that G(x) is continuous on the closed interval and differentiable on the open interval.
Question
| t (years) | 2 | 3 | 5 | 7 | 10 |
| H(t) (meters) | 1.5 | 2 | 6 | 11 | 15 |
The height of a tree at time t is given by a twice-differentiable function H, where H (t) is measured in meters and t is measured in years. Selected values of H (t) are given in the table above.
(a) Use the data in the table to estimate H'(6 ). Using correct units, interpret the meaning of H'(6 ) in the context of the problem.
(b) Explain why there must be at least one time t, for 2 < t< 10 , such that H'(t) = 2.
(c) Use a trapezoidal sum with the four subintervals indicated by the data in the table to approximate the average height of the tree over the time interval 2 ≤ t ≤ 10.
(d) The height of the tree, in meters, can also be modeled by the function G, given by \(G(x)=\frac{100x}{1+x},\) where x is the diameter of the base of the tree, in meters. When the tree is 50 meters tall, the diameter of the base of the tree is increasing at a rate of 0.03 meter per year. According to this model, what is the rate of change of the height of the tree with respect to time, in meters per year, at the time when the tree is 50 meters tall?
Answer/Explanation
Ans:
(a) \(H'(6)\approx \frac{H(7)-H(5)}{7-5}=\frac{11-6}{2}=\frac{5}{2}\)
H′(6) is the rate at which the height of the tree is changing, in meters per year, at time t = 6 years.
(b) \(\frac{H(5)-H(3)}{5-3}=\frac{6-2}{2}=2\)
Because H is differentiable on 3 ≤ t ≤ 5, H is continuous on 3 ≤ t ≤ 5.By the Mean Value Theorem, there exists a value c, 3 < c < 5, such that H ‘(c) = 2.
(c) The average height of the tree over the time interval 2 ≤ t ≤ 10 is given by \(\frac{1}{10-2}\int_{2}^{10}H(t)dt.\)
\(\frac{1}{8}\int_{2}^{10}H(t)dt\approx \frac{1}{8}\left ( \frac{1.5+2}{2}\cdot 1+\frac{2+6}{2}\cdot 2+\frac{6+11}{2}\cdot 2+\frac{11+15}{2}\cdot 3 \right )\)
\(=\frac{1}{8}(65.75)=\frac{263}{32}\)
The average height of the tree over the time interval 2 ≤ t ≤ 10 is \(\frac{263}{32}\) meters.
(d) G(x) = 50 ⇒ x = 1
\(\frac{d}{dt}(G(x))=\frac{d}{dx}(G(x))\cdot \frac{dx}{dt}=\frac{(1+x)100-100x\cdot 1}{(1+x)^{2}}\cdot \frac{dx}{dt}=\frac{100}{(1+x)^{2}}\cdot \frac{dx}{dt}\)
\(\frac{d}{dt}(G(x))|_{x=1}=\frac{100}{(1+1)^{2}}\cdot 0.03=\frac{3}{4}\)
According to the model, the rate of change of the height of the tree with respect to time when the tree is 50 meters tall is \(\frac{3}{4}\) meter per year.
Question
The rate of change in temperature of a greenhouse from 7 p.m. to 7 a.m. is given by the function: \(f(t)=-3\sin \left ( \frac{t}{3} \right )\) where temperature is measured in degrees Fahrenheit and t is the number of hours after 7 p.m.
(A) If at 7 p.m. the temperature is 1058F, find the temperature of the greenhouse at 2 a.m.
(B) Write an integral expression to represent the temperature of the greenhouse at time t, where t is between 7 p.m. and 7 a.m.
(C) Find the average change in temperature of the greenhouse between 7 p.m. and 7 a.m. to the nearest 10th of a degree.
(D) Is there a point during the night when the rate of change it temperature of the greenhouse is the average change in temperature from part (c)? If yes, state the time. Either way, show all work to justify your answer.Ans:
Answer/Explanation
(A)F(0)=105,f(t) represents the rate of change of the temperature, and 2 a.m. is 7h after 7p.m.
\(F(7)=105+\int_{0}^{7}(-3\sin (t/3))dt\Rightarrow F(7)=105+9\cos (t/3)|^{7}_{0}\Rightarrow F(7)\approx 89.78\)
(B)\(F(t)=105+\int_{0}^{t}(-3\sin (x/3))dx\)
(C) Average value=\(\frac{1}{12-0}\int_{0}^{12}(-3\sin (t/3))dt\approx -1.24^{\circ}F/hour\)
(D) Since f(t) is continuous on [0, 12], by the Mean Value Theorem for integrals there
exists a number c in [0, 12] such that \(\int_{0}^{12}f(t)dt=f(c)(b-a)\)
You can use our solution from part (d) to show that f(c)=-1.24. Now solve for c.
\(f(c)=-1.24\Rightarrow -3\sin (t/3)=-1.24\Rightarrow \sin (t/3)=0.413\Rightarrow t/3=\sin ^{-1}(0.413)\Rightarrow t\approx 1.28\)
Approximately 1.28 h after 7 p.m., the rate of change of the temperature in the greenhouse equals the average rate of change of the temperature for the night. Now convert 0.28 to minutes to find the time.
\((0.28h)\times (60)=16.8min\approx 17 min\)
Question
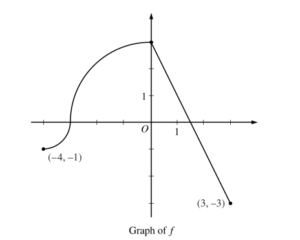
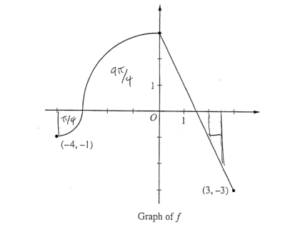
The continuous function f is defined on the interval \(-4\leq x\leq 3.\) The graph of f consists of two quarter circles and one line segment, as shown in the figure above. Let \(g(x)=2x+\int_{0}^{x}f(t)dt.\)
(a) Find g(-3). Find g’(x) and evaluate g’(-3).
(b) Determine the x-coordinate of the point at which g has an absolute maximum on the interval \(-4\leq x\leq 3.\)
(c) Find all values of x on the interval -4 < x < 3 for which the graph of g has a point of inflection. Give a reason for your answer. (d) Find the average rate of change of f on the interval \(-4\leq x\leq 3.\) There is no point c, -4 < c < 3, for which f’(c) is equal to that average rate of change. Explain why this statement does not contradict the Mean Value Theorem.
Answer/Explanation
Ans:
(a)
\(g(-3)=2\cdot (-3)+\int_{0}^{-3}f(t)dt=-6-\frac{9\pi }{4}\)
\(g'(x)=d/dx \left ( 2x+\int_{0}^{x}f(t)dt \right )=2+f(x)\)
g'(-3) = 2 + f(-3) = 2 + 0 = 2
(b)
g'(x) = 0 2 + f(x) = 0
f(x) = -2
x = 5/2
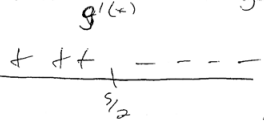
x = 5/2, because g’ check endpoints going from t to – proves it as the only relative maximum and g(5/2) is greater than g at either end point.
\(g(-4)= -8+\int_{0}^{-4}f(t)dt\)
= -8 – 2π
\(g(5/2)= 5+\int_{0}^{5/2}f(t)dt=5+\frac{5}{4}\)
\(g(3)= 6+\int_{0}^{3}f(t)dt=6\)
(c)
g”(x) = d/dx (g(x)) = f'(x)
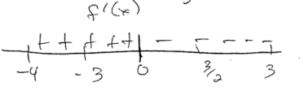
The only point of inflection for g is at x = 0, since f'(x), which is equivalent to g”, only changes signs at x = 0 on the interval -4 ≤ x ≤3
(d)
Avg. Rate of change = \(\frac{f(x)-f(-4)}{3-(-4)}\)
\(=\frac{-3–1}{3+4}=\frac{-2}{7}\)
Because Mean value Theorem only applies when the function is continuous AND differentiable on the interval, which doesn’t apply here since f(x) isn’t differentiable at x = 0.