Question
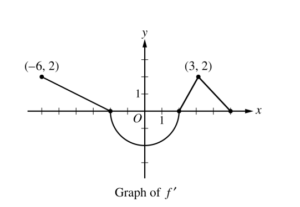
The function f is differentiable on the closed interval [−6, 5 ] and satisfies f (−2 )2 = 7. The graph of f ‘, the derivative of f, consists of a semicircle and three line segments, as shown in the figure above.
(a) Find the values of f (−6) and f (5).
(b) On what intervals is f increasing? Justify your answer.
(c) Find the absolute minimum value of f on the closed interval [−6, 5 ]. Justify your answer.
(d) For each of f “(−5) and f “(3), find the value or explain why it does not exist.
Answer/Explanation
Ans:
(a) \(f(-6)=f(-2)+\int_{-2}^{-6}f'(x)dx=7-\int_{-6}^{-2}f'(x)dx=7-4=3\)
\(f(5)=f(-2)+\int_{-2}^{5}f'(x)dx=7-2\pi +3=10-2\pi \)
(b) f ′ ( x) > 0 on the intervals [− 6, -2) and (2, 5). Therefore, f is increasing on the intervals [− 6, -2] and [2, 5] .
(c) The absolute minimum will occur at a critical point where f ‘ (x) = 0 or at an endpoint.
f ′( x) = 0 ⇒x = – 2, x = 2
| x | f(x) |
-6 -2 2 5 | 3 7 7-2π 10-2π |
The absolute minimum value is f (2) = 7 − 2π
(d) \(f”(-5)=\frac{2-0}{-6-(-2)}=-\frac{1}{2}\)
\(\lim_{x\rightarrow 3^{-}}\frac{f'(x)-f'(3)}{x-3}=2 and \lim_{x\rightarrow 3^{+}}\frac{f'(x)-f'(3)}{x-3}=-1\)
f ′′(3) does not exist because
\(\lim_{x\rightarrow 3^{-}}\frac{f'(x)-f'(3)}{x-3}\neq \lim_{x\rightarrow 3^{+}}\frac{f'(x)-f'(3)}{x-3}.\)
Question
Consider the differential equation \(\frac{dy}{dx}=2x – y.\)
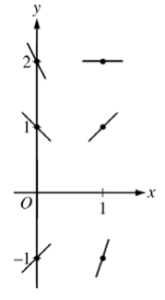
(a) On the axes provided, sketch a slope field for the given differential equation at the six points indicated.
(b) Find \(\frac{d^{2}y}{dx^{2}}\) in terms of x and y. Determine the concavity of all solution curves for the given differential equation in Quadrant II. Give a reason for your answer.
(c) Let y = f(x) be the particular solution to the differential equation with the initial condition f(2) = 3. Does f have a relative minimum, a relative maximum, or neither at x =2 ? Justify your answer.
(d) Find the values of the constants m and b for which y = mx + b is a solution to the differential equation.
Answer/Explanation
Ans:
(a) Slopes where x = 0
slopes where x = 1
(b) \(\frac{d^{2}y}{dx^{2}}= 2 – \frac{dy}{dx}= 2 -(2x-y)=2-2x+y\)
In Quadrant II, x < 0 and y > 0, so 2 – 2x + y > 0. Therefore, all solution curves are concave up in Quadrant II.
(c) \(\frac{dy}{dx}|_{(x,y)=(2,3)}=2(2)-3 = 1\neq 0\)
Therefore, f has neither a relative minimum nor a relative maximum at x = 2.
(d) \(y = mx + b \Rightarrow \frac{dy}{dx}=\frac{d}{dx}(mx + b)=m\)
2x – y = m
2x – (mx + b) = m
(2 – m)x – (m + b) = 0
2 – m = 0 ⇒ m = 2
b = -m ⇒ b = -2
Therefore, m = 2 and b = -2.
Question
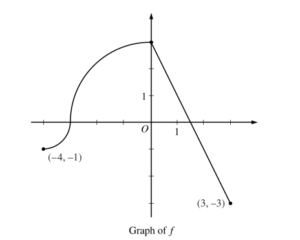
The continuous function f is defined on the interval \(-4\leq x\leq 3.\) The graph of f consists of two quarter circles and one line segment, as shown in the figure above. Let \(g(x)=2x+\int_{0}^{x}f(t)dt.\)
(a) Find g(-3). Find g’(x) and evaluate g’(-3).
(b) Determine the x-coordinate of the point at which g has an absolute maximum on the interval \(-4\leq x\leq 3.\)
(c) Find all values of x on the interval -4 < x < 3 for which the graph of g has a point of inflection. Give a reason for your answer. (d) Find the average rate of change of f on the interval \(-4\leq x\leq 3.\) There is no point c, -4 < c < 3, for which f’(c) is equal to that average rate of change. Explain why this statement does not contradict the Mean Value Theorem.
Answer/Explanation
Ans:
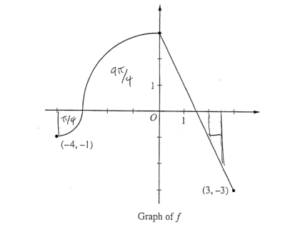
(a)
\(g(-3)=2\cdot (-3)+\int_{0}^{-3}f(t)dt=-6-\frac{9\pi }{4}\)
\(g'(x)=d/dx \left ( 2x+\int_{0}^{x}f(t)dt \right )=2+f(x)\)
g'(-3) = 2 + f(-3) = 2 + 0 = 2
(b)
g'(x) = 0 2 + f(x) = 0
f(x) = -2
x = 5/2
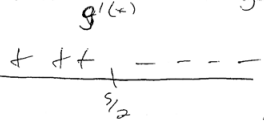
x = 5/2, because g’ check endpoints going from t to – proves it as the only relative maximum and g(5/2) is greater than g at either end point.
\(g(-4)= -8+\int_{0}^{-4}f(t)dt\)
= -8 – 2π
\(g(5/2)= 5+\int_{0}^{5/2}f(t)dt=5+\frac{5}{4}\)
\(g(3)= 6+\int_{0}^{3}f(t)dt=6\)
(c)
g”(x) = d/dx (g(x)) = f'(x)
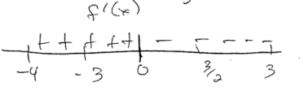
The only point of inflection for g is at x = 0, since f'(x), which is equivalent to g”, only changes signs at x = 0 on the interval -4 ≤ x ≤3
(d)
Avg. Rate of change = \(\frac{f(x)-f(-4)}{3-(-4)}\)
\(=\frac{-3–1}{3+4}=\frac{-2}{7}\)
Because Mean value Theorem only applies when the function is continuous AND differentiable on the interval, which doesn’t apply here since f(x) isn’t differentiable at x = 0.
Question

Let f be the continuous function defined on [-4, 3] whose graph, consisting of three line segments and a semicircle centered at the origin, is given above. Let g be the function given by \(g(x)=\int_{1}^{x}f(t)dt.\)
(a) Find the values of g(2) and g(-2).
(b) For each of g'(-3) and g”(-3) , find the value or state that it does not exist.
(c) Find the x-coordinate of each point at which the graph of g has a horizontal tangent line. For each of these points, determine whether g has a relative minimum, relative maximum, or neither a minimum nor a maximum at the point. Justify your answers.
(d) For -4 < x < 3, find all values of x for which the graph of g has a point of inflection. Explain your reasoning.
Answer/Explanation
Ans:
(a) \(g(2)=\int_{1}^{2}f(t)dt=-\frac{1}{2}(1)\left ( \frac{1}{2} \right )=-\frac{1}{4}\)
\(g(-2)=\int_{1}^{-2}f(t)dt=-\int_{-2}^{1}f(t)dt\)
\(= -\left ( \frac{3}{2}-\frac{\pi }{2} \right )=\frac{\pi }{2}-\frac{3}{2}\)
(b) \(g'(x)=f(x)\Rightarrow g'(-3)=f(-3)=2\)
\(g”(x)=f'(x)\Rightarrow g”(-3)=f'(-3)=1\)
(c) The graph of g has a horizontal tangent line where gx f'(x) = f(x) = 0. This occurs at x = −1 and x = 1.
g′(x ) changes sign from positive to negative at x = −1. Therefore, g has a relative maximum at x = −1.
g′(x) does not change sign at x = 1. Therefore, g has neither a relative maximum nor a relative minimum at x = 1.
(d) The graph of g has a point of inflection at each of x = −2, x = 0, and x = 1 because g′′(x) = f'(x) changes sign at each of these values.