Question
At time t≥ 0 , a particle moving in the xy-plane has velocity vector given by \(v(t)=\left \langle 3,2^{-t^{2}} \right \rangle\). If the particle is at the point \((1,\frac{1}{2})\) at time t= 0 , how far is the particle from the origin at time t= 1 ?
A 2.304
B 3.107
C 4.209
D 5.310
Answer/Explanation
Question
If a particle moves in the xy-plane so that at time t > 0 its position vector is \(\left ( ln(t^{2}+2t),2t^{2} \right )\), then at time t = 2, its velocity vector is
(A)\(\left ( \frac{3}{4},8 \right )\)
(B)\(\left ( \frac{3}{4},4 \right )\)
(C)\(\left ( \frac{1}{8},8 \right )\)
(D)\(\left ( \frac{1}{8},4 \right )\)
(E)\(\left (- \frac{5}{16},4 \right )\)
Answer/Explanation
Ans:A
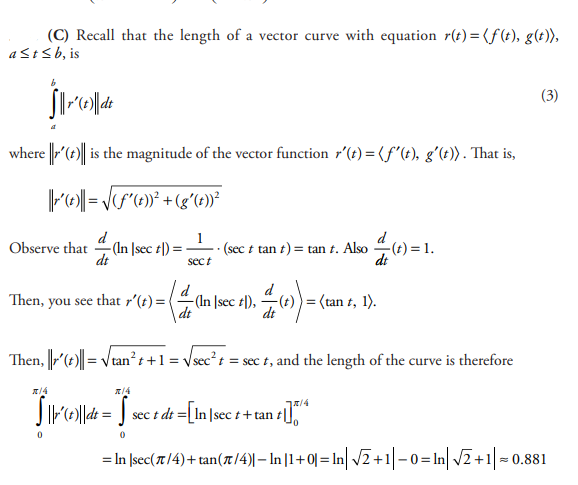
Question
Find the length of the vector curve \(r(t)=\left \langle \ln \left | \sec t \right | ,t\right \rangle\) from t = 0 to \(t=\frac{\pi }{4}\)
(A)\(\ln \left | 1-\frac{1}{\sqrt{2}} \right |\)
(B) \(\ln \left | \sqrt{5}-2\right |\)
(C) \(\ln \left | \sqrt{2}+1\right |\)
(D)\(\ln \left | 2-\sqrt{2}\right |\)
Answer/Explanation
Ans:(C)