Question
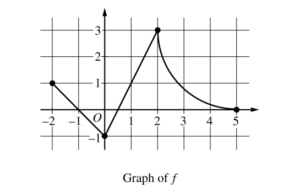
The continuous function f is defined on the closed interval −6 ≤ x ≤ 5. The figure above shows a portion of the graph of f, consisting of two line segments and a quarter of a circle centered at the point (5, 3). It is known that the point \(\left ( 3,3-\sqrt{5} \right )\) is on the graph of f.
(a) If \(\int_{-6}^{5}f(x)dx=7,\) find the value of \(\int_{-6}^{-2}f(x)dx\) Show the work that leads to your answer.
(b) Evaluate \(\int_{3}^{5}(2f'(x)+4)dx.\)
(c) The function g is given by \(\int_{-2}^{x}f(t)dt.\) Find the absolute maximum value of g on the interval − ≤ x ≤ 52 . Justify your answer.
(d) Find \(\lim_{x\rightarrow 1}\frac{10^{x}-3f'(x)}{f(x)-arctan x}.\)
Answer/Explanation
Ans:
(a) \(\int_{-6}^{5}f(x)dx=\int_{-6}^{-2}f(x)dx+\int_{-2}^{5}f(x)dx\)
\(\Rightarrow 7=\int_{-6}^{-2}f(x)dx+2+\left ( 9-\frac{9\pi }{4} \right )\)
\(\Rightarrow \int_{-6}^{-2}f(x)dx=7-\left ( 11-\frac{9\pi }{4} \right )=\frac{9\pi }{4}-4\)
(b) \(\int_{3}^{5}(2f'(x)+4)dx=2\int_{3}^{5}f'(x)+\int_{3}^{5}4dx\)
= 2 (f(5)- f(3)) + 4 (5-3)
\(=2(0-(3-\sqrt{5}))+8\)
\(=2(-3+\sqrt{5})+8=2+2\sqrt{5}\)
-OR-
\(\int_{3}^{5}(2f'(x)+4)dx=_{x=3}[2f(x)+4x]^{x=5}\)
=(2f(5) + 20) – (2 f(3) + 12)
\(=(2.0+20)-(2(3-\sqrt{5})+12)\)
\(=2+2\sqrt{5}\)
(c) \(g'(x)=f(x)=0\Rightarrow x=-1, x=\frac{1}{2}, x=5\)
| x | g(x) |
-2 -1 \(\frac{1}{2}\) 5 | 0 \(\frac{1}{2}\) \(-\frac{1}{4}\) \(11-\frac{9\pi }{4}\) |
On the interval -2 ≤ x ≤ 5, the absolute maximum value of g is g(5) = \(11-\frac{9\pi }{4}.\)
(d) \(\lim_{x\rightarrow 1}\frac{10^{x}-3f'(x)}{f(x)-arctan x}=\frac{10^{1}-3f'(1)}{f(1)-arctan 1}\)
\(=\frac{10-3.2}{1-arctan 1}=\frac{4}{1-\frac{\pi }{4}}\)
Question
| x | f(x) | f'(x) | g(x) | g'(x) |
| 1 | -6 | 3 | 2 | 8 |
| 2 | 2 | -2 | -3 | 0 |
| 3 | 8 | 7 | 6 | 2 |
| 6 | 4 | 5 | 3 | -1 |
The functions f and g have continuous second derivatives. The table above gives values of the functions and their derivatives at selected values of x.
(a) Let k(x) = f(g(x)). Write an equation for the line tangent to the graph of k at x = 3.
(b) Let \(h(x)=\frac{g(x)}{f(x)}.\) Find h'(1).
(c) Evaluate \(\int_{1}^{3}f”(2x)dx.\)
Answer/Explanation
Ans:
(a) k(3) = f(g(3)) = f(6) = 4
k'(3) = f'(g(3))·g'(3) = f'(6)·2=5·2 =10
An equation for the tangent line is y = 10(x -3) +4.
(b) \(h'(1)=\frac{f(1)\cdot g'(1)-g(1)\cdot f'(1)}{(f(1))^{2}}\)
\(=\frac{(6)\cdot 8-2\cdot 3}{(-6)^{2}}=\frac{-54}{36}=-\frac{3}{2}\)
(c) \(\int_{1}^{3}f”(2x)dx=\frac{1}{2}\left [ f'(2x) \right ]_{1}^{3}=\frac{1}{2}\left [ f'(6)-f'(2) \right ]\)
\(=\frac{1}{2}\left [ 5-(-2) \right ]=\frac{7}{2}\)
Question
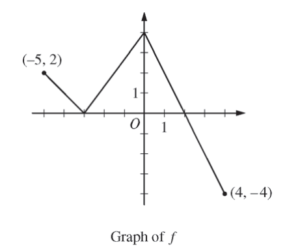
The function f is defined on the closed interval [5, 4] . The graph of f consists of three line segments and is shown in the figure above. Let g be the function defined by \(g(x)=\int_{-3}^{x}f(t)dt.\)
(a) Find g(3).
(b) On what open intervals contained in -5 < x < 4 is the graph of g both increasing and concave down? Give a reason for your answer.
(c) The function h is defined by \(h(x)=\frac{g(x)}{5x}.\) Find h'(3).
(d) The function p is defined by \(p(x)=f(x^{2}-x).\) Find the slope of the line tangent to the graph of p at the point where x = -1.
Answer/Explanation
Ans:
(a) \(g(3)=\int_{-3}^{3}f(t)dt=6+4-1=9\)
(b) g′(x) = f ( x)
The graph of g is increasing and concave down on the intervals − 5< x < -3 and 0 < x < 2 because g′ = f is positive and decreasing on these intervals.
(c) \(h'(x)=\frac{5xg'(x)-g(x)5}{(5x)^{2}}=\frac{5xg'(x)-5g(x)}{25x^{2}}\)
\(h'(3)=\frac{(5)(3)g'(3)-5g(3)}{25.3^{2}}\)
\(=\frac{15(-2)-5(9)}{225}=\frac{-75}{225}=-\frac{1}{3}\)
(d) p'(x) = f'(x2 – x) (2x – 1)
p’ (-1) = f'(2) (-3) = (-2) (-3) = 6
