Question

A student is given the task of determining the I content of tablets that contain KI and an inert, water-soluble sugar as a filler. A tablet is dissolved in 50.0 mL of distilled water, and an excess of 0.20 M\( Pb(NO_3)_2\)(aq) is added to the solution. A yellow precipitate forms, which is then filtered, washed, and dried. The data from the experiment are shown in the table above.
(a) For the chemical reaction that occurs when the precipitate forms, (i) write a balanced, net-ionic equation for the reaction, and
(ii) explain why the reaction is best represented by a net-ionic equation.
(b) Explain the purpose of drying and weighing the filter paper with the precipitate three times.
(c) In the filtrate solution, is [K] greater than, less than, or equal to Justify \([NO_{3}^{-}]\)? your answer.
(d) Calculate the number of moles of precipitate that is produced in the experiment.
(e) Calculate the mass percent of I in the tablet.
(f) In another trial, the student dissolves a tablet in 55.0 mL of water instead of 50.0 mL of water. Predict whether the experimentally determined mass percent of I will be greater than, less than, or equal to the amount calculated in part
(e). Justify your answer.
g) A student in another lab also wants to determine the I content of a KI tablet but does not have access to Pb\((NO_3)_2\). However, the student does have access to 0.20 M\( AgNO_3\), which reacts with I ̄(aq) to produce AgI(s). The value of Kp for Agl is 8.5 × 10−17.
(i) Will the substitution of \(AgNO_3\) for \(Pb(NO_3)_2\) result in the precipitation of the I- ion from solution? Justify your answer.
(ii) The student only has access to one KI tablet and a balance that can measure to the nearest 0.01 g. Will the student be able to determine the mass of Agl produced to three significant figures? Justify your answer.
▶️Answer/Explanation
a.(i) \(Pb^{2+} + 2I^{-} → Pbl_{2}\)
a.(ii) The net-ionic equation shows the formation of the\( Pbl_{2}\)(s) from \(Pb^{2+}\)(aq) and \(I^{+}\)(aq) ions, omitting the non-reacting species (spectator ions), \(K^{+}\)(aq) and \(NO_{3} ̄\)(aq).
(b) The filter paper and precipitate must be dried several times (to a constant mass) to ensure that all the water has been driven off.
(c) [\(K^{+}\)] is less than \([NO_{3}\)] because the source of the\( NO_{3}^{-}\), the 0.20 M Pb(NO_{3})_{2}(aq), was added in excess.
(d)1.698 g 1.462 g=0.236 g Pbl2(s)
\(0.236g Pbl_{2}\frac{1molPbl_{2}}{461.0gPbl_{2}}=5.12\times 10^{-4} mol Pbl_{2}\)
(e) \(5.12 × 10^{-4}mol Pbl_{2} ×\frac{2 mol I^{-}}{1 mol Pbl_{2}}\)= 1.02 × 10^{-3} mol I^{-1}\)
\(1.02 x 10^{3}mol I ̄ ×\frac{126.91 g I^{-}}{1 mol I^{-}}=0.130 g I^{-}\) in one tablet
\(\frac{0.130 g I^{-}} {0.425 g KI tablet}\)= 0.306 30.6% per KI tablet
(f) The mass percent of\( I^{-}\) will be the same. \(Pb^{2+}\)(aq) was added in excess, ensuring that essentially no I remained in solution. The additional water is removed by filtration and drying, leaving the same mass of dried precipitate.
g (i) Yes. Addition of an excess of 0.20 M \(AgNO_{3}\)(aq) will precipitate all of the I- ion present in the solution because Agl is insoluble, as evidenced by its low value of \(K_{sp}\)
g.(ii) No. If masses can be measured to ±0.01 g, then the mass of the dry Agl(s) precipitate (which is less than 1 g) will be known to only two significant figures.
Question
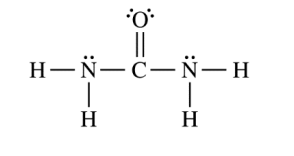
The compound urea, H2NCONH2 , is widely used in chemical fertilizers. The complete Lewis electron-dot diagram for the urea molecule is shown above.
(a) Identify the hybridization of the valence orbitals of the carbon atom in the urea molecule.
(b) Urea has a high solubility in water, due in part to its ability to form hydrogen bonds. A urea molecule and four water molecules are represented in the box below. Draw ONE dashed line (—-) to indicate a possible location of a hydrogen bond between a water molecule and the urea molecule.

The dissolution of urea is represented by the equation above. A student determines that 5.39 grams of H2NCONH2 (molar mass 60.06 g/mol) can dissolve in water to make 5.00 mL of a saturated solution at 20.°C.
(c) Calculate the concentration of urea, in mol/L, in the saturated solution at 20.°C.
(d) The student also determines that the concentration of urea in a saturated solution at 25°C is 19.8 M. Based on this information, is the dissolution of urea endothermic or exothermic? Justify your answer in terms of Le Chatelier’s principle.
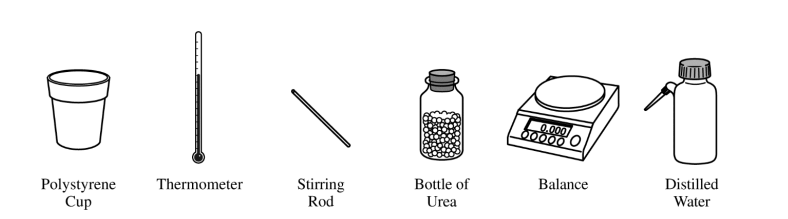
(e) The equipment shown above is provided so that the student can determine the value of the molar heat of solution for urea. Knowing that the specific heat of the solution is 4.18 J/(g⋅°C), list the specific measurements that are required to be made during the experiment.
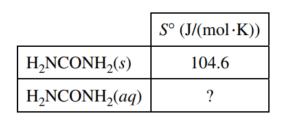
(f) The entropy change for the dissolution of urea, ΔS0soln , is 70.1 J/(mol⋅K) at 25°C. Using the information in the table above, calculate the absolute molar entropy, S°, of aqueous urea.
(g) Using particle-level reasoning, explain why ΔS0soln is positive for the dissolution of urea in water.
(h) The student claims that ΔS° for the process contributes to the thermodynamic favorability of the dissolution of urea at 25°C. Use the thermodynamic information above to support the student’s claim.
▶️Answer/Explanation
Ans:
(a)
| sp2 |
(b)
| A dashed line should connect a hydrogen atom in water to a nitrogen or oxygen atom in urea or an oxygen atom in water to a hydrogen atom in urea. One possible correct response is shown above. |
(c)
5.39 g H2NCONH2 × \(\frac{1 mol}{60.06 g}=0.0897 mol\) \(\frac{0.0897 mol}{0.00500 L}=17.9 M\) |
(d)
| The increased solubility at the higher temperature implies that the dissolution of urea is endothermic. If a saturated solution of urea is heated, then the equilibrium system is stressed. The stress is counteracted by the endothermic dissolution of more urea. |
(e)
| mass of urea, mass of water, initial temperature of water, final temperature of solution |
(f)
ΔS0soln = S0 (H2NCONH2(aq)) – S0 (H2NCONH2(s)) 70.1 J/(mol.K) = S0 (H2NCONH2(aq)) – 104.6 J/(mol.K) S0 (H2NCONH2(aq)) = 174.7 J/(mol.K) |
(g)
| Urea molecules in solution have a greater number of possible arrangements than in solid urea. This increased number of arrangements corresponds to a positive ΔS0soln. |
(h)
| Thermodynamic favorability for a process at standard conditions is determined by the sign of ΔG0 , with ΔG0 =ΔH0 -TΔS0 . Since ΔS0 is positive, the TΔS0 term makes the value of ΔG0 smaller and thus makes the dissolution more thermodynamically favorable. |
