Question
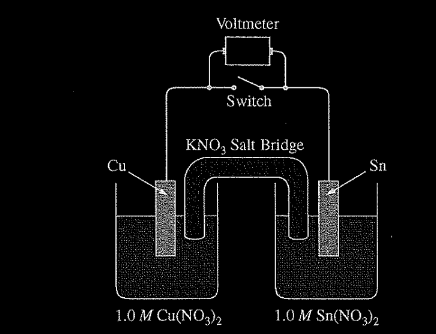
A student is given a standard galvanic cell, represented above, that has a Cu electrode and a Sn electrode. As current flows through the cell, the student determines that the Cu electrode increases in mass and the Sn electrode decreases in mass.
(a) Identify the electrode at which oxidation is occurring. Explain your reasoning based on the student’s observations.
(b) As the mass of the Sn electrode decreases, where does the mass go?
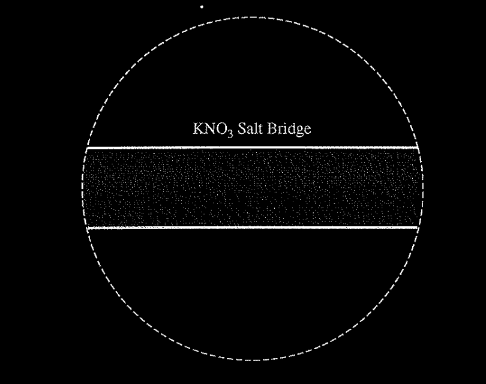
(c) In the expanded view of the center portion of the salt bridge shown in the diagram below, draw and label a particle view of what occurs in the salt bridge as the cell begins to operate. Omit solvent molecules and use arrows to show the movement of particles.
d) A nonstandard cell is made by replacing the 1.0 M solutions of Cu\((NO_3)_2\) and Sn\((NO_3)_2\) in the standard cell with 0.50 M solutions of Cu(NO3)2 and Sn\((NO_{3})_{2}\). The volumes of solutions in the nonstandard cell are identical to those in the standard cell.
(i) Is the cell potential of the nonstandard cell greater than, less than, or equal to the cell potential of the standard cell? Justify your answer.
(ii) Both the standard and nonstandard cells can be used to power an electronic device. Would the nonstandard cell power the device for the same time, a longer time, or a shorter time as compared with the standard cell? Justify your answer.
(e) In another experiment, the student places a new Sn electrode into a fresh solution of 1.0 M\( Cu(NO_{3})_{2}\) ·
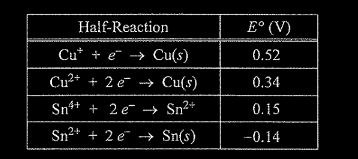
(i) Using information from the table above, write a net-ionic equation for the reaction between the Sn electrode and the Cu\((NO_3)_2\) solution that would be thermodynamically favorable. Justify that the reaction is thermodynamically favorable.
(ii) Calculate the value of \(\Delta G\) for the reaction. Include units with your answer.
▶️Answer/Explanation
(a) Since the Sn electrode is losing mass, Sn atoms must be forming \(Sn^{2+}\)(aq). This process is oxidation. OR because the cell operates, E° must be positive and, based on the E° values from the table, it must be Sn that is oxidized.
(b) The atoms on the Sn electrode are going into the solution as \(Sn^{2+}\) ions.
(c) The response should show at least one \(K^+\) ion moving toward the Cu compartment on the left and at least one \(NO_{3}^-\) ion moving in the opposite direction.
d(i) It is the same. In the cell reaction \(Q= \frac{[Sn^{2+}]}{ [Cu^{2+}]}\) , and the concentrations of \(Sn^{2+}\) and \(Cu^{2+}\) are equal to each other in both cases.
d(ii) The nonstandard cell would power the device for a shorter time because the supply of \(Cu^{2+}\) ions will be exhausted more quickly. OR The nonstandard cell would power the device for a shorter time because the reaction will reach E=0 more quickly.
e (i) \(Cu^{2+}(aq) + Sn(s) → Cu(s) + Sn^{2+}\)(aq) E° is positive \((0.34 V + 0.14 V = 0.48 V)\), therefore the reaction is thermodynamically favorable. OR The cell observations from earlier parts of the question are evidence that the Sn is oxidized and Cu is reduced, therefore E° must be positive.
e.(ii) \(\Delta G^0=-nFE^0\)
\(\Delta G^0=-\frac{2 mol e^-}{mol_{rxn}}\times \frac{96,485}{mol e^-}\times \frac{0.48}{c}=-93,000 J/mol_{rxn}\)
Question.
\(H_{3}BO_{3}(aq)+4HF(g)\rightarrow H_{3}O^{+}(aq)+BF_{4}^{-}(aq)+2H_{2}O(l)\)
Tetrafluoroboric acid is a strong acid with the formula\( HBF_4\). The acid can be prepared by reacting the weak acid \(H_3BO_3\) (molar mass 61.83 g/mol) with HF according to the equation above.
(a) To prepare a solution of \(BF_4^{-}\)(aq), HF(g) is bubbled into a solution containing 50.0 g of\( H_3BO_3\) in a 1 L reaction vessel.
(i) Calculate the maximum number of moles of\( BF_4^{-}\)(aq) that can be produced.
(ii) Calculate the number of liters of HF(g), measured at 273 K and 1.00 atm, that will be consumed if all the\( H_3BO_3\) reacts.
(iii) Will the pH of the solution increase, decrease, or remain the same during the course of the reaction? Justify your answer. In another experiment, a 0.150 M \(BF_4^-\)(aq) solution is prepared by dissolving \(NaBF_4\)(s) in distilled water. The\( BF_{4}^-\)(aq) ions in the solution slowly react with H2O(l) in the reversible reaction represented below.
\(BF_4^-(aq)+H_{2}O(l)\rightleftharpoons BF_{3}OH^{-}(aq)+HF(aq)\)
The concentration of HF is monitored over time, as shown in the graph below.

[HF] reaches a constant value of 0.0174 M when the reaction reaches equilibrium. For the forward reaction, the rate law is rate = kf \([BF_4^{-}\)]. The value of the rate constant kf was experimentally determined to be \(9.00\times 10^{-4}min^{-1}\).
(b) Calculate the rate of the forward reaction after 600. minutes. Include units with your answer. The rate law for the reverse reaction is rate = \(kr [BF_3OH^-]\)[HF].
(c) A student claims that the initial rate of the reverse reaction is equal to zero. Do you agree or disagree with this claim? Justify your answer in terms of the rate law for the reverse reaction.
(d) At equilibrium the forward and reverse reaction rates are equal. Calculate the value of the rate constant for the reverse reaction.
\(H_{3}BO_{3}(aq)+4HF(g)\rightarrow H_{3}O^{+}(aq)+BF_{4}^{-}(aq)+2H_{2}O(l)\)
Tetrafluoroboric acid is a strong acid with the formula \(HBF_4\). The acid can be prepared by reacting the weak acid \(H_3BO_3\) (molar mass 61.83 g/mol) with HF according to the equation above.
▶️Answer/Explanation
a(i) \(50.0gH_{3}BO_{3}\times \frac{1molH_{3}BO_{3}}{61.83g}\times \frac{1molBF_{4}^{-}}{1molH_{3}BO_{3}}\)
a(ii)\( 0.809 mol \times 4 = 3.24 mol\)
PV= nRT
\(V=\frac{nRT}{p}=\frac{(3.24mol)(0.08206Latm mol^{-1}K^{-1})(273K)}{1.00atm}\)
=72.6L
OR
\(0.809 mol \times 4 = 3.24 mol\)
\(\frac{22.4L}{1mol}\times 3.24mol=72.6 L\)
a(iii) As the reaction proceeds,\( H_3O^{+}\) is produced, so the pH
will decrease.
(b) \([BF_4^{-}] = 0.150 M – 0.0174 M = 0.133 M\)
\(rate = (9.00\times 10^{-4}min^{-1})(0.133M)=1.20\times 10^{-4}M min^{-1}\)
(c) Agree. The initial concentration of each product is zero, so the initial rate of the reverse reaction is zero.
(d ) 
Question
A student is studying the properties of CaSO4 and PbSO4. The student has samples of both compounds, which are white powders.
(a) The student tests the electrical conductivity of each solid and observes that neither solid conducts electricity. Describe the structures of the solids that account for their inability to conduct electricity.
The student places excess CaSO4 (s) in a beaker containing 100 mL of water and places excess PbSO4 (s) in another beaker containing 100 mL of water. The student stirs the contents of the beakers and then measures the electrical conductivity of the solution in each beaker. The student observes that the conductivity of the solution in the beaker containing the CaSO4 (s) is higher than the conductivity of the solution in the beaker containing the PbSO4 (s).
(b) Which compound is more soluble in water, CaSO4(s) or PbSO4(s) ? Justify your answer based on the results of the conductivity test.
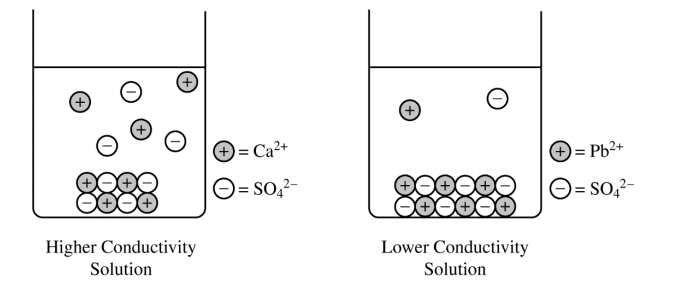
The left side of the diagram below shows a particulate representation of the contents of the beaker containing the CaSO4(s) from the solution conductivity experiment.
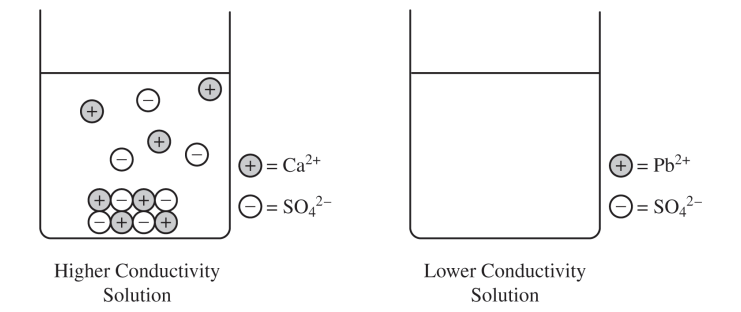
(c) Draw a particulate representation of PbSO4(s) and the ions dissolved in the solution in the beaker on the right in the diagram. Draw the particles to look like those shown to the right of the beaker. Draw an appropriate number of dissolved ions relative to the number of dissolved ions in the beaker on the left.
(d) The student attempts to increase the solubility of CaSO4(s) by adding 10.0 mL of 2 M H2SO4 (aq) to the beaker, and observes that additional precipitate forms in the beaker. Explain this observation.
▶️Answer/Explanation
Ans:
(a) For a correct description:
Ionic solids do not have free-moving ions that are required to carry an electric current. Therefore, there is no conduction of electricity.
(b) For the correct answer and a valid justification:
CaSO4. The greater electrical conductivity of the CaSO4 solution relative to the PbSO4 solution implies a higher concentration of ions, which comes from the dissolution (dissociation) of CaSO4 to a greater extent.
(c) For a correct drawing that shows an equal number of cations and anions:
The drawing shows solid PbSO4 at the bottom of the beaker (similar to the solid shown for CaSO4) and fewer dissociated Pb2+ and SO42− ions in the solution.
(d) For a correct explanation:
The additional precipitate is CaSO4 that forms in response to the increased [SO42−] in solution. According to Le Chatelier’s principle (Q > Ksp), the introduction of SO42− as a
common ion shifts the equilibrium towards the formation of more CaSO4(s).
