Question
Aluminum metal can be recycled from scrap metal by melting the metal to evaporate impurities.
(a) Calculate the amount of heat needed to purify 1.00 mole of Al originally at 298 K by melting it. The melting point of Al is 933 K. The molar heat capacity of Al is 24 J/(mol⋅K), and the heat of fusion of Al is 10.7 kJ/mol.
(b) The equation for the overall process of extracting Al from Al2O3 is shown below. Which requires less energy, recycling existing Al or extracting Al from Al2O3 ? Justify your answer with a calculation.
\(Al_{2}O_{3}(s)\rightarrow 2 Al(s)+\frac{3}{2}O_{2}(g)\) \(\Delta H^{0}=1675 kJ/mol_{rxn}\)
▶️Answer/Explanation
Ans:
(a)
To raise the temperature from 298 K to 933 K: \(q = \frac{24 J}{mol K}\times 1.00 mol\times 635 K=15,000 J = 15 kJ\) It takes 10.7 kJ to melt the Al at 933 K. 15 kJ + 10.7 kJ = 26 kJ |
(b)
For extracting Al from ore: \(1675 kJ/mol_{rxn}\times \frac{1 mol of reaction}{2 mol Al}=837.5 kJ\) per mol of Al producing 1.00 mol of Al from \(Al_2O_3\) requires 837.5 kJ. Because 26 kJ < 837.5 kJ, recycling requires less energy. |
Question
The half-life (t1/2) of the catalyzed isomerization of cis-2-butene gas to produce trans-2-butene gas, represented above, was measured under various conditions, as shown in the table below.
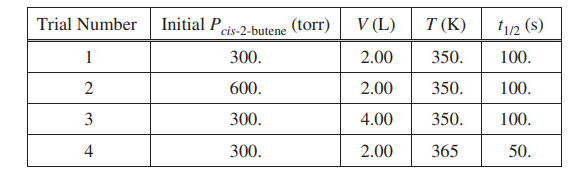
(a) The reaction is first order. Explain how the data in the table are consistent with a first-order reaction.
(b) Calculate the rate constant, k, for the reaction at 350. K. Include appropriate units with your answer.
(c) Is the initial rate of the reaction in trial 1 greater than, less than, or equal to the initial rate in trial 2? Justify your answer.
(d) The half-life of the reaction in trial 4 is less than the half-life in trial 1. Explain why, in terms of activation energy.
▶️Answer/Explanation
Ans:
(a)
| For a first-order reaction, the half-life is independent of reactant concentration (or pressure) at constant T, as shown in trials 1, 2, and 3. |
(b)
| \(k = \frac{0.693}{t_{1/2}}=\frac{0.693}{100.s}=0.00693 s^{-1}\) |
(c)
| The initial rate in trial 1 is less than that in trial 2 because rate = k [cis-2-butene] or rate = kPcis-2-butene (with reference to values from both trials). OR because the initial concentration of cis-2-butene in trial 1 is less than that in trial 2 and k is constant |
(d)
| The temperature is higher in trial 4, meaning that the KEavg of the molecules is greater. Consequently, in this trial a greater fraction of collisions have sufficient energy to overcome the activation energy barrier, thus the rate is greater. |
