Question
A mechanical wheel initially at rest on the floor begins rolling forward with an angular acceleration of 2π rad/\(s^{2}\). If the wheel has a radius of 2 m, what distance does the wheel travel in 3 seconds?
(A) 4π m
(B) 6π m
(C) 16π m
(D) 18π m
▶️Answer/Explanation
Ans:D
Apply Big Five #3 for rotational motion:
\(\theta =\theta _{0}\omega _{0}t+\frac{1}{2}at^{2}=\frac{1}{2}(2\pi rad/s^{2})(3s)^{2}=9\pi rad\)
The circumference of the circle d = 2πr = 2π(2 m) = 4π m. 9π rad is equivalent to 4.5 rotations and a total distance traveled of 4.5 ∙ 4π m = 18π m.
Question
A uniform stick has length L. The moment of inertia about the center of the stick is Io. A particle of mass M is attached to one end of the stick. The moment of inertia of the combined system about the center of the stick is
(A) \(I_{0} + \frac{1}{4}ML^{2}\) (B) \(I_{0} + \frac{1}{2}ML^{2}\) (C) \(I_{0} + \frac{3}{4}ML^{2}\) (D) \(I_{0}+ML^{2}\)
▶️Answer/Explanation
Ans:A
Solution: Itot = \(\sum \)I = I0 + IM = I0 + M(½L)2
Question
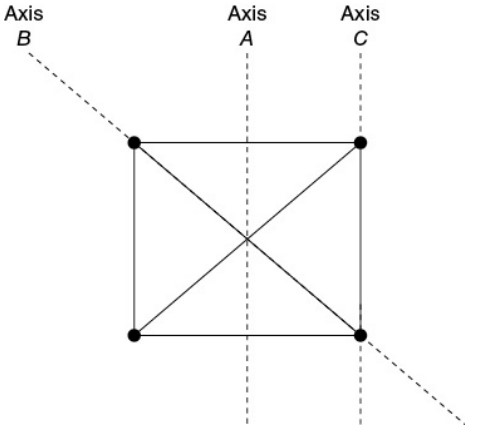
Four identical lead balls with large mass are connected by rigid but very light rods in the square configuration shown in the preceding figure. The balls are rotated about the three labeled axes. Which of the following correctly ranks the rotational inertia I of the balls about each axis?
(A) \(I_{B}>1_{A}=1_{C}\)
(B) \(I_{A}>1_{C}=1_{B}\)
(C) \(I_{C}>1_{A}>1_{B}\)
(D)\(I_{C}>1_{A}=1_{B}\)
▶️Answer/Explanation
Ans:D
The rotational inertia of a point mass is \(MR^{2}\), where \(R\) is the distance from the mass to the axis of rotation. Pretend the side of the square is of length 2 m, and that each mass is 1 kg. For axis A, each mass has rotational inertia \((1kg)(1m)^{2}=1kg\cdot m^{2}\). With four masses total, that’s \(4kg\cdot m^{2}\). For axis B, each mass is \(\sqrt{2}m\) from the axis (the diagonal of the square is \(2\sqrt{2}m\), each mass is half a diagonal from the axis). Each mass has \((1kg)(\sqrt{2}m)^{2}=2kg\cdot m^{2}\). Two masses make a total of \(4kg\cdot m^{2}\). And for axis C, the masses are each 2 m from the axis, so they each have \((1kg)\times(2m)^{2}=4kg\cdot m^{2}\). With two masses, that’s a total of \(8kg\cdot m^{2}\). So this would be ranked axis C, followed by equal axes A and B.
Question
Which of the following must be true for an object at translational equilibrium? Select two answers.
(A) The kinetic energy of the object is 0.
(B) The net force on the object is 0.
(C) The acceleration of the object is 0.
(D) The net torque on the object is 0.
▶️Answer/Explanation
Ans:B, C
An object is defined to be in translational equilibrium of the sum of the forces acting on it is zero. If the net force on the object is equal to 0, the object’s acceleration is also 0. An object at translational equilibrium can have a nonzero kinetic energy and net torque.
Question

Three masses are attached to a 1.5 m long massless bar. Mass 1 is 2 kg and is attached to the far left side of the bar. Mass 2 is 4 kg and is attached to the far right side of the bar. Mass 3 is 4 kg and is attached to the middle of the bar. At what distance from the far left side of the bar can a string be attached to hold the bar up horizontally?
(A) 0.3 m
(B) 0.6 m
(C) 0.9 m
(D) 1.2 m
▶️Answer/Explanation
Ans:C
The position that the string can be attached to hold the bar up horizontally is the center of mass of the bar, which can be calculated using the center of mass equation with the far left side as the origin:
\(x(c.m)=\frac{x_{1}m_{1}+x_{2}m_{2}+x_{3}m_{3}}{m_{1}+m_{2}+m_{3}}=\frac{0m(2kg)+1.5m(4kg)+0.75m(4kg)}{2kg+4kg+4kg}\)
\(=\frac{9}{10}m=0.9m\)
