Question
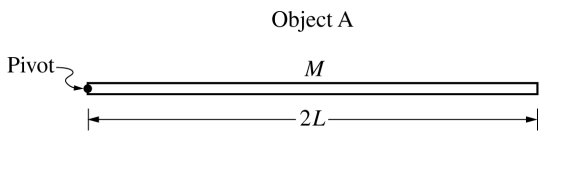
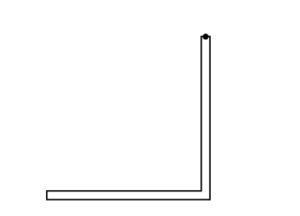
Object A is a long, thin, uniform rod of mass M and length 2L that is free to rotate about a pivot of negligible friction at its left end, as shown above.
(a) Using integral calculus, derive an expression to show that the rotational inertia IA of object A about the pivot is given by \(\frac{4}{3}ML^{2}\) .
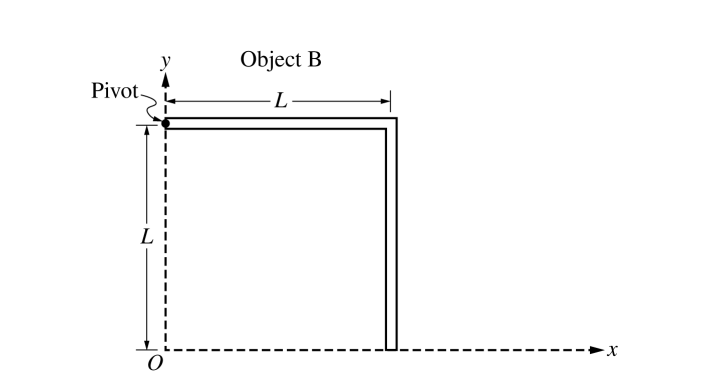
Object B of total mass M is formed by attaching two thin, uniform, identical rods of length L at a right angle to each other. Object B is held in place, as shown above. Express your answers in part (b) in terms of L .
(b) Determine the following for the given coordinate system shown in the figure.
i. The x-coordinate of the center of mass of object B
ii. The y-coordinate of the center of mass of object B
Object B has a rotational inertia of IB about its pivot.
(c) Is the value of IB greater than, less than, or equal to IA ?
_____ Greater than _____ Less than _____ Equal to
Justify your answer.
Object B is released from rest and begins to rotate about its pivot.
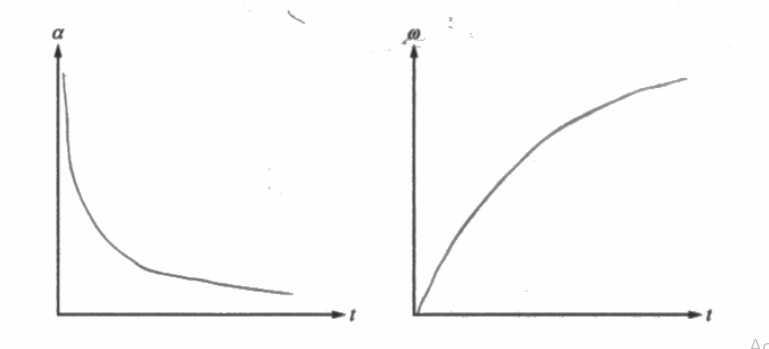
(d) On the axes below, sketch graphs of the magnitude of the angular acceleration α and the angular speed ω of object B as functions of time t from the time it is released to the time its center of mass reaches its lowest point.
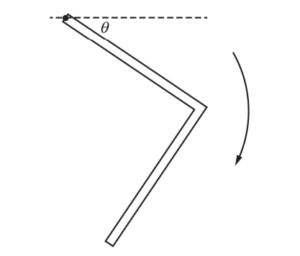
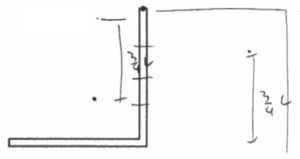
(e) While object B rotates from the horizontal position down through the angle θ shown above, is the magnitude of its angular acceleration increasing, decreasing, or not changing?
_____ Increasing _____ Decreasing _____ Not changing
Justify your answer.
Object B rotates through the position shown above.
(f) Derive an expression for the angular speed of object B when it is in the position shown above. Express your answer in terms of M , L , IB , and physical constants, as appropriate.
Answer/Explanation
Ans:
(a)
I = MR2 \(dm = \frac{m}{2L}dx\)
dI = dmr2 \(I = \int_{0}^{2L}\frac{m}{2L}r^{2}dr\)
\(I = \frac{m}{2L}\int_{0}^{2L}r^{2}dr\)
\(I = \frac{m}{2L}_{4}^{0}\textrm{}\left ( \frac{1}{5}r ^{3}|_{0}^{2L}\right )\)
\(I = \frac{m8L^{2}}{6L}=\frac{4}{3}ML^{2}\)
(b)
i. Each bunch mass m/2
\(X_{am}=\frac{\sum x_{i}m_{i}}{m_{total}}=\frac{\left ( \frac{L}{2} \right )\left ( \frac{M}{2} \right )+L\left ( \frac{M}{2} \right )}{m}=\frac{\frac{3ml}{4}}{m}=\frac{3}{4}L\)
ii.
\(Y_{am}=\frac{\sum x_{i}m_{i}}{m_{total}}=\frac{\left ( \frac{L}{2} \right )\left ( \frac{M}{2} \right )+L\left ( \frac{M}{2} \right )}{m}=\frac{\frac{3ml}{4}}{m}=\frac{3}{4}L\)
(c)
√ Less than
Object as must is distributed further anny thus the phot up to distance 2l1 while object B mast is distributed closer to the point.
(d)
(e)
√ Decreasing
As it rotates towards eunhilibrium, the ampronent of gravity tangent to the motion of its center of mass devengds, so its aneleatun also devenges.
(f)
PE los!
\(mgh =\left ( \frac{1}{2}L \right )(10Lm = 5ML)\)
averted to statements kBs \(w = \sqrt{\frac{10ML}{I_{B}}}\)
1/2 Iw2 = 5ML
Iw2 = 10 ML
Question

A triangular rod of length L and mass M has a nonuniform linear mass density given by the equation λ = γx2, where \(\gamma =\frac{3M}{L^{3}}\) and x is the distance from point P at the left end of the rod.
(a) Using integral calculus, show that the rotational inertia I of the rod about an axis perpendicular to the page and through point P is \(\frac{3}{5}ML^{2}.\)
(b) Determine the horizontal location of the center of mass of the rod relative to point P. Express your answer in terms of L.
(c) For an axis perpendicular to the page, is the value of the rotational inertia of the rod around point P greater than, less than, or equal to the value of the rotational inertia of the rod around the rod’s center of mass?
_____ Greater than _____ Less than _____ Equal to
Justify your answer.
The rod is released from rest in the position shown, and the rod begins to rotate about a horizontal axis perpendicular to the page and through point P.
(d) On the axes below, sketch graphs of the magnitude of the net torque τ on the rod and the angular speed ω of the rod as functions of time t from the time the rod is released until the time its center of mass reaches its lowest point.

(e) As the rod rotates from the horizontal position down through vertical, is the magnitude of the angular acceleration on the rod increasing, decreasing, or not changing?
_____ Increasing _____ Decreasing _____ Not changing
Justify your answer.
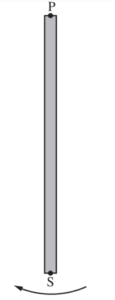
(f) The mass of the rod is 3.0 kg, and the length of the rod is 1.0 m. Calculate the linear speed v of point S as the rod swings through the vertical position shown.
Answer/Explanation
Ans:

(a)
dm = λdx
= δr2dr
\(I = \int r^{2}dm\rightarrow I = \int_{0}^{L}\delta x^{4}dx=\frac{\delta x^{5}}{5}|_{0}^{L}=\frac{\delta L^{5}}{5}-0\)
\(\Rightarrow I = \frac{3M}{L^{3}}\left ( \frac{\delta L^{5}}{5} \right )\rightarrow I = \frac{3ML^{2}}{5}\)
\(I = \frac{3ML^{2}}{5}\)
(b)
\(X_{cm}=\frac{\int rdm}{M}\Rightarrow X_{cm}=\frac{\int_{0}^{L}\delta r3dr}{M}\rightarrow X_{cm}=\frac{\delta r^{4}}{4M}|_{0}^{L}\Rightarrow X_{cm}==\frac{\delta L^{4}}{4M}-0\)
\(X_{cm}=\frac{3M}{L^{3}}\left ( \frac{L^{4}}{4M} \right )\rightarrow X_{cm}=\frac{3}{4}L\)
(c)
√ Greater than
This is became if the rod were ratating e its counter of mass, then the mass would be more centered around the axis then if it were rotating e point P.
(d)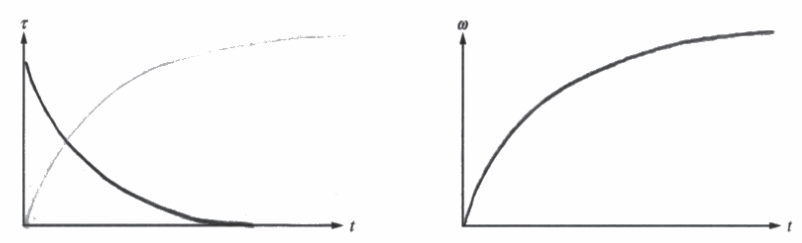
(e)
√ Decreasing
As the rod rotating, it follows ∑τ = Ix
\(\sum \tau =\tau_{g} =\overrightarrow{r}. \times \overrightarrow{F_{g}},\) which has a decreasing magnitude as when the bar rotates, the component of gravity perpendicular to the radius decreases. Thus, the magnitude of angular acceleration decreases.
(f)
\(E_{g} = E_{x}\rightarrow mgh = \frac{1}{2}I\omega^{2} \)
\(mg\frac{3}{4}L = \frac{1}{2}\left ( \frac{3mL^{2}}{5} \right )\omega ^{2}\rightarrow \frac{3mg}{4}L=\frac{3mL^{2}}{10}\)
Question
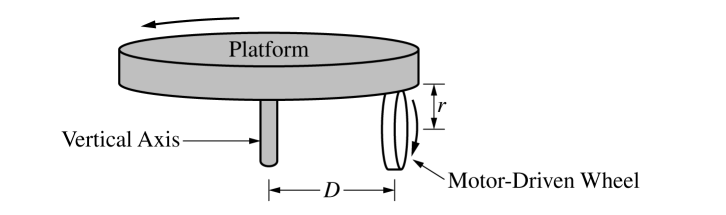
A horizontal circular platform with rotational inertia IP rotates freely without friction on a vertical axis. A small motor-driven wheel that is used to rotate the platform is mounted under the platform and touches it. The wheel has radius r and touches the platform a distance D from the vertical axis of the platform, as shown above. The platform starts at rest, and the wheel exerts a constant horizontal force of magnitude F tangent to the wheel until the platform reaches an angular speed ωP after time Δt . During time Δt , the wheel stays in contact with the
platform without slipping.
(a) Derive an expression for the angular speed ωP of the platform. Express your answer in terms of IP , r, D, F, Δt , and physical constants, as appropriate.
(b) Determine an expression for the kinetic energy of the platform at the moment it reaches angular speed ωP .
Express your answer in terms of IP , r, D, F, Δt , and physical constants, as appropriate.
(c) Derive an expression for the angular speed of the wheel ωW when the platform has reached angular speed ωP . Express your answer in terms of D, r, ωP , and physical constants, as appropriate.

When the platform is spinning at angular speed ωP , the motor-driven wheel is removed. A student holds a disk directly above and concentric with the platform, as shown above. The disk has the same rotational inertia IP as the platform. The student releases the disk from rest, and the disk falls onto the platform. After a short time, the disk and platform are observed to be rotating together at angular speed ωf .
(d) Derive an expression for ωf . Express your answer in terms of ωP , IP , and physical constants, as appropriate.
A student now uses the rotating platform (IP = 3.1 kg•m2 ) to determine the rotational inertia IU of an unknown object about a vertical axis that passes through the object’s center of mass. The platform is rotating at an initial angular speed ωi when the unknown object is dropped with its center of mass directly above the center of the platform. The platform and object are observed to be rotating together at angular speed ωf . Trials are repeated for different values of ωi . A graph of ωif as a function of ωi is shown on the axes below.
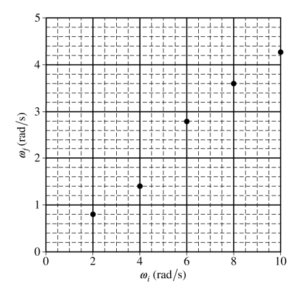
(e)
i. On the graph on the previous page, draw a best-fit line for the data.
ii. Using the straight line, calculate the rotational inertia of the unknown object IU about a vertical axis passing through its center of mass.
(f) The kinetic energy of the spinning platform before the object is dropped on it is Ki . The total kinetic energy of the platform-object system when it reaches angular speed ωf is Kf . Which of the following expressions is true?
____ Kf < Ki ____Ki = Ki ____ Kf > Ki
Justify your answer.
(g) One of the students observes that the center of mass of the object is not actually aligned with the axis of the platform. Is the experimental value of IU obtained in part (e) greater than, less than, or equal to the actual value of the rotational inertia of the unknown object about a vertical axis that passes through its center of mass?
____ Greater than ____ Less than ____ Equal to
Justify your answer.
Answer/Explanation
Ans:
(a)
ωp = O + α (Δt) τnet = Ipα = F•D \(\alpha =\frac{F\cdot D}{I_{p}}\)
\(\omega _{p} = \frac{FD\Delta t}{I _{p}}\) \(\frac{KyM/y\cdot f}{m^{2}\cdot ky}\)
(b)
\(K_{rot} = 1/2 I_{p}{\omega _{p}}^{2}=\frac{I_{p}F^{2}D^{2}\Delta t^{2}}{2{I_{p}}^{2}}=\frac{(FD\Delta t)^{2}}{2I_{p}}\)
(c)
Vp = Vw
ωp • D = ωw • r
ωw = \(\frac{\omega _{p}\cdot D}{r}\)
(d)
Ipωp = 2Ipωf \(\omega _{f} = \frac{\omega _{p}}{2}\)
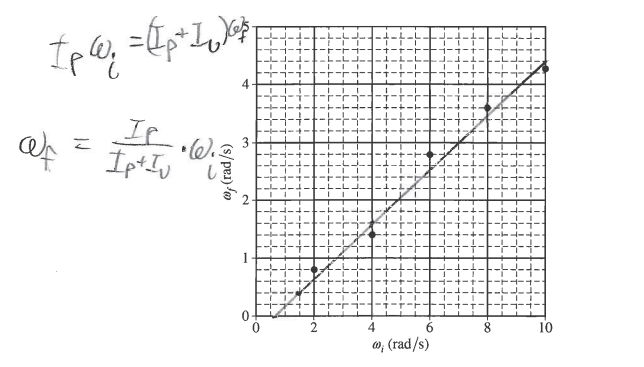
(e)
\(\omega _{f} = \frac{I_{p}}{I_{p}+I_{u}}\cdot \omega _{i}\) \(Slope = \frac{1.2}{2.5}= 0.48\)
\(\omega _{f}(rod/s)= 0.48\cdot \omega _{i}(rod/s)-0.32\)
\(0.48 = \frac{IP}{I_{p}+I_{u}}\) \(0.48I_{p}+0.48I_{u} = I_{p}\)
\(I_{u} = 3.36 kg\cdot m^{2}\)
(f)
X Kf < Ki
Krot = 1/2 Iω2
I increased and ω decreased 24.8 8.2688 liquidly, preserving momentum. The square of ω, however, decreased much more, so Krot decreased.
(g) X Greater than
Iu will be observed to be that of the object when it is some distance t from the contents increasing out perceive value of Iu by about Mx2.
