Question
(a) Define the term electron affinity.
(b) Write an equation for the process corresponding to the second ionisation energy of calcium.
Include state symbols.
Some data relating to calcium and oxygen are listed. Select relevant data from this list for your answers to parts (c), (d) and (e).
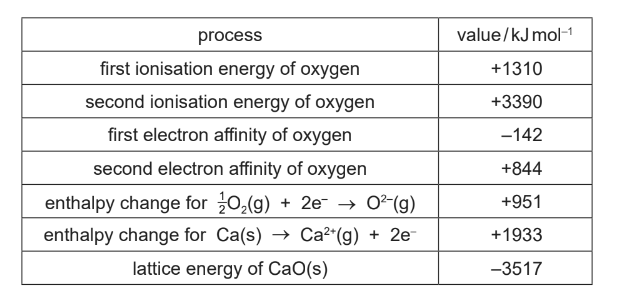
Show all your working.
(d) (i) Suggest why the first electron affinity of oxygen is negative.
(ii) Suggest why the second electron affinity of oxygen is positive.
(e) Calculate the enthalpy of formation of calcium oxide, CaO(s).
(f) The lattice energy of lithium fluoride, LiF(s), is –1022kJmol-1.
Identify the factor that causes the lattice energy of calcium oxide to be more exothermic than
that of lithium fluoride. Explain why this factor causes the difference in lattice energies.
Answer/Explanation
Answer (a) • enthalpy/energy change
• one mole of electrons gained
• by one mole of atoms
• gaseous (atoms)
(b) Ca+(g) → Ca2+(g) + e–
(c) M1: selecting correct data 951, 844, 142 only
M2: evaluation to give 249 (ΔHatom)
OR 2(951) = BE – 2(142) + 2(844)
M3: evaluation to 498 (2 × 249) ecf M2
951 =ΔHatom –142 + 844
ΔHatom = 249
BE = 498 (kJ mol–1)
(d)(i) attraction between nucleus / protons / nuclear charge
and electron
(d)(ii) repulsion between 1– ion / electrons of O–
and electron
(e) M1: selecting correct data 951, 1933, 3517 only (ignore signs)
M2: evaluation to give –633 (Δ Hf) ecf
ΔHf = 951 + 1933 – 3517 = –633 (kJ mol–1)
(f) ionic charge / charge density (of the ions)
greater (attractive) force between the ions
Question
(a) Successive ionisation energies for the elements magnesium to barium are given in the table
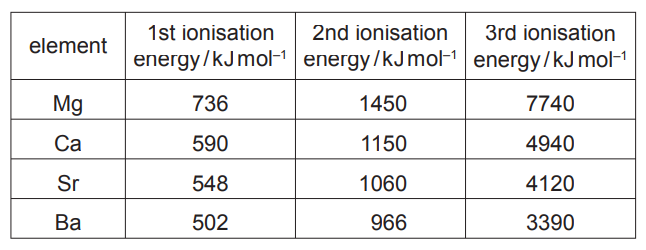
(i) Explain why the first ionisation energies decrease down the group.
……………………………………………………………………………………………………………………… [3]
(ii) Explain why, for each element, there is a large increase between the 2nd and 3rd ionisation energies.
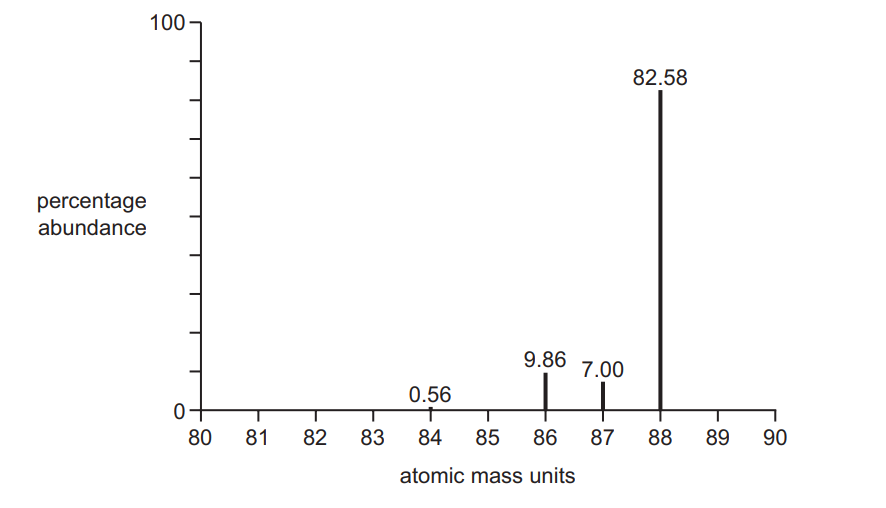
(b) A sample of strontium, atomic number 38, gave the mass spectrum shown. The percentage abundances are given above each peak.
(i) Complete the full electronic configuration of strontium.
$1 s^2 2 s^2 2 p^6$
(ii) Explain why there are four different peaks in the mass spectrum of strontium. [1]
(iii) Calculate the atomic mass, $A_r$, of this sample of strontium.
Give your answer to three significant figures.
$A_{\mathrm{r}}=$
(c) A compound of barium, $\mathbf{A}$, is used in fireworks as an oxidising agent and to produce a green colour.
(i) Explain, in terms of electron transfer, what is meant by the term oxidising agent. [1]
(ii) A has the following percentage composition by mass: $\mathrm{Ba}, 45.1 ; \mathrm{Cl}, 23.4 ; \mathrm{O}, 31.5$.
Calculate the empirical formula of $\mathbf{A}$.
empirical formula of $\mathbf{A}$ [3]
(d) Some reactions involving magnesium and its compounds are shown in the reaction scheme below.
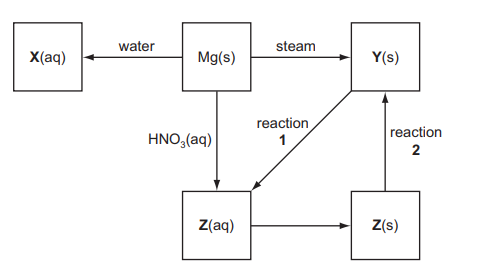
(i) Give the formulae of the compounds $\mathbf{X}, \mathbf{Y}$ and $\mathbf{Z}$.
$\mathbf{x}$
$\mathbf{Y}$
Z [3]
(ii) Name the reagent needed to convert $Y(\mathrm{~s})$ into $Z(\mathrm{Zq})$ in reaction 1 and write an equation for the reaction.
reagent
equation [2]
(iii) How would you convert a sample of $\mathrm{Z}(\mathrm{s})$ into $\mathrm{Y}(\mathrm{s})$ in reaction 2 ?
[1]
$\mathrm{Mg}$ to $\mathbf{X}$
$Z$ to $Y$
▶️Answer/Explanation
Ans:
(a) (i) increasing distance of (outer) electron(s) from nucleus
OR increasing distance of outer/ valence shell from nucleus
increased shielding/ screening (from inner shells)
reduces attraction
(ii) ( $3^{\text {rd }}$ electron for each in) inner/lower energy level/shell/closer to nucleus (than first two)/less shielding
(large) increase in nuclear attraction
(b) (i) $\left(1 s^2 2 s^2 2 p^6\right) 3 s^2 3 p^6 3 d^{10} 4 s^2 4 p^6 5 s^2$
(ii) four isotopes owtte
(iii) $\begin{aligned} & \frac{(84 \times 0.56)+(86 \times 9.86)+(87 \times 7)+(88 \times 82.58)}{100} \\ & =87.7 \text { (must be } 3 \text { sig figs) }\end{aligned}$
(c) (i) (a species that) gains / takes electron(s)
(ii) $\begin{array}{ccc}\mathrm{Ba} & \mathrm{Cl} & \mathrm{O} \\ \frac{45.1}{137} & \frac{23.4}{35.5} & \frac{31.5}{16} \\ \frac{0.329}{0.329} & \frac{0.659}{0.329} & \frac{1.969}{0.329} \\ 1.00 & 2.00 & 5.98 / 6 \\ \text { emp form }=\mathrm{BaCl}_2 \mathrm{O}_6\end{array}$
(d) (i)
$
\begin{aligned}
& \mathbf{X}=\mathrm{Mg}(\mathrm{OH})_2 \\
& \mathbf{Y}=\mathrm{MgO} \\
& \mathbf{Z}=\mathrm{Mg}\left(\mathrm{NO}_3\right)_2
\end{aligned}
$
\begin{tabular}{rl}
(ii) & reagent = nitric acid \\
& $\mathrm{MgO}+2 \mathrm{HNO}_3 \rightarrow \mathrm{Mg}\left(\mathrm{NO}_3\right)_2+\mathrm{H}_2 \mathrm{O}$ \\
(iii) Heat/thermal decomposition
$
\text { (iv) } \begin{aligned}
& \mathrm{Mg}+2 \mathrm{H}_2 \mathrm{O} \rightarrow \mathrm{Mg}(\mathrm{OH})_2+\mathrm{H}_2 \\
& 2 \mathrm{Mg}\left(\mathrm{NO}_3\right)_2 \rightarrow 2 \mathrm{MgO}+4 \mathrm{NO}_2+\mathrm{O}_2
\end{aligned}
$