Question
(a) Silver carbonate, $\mathrm{Ag}_2 \mathrm{CO}_3$, is sparingly soluble in water. The numerical value of the solubility product, $K_{\mathrm{sp}}$, for silver carbonate is $6.3 \times 10^{-12}$ at $25^{\circ} \mathrm{C}$.
(i) Write an expression for the solubility product, $\mathrm{K}_{\mathrm{sp}}$, of $\mathrm{Ag}_2 \mathrm{CO}_3$, and state its units.
$
K_{\mathrm{sp}}=
$ units = ………………………… [2]
(ii) Calculate the equilibrium concentration of $\mathrm{Ag}^{+}$in a saturated solution of $\mathrm{Ag}_2 \mathrm{CO}_3$ at $25^{\circ} \mathrm{C}$.
$\left[\mathrm{Ag}^{+}\right]=$ $\mathrm{moldm}^{-3}$ [1]
$\left[\mathrm{Ag}^{+}\right]=$ $\mathrm{moldm}^{-3}$ [1]
(iii) Solid $\mathrm{Ag}_2 \mathrm{CO}_3$ is stirred at $25^{\circ} \mathrm{C}$ with $0.050 \mathrm{moldm}^{-3} \mathrm{AgNO}_3$ until no more $\mathrm{Ag}_2 \mathrm{CO}_3$ dissolves. Calculate the concentration of carbonate ions, $\left[\mathrm{CO}_3{ }^{2-}\right]$, in this solution.
$
\left[\mathrm{CO}_3{ }^{2-}\right]=
$
$\mathrm{moldm}^{-3}$ [1]
(iv) An electrochemical cell is set up to measure the electrode potential, $E$, for the $\mathrm{Ag}^{+} / \mathrm{Ag}$ half-cell using the saturated $\mathrm{Ag}_2 \mathrm{CO}_3(\mathrm{aq})$ with a standard hydrogen electrode.
Use the Data Booklet, your answer to (a)(ii), and the Nernst equation to calculate the electrode potential, $E$, for this $\mathrm{Ag}^{+} / \mathrm{Ag}$ half-cell.
$E$ for $\mathrm{Ag}^{+} / \mathrm{Ag}$ half-cell $=$ $\mathrm{V}[\mathrm{s}]$
(b) Silver chloride, $\mathrm{AgCl}$, is sparingly soluble in water. The equation for the enthalpy change of solution is shown.
$
\operatorname{AgCl}(\mathrm{s}) \rightarrow \mathrm{Ag}^{+}(\mathrm{aq})+\mathrm{Cl}^{-}(\mathrm{aq}) \quad \Delta H_{\text {sol }}^{\ominus}=+65.5 \mathrm{~kJ} \mathrm{~mol}^{-1}
$
Standard entropies are shown in the table.
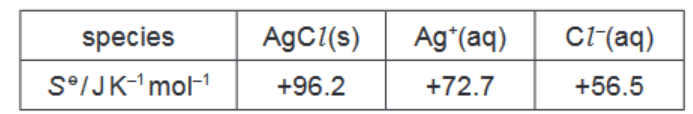
(i) Calculate the standard entropy change of solution, $\Delta S^\theta$.
$\Delta S^{\ominus}=\ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots \ldots . \mathrm{JK}^{-1} \mathrm{~mol}^{-1}[1]$
(ii) Explain, with the aid of a calculation, why $\mathrm{AgCl}$ is insoluble in water at $25^{\circ} \mathrm{C}$.
You should use data from this question and your answer to (b)(i).
(ii) Explain, with the aid of a calculation, why $\mathrm{AgC} l$ is insoluble in water at $25^{\circ} \mathrm{C}$. You should use data from this question and your answer to (b)(i). [3] [Total: 10]
▶️Answer/Explanation
Ans:
(a)(i) $\begin{aligned} & \text { M1 } K_{\mathrm{sp}}=\left[\mathrm{Ag}^{+}\right]^2\left[\mathrm{CO}_3^{2-}\right] \\ & \text { M2 units }=\mathrm{mol}^3 \mathrm{dm}^{-9}\end{aligned}$
7(a)(ii) $\begin{aligned} & x=\sqrt[3]{6} .3 \times 10^{-12} / 4=1.16 \times 10^{-4}\left(\mathrm{~mol} \mathrm{dm}^{-3}\right) \\ & {\left[\mathrm{Ag}^{+}\right]=1.16 \times 10^{-4} \times 2=2.33 \times 10^{-4}\left(\mathrm{~mol} \mathrm{dm^{-3 }}\right) \min 2 \mathrm{sf}} \\ & \end{aligned}$
(a)(iii) $
6.3 \times 10^{-12}=[0.05]^2\left[\mathrm{CO}_3{ }^{2-}\right]
$
$\left[\mathrm{CO}_3{ }^{2-}\right]=2.52 \times 10^{-9}\left(\mathrm{~mol} \mathrm{dm}^{-3}\right) \min 2 \mathrm{sf}$
a)(iv) M1 $E=E^0+0.059 \log \left[\mathrm{Ag}^{+}\right]$
M2 $E=0.80+0.059 \log \left(1.2 \times 10^{-4}\right)=0.57 \mathrm{~V}$ ecf from (a)(ii) $\min 2 \mathrm{sf}$
b)(i) $\Delta S^{\circ}=72.7+56.5-96.2=+33.0 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}$
(b)(ii) M1 $\Delta G=\Delta H^{\ominus}-\mathrm{T} \Delta S^{\ominus}$
M2 $\Delta G=(65.5)-(298 \times 0.033)=+55.7 \mathrm{~kJ}^{-1} \mathrm{~mol}^{-1} \min 3 s f$
M3 $\Delta G=$ positive so not feasible/spontaneous
Question
(a) Manganese(IV) oxide, $\mathrm{MnO}_2$, catalyses the decomposition of hydrogen peroxide, $\mathrm{H}_2 \mathrm{O}_2$, as shown.
$
2 \mathrm{H}_2 \mathrm{O}_2(\mathrm{aq}) \stackrel{\mathrm{MnO}_2}{\longrightarrow} 2 \mathrm{H}_2 \mathrm{O}(\mathrm{l})+\mathrm{O}_2(\mathrm{~g})
$
The mechanism involves the formation of the intermediate species, $\mathrm{Mn}^{2+}$, in the first step which is subsequently used up in the second step.
State and use relevant electrode potentials, $E^{\ominus}$, to construct two equations to show how $\mathrm{MnO}_2$ can catalyse this reaction.
equation 1 ………………………………………………………………………………………………………………….
equation 2 …………………………………………………………………………………………………………………. [3]
(b) The equation for the decomposition of hydrogen peroxide without a catalyst is shown.
$
2 \mathrm{H}_2 \mathrm{O}_2(\mathrm{aq}) \rightarrow 2 \mathrm{H}_2 \mathrm{O}(\mathrm{I})+\mathrm{O}_2(\mathrm{~g})
$
Under certain conditions this reaction is found to be first order with respect to hydrogen peroxide, with a rate constant, $k$, of $2.0 \times 10^{-6} \mathrm{~s}^{-1}$ at $298 \mathrm{~K}$.
Calculate the initial rate of decomposition of a $0.75 \mathrm{~mol} \mathrm{dm}^{-3}$ hydrogen peroxide solution at $298 \mathrm{~K}$.
initial rate $=$ $\mathrm{moldm}^{-3} \mathrm{~s}^{-1}$ [1]
(c) A four-step mechanism is suggested for the reaction between hydrogen peroxide and iodide ions in an acidic solution.
step $1 \mathrm{H}_2 \mathrm{O}_2+\mathrm{I}^{-} \rightarrow \mathrm{IO}^{-}+\mathrm{H}_2 \mathrm{O}$
step $2 \mathrm{H}^{+}+\mathrm{IO}^{-} \rightarrow \mathrm{HIO}$
step $3 \mathrm{HIO}+\mathrm{I}^{-} \rightarrow \mathrm{I}_2+\mathrm{OH}^{-}$
step $4 \mathrm{OH}^{-}+\mathrm{H}^{+} \rightarrow \mathrm{H}_2 \mathrm{O}$
Step 1 is the rate-determining step.
(i) State what is meant by the term rate-determining step. [1]
(ii) Use this mechanism to construct a balanced equation for this reaction. [1]
(iii) Deduce the order of reaction with respect to each of the following.
$\mathrm{H}_2 \mathrm{O}_2=$
$\mathrm{I}^{-}=$
$\mathrm{H}^{+}=$ [1] [Total: 7]
▶️Answer/Explanation
Ans:
M1 data seen $\mathrm{H}_2 \mathrm{O}_2 / \mathrm{H}_2 \mathrm{O}+1.77 \mathrm{~V}$ and $\mathrm{MnO}_2 / \mathrm{Mn}^{2+}+1.23 \mathrm{~V}$ and $\mathrm{O}_2 / \mathrm{H}_2 \mathrm{O}_2+0.68 \mathrm{~V}$ OR $E_{\text {cell }}=0.55 \mathrm{~V}$ (first step) and $0.54 \mathrm{~V}$ (second step)
M2 $\mathrm{MnO}_2+\mathrm{H}_2 \mathrm{O}_2+2 \mathrm{H}^{+} \rightarrow \mathrm{Mn}^{2+}+\mathrm{O}_2+2 \mathrm{H}_2 \mathrm{O}$
$\mathrm{M} 3 \mathrm{Mn}^{2+}+\mathrm{H}_2 \mathrm{O}_2 \rightarrow \mathrm{MnO}_2+2 \mathrm{H}^{+}$
$9(b) \quad$ rate $=2.0 \times 10^{-6} \times 0.75=1.5 \times 10^{-6}$
c)(i) slowest step in overall reaction
(c)(ii) $\begin{aligned} & \mathrm{H}_2 \mathrm{O}_2+2 \mathrm{H}^{+}+2 \mathrm{I}^{-} \rightarrow \mathrm{I}_2+2 \mathrm{H}_2 \mathrm{O} \\ & \text { OR } \mathrm{H}_2 \mathrm{O}_2+2 \mathrm{HI} \rightarrow \mathrm{I}_2+2 \mathrm{H}_2 \mathrm{O}\end{aligned}$
9(c)(iii) $\mathrm{H}_2 \mathrm{O}_2=1$ AND I- $=1$ AND $\mathrm{H}^{+}=0$