Question
(a) State one similarity and one difference between the fields of force produced by an isolated
point charge and by an isolated point mass.
similarity:
difference:
(b) An isolated solid metal sphere A of radius R has charge +Q, as illustrated in Fig. 5.1.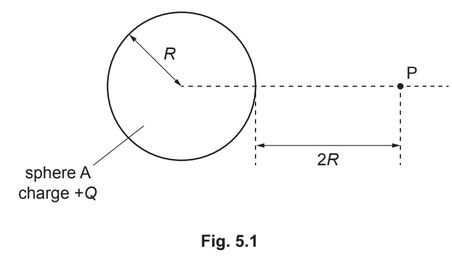
A point P is distance 2R from the surface of the sphere.
Determine an expression that includes the terms R and Q for the electric field strength E at
point P.
E = …………………………………………………
(c) A second identical solid metal sphere B is now placed near sphere A. The centres of the
spheres are separated by a distance 6R, as shown in Fig. 5.2.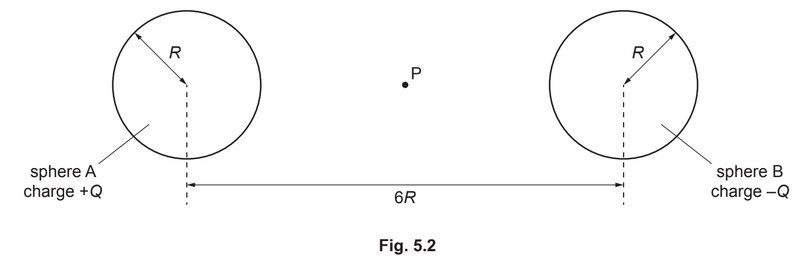
Point P lies midway between spheres A and B.
Sphere B has charge –Q.
Explain why:
(i) the magnitude of the electric field strength at P is given by the sum of the magnitudes of
the field strengths due to each sphere
(ii) the electric field strength at point P due to the charged metal spheres is not, in practice,
equal to 2E, where E is the electric field strength determined in (b).
Answer/Explanation
Answer:
(a) similarity: both are radial
or
both have inverse square (variations)
difference: direction is always/only towards the mass
or
direction can be towards or away from charge
(b) field strength = \(Q / 4 π ε_0x^2\)
\(E = Q / 36 π ε_0R^2\)
(c) (i) fields (due to each sphere) are in same direction
(ii) charges on spheres attract/affect each other
or
charge distribution on each sphere distorted by the other sphere
or
charges on the surface of the spheres move
Question
(a) State what is meant by electric field strength.
(b) Two point charges A and B are situated a distance 15cm apart in a vacuum, as illustrated in Fig. 5.1.
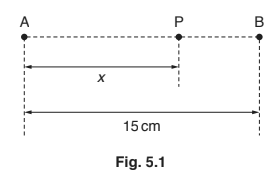
Point P lies on the line joining the charges and is a distance x from charge A.
The variation with distance x of the electric field strength E at point P is shown in Fig. 5.2.

(i) By reference to the direction of the electric field, state and explain whether the charges A and B have the same, or opposite, signs.
(ii) State why, although charge A is a point charge, the electric field strength between x = \(3\)cm and x = \(7\)cm does not obey an inverse-square law.
(iii) Use Fig. 5.2 to determine the ratio = \(\frac{magnitude\, of\, charge\, a}{magnitude\, of\, charge\, b}\)
Answer/Explanation
Ans:
(a) force per unit charge
(force on) positive charge
(b) (i) field changes direction (between A and B)/field is zero at a point (between A and B)
so charges have same sign
(ii) Any one from:
• field is (also) influenced by charge B
• charge A is not isolated/is not the only charge present
• field is due to two/both charges
• field is the resultant of two fields
(iii) E = \(Q / (4 \pi ε_{0} x^{2})\)
at x = \(10\) cm, \(E_{A}\) = \(E_{B}\)
\(Q_{A} / 10^{2}\) = \(Q_{B} / 52\)
