Question
Consider the arithmetic sequence \(u_1\) , \(u_2\) , \(u_3\) , ….
The sum of the first n terms of this sequence is given by \(S_n = n^2 + 4n\).
(a) (i) Find the sum of the first five terms.
(ii) Given that \(S_6\) = 60, find \(u_6\).
(b) Find \(u_1\).
(c) Hence or otherwise, write an expression for \(u_n\) in terms of n.
Consider a geometric sequence, \(v_n\), where \(v_2 = u_1\) and \(v_4 = u_6\).
(d) Find the possible values of the common ratio, r.
(e) Given that \(v_{99} < 0\), find \(v_5\).
Answer/Explanation
Answer:
(a) (i) recognition that n = 5
\(S_5\) = 45
(ii) METHOD 1
recognition that \(S_5 + u_6 = S_6\).
\(u_6\) = 15
METHOD 2
recognition that \(60 = \frac{6}{2} (S_1 + u_6)\)
\(u_6\) = 15
METHOD 3
substituting their \(u_1\) and d values into \(u_1\) + (n – 1)d
\(u_6\) = 15
(b) recognition that \(u_1 = S_1\) (may be seen in (a)) OR substituting their \(u_6\) into \(S_6\)
OR equations for \(S_5\) and \(S_6\) in temrs of \(u_1\) and d
1 + 4 OR 60 = \(\frac{6}{2}\) (\(u_1\) + 15)
\(u_1\) = 5
(c) EITHER
valid attempt to find d (may be seen in (a) or (b))
d = 2
OR
valid attempt to find \(S_n – S_{n-1}\)
\(n^2 + 4n – (n^2 – 2n + 1 + 4n – 4)\)
OR
equating \(n^2 + 4n = \frac{n}{2}(5+u_n)\)
2n + 8 = 5 + \(u_n\) (or equivalent)
THEN
\(u_n\) = 5 + 2(n – 1) OR \(u_n\) = 2n + 3
(d) recognition that \(v_2r^2 = v_4\) OR \((v_3)^2 = v_2 \times v_4\)
\(r^2 = 3\) OR \(v_3 = (±)5\sqrt{3}\)
r = ±\(\sqrt{3}\)
(e) recognition that r is negative
\(v_5\) = -15\(\sqrt{3}\) (=-\(\frac{45}{\sqrt{3}}\))
Question
Filicia baked a very large apple pie that she cuts into slices to share with her friends. The smallest
slice is cut first. The volume of each successive slice of pie forms a geometric sequence.
The second smallest slice has a volume of 30 cm3. The fifth smallest slice has a volume
of 240 cm3.
Find the common ratio of the sequence. [2]
Find the volume of the smallest slice of pie. [2] The apple pie has a volume of 61 425 cm3.
Find the total number of slices Filicia can cut from this pie. [2]
Answer/Explanation
Ans:
(a)
\(u_{1}r= 30 \:and\: u_{1}r^{4}\) = 240,
OR \(30r^{3}= 240 (r^{3}\)=8 )
r=2
(b)
\(u_{1}\times 2= 30 \: OR \: u_{1} \times 2^{4}\)= 240
\(u_{1}\)= 15
(c)
\(\frac{15 (2^{n}-1)}{2-1}\)= 61425
n= 12 (slices)
Question
The first three terms of a geometric sequence are \(\ln {x^{16}}\), \(\ln {x^8}\), \(\ln {x^4}\), for \(x > 0\).
Find the common ratio.
Solve \(\sum\limits_{k = 1}^\infty {{2^{5 – k}}\ln x = 64} \).
Answer/Explanation
Markscheme
correct use \(\log {x^n} = n\log x\) A1
eg\(\,\,\,\,\,\)\(16\ln x\)
valid approach to find \(r\) (M1)
eg\(\,\,\,\,\,\)\(\frac{{{u_{n + 1}}}}{{{u_n}}},{\text{ }}\frac{{\ln {x^8}}}{{\ln {x^{16}}}},{\text{ }}\frac{{4\ln x}}{{8\ln x}},{\text{ }}\ln {x^4} = \ln {x^{16}} \times {r^2}\)
\(r = \frac{1}{2}\) A1 N2
[3 marks]
recognizing a sum (finite or infinite) (M1)
eg\(\,\,\,\,\,\)\({2^4}\ln x + {2^3}\ln x,{\text{ }}\frac{a}{{1 – r}},{\text{ }}{S_\infty },{\text{ }}16\ln x + \ldots \)
valid approach (seen anywhere) (M1)
eg\(\,\,\,\,\,\)recognizing GP is the same as part (a), using their \(r\) value from part (a), \(r = \frac{1}{2}\)
correct substitution into infinite sum (only if \(\left| r \right|\) is a constant and less than 1) A1
eg\(\,\,\,\,\,\)\(\frac{{{2^4}\ln x}}{{1 – \frac{1}{2}}},{\text{ }}\frac{{\ln {x^{16}}}}{{\frac{1}{2}}},{\text{ }}32\ln x\)
correct working (A1)
eg\(\,\,\,\,\,\)\(\ln x = 2\)
\(x = {{\text{e}}^2}\) A1 N3
[5 marks]
Question
The first three terms of a infinite geometric sequence are \(m – 1,{\text{ 6, }}m + 4\), where \(m \in \mathbb{Z}\).
Write down an expression for the common ratio, \(r\).
Hence, show that \(m\) satisfies the equation \({m^2} + 3m – 40 = 0\).
Find the two possible values of \(m\).
Find the possible values of \(r\).
The sequence has a finite sum.
State which value of \(r\) leads to this sum and justify your answer.
The sequence has a finite sum.
Calculate the sum of the sequence.
Answer/Explanation
Markscheme
correct expression for \(r\) A1 N1
eg \(r = \frac{6}{{m – 1}},{\text{ }}\frac{{m + 4}}{6}\)
[2 marks]
correct equation A1
eg \(\frac{6}{{m – 1}} = \frac{{m + 4}}{6},{\text{ }}\frac{6}{{m + 4}} = \frac{{m – 1}}{6}\)
correct working (A1)
eg \((m + 4)(m – 1) = 36\)
correct working A1
eg \({m^2} – m + 4m – 4 = 36,{\text{ }}{m^2} + 3m – 4 = 36\)
\({m^2} + 3m – 40 = 0\) AG N0
[2 marks]
valid attempt to solve (M1)
eg \((m + 8)(m – 5) = 0,{\text{ }}m = \frac{{ – 3 \pm \sqrt {9 + 4 \times 40} }}{2}\)
\(m = – 8,{\text{ }}m = 5\) A1A1 N3
[3 marks]
attempt to substitute any value of \(m\) to find \(r\) (M1)
eg \(\frac{6}{{ – 8 – 1}},{\text{ }}\frac{{5 + 4}}{6}\)
\(r = \frac{3}{2},{\text{ }}r = – \frac{2}{3}\) A1A1 N3
[3 marks]
\(r = – \frac{2}{3}\) (may be seen in justification) A1
valid reason R1 N0
eg \(\left| r \right| < 1,{\text{ }} – 1 < \frac{{ – 2}}{3} < 1\)
Notes: Award R1 for \(\left| r \right| < 1\) only if A1 awarded.
[2 marks]
finding the first term of the sequence which has \(\left| r \right| < 1\) (A1)
eg \( – 8 – 1,{\text{ }}6 \div \frac{{ – 2}}{3}\)
\({u_1} = – 9\) (may be seen in formula) (A1)
correct substitution of \({u_1}\) and their \(r\) into \(\frac{{{u_1}}}{{1 – r}}\), as long as \(\left| r \right| < 1\) A1
eg \({S_\infty } = \frac{{ – 9}}{{1 – \left( { – \frac{2}{3}} \right)}},{\text{ }}\frac{{ – 9}}{{\frac{5}{3}}}\)
\({S_\infty } = – \frac{{27}}{5}{\text{ }}( = – 5.4)\) A1 N3
[4 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider the following sequence of figures.
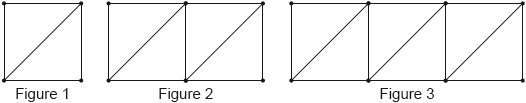
Figure 1 contains 5 line segments.
Given that Figure \(n\) contains 801 line segments, show that \(n = 200\).
Find the total number of line segments in the first 200 figures.
Answer/Explanation
Markscheme
recognizing that it is an arithmetic sequence (M1)
eg\(\,\,\,\,\,\)\(5,{\text{ }}5 + 4,{\text{ }}5 + 4 + 4,{\text{ }} \ldots ,{\text{ }}d = 4,{\text{ }}{u_n} = {u_1} + (n – 1)d,{\text{ }}4n + 1\)
correct equation A1
eg\(\,\,\,\,\,\)\(5 + 4(n – 1) = 801\)
correct working (do not accept substituting \(n = 200\)) A1
eg\(\,\,\,\,\,\)\(4n – 4 = 796,{\text{ }}n – 1 = \frac{{796}}{4}\)
\(n = 200\) AG N0
[3 marks]
recognition of sum (M1)
eg\(\,\,\,\,\,\)\({S_{200}},{\text{ }}{u_1} + {u_2} + \ldots + {u_{200}},{\text{ }}5 + 9 + 13 + \ldots + 801\)
correct working for AP (A1)
eg\(\,\,\,\,\,\)\(\frac{{200}}{2}(5 + 801),{\text{ }}\frac{{200}}{2}{\text{ }}\left( {2(5) + 199(4)} \right)\)
\(80\,600\) A1 N2
[3 marks]