Question:
The following diagram shows the curve \(\frac{x^{2}}{36}+\frac{(y-4)^{2}}{16}=1,\) where h ≤ y ≤ 4.

The curve from point Q to point B is rotated 360° about the y-axis to form the interior surface of a bowl. The rectangle OPQR, of height h cm, is rotated 360° about the y-axis to form a solid base.
The bowl is assumed to have negligible thickness. Given that the interior volume of the bowl is to be 285 cm3 , determine the height of the base.
Answer/Explanation
Ans:
attempts to express x2 in terms of y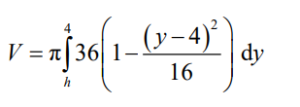
Note: Correct limits are required.
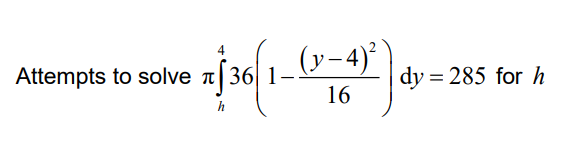
Note: Award M1 for attempting to solve \(36\pi \left ( \frac{h^{3}}{48}-\frac{h^{2}}{4}+\frac{8}{3} \right )=285\) or equivalent for h .
h = 0.7926…
h = 0.793 (cm)
Question
A random sample of nine adults were selected to see whether sleeping well affected their reaction times to a visual stimulus. Each adult’s reaction time was measured twice. The first measurement for reaction time was taken on a morning after the adult had slept well. The second measurement was taken on a morning after the same adult had not slept well. The box and whisker diagrams for the reaction times, measured in seconds, are shown below.

Consider the box and whisker diagram representing the reaction times after sleeping well.
(a) State the median reaction time after sleeping well.
(b) Verify that the measurement of 0.46 seconds is not an outlier.
(c) State why it appears that the mean reaction time is greater than the median reaction time.
Now consider the two box and whisker diagrams.
(d) Comment on whether these box and whisker diagrams provide any evidence that might suggest that not sleeping well causes an increase in reaction time.
Answer/Explanation
Ans:
(a) 0.28(s)
(b) IQR = 0.35 – 0.27 (=0.08) (s)
substituting their IQR into correct expression for upper fence
0.35+1.5×0.08(=0.47)(s)
0.46<0.47
so 0.46 (s) is not an outlier
(c) EITHER
the median is closer to the lower quartile (positively skewed)
OR
the distribution is positively skewed
OR
the range of reaction times below the median is smaller than the range of reaction times above the median
Note: These are sample answers from a range of acceptable correct answers. Award R1 for any correct statement that explains this. Do not award R1 if there is also an incorrect statement, even if another statement in the answer is correct. Accept a correctly and clearly labelled diagram.
(d) EITHER
the distribution for ‘not sleeping well’ is centred at a higher reaction time
OR
the median reaction time after not sleeping well is equal to the upper quartile reaction time after sleeping well
OR
75% of reaction times are <0.35 seconds after sleeping well, compared with 50% after not sleeping well
OR
the sample size of 9 is too small to draw any conclusions
Note: These are sample answers from a range of acceptable correct answers. Accept any relevant correct statement that relates to the median and/or quartiles shown in the box plots. Do not accept a comparison of means. Do not award R1 if there is also an incorrect statement, even if another statement in the answer is correct Award R0 to “correlation does not imply causation”.
