Question
[Maximum mark: 5]
$A$ and $B$ are independent events with $\mathrm{P}(A)=0.28$ and $\mathrm{P}(A \cup B)=0.75$. Find $\mathrm{P}(B)$.
Answer/Explanation
Using the combined events formula, we get
$
\begin{aligned}
\mathrm{P}(A \cup B) & =\mathrm{P}(A)+\mathrm{P}(B)-\mathrm{P}(A \cap B) \\
\mathrm{P}(A \cup B) & =\mathrm{P}(A)+\mathrm{P}(B)-\mathrm{P}(A) \mathrm{P}(B) \quad \text { [by independence] } \\
0.75 & =0.28+\mathrm{P}(B)-0.28 \mathrm{P}(B) \\
\mathrm{P}(B) & \approx 0.653 \quad[\text { by using G.D.C.] }
\end{aligned}
$
Question
[Maximum mark: 6]
The data of the goals scored by players in a futsal club during the winter games are given in the following table.
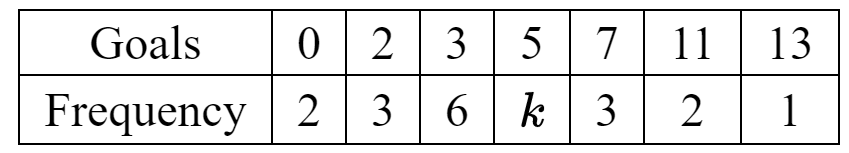
(a) Given that the mean number of goals scored per player is $4.8$, find the value of $k$. [3]
It is discovered that there is a mistake in the data and that two players, who scored 2 and 13 goals, have not been included in the table.
(b) (i) Find the correct mean number of goals scored per player.
(ii) Find the correct standard deviation of the numbers of goals scored per player. [3]
Answer/Explanation
(a) Using the mean formula, we get
$
\begin{aligned}
\bar{x} & =\left[\sum_{i=1}^7 f_i x_i\right] /\left[\sum_{i=1}^7 f_i\right] \\
4.8 & =\frac{(2)(0)+(3)(2)+(6)(3)+(k)(5)+(3)(7)+(2)(11)+(1)(13)}{2+3+6+k+3+2+1} \\
4.8 & =\frac{5 k+80}{k+17} \\
k & =8 \quad \text { [by using G.D.C.] }
\end{aligned}
$
(b) (i) Using the mean formula, we obtain
$
\begin{aligned}
\bar{x}_{\text {correct }} & =\frac{(5(8)+80)+(1)(2)+(1)(13)}{(8+17)+1+1} \\
& =5
\end{aligned}
$
(ii) Using G.D.C. we find
$
\sigma_{\text {correct }} \approx 3.45
$
