Question
State which of the following sets of data are discrete.
(i) Speeds of cars travelling along a road.
(ii) Numbers of members in families.
(iii) Maximum daily temperatures.
(iv) Heights of people in a class measured to the nearest cm.
(v) Daily intake of protein by members of a sporting team.[2]
The boxplot below shows the statistics for a set of data.
For this data set write down the value of
(i) the median
(ii) the upper quartile
(iii) the minimum value present[3]
Write down three different integers whose mean is 10.[1]
Answer/Explanation
Markscheme
(ii) and (iv) are discrete. (A1)(A1)
Award (A1)(A0) for both correct and one incorrect.
Award (A1)(A0) for one correct and two incorrect.
Otherwise, (A0)(A0). (C2)[2 marks]
(i) Median = 10 (A1)
(ii) Q3 = 12 (A1)
(iii) Min value = 1 (±0.2) (A1) (C3)[3 marks]
Any three different integers whose mean is 10 e.g. 9, 10, 11. (A1) (C1)[1 mark]
Question
A survey was conducted of the number of bedrooms in \(208\) randomly chosen houses. The results are shown in the following table.
State whether the data is discrete or continuous.[1]
Write down the mean number of bedrooms per house.[2]
Write down the standard deviation of the number of bedrooms per house.[1]
Find how many houses have a number of bedrooms greater than one standard deviation above the mean.[2]
Answer/Explanation
Markscheme
Discrete (A1) (C1)[1 mark]
For attempting to find \(\sum fx/\sum f\) (M1)
\(2.73\) (A1) (C2)
Note: for (b) and (c), if both mean and standard deviation given to 2 significant figures.
Award (C1)(C0)(AP) for \(2.7\). Award (A1)(ft) for \(1.3\) ((AP) already deducted).[2 marks]
\(1.34\) (A1) (C1)
Note: for (b) and (c), if both mean and standard deviation given to 2 significant figures.
Award (C1)(C0)(AP) for \(2.7\). Award (A1)(ft) for \(1.3\) ((AP) already deducted).[1 mark]
Attempt to find their mean \( + \) their standard deviation (can be implied) (M1)
\(23\), (ft) their mean and standard deviation. (A1)(ft) (C2)[2 marks]
Question
The following table shows the number of errors per page in a 100 page document.
State whether the data is discrete, continuous or neither.[1]
Find the mean number of errors per page.[2]
Find the median number of errors per page.[2]
Write down the mode.[1]
Answer/Explanation
Markscheme
Discrete (A1) (C1)[1 mark]
\(\frac{{0 + 24 + 40 + 51 + 44}}{{100}} = \frac{{159}}{{100}} = 1.59\) (M1)(A1) (C2)
Notes: Award (M1) for correctly substituted formula.
Award (M1)(A1) for 1 or 2 if 1.59 is seen.
Award (M0)(A0) for 1 or 2 seen with no working.[2 marks]
1 (M1)(A1) (C2)
Note: Award (M1) for attempt to order raw data (if frequency table not used) or (M1) for indicating halfway between 50th and 51st result or (M1) for 50th percentile seen.[2 marks]
0 (A1) (C1)[1 mark]
Question
A survey was carried out on a road to determine the number of passengers in each car (excluding the driver). The table shows the results of the survey.
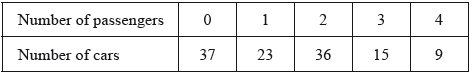
State whether the data is discrete or continuous.[1]
Write down the mode.[1]
Use your graphic display calculator to find
(i) the mean number of passengers per car;
(ii) the median number of passengers per car;
(iii) the standard deviation.[4]
Answer/Explanation
Markscheme
discrete (A1) (C1)[1 mark]
(i) \(1.47\) \((1.46666…)\) (A2)
Note: Award (M1) for \(\frac{{176}}{{120}}\) seen.
Accept \(1\) or \(2\) as a final answer if \(1.4666…\) or \(1.47\) seen.
(ii) \(1.5\) (A1)
(iii) \(1.25\) \((1.25122…)\) (A1) (C4)[4 marks]
Question
In a particular week, the number of eggs laid by each hen on a farm was counted. The results are summarized in the following table.
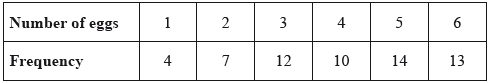
State whether these data are discrete or continuous.[1]
Write down
(i) the number of hens on the farm;
(ii) the modal number of eggs laid.[2]
Calculate
(i) the mean number of eggs laid;
(ii) the standard deviation.[3]
Answer/Explanation
Markscheme
discrete (A1) (C1)
(i) 60 (A1)
(ii) 5 (A1) (C2)
(i) \(\frac{{1 \times 4 + 2 \times 7 + 3 \times 12 \ldots }}{{60}}\) (M1)
Notes: Award (M1) for an attempt to substitute into the “mean of a set of data” formula, with at least three correct terms in the numerator.
Denominator must be 60.
Follow through from part (b)(i), only if work is seen.
\( = 4.03{\text{ }}(4.03333 \ldots )\) (A1)
Notes: Award at most (M1)(A0) for an answer of 4 but only if working seen.
(ii) \(1.54{\text{ }}(1.53803 \ldots )\) (A1) (C3)

