Question
Given \(f (x) = x^2 − 3x^{−1}, x \in {\mathbb{R}}, – 5 \leqslant x \leqslant 5, x \ne 0\),
A football is kicked from a point A (a, 0), 0 < a < 10 on the ground towards a goal to the right of A.
The ball follows a path that can be modelled by part of the graph
\(y = − 0.021x^2 + 1.245x − 6.01, x \in {\mathbb{R}}, y \geqslant 0\).
x is the horizontal distance of the ball from the origin
y is the height above the ground
Both x and y are measured in metres.
Write down the equation of the vertical asymptote.[1]
Find \(f ′(x)\).[2]
Using your graphic display calculator or otherwise, write down the coordinates of any point where the graph of \(y = f (x)\) has zero gradient.[2]
Write down all intervals in the given domain for which \(f (x)\) is increasing.[3]
Using your graphic display calculator or otherwise, find the value of a.[1]
Find \(\frac{{dy}}{{dx}}\).[2]
(i) Use your answer to part (b) to calculate the horizontal distance the ball has travelled from A when its height is a maximum.
(ii) Find the maximum vertical height reached by the football.[4]
Draw a graph showing the path of the football from the point where it is kicked to the point where it hits the ground again. Use 1 cm to represent 5 m on the horizontal axis and 1 cm to represent 2 m on the vertical scale.[4]
The goal posts are 35 m from the point where the ball is kicked.
At what height does the ball pass over the goal posts?[2]
Answer/Explanation
Markscheme
equation of asymptote is x = 0 (A1)
(Must be an equation.)
[1 mark]
\(f ‘(x) = 2x + 3x^{-2}\) (or equivalent) (A1) for each term (A1)(A1)
[2 marks]
stationary point (–1.14, 3.93) (G1)(G1)(ft)
(-1,4) or similar error is awarded (G0)(G1)(ft). Here and also as follow through in part (d) accept exact values \( – {\left( {\frac{3}{2}} \right)^{\frac{1}{3}}}\)for the x coordinate and \(3{\left( {\frac{3}{2}} \right)^{\frac{2}{3}}}\) for the y coordinate.
OR \(2x + \frac{3}{{{x^2}}} = 0\) or equivalent
Correct coordinates as above (M1)
Follow through from candidate’s \(f ′(x)\). (A1)(ft)
[2 marks]
In all alternative answers for (d), follow through from candidate’s x coordinate in part (c).
Alternative answers include:
–1.14 ≤ x < 0, 0 < x < 5 (A1)(A1)(ft)(A1)
OR [–1.14,0), (0,5)
Accept alternative bracket notation for open interval ] [. (Union of these sets is not correct, award (A2) if all else is right in this case.)
OR \( – 1.14 \leqslant x < 5,x \ne 0\)
In all versions 0 must be excluded (A1). -1.14 must be the left bound . 5 must be the right bound (A1). For \(x \geqslant – 1.14\) or \(x > – 1.14\) alone, award (A1). For \( – 1.4 \leqslant x < 0\) together with \(x > 0\) award (A2).
[3 marks]
a = 5.30 (3sf) (Allow (5.30, 0) but 5.3 receives an (AP).) (A1)
[1 mark]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = – 0.042x + 1.245\) (A1) for each term. (A1)(A1)
[2 marks]
Unit penalty (UP) is applicable where indicated in the left hand column.
(i) Maximum value when \(f ‘ (x) = 0\), \( – 0.042x + 1.245 = 0\), (M1)
(M1) is for either of the above but at least one must be seen.
(x = 29.6.)
Football has travelled 29.6 – 5.30 = 24.3 m (3sf) horizontally. (A1)(ft)
For answer of 24.3 m with no working or for correct subtraction of 5.3 from candidate’s x-coordinate at the maximum (if not 29.6), award (A1)(d).
(UP) (ii) Maximum vertical height, f (29.6) = 12.4 m (M1)(A1)(ft)(G2)
(M1) is for substitution into f of a value seen in part (c)(i). f(24.3) with or without evaluation is awarded (M1)(A0). For any other value without working, award (G0). If lines are seen on the graph in part (d) award (M1) and then (A1) for candidate’s value \( \pm 0.5\) (3sf not required.)
[4 marks]
(not to scale)
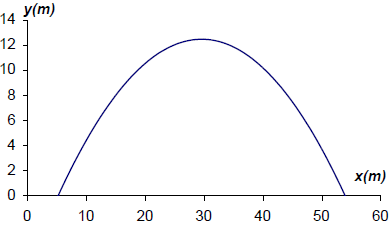(A1)(A1)(A1)(ft)(A1)(ft)
Award (A1) for labels (units not required) and scale, (A1)(ft) for max(29.6,12.4), (A1)(ft) for x-intercepts at 5.30 and 53.9, (all coordinates can be within 0.5), (A1) for well-drawn parabola ending at the x-intercepts.
[4 marks]
Unit penalty (UP) is applicable where indicated in the left hand column.
(UP) f (40.3) = 10.1 m (3sf).
Follow through from (a). If graph used, award (M1) for lines drawn and (A1) for candidate’s value \( \pm 0.5\). (3sf not required). (M1)(A1)(ft)(G2)
[2 marks]
Question
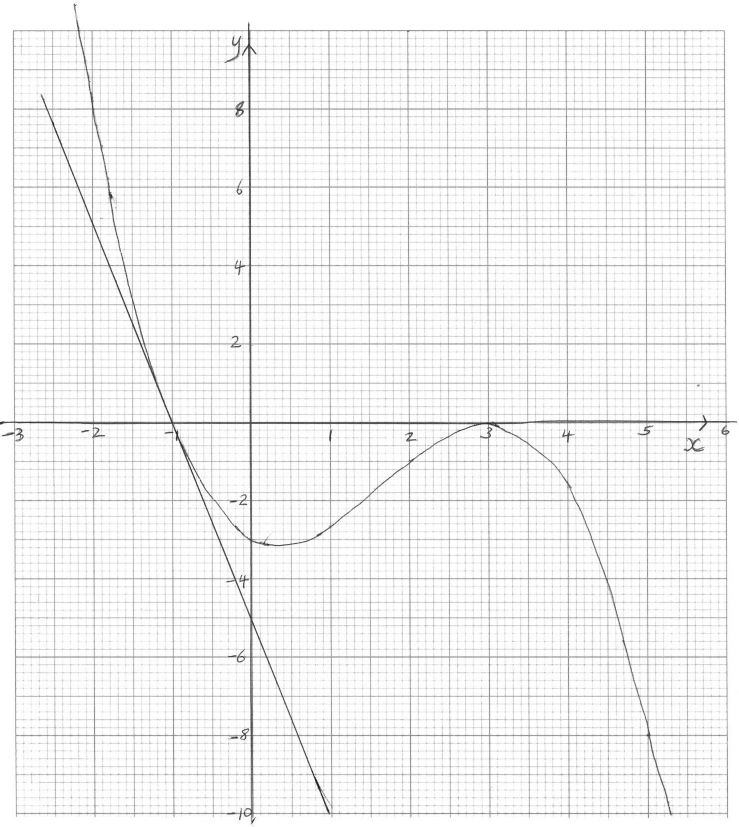
Consider the curve \(y = {x^3} + \frac{3}{2}{x^2} – 6x – 2\) .
(i) Write down the value of \(y\) when \(x\) is \(2\).
(ii) Write down the coordinates of the point where the curve intercepts the \(y\)-axis.[3]
Sketch the curve for \( – 4 \leqslant x \leqslant 3\) and \( – 10 \leqslant y \leqslant 10\). Indicate clearly the information found in (a).[4]
Find \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) .[3]
Let \({L_1}\) be the tangent to the curve at \(x = 2\).
Let \({L_2}\) be a tangent to the curve, parallel to \({L_1}\).
(i) Show that the gradient of \({L_1}\) is \(12\).
(ii) Find the \(x\)-coordinate of the point at which \({L_2}\) and the curve meet.
(iii) Sketch and label \({L_1}\) and \({L_2}\) on the diagram drawn in (b).[8]
It is known that \(\frac{{{\text{d}}y}}{{{\text{d}}x}} > 0\) for \(x < – 2\) and \(x > b\) where \(b\) is positive.
(i) Using your graphic display calculator, or otherwise, find the value of \(b\).
(ii) Describe the behaviour of the curve in the interval \( – 2 < x < b\) .
(iii) Write down the equation of the tangent to the curve at \(x = – 2\).[5]
Answer/Explanation
Markscheme
(i) \(y = 0\) (A1)
(ii) \((0{\text{, }}{- 2})\) (A1)(A1)
Note: Award (A1)(A0) if brackets missing.
OR
\(x = 0{\text{, }}y = – 2\) (A1)(A1)
Note: If coordinates reversed award (A0)(A1)(ft). Two coordinates must be given.
[3 marks]
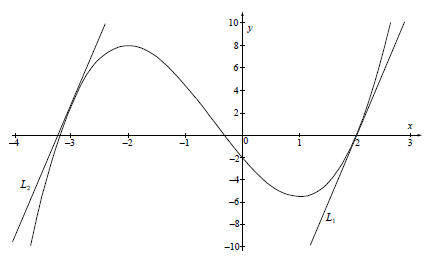(A4)
Notes: (A1) for appropriate window. Some indication of scale on the \(x\)-axis must be present (for example ticks). Labels not required. (A1) for smooth curve and shape, (A1) for maximum and minimum in approximately correct position, (A1) for \(x\) and \(y\) intercepts found in (a) in approximately correct position.
[4 marks]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = 3{x^2} + 3x – 6\) (A1)(A1)(A1)
Note: (A1) for each correct term. Award (A1)(A1)(A0) at most if any other term is present.
[3 marks]
(i) \(3 \times 4 + 3 \times 2 – 6 = 12\) (M1)(A1)(AG)
Note: (M1) for using the derivative and substituting \(x = 2\) . (A1) for correct (and clear) substitution. The \(12\) must be seen.
(ii) Gradient of \({L_2}\) is \(12\) (can be implied) (A1)
\(3{x^2} + 3x – 6 = 12\) (M1)
\(x = – 3\) (A1)(G2)
Note: (M1) for equating the derivative to \(12\) or showing a sketch of the derivative together with a line at \(y = 12\) or a table of values showing the \(12\) in the derivative column.
(iii) (A1) for \({L_1}\) correctly drawn at approx the correct point (A1)
(A1) for \({L_2}\) correctly drawn at approx the correct point (A1)
(A1) for 2 parallel lines (A1)
Note: If lines are not labelled award at most (A1)(A1)(A0). Do not accept 2 horizontal or 2 vertical parallel lines.
[8 marks]
(i) \(b = 1\) (G2)
(ii) The curve is decreasing. (A1)
Note: Accept any valid description.
(iii) \(y = 8\) (A1)(A1)(G2)
Note: (A1) for “\(y =\) a constant”, (A1) for \(8\).
[5 marks]
Question
Consider the function \(f(x) = 3x + \frac{{12}}{{{x^2}}},{\text{ }}x \ne 0\).
Differentiate \(f (x)\) with respect to \(x\).[3]
Calculate \(f ′(x)\) when \(x = 1\).[2]
Use your answer to part (b) to decide whether the function, \(f\) , is increasing or decreasing at \(x = 1\). Justify your answer.[2]
Solve the equation \(f ′(x) = 0\).[3]
The graph of f has a local minimum at point P. Let T be the tangent to the graph of f at P.
Write down the coordinates of P.[2]
The graph of f has a local minimum at point P. Let T be the tangent to the graph of f at P.
Write down the gradient of T.[1]
The graph of f has a local minimum at point P. Let T be the tangent to the graph of f at P.
Write down the equation of T.[2]
Sketch the graph of the function f, for −3 ≤ x ≤ 6 and −7 ≤ y ≤ 15. Indicate clearly the point P and any intercepts of the curve with the axes.[4]
On your graph draw and label the tangent T.[2]
T intersects the graph of f at a second point. Write down the x-coordinate of this point of intersection.[1]
Answer/Explanation
Markscheme
\(f’ (x) = 3 – \frac{24}{x^3}\) (A1)(A1)(A1)
Note: Award (A1) for 3, (A1) for –24, (A1) for x3 (or x−3). If extra terms present award at most (A1)(A1)(A0).
[3 marks]
\(f ‘(1) = -21\) (M1)(A1)(ft)(G2)
Note: (ft) from their derivative only if working seen.
[2 marks]
Derivative (gradient, slope) is negative. Decreasing. (R1)(A1)(ft)
Note: Do not award (R0)(A1).
[2 marks]
\(3 – \frac{{24}}{{{x^3}}} = 0\) (M1)
\(x^3 = 8\) (A1)
\(x = 2\) (A1)(ft)(G2)
[3 marks]
(2, 9) (Accept x = 2, y = 9) (A1)(A1)(G2)
Notes: (ft) from their answer in (d).
Award (A1)(A0) if brackets not included and not previously penalized.
[2 marks]
0 (A1)
[1 mark]
y = 9 (A1)(A1)(ft)(G2)
Notes: Award (A1) for y = constant, (A1) for 9.
Award (A1)(ft) for their value of y in (e)(i).
[2 marks]
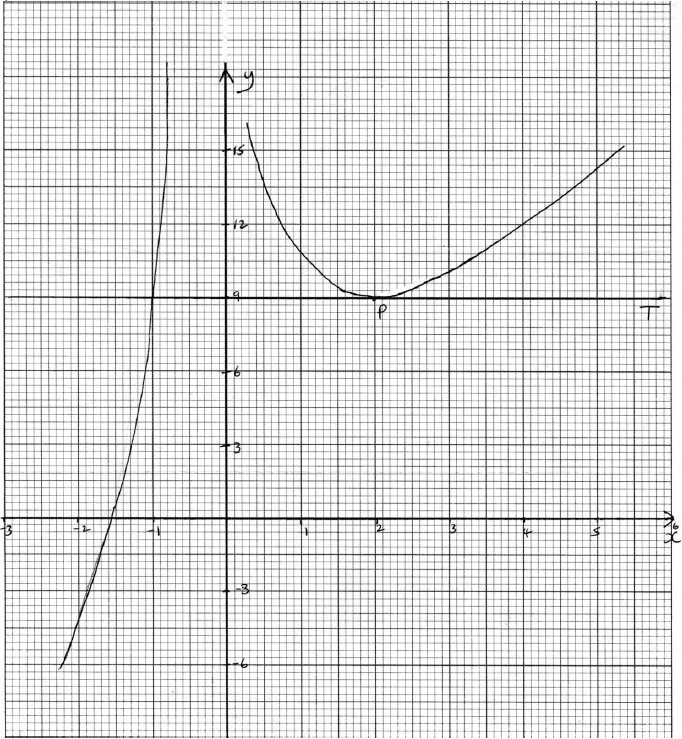(A4)
Notes: Award (A1) for labels and some indication of scale in the stated window.
Award (A1) for correct general shape (curve must be smooth and must not cross the y-axis).
Award (A1) for x-intercept seen in roughly the correct position.
Award (A1) for minimum (P).
[4 marks]
Tangent drawn at P (line must be a tangent and horizontal). (A1)
Tangent labeled T. (A1)
Note: (ft) from their tangent equation only if tangent is drawn and answer is consistent with graph.
[2 marks]
x = −1 (G1)(ft)
[1 mark]
Question
A function is defined by \(f(x) = \frac{5}{{{x^2}}} + 3x + c,{\text{ }}x \ne 0,{\text{ }}c \in \mathbb{Z}\).
Write down an expression for \(f ′(x)\).[4]
Consider the graph of f. The graph of f passes through the point P(1, 4).
Find the value of c.[2]
There is a local minimum at the point Q.
Find the coordinates of Q.[4]
There is a local minimum at the point Q.
Find the set of values of x for which the function is decreasing.[3]
Let T be the tangent to the graph of f at P.
Show that the gradient of T is –7.[2]
Let T be the tangent to the graph of f at P.
Find the equation of T.[2]
T intersects the graph again at R. Use your graphic display calculator to find the coordinates of R.[2]
Answer/Explanation
Markscheme
\(f'(x) = \frac{{ – 10}}{{{x^3}}} + 3\) (A1)(A1)(A1)(A1)
Note: Award (A1) for −10, (A1) for x3 (or x−3), (A1) for 3, (A1) for no other constant term.
[4 marks]
4 = 5 + 3 + c (M1)
Note: Award (M1) for substitution in f (x).
c = −4 (A1)(G2)
[2 marks]
\(f ‘(x) = 0\) (M1)
\(0 = \frac{{ – 10}}{{{x^3}}} + 3\) (A1)(ft)
(1.49, 2.72) (accept x = 1.49 y = 2.72) (A1)(ft)(A1)(ft)(G3)
Notes: If answer is given as (1.5, 2.7) award (A0)(AP)(A1).
Award at most (M1)(A1)(A1)(A0) if parentheses not included. (ft) from their (a).
If no working shown award (G2)(G0) if parentheses are not included.
OR
Award (M2) for sketch, (A1)(ft)(A1)(ft) for correct coordinates. (ft) from their (b). (M2)(A1)(ft)(A1)(ft)
Note: Award at most (M2)(A1)(ft)(A0) if parentheses not included.
[4 marks]
0 < x < 1.49 OR 0 < x ≤ 1.49 (A1)(A1)(ft)(A1)
Notes: Award (A1) for 0, (A1)(ft) for 1.49 and (A1) for correct inequality signs.
(ft) from their x value in (c) (i).
[3 marks]
For P(1, 4) \(f ‘(1) = – 10 + 3\) (M1)(A1)
\(= -7\) (AG)
Note: Award (M1) for substituting \(x = 1\) into their \(f ‘(x)\). (A1) for \(-10 + 3\).
\(-7\) must be seen for (A1) to be awarded.
[2 marks]
\(4 = -7 \times 1 + c\) \(11 = c\) (A1)
\(y = -7 x + 11\) (A1)
[2 marks]
Point of intersection is R(−0.5, 14.5) (A1)(ft)(A1)(ft)(G2)(ft)
Notes: Award (A1) for the x coordinate, (A1) for the y coordinate.
Allow (ft) from candidate’s (d)(ii) equation and their (b) even with no working seen.
Award (A1)(ft)(A0) if brackets not included and not previously penalised.
[2 marks]
Question
The function \(f(x)\) is defined by \(f(x) = 1.5x + 4 + \frac{6}{x}{\text{, }}x \ne 0\) .
Write down the equation of the vertical asymptote.[2]
Find \(f'(x)\) .[3]
Find the gradient of the graph of the function at \(x = – 1\).[2]
Using your answer to part (c), decide whether the function \(f(x)\) is increasing or decreasing at \(x = – 1\). Justify your answer.[2]
Sketch the graph of \(f(x)\) for \( – 10 \leqslant x \leqslant 10\) and \( – 20 \leqslant y \leqslant 20\) .[4]
\({{\text{P}}_1}\) is the local maximum point and \({{\text{P}}_2}\) is the local minimum point on the graph of \(f(x)\) .
Using your graphic display calculator, write down the coordinates of
(i) \({{\text{P}}_1}\) ;
(ii) \({{\text{P}}_2}\) .[4]
Using your sketch from (e), determine the range of the function \(f(x)\) for \( – 10 \leqslant x \leqslant 10\) .[3]
Answer/Explanation
Markscheme
\(x = 0\) (A1)(A1)
Note: Award (A1) for \(x = {\text{constant}}\), (A1) for \(0\).
[2 marks]
\(f'(x) = 1.5 – \frac{6}{{{x^2}}}\) (A1)(A1)(A1)
Notes: Award (A1) for \(1.5\), (A1) for \( – 6\), (A1) for \({x^{ – 2}}\) . Award (A1)(A1)(A0) at most if any other term present.
[3 marks]
\(1.5 – \frac{6}{{( – 1)}}\) (M1)
\( = – 4.5\) (A1)(ft)(G2)
Note: Follow through from their derivative function.
[2 marks]
Decreasing, the derivative (gradient or slope) is negative (at \(x = – 1\)) (A1)(R1)(ft)
Notes: Do not award (A1)(R0). Follow through from their answer to part (c).
[2 marks]
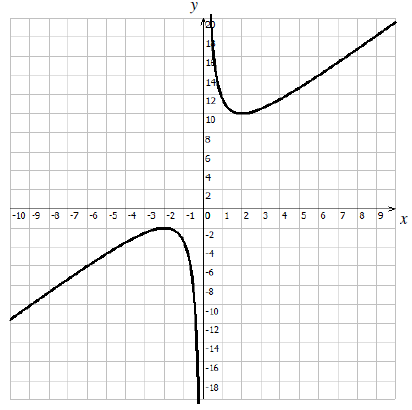(A4)
Notes: Award (A1) for labels and some indication of scales and an appropriate window.
Award (A1) for correct shape of the two unconnected, and smooth branches.
Award (A1) for the maximum and minimum points in the approximately correct positions.
Award (A1) for correct asymptotic behaviour at \(x = 0\) .
Notes: Please be rigorous.
The axes need not be drawn with a ruler.
The branches must be smooth and single continuous lines that do not deviate from their proper direction.
The max and min points must be symmetrical about point \((0{\text{, }}4)\) .
The \(y\)-axis must be an asymptote for both branches.
[4 marks]
(i) \(( – 2{\text{, }} – 2)\) or \(x = – 2\), \(y = – 2\) (G1)(G1)
(ii) \((2{\text{, }}10)\) or \(x = 2\), \(y = 10\) (G1)(G1)
[4 marks]
\(\{ – 2 \geqslant y\} \) or \(\{ y \geqslant 10\} \) (A1)(A1)(ft)(A1)
Notes: (A1)(ft) for \(y > 10\) or \(y \geqslant 10\) . (A1)(ft) for \(y < – 2\) or \(y \leqslant – 2\) . (A1) for weak (non-strict) inequalities used in both of the above. Follow through from their (e) and (f).
[3 marks]
Question
Consider the function \(f(x) = – \frac{1}{3}{x^3} + \frac{5}{3}{x^2} – x – 3\).
Sketch the graph of y = f (x) for −3 ≤ x ≤ 6 and −10 ≤ y ≤ 10 showing clearly the axes intercepts and local maximum and minimum points. Use a scale of 2 cm to represent 1 unit on the x-axis, and a scale of 1 cm to represent 1 unit on the y-axis.[4]
Find the value of f (−1).[2]
Write down the coordinates of the y-intercept of the graph of f (x).[1]
Find f ‘(x).[3]
Show that \(f'( – 1) = – \frac{{16}}{3}\).[1]
Explain what f ‘(−1) represents.[2]
Find the equation of the tangent to the graph of f (x) at the point where x is –1.[2]
Sketch the tangent to the graph of f (x) at x = −1 on your diagram for (a).[2]
P and Q are points on the curve such that the tangents to the curve at these points are horizontal. The x-coordinate of P is a, and the x-coordinate of Q is b, b > a.
Write down the value of
(i) a ;
(ii) b .[2]
P and Q are points on the curve such that the tangents to the curve at these points are horizontal. The x-coordinate of P is a, and the x-coordinate of Q is b, b > a.
Describe the behaviour of f (x) for a < x < b.[1]
Answer/Explanation
Markscheme
(A1) for indication of window and labels. (A1) for smooth curve that does not enter the first quadrant, the curve must consist of one line only.
(A1) for x and y intercepts in approximately correct positions (allow ±0.5).
(A1) for local maximum and minimum in approximately correct position. (minimum should be 0 ≤ x ≤ 1 and –2 ≤ y ≤ –4 ), the y-coordinate of the maximum should be 0 ± 0.5. (A4)
[4 marks]
\(-\frac{1}{3}(-1)^3 + \frac{5}{3}(-1)^2 – (-1) – 3 \) (M1)
Note: Award (M1) for substitution of –1 into f (x)
= 0 (A1)(G2)
[2 marks]
(0, –3) (A1)
OR
x = 0, y = –3 (A1)
Note: Award (A0) if brackets are omitted.
[1 mark]
\(f'(x) = – {x^2} + \frac{{10}}{3}x – 1\) (A1)(A1)(A1)
Note: Award (A1) for each correct term. Award (A1)(A1)(A0) at most if there are extra terms.
[3 marks]
\(f'( – 1) = – {( – 1)^2} + \frac{{10}}{3}( – 1) – 1\) (M1)
\(= -\frac{16}{3}\) (AG)
Note: Award (M1) for substitution of x = –1 into correct derivative only. The final answer must be seen.
[1 mark]
f ‘(–1) gives the gradient of the tangent to the curve at the point with x = –1. (A1)(A1)
Note: Award (A1) for “gradient (of curve)”, (A1) for “at the point with x = –1”. Accept “the instantaneous rate of change of y” or “the (first) derivative”.
[2 marks]
\(y = – \frac{16}{3} x + c\) (M1)
Note: Award (M1) for \(-\frac{16}{3}\) substituted in equation.
\(0 = – \frac{16}{3} \times (-1) + c \)
\(c = – \frac{16}{3}\)
\(y = – \frac{{16}}{3}x – \frac{{16}}{3}\) (A1)(G2)
Note: Accept y = –5.33x – 5.33.
OR
\((y – 0) = \frac{{-16}}{3}(x + 1)\) (M1)(A1)(G2)
Note: Award (M1) for \( – \frac{{16}}{3}\) substituted in equation, (A1) for correct equation. Follow through from their answer to part (b). Accept y = –5.33 (x +1). Accept equivalent equations.
[2 marks]
(A1)(ft) for a tangent to their curve drawn.
(A1)(ft) for their tangent drawn at the point x = –1. (A1)(ft)(A1)(ft)
Note: Follow through from their graph. The tangent must be a straight line otherwise award at most (A0)(A1).
[2 marks]
(i) \(a = \frac{1}{3}\) (G1)
(ii) \(b = 3\) (G1)
Note: If a and b are reversed award (A0)(A1).
[2 marks]
f (x) is increasing (A1)
[1 mark]
Question
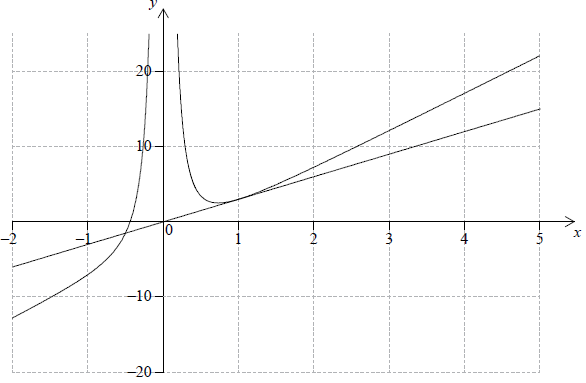
Consider the function \(g(x) = bx – 3 + \frac{1}{{{x^2}}},{\text{ }}x \ne 0\).
Write down the equation of the vertical asymptote of the graph of y = g(x) .[2]
Write down g′(x) .[3]
The line T is the tangent to the graph of y = g(x) at the point where x = 1. The gradient of T is 3.
Show that b = 5.[2]
The line T is the tangent to the graph of y = g(x) at the point where x = 1. The gradient of T is 3.
Find the equation of T.[3]
Using your graphic display calculator find the coordinates of the point where the graph of y = g(x) intersects the x-axis.[2]
(i) Sketch the graph of y = g(x) for −2 ≤ x ≤ 5 and −15 ≤ y ≤ 25, indicating clearly your answer to part (e).
(ii) Draw the line T on your sketch.[6]
Using your graphic display calculator find the coordinates of the local minimum point of y = g(x) .[2]
Write down the interval for which g(x) is increasing in the domain 0 < x < 5 .[2]
Answer/Explanation
Markscheme
x = 0 (A1)(A1)
Notes: Award (A1) for x=constant, (A1) for 0. Award (A0)(A0) if answer is not an equation.
[2 marks]
\(b – \frac{2}{{{x^3}}}\) (A1)(A1)(A1)
Note: Award (A1) for b, (A1) for −2, (A1) for \(\frac{1}{{{x^3}}}\) (or x−3). Award at most (A1)(A1)(A0) if extra terms seen.
[3 marks]
\(3 = b – \frac{2}{{{{(1)}^3}}}\) (M1)(M1)
Note: Award (M1) for substituting 1 into their gradient function, (M1) for equating their gradient function to 3.
b = 5 (AG)
Note: Award at most (M1)(A0) if final line is not seen or b does not equal 5.
[2 marks]
g(1) = 3 or (1, 3) (seen or implied from the line below) (A1)
3 = 3 × 1 + c (M1)
Note: Award (M1) for correct substitution of their point (1, 3) and gradient 3 into equation y = mx + c. Follow through from their point of tangency.
y = 3x (A1)(ft)(G2)
OR
y − 3 = 3(x − 1) (M1)(A1)(ft)(G2)
Note: Award (M1) for substitution of gradient 3 and their point (1, 3) into y − y1 = m(x − x1), (A1)(ft) for correct substitutions. Follow through from their point of tangency. Award at most (A1)(M1)(A0)(ft) if further incorrect working seen.
[3 marks]
(−0.439, 0) ((−0.438785…, 0)) (G1)(G1)
Notes: If no parentheses award at most (G1)(G0). Accept x = 0.439, y = 0.
[2 marks]
(i)
Award (A1) for labels and some indication of scale in the stated window.
Award (A1) for correct general shape (curve must be smooth and must not cross the y-axis)
Award (A1)(ft) for x-intercept consistent with their part (e).
Award (A1) for local minimum in the first quadrant. (A1)(A1)(A1)(ft)(A1)
(ii) Tangent to curve drawn at approximately x = 1 (A1)(A1)
Note: Award (A1) for a line tangent to curve approximately at x = 1. Must be a straight line for the mark to be awarded. Award (A1)(ft) for line passing through the origin. Follow through from their answer to part (d).
[6 marks]
(0.737, 2.53) ((0.736806…, 2.52604…)) (G1)(G1)
Notes: Do not penalize for lack of parentheses if already penalized in (e). Accept x = 0.737, y = 2.53.
[2 marks]
0.737 < x < 5 OR (0.737;5) (A1)(A1)(ft)
Notes: Award (A1) for correct strict or weak inequalities with x seen if the interval is given as inequalities, (A1)(ft) for 0.737 and 5 or their value from part (g).
[2 marks]
Question
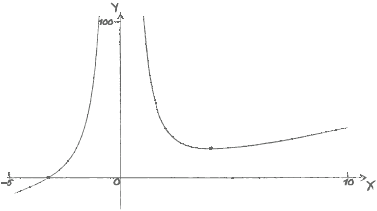
Consider the function \(f(x) = \frac{{96}}{{{x^2}}} + kx\), where \(k\) is a constant and \(x \ne 0\).
Write down \(f'(x)\).[3]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Show that \(k = 3\).[2]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Find \(f(2)\).[2]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Find \(f'(2)\)[2]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Find the equation of the normal to the graph of \(y = f(x)\) at the point where \(x = 2\).
Give your answer in the form \(ax + by + d = 0\) where \(a,{\text{ }}b,{\text{ }}d \in \mathbb{Z}\).[3]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Sketch the graph of \(y = f(x)\), for \( – 5 \leqslant x \leqslant 10\) and \( – 10 \leqslant y \leqslant 100\).[4]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
Write down the coordinates of the point where the graph of \(y = f(x)\) intersects the \(x\)-axis.[2]
The graph of \(y = f(x)\) has a local minimum point at \(x = 4\).
State the values of \(x\) for which \(f(x)\) is decreasing.[2]
Answer/Explanation
Markscheme
\(\frac{{ – 192}}{{{x^3}}} + k\) (A1)(A1)(A1)
Note: Award (A1) for \(-192\), (A1) for \({x^{ – 3}}\), (A1) for \(k\) (only).
at local minimum \(f'(x) = 0\) (M1)
Note: Award (M1) for seeing \(f'(x) = 0\) (may be implicit in their working).
\(\frac{{ – 192}}{{{4^3}}} + k = 0\) (A1)
\(k = 3\) (AG)
Note: Award (A1) for substituting \(x = 4\) in their \(f'(x) = 0\), provided it leads to \(k = 3\). The conclusion \(k = 3\) must be seen for the (A1) to be awarded.
\(\frac{{96}}{{{2^2}}} + 3(2)\) (M1)
Note: Award (M1) for substituting \(x = 2\) and \(k = 3\) in \(f(x)\).
\( = 30\) (A1)(G2)
\(\frac{{ – 192}}{{{2^3}}} + 3\) (M1)
Note: Award (M1) for substituting \(x = 2\) and \(k = 3\) in their \(f'(x)\).
\( = – 21\) (A1)(ft)(G2)
Note: Follow through from part (a).
\(y – 30 = \frac{1}{{21}}(x – 2)\) (A1)(ft)(M1)
Notes: Award (A1)(ft) for their \(\frac{1}{{21}}\) seen, (M1) for the correct substitution of their point and their normal gradient in equation of a line.
Follow through from part (c) and part (d).
OR
gradient of normal \( = \frac{1}{{21}}\) (A1)(ft)
\(30 = \frac{1}{{21}} \times 2 + c\) (M1)
\(c = 29\frac{{19}}{{21}}\)
\(y = \frac{1}{{21}}x + 29\frac{{19}}{{21}}\;\;\;(y = 0.0476x + 29.904)\)
\(x – 21y + 628 = 0\) (A1)(ft)(G2)
Notes: Accept equivalent answers.
(A1)(A1)(A1)(A1)
Notes: Award (A1) for correct window (at least one value, other than zero, labelled on each axis), the axes must also be labelled; (A1) for a smooth curve with the correct shape (graph should not touch \(y\)-axis and should not curve away from the \(y\)-axis), on the given domain; (A1) for axis intercept in approximately the correct position (nearer \(-5\) than zero); (A1) for local minimum in approximately the correct position (first quadrant, nearer the \(y\)-axis than \(x = 10\)).
If there is no scale, award a maximum of (A0)(A1)(A0)(A1) – the final (A1) being awarded for the zero and local minimum in approximately correct positions relative to each other.
\(( – 3.17,{\text{ }}0)\;\;\;\left( {( – 3.17480 \ldots ,{\text{ 0)}}} \right)\) (G1)(G1)
Notes: If parentheses are omitted award (G0)(G1)(ft).
Accept \(x = – 3.17,{\text{ }}y = 0\). Award (G1) for \(-3.17\) seen.
\(0 < x \leqslant 4{\text{ or }}0 < x < 4\) (A1)(A1)
Notes: Award (A1) for correct end points of interval, (A1) for correct notation (note: lower inequality must be strict).
Award a maximum of (A1)(A0) if \(y\) or \(f(x)\) used in place of \(x\).
Question
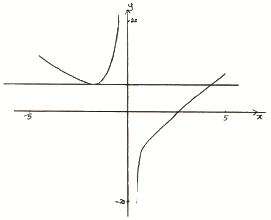
Consider the function \(f(x) = 0.5{x^2} – \frac{8}{x},{\text{ }}x \ne 0\).
Find \(f( – 2)\).[2]
Find \(f'(x)\).[3]
Find the gradient of the graph of \(f\) at \(x = – 2\).[2]
Let \(T\) be the tangent to the graph of \(f\) at \(x = – 2\).
Write down the equation of \(T\).[2]
Let \(T\) be the tangent to the graph of \(f\) at \(x = – 2\).
Sketch the graph of \(f\) for \( – 5 \leqslant x \leqslant 5\) and \( – 20 \leqslant y \leqslant 20\).
Let \(T\) be the tangent to the graph of \(f\) at \(x = – 2\).
Draw \(T\) on your sketch.[2]
The tangent, \(T\), intersects the graph of \(f\) at a second point, P.
Use your graphic display calculator to find the coordinates of P.[2]
Answer/Explanation
Markscheme
\(0.5 \times {( – 2)^2} – \frac{8}{{ – 2}}\) (M1)
Note: Award (M1) for substitution of \(x = – 2\) into the formula of the function.
\(6\) (A1)(G2)
\(f'(x) = x + 8{x^{ – 2}}\) (A1)(A1)(A1)
Notes: Award (A1) for \(x\), (A1) for \(8\), (A1) for \({x^{ – 2}}\) or \(\frac{1}{{{x^2}}}\) (each term must have correct sign). Award at most (A1)(A1)(A0) if there are additional terms present or further incorrect simplifications are seen.
\(f'( – 2) = – 2 + 8{( – 2)^{ – 2}}\) (M1)
Note: Award (M1) for \(x = – 2\) substituted into their \(f'(x)\) from part (b).
\( = 0\) (A1)(ft)(G2)
Note: Follow through from their derivative function.
\(y = 6\;\;\;\)OR\(\;\;\;y = 0x + 6\;\;\;\)OR\(\;\;\;y – 6 = 0(x + 2)\) (A1)(ft)(A1)(ft)(G2)
Notes: Award (A1)(ft) for their gradient from part (c), (A1)(ft) for their answer from part (a). Answer must be an equation.
Award (A0)(A0) for \(x = 6\).
(A1)(A1)(A1)(A1)
Notes: Award (A1) for labels and some indication of scales in the stated window. The point \((-2,{\text{ }}6)\) correctly labelled, or an \(x\)-value and a \(y\)-value on their axes in approximately the correct position, are acceptable indication of scales.
Award (A1) for correct general shape (curve must be smooth and must not cross the \(y\)-axis).
Award (A1) for \(x\)-intercept in approximately the correct position.
Award (A1) for local minimum in the second quadrant.
Tangent to graph drawn approximately at \(x = – 2\) (A1)(ft)(A1)(ft)
Notes: Award (A1)(ft) for straight line tangent to curve at approximately \(x = – 2\), with approximately correct gradient. Tangent must be straight for the (A1)(ft) to be awarded.
Award (A1)(ft) for (extended) line passing through approximately their \(y\)-intercept from (d). Follow through from their gradient in part (c) and their equation in part (d).
\((4,{\text{ }}6)\;\;\;\)OR\(\;\;\;x = 4,{\text{ }}y = 6\) (G1)(ft)(G1)(ft)
Notes: Follow through from their tangent from part (d). If brackets are missing then award (G0)(G1)(ft).
If line intersects their graph at more than one point (apart from \(( – 2,{\text{ }}6)\)), follow through from the first point of intersection (to the right of \( – 2\)).
Award (G0)(G0) for \(( – 2,{\text{ }}6)\).
Question
Consider the function \(g(x) = {x^3} + k{x^2} – 15x + 5\).
The tangent to the graph of \(y = g(x)\) at \(x = 2\) is parallel to the line \(y = 21x + 7\).
Find \(g'(x)\).[3]
Show that \(k = 6\).[2]
Find the equation of the tangent to the graph of \(y = g(x)\) at \(x = 2\). Give your answer in the form \(y = mx + c\).[3]
Use your answer to part (a) and the value of \(k\), to find the \(x\)-coordinates of the stationary points of the graph of \(y = g(x)\).[3]
Find \(g’( – 1)\).[2]
Hence justify that \(g\) is decreasing at \(x = – 1\).[1]
Find the \(y\)-coordinate of the local minimum.[2]
Answer/Explanation
Markscheme
\(3{x^2} + 2kx – 15\) (A1)(A1)(A1)
Note: Award (A1) for \(3{x^2}\), (A1) for \(2kx\) and (A1) for \( – 15\). Award at most (A1)(A1)(A0) if additional terms are seen.
[3 marks]
\(21 = 3{(2)^2} + 2k(2) – 15\) (M1)(M1)
Note: Award (M1) for equating their derivative to 21. Award (M1) for substituting 2 into their derivative. The second (M1) should only be awarded if correct working leads to the final answer of \(k = 6\).
Substituting in the known value, \(k = 6\), invalidates the process; award (M0)(M0).
\(k = 6\) (AG)
[2 marks]
\(g(2) = {(2)^3} + (6){(2)^2} – 15(2) + 5{\text{ }}( = 7)\) (M1)
Note: Award (M1) for substituting 2 into \(g\).
\(7 = 21(2) + c\) (M1)
Note: Award (M1) for correct substitution of 21, 2 and their 7 into gradient intercept form.
OR
\(y – 7 = 21(x – 2)\) (M1)
Note: Award (M1) for correct substitution of 21, 2 and their 7 into gradient point form.
\(y = 21x – 35\) (A1) (G2)
[3 marks]
\(3{x^2} + 12x – 15 = 0\) (or equivalent) (M1)
Note: Award (M1) for equating their part (a) (with \(k = 6\) substituted) to zero.
\(x = – 5,{\text{ }}x = 1\) (A1)(ft)(A1)(ft)
Note: Follow through from part (a).
[3 marks]
\(3{( – 1)^2} + 12( – 1) – 15\) (M1)
Note: Award (M1) for substituting \( – 1\) into their derivative, with \(k = 6\) substituted. Follow through from part (a).
\( = – 24\) (A1)(ft) (G2)
[2 marks]
\(g’( – 1) < 0\) (therefore \(g\) is decreasing when \(x = – 1\)) (R1)
[1 marks]
\(g(1) = {(1)^3} + (6){(1)^2} – 15(1) + 5\) (M1)
Note: Award (M1) for correctly substituting 6 and their 1 into \(g\).
\( = – 3\) (A1)(ft) (G2)
Note: Award, at most, (M1)(A0) or (G1) if answer is given as a coordinate pair. Follow through from part (c).
[2 marks]
Question
Consider the function \(f(x) = – {x^4} + a{x^2} + 5\), where \(a\) is a constant. Part of the graph of \(y = f(x)\) is shown below.
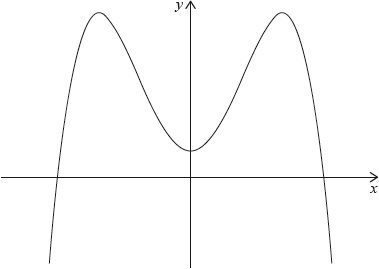
It is known that at the point where \(x = 2\) the tangent to the graph of \(y = f(x)\) is horizontal.
There are two other points on the graph of \(y = f(x)\) at which the tangent is horizontal.
Write down the \(y\)-intercept of the graph.[1]
Find \(f'(x)\).[2]
Show that \(a = 8\).[2]
Find \(f(2)\).[2]
Write down the \(x\)-coordinates of these two points;[2]
Write down the intervals where the gradient of the graph of \(y = f(x)\) is positive.[2]
Write down the range of \(f(x)\).[2]
Write down the number of possible solutions to the equation \(f(x) = 5\).[1]
The equation \(f(x) = m\), where \(m \in \mathbb{R}\), has four solutions. Find the possible values of \(m\).[2]
Answer/Explanation
Markscheme
5 (A1)
Note: Accept an answer of \((0,{\text{ }}5)\).
[1 mark]
\(\left( {f'(x) = } \right) – 4{x^3} + 2ax\) (A1)(A1)
Note: Award (A1) for \( – 4{x^3}\) and (A1) for \( + 2ax\). Award at most (A1)(A0) if extra terms are seen.
[2 marks]
\( – 4 \times {2^3} + 2a \times 2 = 0\) (M1)(M1)
Note: Award (M1) for substitution of \(x = 2\) into their derivative, (M1) for equating their derivative, written in terms of \(a\), to 0 leading to a correct answer (note, the 8 does not need to be seen).
\(a = 8\) (AG)
[2 marks]
\(\left( {f(2) = } \right) – {2^4} + 8 \times {2^2} + 5\) (M1)
Note: Award (M1) for correct substitution of \(x = 2\) and \(a = 8\) into the formula of the function.
21 (A1)(G2)
[2 marks]
\((x = ){\text{ }} – 2,{\text{ }}(x = ){\text{ 0}}\) (A1)(A1)
Note: Award (A1) for each correct solution. Award at most (A0)(A1)(ft) if answers are given as \(( – 2{\text{ }},21)\) and \((0,{\text{ }}5)\) or \(( – 2,{\text{ }}0)\) and \((0,{\text{ }}0)\).
[2 marks]
\(x < – 2,{\text{ }}0 < x < 2\) (A1)(ft)(A1)(ft)
Note: Award (A1)(ft) for \(x < – 2\), follow through from part (d)(i) provided their value is negative.
Award (A1)(ft) for \(0 < x < 2\), follow through only from their 0 from part (d)(i); 2 must be the upper limit.
Accept interval notation.
[2 marks]
\(y \leqslant 21\) (A1)(ft)(A1)
Notes: Award (A1)(ft) for 21 seen in an interval or an inequality, (A1) for “\(y \leqslant \)”.
Accept interval notation.
Accept \( – \infty < y \leqslant 21\) or \(f(x) \leqslant 21\).
Follow through from their answer to part (c)(ii). Award at most (A1)(ft)(A0) if \(x\) is seen instead of \(y\). Do not award the second (A1) if a (finite) lower limit is seen.
[2 marks]
3 (solutions) (A1)
[1 mark]
\(5 < m < 21\) or equivalent (A1)(ft)(A1)
Note: Award (A1)(ft) for 5 and 21 seen in an interval or an inequality, (A1) for correct strict inequalities. Follow through from their answers to parts (a) and (c)(ii).
Accept interval notation.
[2 marks]
Question
A function \(f\) is given by \(f(x) = (2x + 2)(5 – {x^2})\).
The graph of the function \(g(x) = {5^x} + 6x – 6\) intersects the graph of \(f\).
Find the exact value of each of the zeros of \(f\).[3]
Expand the expression for \(f(x)\).[1]
Find \(f’(x)\).[3]
Use your answer to part (b)(ii) to find the values of \(x\) for which \(f\) is increasing.[3]
Draw the graph of \(f\) for \( – 3 \leqslant x \leqslant 3\) and \( – 40 \leqslant y \leqslant 20\). Use a scale of 2 cm to represent 1 unit on the \(x\)-axis and 1 cm to represent 5 units on the \(y\)-axis.[4]
Write down the coordinates of the point of intersection.[2]
Answer/Explanation
Markscheme
\( – 1,{\text{ }}\sqrt 5 ,{\text{ }} – \sqrt 5 \) (A1)(A1)(A1)
Note: Award (A1) for –1 and each exact value seen. Award at most (A1)(A0)(A1) for use of 2.23606… instead of \(\sqrt 5 \).
[3 marks]
\(10x – 2{x^3} + 10 – 2{x^2}\) (A1)
Notes: The expansion may be seen in part (b)(ii).
[1 mark]
\(10 – 6{x^2} – 4x\) (A1)(ft)(A1)(ft)(A1)(ft)
Notes: Follow through from part (b)(i). Award (A1)(ft) for each correct term. Award at most (A1)(ft)(A1)(ft)(A0) if extra terms are seen.
[3 marks]
\(10 – 6{x^2} – 4x > 0\) (M1)
Notes: Award (M1) for their \(f’(x) > 0\). Accept equality or weak inequality.
\( – 1.67 < x < 1{\text{ }}\left( { – \frac{5}{3} < x < 1,{\text{ }} – 1.66666 \ldots < x < 1} \right)\) (A1)(ft)(A1)(ft)(G2)
Notes: Award (A1)(ft) for correct endpoints, (A1)(ft) for correct weak or strict inequalities. Follow through from part (b)(ii). Do not award any marks if there is no answer in part (b)(ii).
[3 marks]
(A1)(A1)(ft)(A1)(ft)(A1)
Notes: Award (A1) for correct scale; axes labelled and drawn with a ruler.
Award (A1)(ft) for their correct \(x\)-intercepts in approximately correct location.
Award (A1) for correct minimum and maximum points in approximately correct location.
Award (A1) for a smooth continuous curve with approximate correct shape. The curve should be in the given domain.
Follow through from part (a) for the \(x\)-intercepts.
[4 marks]
\((1.49,{\text{ }}13.9){\text{ }}\left( {(1.48702 \ldots ,{\text{ }}13.8714 \ldots )} \right)\) (G1)(ft)(G1)(ft)
Notes: Award (G1) for 1.49 and (G1) for 13.9 written as a coordinate pair. Award at most (G0)(G1) if parentheses are missing. Accept \(x = 1.49\) and \(y = 13.9\). Follow through from part (b)(i).
[2 marks]
Question
Consider the curve y = 2x3 − 9x2 + 12x + 2, for −1 < x < 3
Sketch the curve for −1 < x < 3 and −2 < y < 12.[4]
A teacher asks her students to make some observations about the curve.
Three students responded.
Nadia said “The x-intercept of the curve is between −1 and zero”.
Rick said “The curve is decreasing when x < 1 ”.
Paula said “The gradient of the curve is less than zero between x = 1 and x = 2 ”.
State the name of the student who made an incorrect observation.[1]
Find the value of y when x = 1 .[2]
Find \(\frac{{{\text{dy}}}}{{{\text{dx}}}}\).[3]
Show that the stationary points of the curve are at x = 1 and x = 2.[2]
Given that y = 2x3 − 9x2 + 12x + 2 = k has three solutions, find the possible values of k.[3]
Answer/Explanation
Markscheme
(A1)(A1)(A1)(A1)
Note: Award (A1) for correct window (condone a window which is slightly off) and axes labels. An indication of window is necessary. −1 to 3 on the x-axis and −2 to 12 on the y-axis and a graph in that window.
(A1) for correct shape (curve having cubic shape and must be smooth).
(A1) for both stationary points in the 1st quadrant with approximate correct position,
(A1) for intercepts (negative x-intercept and positive y intercept) with approximate correct position.
[4 marks]
Rick (A1)
Note: Award (A0) if extra names stated.
[1 mark]
2(1)3 − 9(1)2 + 12(1) + 2 (M1)
Note: Award (M1) for correct substitution into equation.
= 7 (A1)(G2)
[2 marks]
6x2 − 18x + 12 (A1)(A1)(A1)
Note: Award (A1) for each correct term. Award at most (A1)(A1)(A0) if extra terms seen.
[3 marks]
6x2 − 18x + 12 = 0 (M1)
Note: Award (M1) for equating their derivative to 0. If the derivative is not explicitly equated to 0, but a subsequent solving of their correct equation is seen, award (M1).
6(x − 1)(x − 2) = 0 (or equivalent) (M1)
Note: Award (M1) for correct factorization. The final (M1) is awarded only if answers are clearly stated.
Award (M0)(M0) for substitution of 1 and of 2 in their derivative.
x = 1, x = 2 (AG)
[2 marks]
6 < k < 7 (A1)(A1)(ft)(A1)
Note: Award (A1) for an inequality with 6, award (A1)(ft) for an inequality with 7 from their part (c) provided it is greater than 6, (A1) for their correct strict inequalities. Accept ]6, 7[ or (6, 7).
[3 marks]