Question
The diagram below shows the line PQ, whose equation is x + 2y = 12. The line intercepts the axes at P and Q respectively.

Find the coordinates of P and of Q.[3]
A second line with equation x − y = 3 intersects the line PQ at the point A. Find the coordinates of A.[3]
Answer/Explanation
Markscheme
\(0 + 2y = 12\) or \(x + 2(0) = 12\) (M1)
P(0, 6) (accept \(x = 0\), \(y = 6\)) (A1)
Q(12, 0) (accept \(x = 12\), \(y = 0\)) (A1) (C3)
Notes: Award (M1) for setting either value to zero.
Missing coordinate brackets receive (A0) the first time this occurs.
Award (A0)(A1)(ft) for P(0, 12) and Q(6, 0).[3 marks]
\(x + 2(x – 3) = 12\) (M1)
(6, 3) (accept \(x = 6\), \(y = 3\)) (A1)(A1) (C3)
Note: (A1) for each correct coordinate.
Missing coordinate brackets receive (A0)(A1) if this is the first time it occurs.[3 marks]
Question
The diagram shows the points M(a, 18) and B(24, 10) . The straight line BM intersects the y-axis at A(0, 26). M is the midpoint of the line segment AB.
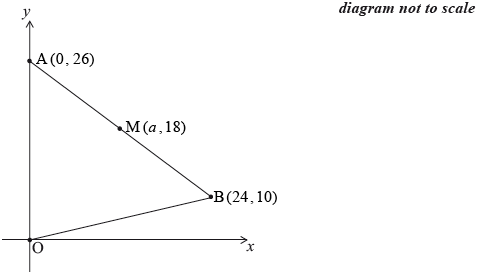
Write down the value of \(a\).[1]
Find the gradient of the line AB.[2]
Decide whether triangle OAM is a right-angled triangle. Justify your answer.[3]
Answer/Explanation
Markscheme
12 (A1) (C1)
Note: Award (A1) for \(\left( {12,18} \right)\).[1 mark]
\(\frac{{26 – 10}}{{0 – 24}}\) (M1)
Note: Accept \(\frac{{26 – 18}}{{0 – 12}}\) or \(\frac{{18 – 10}}{{12 – 24}}\) (or equivalent).
\( = – \frac{2}{3}{\text{ }}\left( { – \frac{{16}}{{24}},{\text{ }} – 0.666666 \ldots } \right)\) (A1) (C2)
Note: If either of the alternative fractions is used, follow through from their answer to part (a).
The answer is now (A1)(ft).[2 marks]
gradient of \({\text{OM}} = \frac{3}{2}\) (A1)(ft)
Note: Follow through from their answer to part (b).
\( – \frac{2}{3} \times \frac{3}{2}\) (M1)
Note: Award (M1) for multiplying their gradients.
Since the product is \(-1\), OAM is a right-angled triangle (R1)(ft)
Notes: Award the final (R1) only if their conclusion is consistent with their answer for the product of the gradients.
The statement that OAM is a right-angled triangle without justification is awarded no marks.
OR
\({(26 – 18)^2} + {12^2}\) and \({12^2} + {18^2}\) (A1)(ft)
\(\left( {{{(26 – 18)}^2} + {{12}^2}} \right) + ({12^2} + {18^2}) = {26^2}\) (M1)
Note: This method can also be applied to triangle OMB.
Follow through from (a).
Hence a right angled triangle (R1)(ft)
Note: Award the final (R1) only if their conclusion is consistent with their (M1) mark.
OR
\(OA = OB = 26\) (cm) an isosceles triangle (A1)
Note: Award (A1) for \(OA = 26\) (cm) and \(OB = 26\) (cm).
Line drawn from vertex to midpoint of base is perpendicular to the base (M1)
Conclusion (R1) (C3)
Note: Award, at most (A1)(M0)(R0) for stating that OAB is an isosceles triangle without any calculations.[3 marks]
Question
[Maximum mark: 7] [with / without GDC]
The following three lines \(l\)1, \(l\)2, and \(l\)3 are defined with equations
\(l\)1 : x + y = 5 , \(l\)2 : x – 2y = 8 , \(l\)3 : x = – 2
and are shown in the figure below.
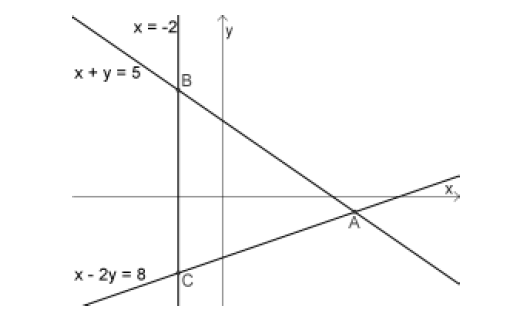
(a) Find the coordinates of the common point A between the lines \(l\)1 and \(l\)2.
(b) Write down the coordinates of the common point B between the lines \(l\)1 and \(l\)3 and
of the common point C between the lines \(l\)2 and \(l\)3.
(c) Hence, find the area of the triangle ABC.
Answer/Explanation
Ans.
(a) A(6,-1) (b) B(-2,7) C(-2,-5) (c) Area=\(\frac{12×8}{2}=48\)
Question
[Maximum mark: 8] [without GDC]
Consider the points A(2,7), B(5,11)
(a) Find the gradient of the line (AB).
(b) Write down the gradient of a perpendicular line to (AB).
(c) Let M be the midpoint M of the line segment [AB]. Find the coordinates of M.
(d) Find the distance between A and B (i.e. the length AB).
(e) Find the coordinates of the point C if B is the midpoint of the line segment [AC].
Answer/Explanation
Ans.
(a) \(m_{AB}=\frac{4}{3}\) (b) \(m_{AB}=-\frac{3}{4}\) (c) \(M(\frac{7}{2},9)\) (d) \(d=5\) (e)(8,15)
