Question
The following table shows the average weights ( y kg) for given heights (x cm) in a population of men.
| Heights (x cm) | 165 | 170 | 175 | 180 | 185 |
| Weights (y kg) | 67.8 | 70.0 | 72.7 | 75.5 | 77.2 |
The relationship between the variables is modelled by the regression equation \(y = ax + b\).
Write down the value of \(a\) and of \(b\).
The relationship between the variables is modelled by the regression equation \(y = ax + b\).
Hence, estimate the weight of a man whose height is 172 cm.
Write down the correlation coefficient.
State which two of the following describe the correlation between the variables.
| strong | zero | positive |
| negative | no correlation | weak |
Answer/Explanation
Markscheme
\(a = 0.486\) (exact) A1 N1
\(b = – 12.41\) (exact), \(-12.4\) A1 N1
[2 marks]
correct substitution (A1)
eg \(0.486(172) – 12.41\)
\(71.182\)
\(71.2\) (kg) A1 N2
[2 marks]
\(r = 0.997276\)
\(r = 0.997\) A1 N1
[1 mark]
strong, positive (must have both correct) A2 N2
[2 marks]
Question
The following table shows the amount of fuel (\(y\) litres) used by a car to travel certain distances (\(x\) km).
| Distance (x km) | 40 | 75 | 120 | 150 | 195 |
| Amount of fuel (y litres) | 3.6 | 6.5 | 9.9 | 13.1 | 16.2 |
This data can be modelled by the regression line with equation \(y = ax + b\).
Write down the value of \(a\) and of \(b\).
Explain what the gradient \(a\) represents.
Use the model to estimate the amount of fuel the car would use if it is driven \(110\) km.
Answer/Explanation
Markscheme
\(a = 0.0823604{\text{, }}b = 0.306186\)
\(a = 0.0824{\text{, }}b = 0.306\) A1A1 N2
[2 marks]
correct explanation with reference to number of litres
required for \(1\) km A1 N1
eg \(a\) represents the (average) amount of fuel (litres) required to drive \(1\) km, (average) litres per kilometre, (average) rate of change in fuel used for each km travelled
[1 marks]
valid approach (M1)
eg \(y = 0.0824(110) + 0.306\), sketch
\(9.36583\)
\(9.37\) (litres) A1 N2
[2 marks]
Question
The following table shows the Diploma score \(x\) and university entrance mark \(y\) for seven IB Diploma students.
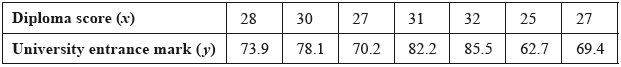
Find the correlation coefficient.
The relationship can be modelled by the regression line with equation \(y = ax + b\).
Write down the value of \(a\) and of \(b\).
Rita scored a total of \(26\) in her IB Diploma.
Use your regression line to estimate Rita’s university entrance mark.
Answer/Explanation
Markscheme
evidence of set up (M1)
eg\(\;\;\;\)correct value for \(r\) (or for \(a\) or \(r\), seen in (b))
\(0.996010\)
\(r = 0.996\;\;\;[0.996,{\text{ }}0.997]\) A1 N2
[2 marks]
\(a = 3.15037,{\text{ }}b = – 15.4393\)
\(a = 3.15{\text{ }}[3.15,{\text{ }}3.16],{\text{ }}b = – 15.4{\text{ }}[ – 15.5,{\text{ }} – 15.4]\) A1A1 N2
[2 marks]
substituting \(26\) into their equation (M1)
eg\(\;\;\;\)\(y = 3.15(26) – 15.4\)
\(66.4704\)
\(66.5{\text{ }}[66.4,{\text{ }}66.5]\) A1 N2
[2 marks]
Total [6 marks]
Question
The following table shows the average number of hours per day spent watching television by seven mothers and each mother’s youngest child.
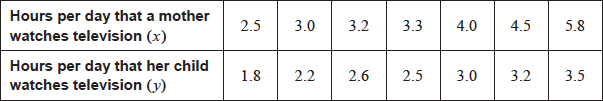
The relationship can be modelled by the regression line with equation \(y = ax + b\).
(i) Find the correlation coefficient.
(ii) Write down the value of \(a\) and of \(b\).
Elizabeth watches television for an average of \(3.7\) hours per day.
Use your regression line to predict the average number of hours of television watched per day by Elizabeth’s youngest child. Give your answer correct to one decimal place.
Answer/Explanation
Markscheme
(i) evidence of valid approach (M1)
eg\(\;\;\;\)\(1\) correct value for \(r\), (or for \(a\) or \(b\), seen in (ii))
\(0.946591\)
\(r = 0.947\) A1 N2
(ii) \(a = 0.500957,{\text{ }}b = 0.803544\)
\(a = 0.501,{\text{ }}b = 0.804\) A1A1 N2
[4 marks]
substituting \(x = 3.7\) into their equation (M1)
eg\(\;\;\;0.501(3.7) + 0.804\)
\(2.65708\;\;\;\)(\(2\) hours \(39.4252\) minutes) (A1)
\(y = 2.7\) (hours) (must be correct \(1\) dp, accept \(2\) hours \(39.4\) minutes) A1 N3
[3 marks]
Total [7 marks]
Question
The following table shows values of ln x and ln y.
The relationship between ln x and ln y can be modelled by the regression equation ln y = a ln x + b.
Find the value of a and of b.
Use the regression equation to estimate the value of y when x = 3.57.
The relationship between x and y can be modelled using the formula y = kxn, where k ≠ 0 , n ≠ 0 , n ≠ 1.
By expressing ln y in terms of ln x, find the value of n and of k.
Answer/Explanation
Markscheme
valid approach (M1)
eg one correct value
−0.453620, 6.14210
a = −0.454, b = 6.14 A1A1 N3
[3 marks]
correct substitution (A1)
eg −0.454 ln 3.57 + 6.14
correct working (A1)
eg ln y = 5.56484
261.083 (260.409 from 3 sf)
y = 261, (y = 260 from 3sf) A1 N3
Note: If no working shown, award N1 for 5.56484.
If no working shown, award N2 for ln y = 5.56484.
[3 marks]
METHOD 1
valid approach for expressing ln y in terms of ln x (M1)
eg \({\text{ln}}\,y = {\text{ln}}\,\left( {k{x^n}} \right),\,\,{\text{ln}}\,\left( {k{x^n}} \right) = a\,{\text{ln}}\,x + b\)
correct application of addition rule for logs (A1)
eg \({\text{ln}}\,k + {\text{ln}}\,\left( {{x^n}} \right)\)
correct application of exponent rule for logs A1
eg \({\text{ln}}\,k + n\,{\text{ln}}\,x\)
comparing one term with regression equation (check FT) (M1)
eg \(n = a,\,\,b = {\text{ln}}\,k\)
correct working for k (A1)
eg \({\text{ln}}\,k = 6.14210,\,\,\,k = {e^{6.14210}}\)
465.030
\(n = – 0.454,\,\,k = 465\) (464 from 3sf) A1A1 N2N2
METHOD 2
valid approach (M1)
eg \({e^{{\text{ln}}\,y}} = {e^{a\,{\text{ln}}\,x + b}}\)
correct use of exponent laws for \({e^{a\,{\text{ln}}\,x + b}}\) (A1)
eg \({e^{a\,{\text{ln}}\,x}} \times {e^b}\)
correct application of exponent rule for \(a\,{\text{ln}}\,x\) (A1)
eg \({\text{ln}}\,{x^a}\)
correct equation in y A1
eg \(y = {x^a} \times {e^b}\)
comparing one term with equation of model (check FT) (M1)
eg \(k = {e^b},\,\,n = a\)
465.030
\(n = – 0.454,\,\,k = 465\) (464 from 3sf) A1A1 N2N2
METHOD 3
valid approach for expressing ln y in terms of ln x (seen anywhere) (M1)
eg \({\text{ln}}\,y = {\text{ln}}\,\left( {k{x^n}} \right),\,\,{\text{ln}}\,\left( {k{x^n}} \right) = a\,{\text{ln}}\,x + b\)
correct application of exponent rule for logs (seen anywhere) (A1)
eg \({\text{ln}}\,\left( {{x^a}} \right) + b\)
correct working for b (seen anywhere) (A1)
eg \(b = {\text{ln}}\,\left( {{e^b}} \right)\)
correct application of addition rule for logs A1
eg \({\text{ln}}\,\left( {{e^b}{x^a}} \right)\)
comparing one term with equation of model (check FT) (M1)
eg \(k = {e^b},\,\,n = a\)
465.030
\(n = – 0.454,\,\,k = 465\) (464 from 3sf) A1A1 N2N2
[7 marks]

