Question
The graph of a function f passes through the point (ln4, 20).
Given that f ′(x) = 6e2x , find f (x).
Answer/Explanation
Ans:
evidence of integration
eg \(\int f'(x)dx, \int 6e^{2x}\)
correct integration (accept missing + c)
eg \(\frac{1}{2}\times 6e^{2x}, 3e^{2x}\) +c
substituting initial condition into their integrated expression (must have +c )
eg \(3e^{2\times ln4}\)+c = 20
correct application of log (\(a^{b})\)= b log a rule (seen anywhere)
eg 2ln4 = ln16, eln16, ln42
correct application of elna= a rule (seen anywhere)
eg \(e^{ln16}= 16, (e^{ln4})^{2}=4^{2}\)
correct working
eg \(3\times 16+c= 20, 3\times (4^{2})+c= 20, c=20 c=-28\)
\(f(x)=3e^{2x}- 28\)
Question
Let \(f(x) = \int {\frac{{12}}{{2x – 5}}} {\rm{d}}x\) , \(x > \frac{5}{2}\) . The graph of \(f\) passes through (\(4\), \(0\)) .
Find \(f(x)\) .
Answer/Explanation
Markscheme
attempt to integrate which involves \(\ln \) (M1)
eg \(\ln (2x – 5)\) , \(12\ln 2x – 5\) , \(\ln 2x\)
correct expression (accept absence of \(C\))
eg \(12\ln (2x – 5)\frac{1}{2} + C\) , \(6\ln (2x – 5)\) A2
attempt to substitute (4,0) into their integrated f (M1)
eg \(0 = 6\ln (2 \times 4 – 5)\) , \(0 = 6\ln (8 – 5) + C\)
\(C = – 6\ln 3\) (A1)
\(f(x) = 6\ln (2x – 5) – 6\ln 3\) \(\left( { = 6\ln \left( {\frac{{2x – 5}}{3}} \right)} \right)\) (accept \(6\ln (2x – 5) – \ln {3^6}\) ) A1 N5
Note: Exception to the FT rule. Allow full FT on incorrect integration which must involve \(\ln\).
[6 marks]
Question
Given that \(\int_0^5 {\frac{2}{{2x + 5}}} {\rm{d}}x = \ln k\) , find the value of k .
Answer/Explanation
Markscheme
correct integration, \(2 \times \frac{1}{2}\ln (2x + 5)\) A1A1
Note: Award A1 for \(2 \times \frac{1}{2}( = 1)\) and A1 for \(\ln (2x + 5)\) .
evidence of substituting limits into integrated function and subtracting (M1)
e.g. \(\ln (2 \times 5 + 5) – \ln (2 \times 0 + 5)\)
correct substitution A1
e.g. \(\ln 15 – \ln 5\)
correct working (A1)
e.g. \(\ln \frac{{15}}{5},\ln 3\)
\(k = 3\) A1 N3
[6 marks]
Question
Let \(f(x) = \int {\frac{{12}}{{2x – 5}}} {\rm{d}}x\) , \(x > \frac{5}{2}\) . The graph of \(f\) passes through (\(4\), \(0\)) .
Find \(f(x)\) .
Answer/Explanation
Markscheme
attempt to integrate which involves \(\ln \) (M1)
eg \(\ln (2x – 5)\) , \(12\ln 2x – 5\) , \(\ln 2x\)
correct expression (accept absence of \(C\))
eg \(12\ln (2x – 5)\frac{1}{2} + C\) , \(6\ln (2x – 5)\) A2
attempt to substitute (4,0) into their integrated f (M1)
eg \(0 = 6\ln (2 \times 4 – 5)\) , \(0 = 6\ln (8 – 5) + C\)
\(C = – 6\ln 3\) (A1)
\(f(x) = 6\ln (2x – 5) – 6\ln 3\) \(\left( { = 6\ln \left( {\frac{{2x – 5}}{3}} \right)} \right)\) (accept \(6\ln (2x – 5) – \ln {3^6}\) ) A1 N5
Note: Exception to the FT rule. Allow full FT on incorrect integration which must involve \(\ln\).
[6 marks]
Question
Let \(f:x \mapsto {\sin ^3}x\) .
(i) Write down the range of the function f .
(ii) Consider \(f(x) = 1\) , \(0 \le x \le 2\pi \) . Write down the number of solutions to this equation. Justify your answer.
Find \(f'(x)\) , giving your answer in the form \(a{\sin ^p}x{\cos ^q}x\) where \(a{\text{, }}p{\text{, }}q \in \mathbb{Z}\) .
Let \(g(x) = \sqrt 3 \sin x{(\cos x)^{\frac{1}{2}}}\) for \(0 \le x \le \frac{\pi }{2}\) . Find the volume generated when the curve of g is revolved through \(2\pi \) about the x-axis.
Answer/Explanation
Markscheme
(i) range of f is \([ – 1{\text{, }}1]\) , \(( – 1 \le f(x) \le 1)\) A2 N2
(ii) \({\sin ^3}x \Rightarrow 1 \Rightarrow \sin x = 1\) A1
justification for one solution on \([0{\text{, }}2\pi ]\) R1
e.g. \(x = \frac{\pi }{2}\) , unit circle, sketch of \(\sin x\)
1 solution (seen anywhere) A1 N1
[5 marks]
\(f'(x) = 3{\sin ^2}x\cos x\) A2 N2
[2 marks]
using \(V = \int_a^b {\pi {y^2}{\rm{d}}x} \) (M1)
\(V = \int_0^{\frac{\pi }{2}} {\pi (\sqrt 3 } \sin x{\cos ^{\frac{1}{2}}}x{)^2}{\rm{d}}x\) (A1)
\( = \pi \int_0^{\frac{\pi }{2}} {3{{\sin }^2}x\cos x{\rm{d}}x} \) A1
\(V = \pi \left[ {{{\sin }^3}x} \right]_0^{\frac{\pi }{2}}\) \(\left( { = \pi \left( {{{\sin }^3}\left( {\frac{\pi }{2}} \right) – {{\sin }^3}0} \right)} \right)\) A2
evidence of using \(\sin \frac{\pi }{2} = 1\) and \(\sin 0 = 0\) (A1)
e.g. \(\pi \left( {1 – 0} \right)\)
\(V = \pi \) A1 N1
[7 marks]
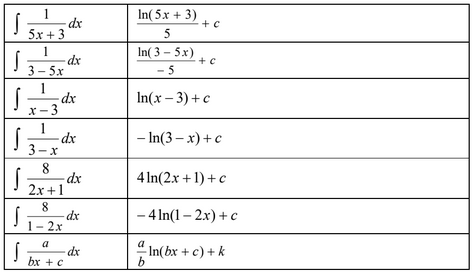
Question
Find the following integrals
Answer/Explanation
Ans: