Question
A pendulum bob is displaced until its centre is 30 mm above its rest position and then released. The motion of the pendulum is lightly damped.
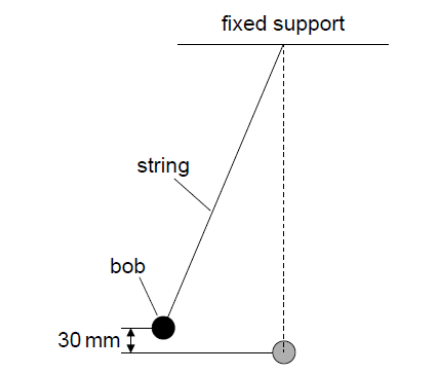
a. Describe what is meant by damped motion.[1]
b. After one complete oscillation, the height of the pendulum bob above the rest position has decreased to $28 \mathrm{~mm}$. Calculate the $Q$ factor.[2]
c. The point of suspension now vibrates horizontally with small amplitude and frequency $0.80 \mathrm{~Hz}$, which is the natural frequency of the pendulum.
The amount of damping is unchanged.

When the pendulum oscillates with a constant amplitude the energy stored in the system is 20 mJ. Calculate the average power, in W, delivered to the pendulum by the driving force.
▶️Answer/Explanation
Ans:
a. a situation in which a resistive force opposes the motion
OR amplitude/energy decreases with time
b. $\mathrm{Q}=2 \pi \times \frac{30}{30-28}=94.25 \approx 94$
c. $94=2 \pi \times 0.80 \times \frac{0.020}{\text { power loss }}$ power added $=1.1 \times 10^{-3} « \mathrm{~W} »$
Question
The graph shows the variation with time t of the total energy E of a damped oscillating system.
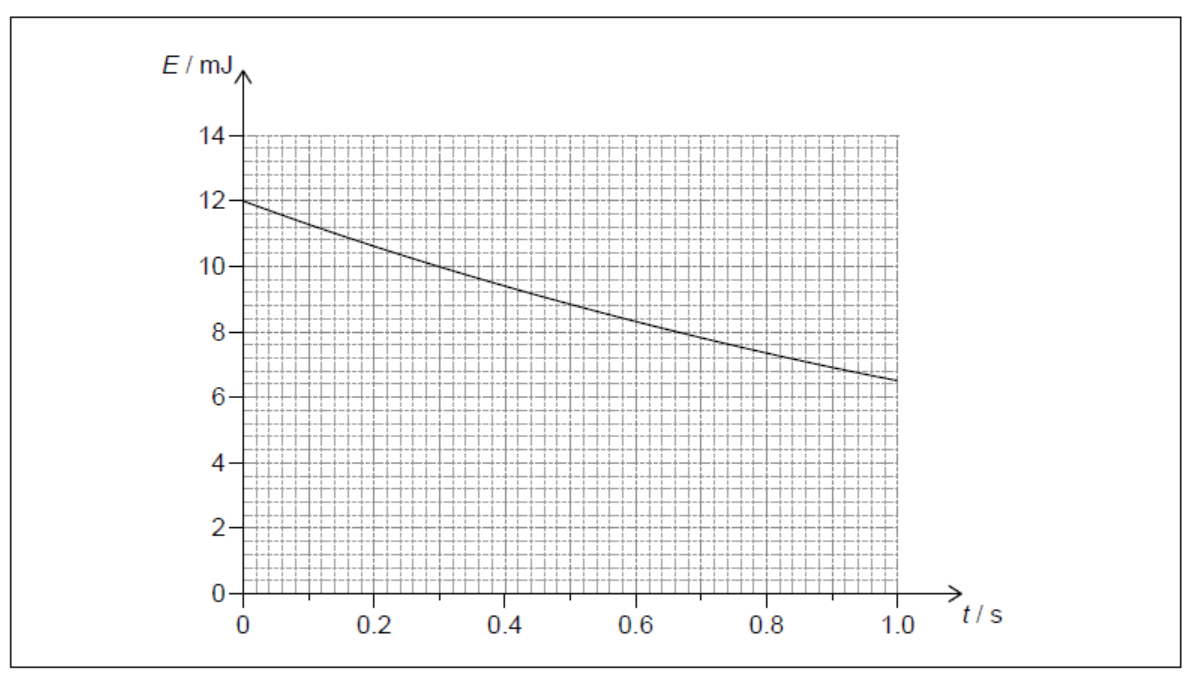
a. The $Q$ factor for the system is 25 . Determine the period of oscillation for this system. [3]
b. Another system has the same initial total energy and period as that in (a) but its $Q$ factor is greater than 25 . Without any calculations, draw on the
graph, the variation with time of the total energy of this system.[1]
▶️Answer/Explanation
Ans:
a. ALTERNATIVE 1
$
\begin{gathered}
« Q=2 \pi \frac{E_0}{E_0-E_1} » \Rightarrow E_1=\left(1-\frac{2 \pi}{Q}\right) E_0 \checkmark \\
E_1 \ll=\left(1-\frac{2 \pi}{25}\right) \times 12 »=9.0 \ll \mathrm{mJ} »
\end{gathered}
$
reading off the graph, period is 0.48 «s»
Allow correct use of any value of $E_0$, not only at the time $=0$.
Allow answer from interval $0.42-0.55 \mathrm{~s}$
ALTERNATIVE 2
use of $Q=2 \pi f \frac{\text { energy stored }}{\text { power loss }}$
energy stored $=12$ «mJ» $A N D$ power loss $=5.6$ «mJ/s»
«f $=1.86 \mathrm{~s}$ so» period is 0.54 «s» $\checkmark$
Allow answer from interval $0.42-0.55 \mathrm{~s}$.
Award [3] for bald correct answer.
b. similar shape graph starting at $12 \mathrm{~mJ}$ and above the original