Question
The function f is defined by f(x) = \(\frac{7x + 7}{2x – 4}\) for \(x\epsilon \mathbb{R}\), x ≠ 2.
(a) Find the zero of f(x).
(b) For the graph of y = f(x), write down the equation of
(i) the vertical asymptote;
(ii) the horizontal asymptote.
(c) Find \(f^{-1}\) (x), the inverse function of f(x).
Answer/Explanation
Answer:
(a) recognizing f(x) = 0
x = -1
(b) (i) x = 2 (must be an equation with x)
(ii) y = \(\frac{7}{2}\) (must be an equation with y)
(c) EITHER
interchanging x and y
2xy – 4x = 7y + 7
correct working with y terms on the same side: 2xy – 7y = 4x + 7
OR
2yx – 4y = 7x +7
correct working with x terms on the same side: 2yx – 7x = 4x + 7
interchanging x and y OR making x the subject x = \(\frac{4y + 7}{2y – 7}\)
THEN
\(f^{-1}\)(x) = \(\frac{4x + 7}{2x – 7}\) (or equivalent) (\(x ≠ \frac{7}{2}\))
Question
Let \(f(x) = m – \frac{1}{x}\), for \(x \ne 0\). The line \(y = x – m\) intersects the graph of \(f\) in two distinct points. Find the possible values of \(m\).
Answer/Explanation
Markscheme
valid approach (M1)
eg\(\,\,\,\,\,\)\(f = y,{\text{ }}m – \frac{1}{x} = x – m\)
correct working to eliminate denominator (A1)
eg\(\,\,\,\,\,\)\(mx – 1 = x(x – m),{\text{ }}mx – 1 = {x^2} – mx\)
correct quadratic equal to zero A1
eg\(\,\,\,\,\,\)\({x^2} – 2mx + 1 = 0\)
correct reasoning R1
eg\(\,\,\,\,\,\)for two solutions, \({b^2} – 4ac > 0\)
correct substitution into the discriminant formula (A1)
eg\(\,\,\,\,\,\)\({( – 2m)^2} – 4\)
correct working (A1)
eg\(\,\,\,\,\,\)\(4{m^2} > 4,{\text{ }}{m^2} = 1\), sketch of positive parabola on the \(x\)-axis
correct interval A1 N4
eg\(\,\,\,\,\,\)\(\left| m \right| > 1,{\text{ }}m < – 1\) or \(m > 1\)
[7 marks]
Question
Let \(f(x) = 3x – 2\) and \(g(x) = \frac{5}{{3x}}\), for \(x \ne 0\).
Let \(h(x) = \frac{5}{{x + 2}}\), for \(x \geqslant 0\). The graph of h has a horizontal asymptote at \(y = 0\).
Find \({f^{ – 1}}(x)\).[2]
Show that \(\left( {g \circ {f^{ – 1}}} \right)(x) = \frac{5}{{x + 2}}\).[2]
Find the \(y\)-intercept of the graph of \(h\).[2]
Hence, sketch the graph of \(h\).[3]
For the graph of \({h^{ – 1}}\), write down the \(x\)-intercept;[1]
For the graph of \({h^{ – 1}}\), write down the equation of the vertical asymptote.[1]
Given that \({h^{ – 1}}(a) = 3\), find the value of \(a\).[3]
Answer/Explanation
Markscheme
interchanging \(x\) and \(y\) (M1)
eg \(x = 3y – 2\)
\({f^{ – 1}}(x) = \frac{{x + 2}}{3}{\text{ }}\left( {{\text{accept }}y = \frac{{x + 2}}{3},{\text{ }}\frac{{x + 2}}{3}} \right)\) A1 N2
[2 marks]
attempt to form composite (in any order) (M1)
eg \(g\left( {\frac{{x + 2}}{3}} \right),{\text{ }}\frac{{\frac{5}{{3x}} + 2}}{3}\)
correct substitution A1
eg \(\frac{5}{{3\left( {\frac{{x + 2}}{3}} \right)}}\)
\(\left( {g \circ {f^{ – 1}}} \right)(x) = \frac{5}{{x + 2}}\) AG N0
[2 marks]
valid approach (M1)
eg \(h(0),{\text{ }}\frac{5}{{0 + 2}}\)
\(y = \frac{5}{2}{\text{ }}\left( {{\text{accept (0, 2.5)}}} \right)\) A1 N2
[2 marks]
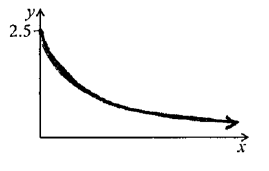A1A2 N3
Notes: Award A1 for approximately correct shape (reciprocal, decreasing, concave up).
Only if this A1 is awarded, award A2 for all the following approximately correct features: y-intercept at \((0, 2.5)\), asymptotic to x-axis, correct domain \(x \geqslant 0\).
If only two of these features are correct, award A1.
[3 marks]
\(x = \frac{5}{2}{\text{ }}\left( {{\text{accept (2.5, 0)}}} \right)\) A1 N1
[1 mark]
\(x = 0\) (must be an equation) A1 N1
[1 mark]
METHOD 1
attempt to substitute \(3\) into \(h\) (seen anywhere) (M1)
eg \(h(3),{\text{ }}\frac{5}{{3 + 2}}\)
correct equation (A1)
eg \(a = \frac{5}{{3 + 2}},{\text{ }}h(3) = a\)
\(a = 1\) A1 N2
[3 marks]
METHOD 2
attempt to find inverse (may be seen in (d)) (M1)
eg \(x = \frac{5}{{y + 2}},{\text{ }}{h^{ – 1}} = \frac{5}{x} – 2,{\text{ }}\frac{5}{x} + 2\)
correct equation, \(\frac{5}{x} – 2 = 3\) (A1)
\(a = 1\) A1 N2
[3 marks]
Question
Let \(f(x) = p + \frac{9}{{x – q}}\), for \(x \ne q\). The line \(x = 3\) is a vertical asymptote to the graph of \(f\).
Write down the value of \(q\).[1]
The graph of \(f\) has a \(y\)-intercept at \((0,{\text{ }}4)\).
Find the value of \(p\).[4]
The graph of \(f\) has a \(y\)-intercept at \((0,{\text{ }}4)\).
Write down the equation of the horizontal asymptote of the graph of \(f\).[1]
Answer/Explanation
Markscheme
\(q = 3\) A1 N1
[1 mark]
correct expression for \(f(0)\) (A1)
eg\(\;\;\;p + \frac{9}{{0 – 3}},{\text{ }}4 = p + \frac{9}{{ – q}}\)
recognizing that \(f(0) = 4\;\;\;\)(may be seen in equation) (M1)
correct working (A1)
eg\(\;\;\;4 = p – 3\)
\(p = 7\) A1 N3
[3 marks]
\(y = 7\;\;\;\)(must be an equation, do not accept \(p = 7\) A1 N1
[1 mark]
Total [6 marks]
Question
Let \(f(x)=\frac{2x-4}{x+2}\) (a) Complete the following table 
(b)Find the corresponding position to the horizontal asymptote of f(x), under the following transformations: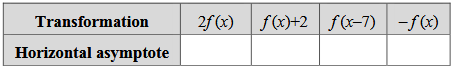 
(c)The point A(3,0.5) lies on the graph of )(xf. Find the corresponding position to the point A under the transformation y = 2f (3x)+5 (d) Sketch the graphs of f(x) and \(\frac{1}{f(x)}\) by indicating any asymptotes and intersections with x- and y- axes.
(e) Sketch the graph of f-1(x) by indicating any asymptotes and intersections with x- and y- axes. 
Answer/Explanation
Ans
(a) 
(b)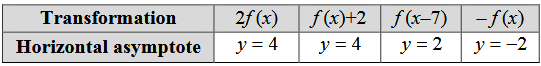 
(c) The image of point A(3,0.5) under the transformation 2f (3x)+5 is A ́(1,7). Step by step: f(x) (3,0.5) f(3x) (1,0.5) 2f(3x) (1,2) 2f(3x)+5 (1,6)(d), (e) \(f(x)=\frac{2x-4}{x+2}, \frac{1}{f(x)}=\frac{x+2}{2x-4}, f^{-1}(x)=\frac{-2x-4}{x-2}\) However, you should be able to sketch the graphs without a GDC 