Question
P (4, 1) and Q (0, –5) are points on the coordinate plane. Determine the
a. (i) coordinates of M, the midpoint of P and Q.
▶️Answer/Explanation
Ans: (2, – 2) parentheses not required. (A1)
▶️Answer/Explanation
Ans: gradient of PQ \( = \left( {\frac{{ – 5 – 1}}{{0 – 4}}} \right) = \frac{6}{4} = \frac{3}{2}(1.5)\) (M1)(A1)
(M1) for gradient formula with correct substitution
Award (A1) for \(y = \frac{3}{2}x – 5\) with no other working
▶️Answer/Explanation
Ans: gradient of perpendicular is \( – \frac{2}{3}\) (A1)(ft) (C4)[4 marks]
▶️Answer/Explanation
Ans: \(\left( {\frac{{k + 2}}{{0 – 2}}} \right) = – \frac{2}{3}\), \(k = – \frac{2}{3}\) or \(y = – \frac{2}{3}x + c\), \(c = – \frac{2}{3}\therefore k = – \frac{2}{3}\) (M1)(A1)(ft)
Allow (\(0, – \frac{2}{3}\))
(M1) is for equating gradients or substituting gradient into \(y = mx + c\) (C2)[2 marks]
Question
The mid-point, M, of the line joining A(s , 8) to B(−2, t) has coordinates M(2, 3).
a. Calculate the values of s and t.[2]
▶️Answer/Explanation
Ans:
\(s = 6\) (A1)
\(t = – 2\) (A1) (C2)[2 marks]
▶️Answer/Explanation
(A1) for gradient of AM or BM \( = \frac{5}{4}\)
\({\text{Perpendicular gradient}} = – \frac{4}{5}\) (A1)(ft)
Equation of perpendicular bisector is
\(y = – \frac{4}{5}x + c\)
\(3 = – \frac{4}{5}(2) + c\) (M1)
\(c = 4.6\)
\(y = -0.8x + 4.6\)
or \(5y = -4x + 23\) (A1)(ft) (C4)[4 marks]
Question
a.Write down the gradient of the line \(y = 3x + 4\).[1]
▶️Answer/Explanation
Ans: \(3\) (A1) (C1)[1 mark]
b. Find the gradient of the line which is perpendicular to the line \(y = 3x + 4\).[1]
▶️Answer/Explanation
Ans: \( – 1/3\) (ft) from (a) (A1)(ft) (C1)[1 mark]
c.Find the equation of the line which is perpendicular to \(y = 3x + 4\) and which passes through the point \((6{\text{, }}7)\).[2]
▶️Answer/Explanation
Ans: Substituting \((6{\text{, }}7)\) in \(y ={\text{their }}mx + c\) or equivalent to find \(c\). (M1)
\(y = \frac{{ – 1}}{3}x + 9\) or equivalent (A1)(ft) (C2)[2 marks]
d. Find the coordinates of the point of intersection of these two lines.[2]
▶️Answer/Explanation
Ans: \((1.5{\text{, }}8.5)\) (A1)(A1)(ft) (C2)
Note: Award (A1) for \(1.5\), (A1) for \(8.5\). (ft) from (c), brackets not required.[2 marks]
Question
A straight line, \({L_1}\) , has equation \(x + 4y + 34 = 0\) .
Find the gradient of \({L_1}\) .[2]
a. The equation of line \({L_2}\) is \(y = mx\) . \({L_2}\) is perpendicular to \({L_1}\) .
Find the value of \(m\).[2]
▶️Answer/Explanation
Ans: \(4y = – x – 34\) or similar rearrangement (M1)
\({\text{Gradient}} = – \frac{1}{4}\) (A1) (C2)
▶️Answer/Explanation
Ans: \(m = 4\) (A1)(ft)(A1)(ft) (C2)
Note: (A1) Change of sign
(A1) Use of reciprocal[2 marks]
▶️Answer/Explanation
Ans: Reasonable attempt to solve equations simultaneously (M1)
\(( – 2{\text{, }} – 8)\) (A1)(ft) (C2)
Note: Accept \(x = – 2\) \(y = – 8\). Award (A0) if brackets not included.[2 marks]
Question
A and B are points on a straight line as shown on the graph below.
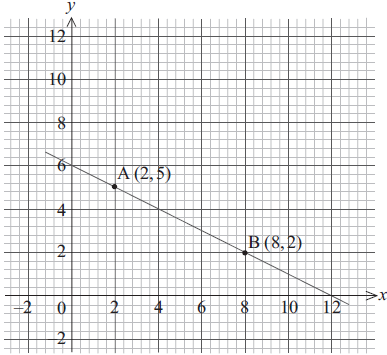
a.Write down the y-intercept of the line AB.[1]
▶️Answer/Explanation
Ans: 6
OR
(0, 6) (A1) (C1)[1 mark]
▶️Answer/Explanation
Ans: \(\frac{{(2 – 5)}}{{(8 – 2)}}\) (M1)
Note: Award (M1) for substitution in gradient formula.
\( = – \frac{1}{2}\) (A1) (C2)[2 marks]
Show θ on the diagram.[1]
▶️Answer/Explanation
Ans: Angle clearly identified. (A1) (C1)[1 mark]
Calculate the size of θ.[2]
▶️Answer/Explanation
Ans: \(\tan \theta = \frac{1}{2}\) (or equivalent fraction) (M1)
\(\theta = 26.6^\circ\) (A1)(ft) (C2)
Note: (ft) from (b).
Accept alternative correct trigonometrical methods.[2 marks]
