Question
A botanist is conducting an experiment which studies the growth of plants.
The heights of the plants are measured on seven different days.
The following table shows the number of days, d, that the experiment has been running and the average height, hcm, of the plants on each of those days.
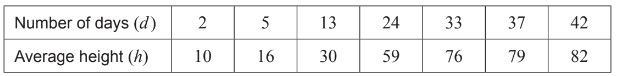
(a) The regression line of h on d for this data can be written in the form h = ad + b.
Find the value of a and the value of b.
(b) Write down the value of the Pearson’s product-moment correlation coefficient, r.
(c) Use your regression line to estimate the average height of the plants when the
experiment has been running for 20 days.
▶️Answer/Explanation
Answer:
(a) a =1.93258…, b = 7.21662…
a =1.93, b = 7.22
(b) r = 0.991087…
r = 0.991
(c) attempt to substitute d = 20 into their equation
height 45.8683… =
height 45.9 = (cm)
Question
At a café, the waiting time between ordering and receiving a cup of coffee is dependent upon the number of customers who have already ordered their coffee and are waiting to receive it. Sarah, a regular customer, visited the café on five consecutive days. The following table shows the number of customers, x , ahead of Sarah who have already ordered and are waiting to receive their coffee and Sarah’s waiting time, y minutes.
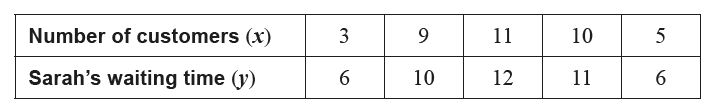
The relationship between x and y can be modelled by the regression line of y on x with equation y = ax + b .
(a) (i) Find the value of a and the value of b
(ii) Write down the value of Pearson’s product-moment correlation coefficient, r . [3]
(b) Interpret, in context, the value of a found in part (a)(i). [1]
On another day, Sarah visits the café to order a coffee. Seven customers have already ordered their coffee and are waiting to receive it.
(c) Use the result from part (a)(i) to estimate Sarah’s waiting time to receive her coffee. [2]
▶️Answer/Explanation
Ans:
(a)(i) From graphing calculator, we have $y=0.805x+2.88$, i.e., $a=0.805$ and $b=2.88$.
(a)(ii) Again, from graphing calculator, $r=0.978$.
(b) For each increase in customer ($x$), the corresponding waiting time ($y$) increases by $a=0.805$ minutes.
(c) When $x=7$, we have $y=8.52$, thus, Sarah has to wait for $8.52$ minutes to receive her coffee.
