Question
A mass–spring system oscillates horizontally on a frictionless surface. The mass has an acceleration a when its displacement from its equilibrium position is x .
The variation of a with x is modelled in two different ways, A and B, by the graphs shown.

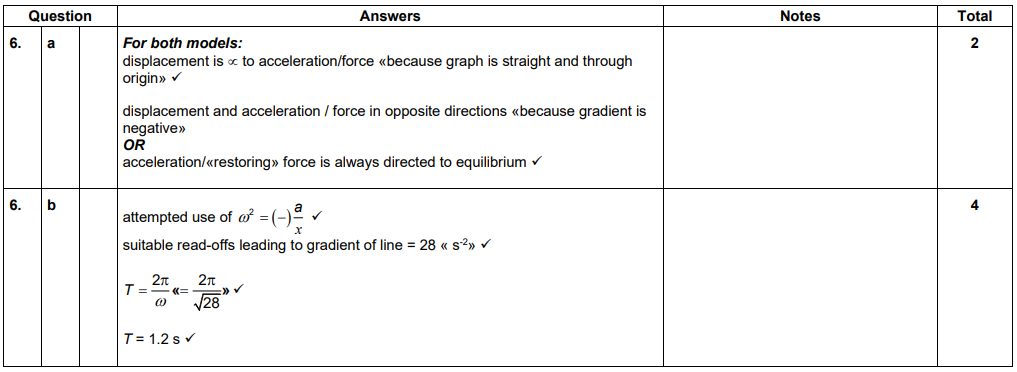
(a) Outline two reasons why both models predict that the motion is simple harmonic
when a is small. [2]
(b) Determine the time period of the system when a is small. [4]
(c) Outline, without calculation, the change to the time period of the system for the model
represented by graph B when a is large. [2]
(d) The graph shows for model A the variation with x of elastic potential energy Ep stored
in the spring.

Describe the graph for model B. [2]
Answer/Explanation
Ans.

This question is about simple harmonic motion (SHM) and sound.
The diagram shows a section of continuous track of a long-playing (LP) record. The stylus (needle) is placed in the track of the record.

As the LP record rotates, the stylus moves because of changes in the width and position of the track. These movements are converted into sound waves by an electrical system and a loudspeaker.
A recording of a single-frequency musical note is played. The graph shows the variation in horizontal acceleration of the stylus with horizontal displacement.

The mass of the stylus is \(5.5 \times {10^{ – 4}}{\text{ kg}}\). Determine the maximum kinetic energy of the stylus.
Answer/Explanation
Markscheme
\({x_0} = 4.8 \times {10^{ – 5}}{\text{ (m)}}\);
\({E_{\text{k}}} = \frac{1}{2}m{\omega ^2}x_0^2 = 9.9 \times {10^{ – 7}}{\text{ (J)}}\)\(\,\,\,\)or\(\,\,\,\)\(1.0 \times {10^{ – 6}}{\text{ (J)}}\);
Allow [2] for a bald correct answer.