Question
(a) The table shows some values for \(y=2x^{3}-4x^{2}+3.\)

(i) Complete the table.
(ii) On the grid, draw the graph of \(y=2x^{3}-4x^{2}+3\) for \(-1\leq x\leq 2.\)

(iii) Use your graph to solve the equation \(2x^{3}-4x^{2}+3=1.5\)
x = …………………… or x = …………………… or x = ……………………
(iv) The equation \(2x^{3}-4x^{2}+3=k\) has only one solution for \(-1\leq k\leq 2.\)
Write down a possible integer value of k.
………………………………………….
(b)
(i) On the grid, draw the tangent to the curve at x = 1.
(ii) Use your tangent to estimate the gradient of the curve at x = 1.
………………………………………….
(iii) Write down the equation of your tangent in the form y=mx+c
y = ………………………………………….
Answer/Explanation
(a)(i) 3 2.25 1
(ii) Fully correct smooth curve
(iii) −0.6 to −0.51, 0.75 to 0.85,
1.7 to 1.85
(iv) −3 or −2 or −1 or 0
(b)(i) Tangent ruled at x = 1
(ii) 4.4 to 5.6
(iii) y = (4.4 to 5.6)x – (1.8 to 2.2)
Question
(a) Find the gradient of the curve \(y=2x^{3}-7x+4\) when x=-2
………………………………………..
(b) A is the point (7, 2) and B is the point (−5, 8).
(i) Calculate the length of AB.
………………………………………….
(ii) Find the equation of the line that is perpendicular to AB and that passes through
the point (−1, 3).
Give your answer in the form y=mx+c.
y = …………………………………………
(iii) AB is one side of the parallelogram ABCD and
•\( \vec{BC}=\begin{pmatrix}-a\\ -b\end{pmatrix}\) where a>0 and b>0.
• the gradient of BC is 1
• \(\left | \vec{BC} \right |= 8.\)
Find the coordinates of D.
( ………………….. , ………………….)
Answer/Explanation
(a) 17
(b)(i) 13.4 or 13.41 to 13.42
(b)(ii)y=2x+5 final answer
(iii) ( 5, 0)
Question
(a) The grid shows the graph of \(y=a+bx^{2}\)
The graph passes through the points with coordinates (0, 4) and (1, 1).
(i) Find the value of a and the value of b.
a = …………………………………………
b = …………………………………………
(ii) Write down the equation of the tangent to the graph at (0, 4).
………………………………………….
(iii) The equation of the tangent to the graph at x =-1 is y=6x+7.
Find the equation of the tangent to the graph at x = 1.
………………………………………….
(b) The table shows some values for\( y=1+\frac{5}{3-x} \)for \(-2\leq x\leq 1.5.\)
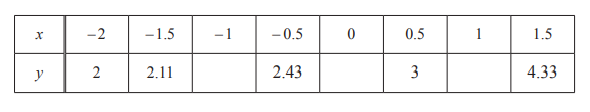
(i) Complete the table.
(ii) On the grid, draw the graph of \(y=1+\frac{5}{3-x}\) for \(-2\leq x\leq 1.5.\)
(c) (i) Write down the values of x where the two graphs intersect.
x = ………………. or x = ………………
(ii) The answers to part(c)(i) are two solutions of a cubic equation in terms of x.
Find this equation in the form \(ax^{3}+bx^{2}+cx+d=0\) where a, b, c and d are integers.
…………………………………………………………………….
Answer/Explanation
(a)(i) [a = ] 4
[b = ] – 3
(ii)y=4
(iii) y = − 6x + 7 oe final answer
(b)(i) 2.25 2.67 3.5
(ii) correct curve

(c)(i) –0.78 to –0.72 and 0.55 to 0.59
(ii)\(3x^{3}-9x^{2}-3x+4=0 \)final answer
