Question
(a) Naga has n marbles.
Panav has three times as many marbles as Naga.
Naga loses 5 marbles and Panav buys 10 marbles.
Together they now have more than 105 marbles.
Write down and solve an inequality in n.
………………………………………….
(b) y is inversely proportional to \(x^{2}.\)
When x = 4, y = 7.5.
Find y when x = 5.
y = ………………………………………….
(c) Find the nth term of each sequence.
(i) 4 2 0 -2 -4 …
………………………………………….
(ii) 1 7 17 31 49 …
………………………………………….
Answer/Explanation
(a) n − 5 + 3n + 10 > 105 or better
n > 25 final answer
(b) 4.8
(c)(i) 6 − 2n final answer
(ii) \(2n^{2}-1\) final answer
Question
(a) Solve the simultaneous equations.
You must show all your working.[4]
6x+5y = 27
5x-3y = 44
x =
y =
(b) y is inversely proportional to (x+3)2.
When x=2, y=8.
Find y when x=7.
y = [3]
(c) Solve the inequality.[3]
\(3\left ( x-2 \right )< 7\left ( x+2 \right )\)
Answer/Explanation
Ans:
10(a) correctly equating one set of coefficients
correct method to eliminate one variable
x = 7
y = −3
10(b) 2
10(c) x > −5 final answer
Question
Bernie buys x packets of seeds and y plants for his garden.
He wants to buy more packets of seeds than plants.
The inequality x y 2 shows this information.
He also wants to buy
• less than 10 packets of seeds
• at least 2 plants.
(a) Write down two more inequalities in x or y to show this information.
(b) Each packet of seeds costs $1 and each plant costs $3.
The maximum amount Bernie can spend is $21.
Write down another inequality in x and y to show this information.
(c) The line x = y is drawn on the grid.
Draw three more lines to show your inequalities and shade the unwanted regions.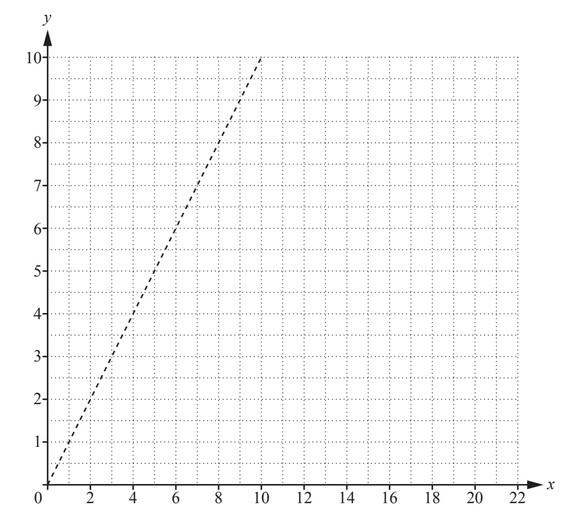
(d) Bernie buys 8 packets of seeds.
(i) Find the maximum number of plants he can buy.
(ii) Find the total cost of these packets of seeds and plants.
$ ………………………………………….
Answer/Explanation
Answer:
(a) x < 10 oe
\(y \leq 2\) oe
(b) \(x + 3y \leq 21\) oe
(c) ruled broken line x = 10
ruled line y = 2
ruled line from (0, 7) to (21, 0)
correct region indicated cao
(d) (i) 4
(ii) 20